There is a strong connection between convexity and inequalities. So, techniques from each concept applies to the other due to the strong correlation between them; specifically, in the past few years. In this attempt, we consider the Hermite-Hadamard inequality and related inequalities for the class of functions whose absolute value of the third derivative are quasi-convex functions. Finally, the applications of our findings for special functions and particular functions are pointed out.
1.
Introduction
Many research papers have studied the properties of convex functions that make this concept interesting in mathematical analysis [1,2,3,4]. In recent years, important generalizations have been made in the context of convexity: quasi-convex [5], pseudo-convex [6], invex and preinvex [7], strongly convex [8], approximately convex [9], MT-convex [10], (α,m)-convex [11], and strongly (s,m)-convex [12,13,14,15]. Here, we recall the notion of convexity: A function g:[β1,β2]⊂R→R is said to be convex if the following inequality holds
Now, we recall our basic definition, the so-called quasi-convex function.
Definition 1.1 ([16]). A function g:[β1,β2]→R is said quasi-convex on [β1,β2] if
for any x,y∈[β1,β2] and t∈[0,1].
It is important to note that, any convex function is a quasi-convex but the reverse is not true. In the following example we explain that fact.
Example 1.1 ([5]). The function h:[−2,2]→R, defined by
is not convex on [−2,2] but it is easy to see that the function is quasi-convex on [−2,2].
Notice that h is quasi-convex if and only if all the level sets of h are intervals (convex sets of the line).
The use of the convex function to study the integral inequalities have been deeply investigated, especially for the well-known inequality of Hermite-Hadamard type (HH-type inequality). The HH-type inequalities are one of the most important type inequalities and have a strong relationship to convex functions. In 1893 Hermite and Hadamard [17] found independently that for any convex function g:[β1,β2]→R, the inequality
holds.
In the field of mathematical analysis, many scholars have focused on defining new convexity and implementing of the problems based on their features. The features that make the results different from each other include lower and higher order derivative of the function. The differential equations with impulse perturbations lie in a special significant position in the theory of differential equations. Among them, integral inequality methods are the important tools to investigate the qualitative characteristics of solutions of different kinds of equations such as differential equations, difference equations, partial differential equations, and impulsive differential equations; see [18,19,20,21,22,23] for more details.
The HH-type inequality (1.3) has been applied to various convex functions like s-geometrically convex functions [24], GA-convex functions [25], MT-convex function [10], (α,m)-convex functions [26] and many other types can be found in [27]. Besides, the HH-type inequality (1.3) has been applied to a numerous type of convex functions in the sense of fractional calculus like F-convex functions [28], λψ-convex functions [29], MT-convex functions [30], (α,m)-convex functions [11], new class of convex functions [31] and many other types can be found in the literature. Meanwhile, it has been applied to other models of fractional calculus like standard Riemann-Liouville fractional operators [32,33], conformable fractional operators [34,35,36], generalized fractional operators [37], ψ-RL-fractional operators [38,39], Tempered fractional operators [40], and AB- and Prabhakar fractional operators [41].
In view of the above indices, we extend the work done in [42] to establish some modified HH-type inequalities for the 3-times differentiable quasi-convex functions.
2.
Main results
This section deals with our main results. Throughout this paper, we mean g∈L[β1,β2] that the function g is differential and continuous on [β1,β2].
Lemma 2.1. Suppose that g:J⊂R→R is a differentiable function such that β1,β2∈J with β1<β2. If g‴∈L[β1,β2], then we have
Proof. By applying integration by parts three times to get
Making use of change of the variable x=tβ1+β22+(1−t)β1 for t∈[0,1] and multiplying by (β2−β1)396 on both sides, we obtain
Analogously, we can deduce
Finally, by adding (2.3) and (2.4), we get the required identity (2.1).
Remark 2.1. Notice that f being quasi-convex is not equivalent to |f| being quasi-convex. For instance, g(x)=x2−1 is only quasi-convex (but not |g(x)|), whereas g(x)=1 if x∈[−1,1] and g(x)=−1 otherwise, is not quasi-convex, but |g(x)|=1 is quasi-convex.
Theorem 2.1. Suppose that g:J⊆[0,+∞)→R is a differentiable function such that g‴∈L[β1,β2], where β1,β2∈J with β1<β2. If |g‴| is quasi-convex function on [β1,β2], then we have
where K=max{|g‴(β1+β22)|,|g‴(β1)|}+max{|g‴(β1+β22)|,|g‴(β2)|}.
Proof. Making use of Lemma 2.1 and the quasi-convexity |g‴|, we have that
This rearranges to the desired result.
Example 2.1. To clarify the following expression occurs in Theorem 2.1
we consider the function g(x)=xx2+2 on the interval [β1,β2]=[0,1]. Then, we have
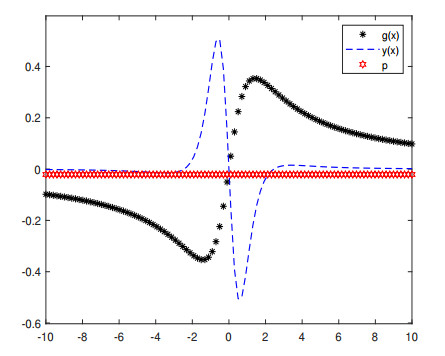
Figure 1 demonstrates the intersections and relationships between the functions g(x),y(x) and the point p geometrically.
Corollary 2.1. Let the assumptions of Theorem 2.1 be valid and let
Then,
(i) if |g‴| is increasing, then we have
(ii) if |g‴| is decreasing, then we have
(iii) if g‴(β1+β22)=0, then we have
(iv) if g‴(β1)=g‴(β2)=0, then we have
Theorem 2.2. Suppose that g:J⊆[0,+∞)→R is a differentiable function such that g‴∈L[β1,β2], where β1,β2∈J with β1<β2. If |g‴|q is quasi-convex function on [β1,β2] and q>1 with 1p+1q=1, then we have
where Kq=(max{|g‴(β1+β22)|q,|g‴(β1)|q})1q+(max{|g‴(β1+β22)|q,|g‴(β2)|q})1q.
Proof. Let p>1. Then from Lemma 2.1 and using the Hölder inequality, we can deduce
The quasi-convexity of |g‴|q on [β1,β2] implies that
and
Therefore, we obtain
where we used the identities
Thus, our proof is completely done.
Corollary 2.2. Let the assumptions of Theorem 2.2 be valid and let
Then,
(i) if |g‴| is increasing, then we have
(ii) if |g‴| is decreasing, then we have
(iii) if g‴(β1+β22)=0, then we have
(iv) if g‴(β1)=g‴(β2)=0, then we have
Theorem 2.3. Suppose that g:J⊆[0,+∞)→R is a differentiable function such that g‴∈L[β1,β2], where β1,β2∈J with β1<β2. If |g‴|q is quasi-convex function on [β1,β2] and q≥1 with 1p+1q=1, then we have
where Kq is as before.
Proof. From Lemma 2.1, properties of modulus, and power mean inequality, we have
Then, by using the quasi-convexity of |g‴|q on [β1,β2], we have
and
Therefore, we obtain
Hence, our proof is completely done.
Remark 2.2. If q=pp−1 (p>1), the constants of Theorem 2.2 are improved, since 1(3p+1)1p<1.
The following corollary improves the inequalities (2.7)–(2.10).
Corollary 2.3. Let the assumptions of Theorem 2.3 be valid and let
Then,
(i) If |g‴| is increasing, we obtain (2.7).
(ii) If |g‴| is decreasing, we obtain (2.8).
(iii) If g‴(β1+β22)=0, we obtain (2.9),
(iv) if g‴(β1)=g‴(β2)=0, we obtain (2.10).
3.
Applications
3.1. Applications for special means
Consider the special means of positive real numbers β1>0 and β2>0, define by:
● Arithmetic Mean:
● Logarithmic mean:
● Generalized log-mean:
Remark 3.1. Let 0<α≤1 and x>0. Then, we consider
For each x,y>0 and t∈[0,1], we see that (tx+(1−t)y)α≤tαxα+(1−t)αyα, then we see that g‴(x) is α-convex function on (0,+∞) and g(β1+β22)=Aα+3(β1,β2)(α+1)(α+2)(α+3). Furthermore, we have
Above we used the definition of α-convexity [11]: A function g:[0,r]→R,r>0 is said to be α-convex, if the following holds:
Proposition 3.1. Let 0<α≤1 andβ1,β2∈R+ with β1<β2, then we have
Proof. Since xα is quasi-convex for each x>0 and α∈(0,1) because every non-decreasing continuous function is also quasi-convex, so the assertion follows from inequality (2.5) with g(x)=xα+3(α+1)(α+2)(α+3).
Proposition 3.2. Let β1,β2∈R such that β1<β2 and [β1,β2]⊂(0,+∞), then we have
Proof. The assertion follows from inequality (2.11) with g(x)=1x, x∈[β1,β2].
Proposition 3.3. Let β1,β2∈R with 0<β1<β2 and n∈N, k≥5, then for all q≥1, we have
Proof. Let g(x)=xk, x∈[β1,β2], k∈N with k≥5, then we have
and it is easy to see that g‴ is an increasing and quasi-convex function. Then, by applying Corollary 2.2(ⅰ) above, we have
where
Then, by applying Corollary 2.1(9) to g above, we get
and this completes the proof.
3.2. Application for particular functions
Here, we consider two particular functions.
● First, we define g:R→R, by g(x)=ex.
Then, we have g‴(x)=ex and
By applying inequality (2.7) for above ||g‴||∞, we can deduce
Particularly for β1=0, it follows that
and for β2=1, it follows that
● Now, we define g:R+→R, by g(x)=1x.
Then, since |g‴(x)|=6x4 is quasi-convex in [β1,β2]⊂R+ and
By applying inequality (2.8) to the function g above, we get
In view of (3.2) and Proposition 2, we can deduce
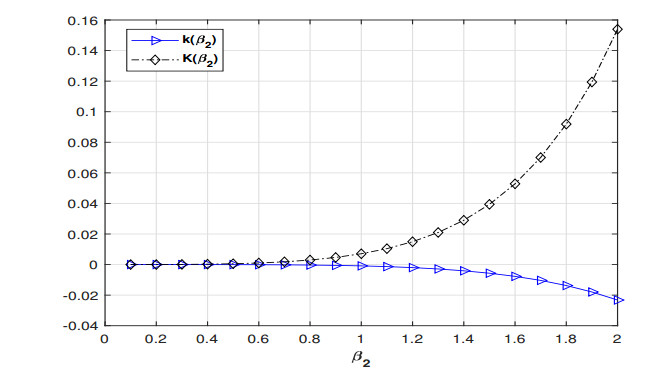
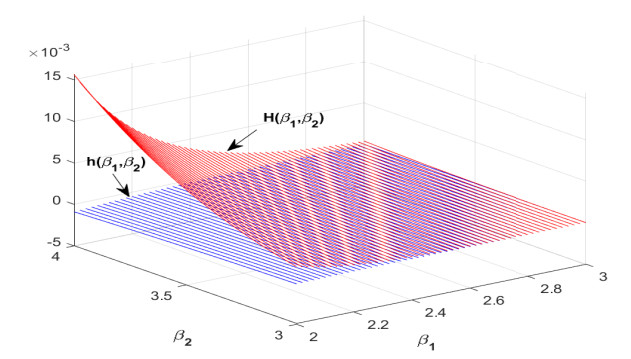
For further illustration on the inequalities (3.1) and (3.2), we present some plot examples. Figures 2 and 3 illustrate the inequalities (3.1) and (3.2), respectively.
Let
and
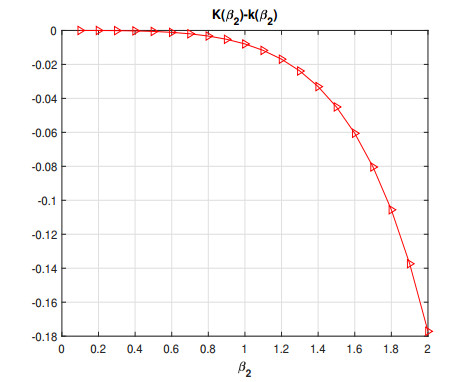
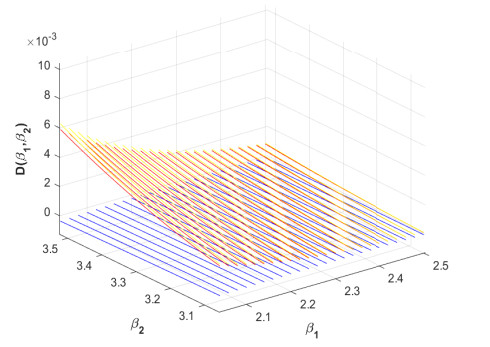
Furthermore, Figures 4 and 5 show K(β2)−k(β2) and D(β1,β2):=H(β1,β2)−h(β1,β2), receptively. From Figure 5, we can see that all values of D(β1,β2) are positive which confirms the validity of (3.2).
4.
Conclusions
In this paper we have established new Hermite–Hadamard inequality mainly motivated by Alomari et al in [42] for quasi-convex functions with g∈C3([β1,β2]) such that g‴∈L([β1,β2]) and we give some applications to some special means and for some particular functions. We hope that the ideas used in this paper may inspire interested readers to explore some new applications.
We believe that our results, this new understanding of Hermite-Hadamard integral inequalities for quasi-convex functions, will be vital information for the future studies of these models of integral inequality. One can obtain the similar results for other kind of convex functions
Acknowledgements
We would like to express our special thanks to the editor and referees. Also, the third author would like to thank Prince Sultan University for funding this work through research group Nonlinear Analysis Methods in Applied Mathematics (NAMAM) group number RG-DES-2017-01-17.
Conflict of interest
The authors declare no conflict of interest.









 DownLoad:
DownLoad: