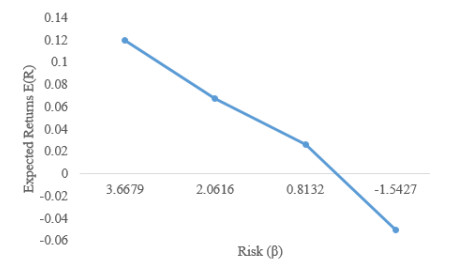
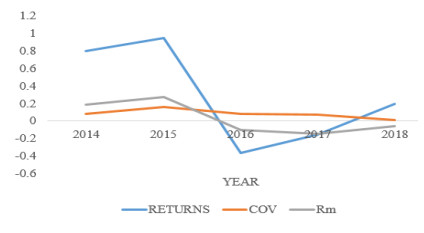
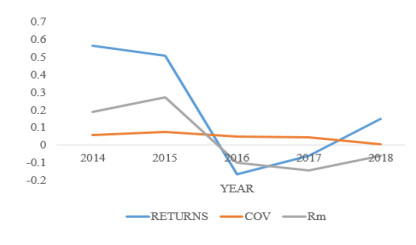
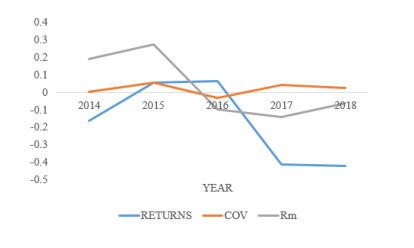
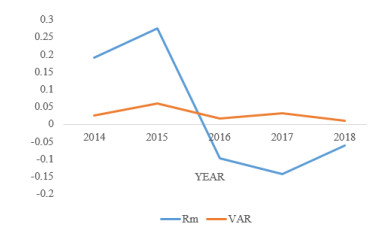
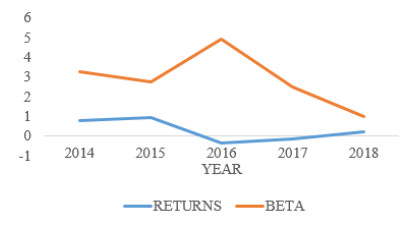
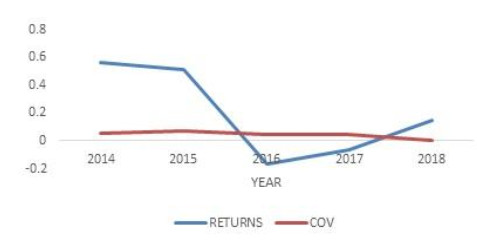
Capital asset pricing model (CAPM) is one of the widely used asset pricing models in modern securities theory. This mathematical model can help investors understand the relationship between expected returns and investment risk. To help energy commodity investors (especially Deutsche Bank) make the best decisions in investment management, this paper uses the CAPM and some statistical tools (variance, covariance and mean) to study risks on the expected return of investing in four common Deutsche Bank (DB) crude oil assets (DB crude oil double short, SZO-DB crude oil short order, OLO-DB crude oil short position, DBO-Invesco DB Petroleum Fund). The result reveals that DTO-DB Crude oil Double Short has the highest beta risk and highest expected return. And the higher the risk, the higher the expected return, and vice versa, that is, the risk is directly proportional to the expected return. In addition, the results also show that 73% of the investoros wealth can be spent on a risky asset in DTO-DB Crude oil Double Short, 67% in SZO-DB Crude oil Short, 16% in OLO-DB Crude oil Short. Since the expected returns of DBO-Invesco DB Crude oil fund has a negative risk with negative expected returns, the investment in DBO-Invesco DB Crude oil will result in having a loss from the investment.
1.
Introduction
Habitat fragmentation is usually observed in nature related with heterogeneity in the distribution of resources. For example, food, water, shelter sites, physical factors such as temperature, light, moisture, and any feature be able to affect the growth rate of the population of a given species [1]. These fragments, also known as patches, are not completely isolated because they are coupled by the motion of individuals in space. Therefore, mathematicians and ecologists apply diffusion models to explain many ecological problems [1,2,3,4,5,6,7]. One of the classical population diffusion model [7]:
where xi(t) stands for the density of patch i at time t; ri stands for the population growth rate of patch i; dij stands for the dispersal rate of the population from the i-th patch to j-th patch, i=1,2,i≠j.
The above diffusion processes are all based on the random movement of matter in space. However, many ecologists have found that there are many practical phenomena that cannot be explained by simple diffusion population models, such as, tripping and killing pests. In general, an important feature of many biological individuals is that they can perceive external signals and cues from a specific stimulus, especially vertebrates. Due to the attraction of some external signals, species may move in specific directions, which is called chemotaxis [8,9,10,11]. Colombo and Anteneodo proposed a model to consider the interplay between spatial dispersal and environment spatiotemporal fluctuations in meta-population dynamics [1]. Li and Guo studied a reaction-diffusion model with chemotaxis and nonlocal delay effect [9]. In [12,13], they showed that vertebrates have better sensory and differentiated nervous systems than invertebrates, which can transmit sensory information in the polluted environment to the region of brain where it can analysis and make corresponding processing, either bear the concentration of toxins in the habitat or escape from the area. Wei and Chen [12] proposed a single-speices population model with psychological effects in the polluted environment:
where ce(t) and c0(t) denote the concentration of toxicant in the environment and organism at time t respectively, ue(t) represents the input rate of external toxins to the habitat at time t, and, it is a continuous and bounded non-negative function. Coefficients r,r0,a,k,g,m,h,λ and α are positive constants, and their biological significance has been given in [12].
As we all know, with the influence of human economic activities, not only habitats of population are destroyed, but also the environment of habitats are polluted. The survival of those unprotected populations will be seriously threatened, even human beings, therefore, it is necessary to consider the effect of toxins in polluted patches on the population [14,15,16,17]. The "psychological effect" mentioned in [12,13] is also due to the response of biological individuals to the stimulation of environmental toxins in polluted environment, in other words, it is "chemotaxis". Considering the chemotaxis of biological individuals, the single-species population in heavily polluted patches will increase their diffusion to other nonpolluting or lightly polluted patches, while the populations of lightly polluted or nonpolluting patches will slow down their diffusion to heavily polluted patches under the influence of chemotaxis. In order to understand the effect of chemotaxis on population survival, we suppose that patch 1 is heavily polluted patch, and patch 2 is nonpolluting patch. On the basis of previous studies, we propose a single-species population diffusion model with chemotaxis in polluted environment:
where λi(0≤λi≤1) denotes the contact rate between the single-species population and the environment toxicant. The initial value satisfies x1(0)>0,x2(0)>0,co(0)≥0,ce(0)≥0.
However, in nature, the population will be more or less disturbed by various random factors, which usually composed of many tiny and independent random disturbances, such as temperature, weather and climate change. May [18] has pointed out that even the smallest environmental randomness resulted in a qualitatively different result from the deterministic one. In recent years, stochastic population models have received a lot of attention [19,20,21,22,23,24,25]. Zou and Fan studied a single-species stochastic linear diffusion system [23]. Zu and Jiang focused on the extinction, stochastic persistence and stationary distribution of a single-species stochastic model with directed diffusion [24]. Liu and Bai considered a stochastic logistic population with biased diffusion [25]. Studies of single-species stochastic population models with migrations between the nature preserve and natural environment had received increasing attention in recent works [26,27,28,29]. But, few studies discuss the single-species population diffusion model with chemotaxis in population environment.
In this paper, we assume that the white noise mainly affects the intrinsic growth rate ri of system (1.3), we thus model the single-species population diffusion system by replacing the intrinsic growth rate ri of system (1.3) by a stochastic process ri→ri+σidB(t)dt, i = 1, 2., where dB(t)dt denotes white noise, σ2i represents the density of white noise. We therefore derive a single-species stochastic diffusion system with chemotaxis in polluted environment as follows:
Remark 1.1. [17]. Since c0(t) and ce(t) denote the concentration of toxicant, thus, 0≤ce(t)≤1, 0≤c0(t)≤1, with this end in view, we need the following constraints f≤g+m, 0≤u(t)≤u<h.
Because the latter two equations in model (1.4) are linear, we only need to discuss the following subsystem here:
2.
Preliminaries
In this paper, unless otherwise noted, let (Ω,F,P) is a complete probability space with a filtration {F}t≥0 satisfying the usual conditions. (i.e., it is right continuous and contains all P-null sets)
For the convenience of later discussion, some notations, lemma and theorem are given in this section:
Definition 1. (1) population x(t) is said to be extinction if limt→+∞x(t)=0,a.s.;
(2) population x(t) is said to be strongly persistent if lim inft→+∞x(t)>0;
(3) population x(t) is said to be strongly persistent in the mean if lim inft→+∞⟨x(t)⟩>0.
Lemma 2.1. (see[22]) Suppose that x(t)∈C(Ω×[0,+∞),R+).
(1) If there are λ and positive constants λ0, T, such that lnx(t)≤λt−λ0∫t0x(s)ds+n∑i=1βidBi(t),t≥T,
where βi(1≤i≤n) is constant, then
(2) If there are positive constants λ, λ0 and T, such that lnx(t)≥λt−λ0∫t0x(s)ds+n∑i=1βidBi(t),t≥T.
Then lim inft→+∞t−1∫t0x(s)ds≥λλ0,a.s.
Theorem 2.2. (see[19]) Let M(t) be a continuous local martingale and M(0)=0, then
(1) If limt→+∞⟨M(t),M(t)⟩t=∞,a.s., ⇒ limt→+∞M(t)⟨M(t),M(t)⟩=0,a.s.
(2) If limt→+∞⟨M(t),M(t)⟩t<∞,a.s., ⇒ limt→+∞M(t)t=0,a.s.
Lemma 2.3. (see[22]) Stochastic population equation dx(t)=x(t)(r−ax(t))dt+σx(t)dB(t), where r, a and σ are positive constants.
(1) If r−0.5σ2>0, have limt→+∞⟨x(t)⟩=r−0.5σ2a,limt→+∞lnx(t)t=0,a.s.
(2) If r−0.5σ2<0, have limt→+∞x(t)=0,a.s.
Lemma 2.4. If limt→+∞ue(t)=¯ue, then
Proof. From the last two equations of model (1.4), for all ϵ>0, we can imply that
By standard comparison theorem obtains that
which implies that
Thus, it easily obtain that
3.
Survival analysis of deterministic population model (1.3)
AssumptionH1:~r1=r1−r0(c0)∗−d12(1+λ1(ce)∗1+α(c2e)∗)<0, ~r2=r2−d21(1−λ2(ce)∗1+α(c2e)∗)<0.
AssumptionH2:˜d=d21(1−λ2(ce)∗1+α(c2e)∗)d12(1+λ1c∗e1+α(c2e)∗)−˜r1˜r2<0.
AssumptionH3:^r1=r1−r0(c0)∗−d12(1+λ1(ce)∗1+α(c2e)∗)<0, ^r2=r2−d21(1−λ2(ce)∗1+α(c2e)∗)<0.
AssumptionH4:ˆd=d12(1+λ1(ce)∗1+α(c2e)∗)d21(1−λ2c∗e1+α(c2e)∗)−^r1^r2<0.
Theorem 3.1. Let x(t)=(x1(t),x2(t)) be the solution of the first two equations of (3) with the initial value x(0)∈R2+,
(1) Suppose Assumption H1 and H2 hold simultaneously, single-species x will be extinct.
(2) Suppose Assumption H3 or H4 are not true, single-species x is strongly persistent.
Proof. It follows from the first two equations of (3) that,
Comparison system
and
with zi(0)=yi(0)=xi(0)>0,i=1,2. By comparison theorem, we have zi(t)≤xi(t)≤yi(t),i=1,2.
(1) If Assumption H1 and H2 hold simultaneously, it is easy to see that the eigenvalue of system (6) at equilibrium point (0, 0) has negative real part and is quasi-monotone non-decreasing. Since xi(t)≥yi(t),i=1,2. have limt→+∞xi(t)=0,i=1,2.a.s.
(2) If Assumption H3 or H4 are not true, the proof is similar to [6]. we know that system (3.2) have positive equilibrium point z_ and zero equilibrium point. According to the conclusion and proof of Theorem 1 (see Allen[6]), system (7) is unstable at zero equilibrium, but stable at positive equilibrium point z_, then limt→+∞z(t)=z_. By virtue of, zi(t)≤xi(t),i=1,2., we have, lim inft→+∞x(t)≥z_, that is, population x is strongly persistent.
The proof is completes.
According to the Theorem 3.1's (1), if Assumption H1 and H2 simultaneously true, population x will die out. By ˜r1<0 and ˜r2<0, we get
by virtue of ˜d=d12d21(1+λ1c∗e1+α(c2e)∗)(1−λ2(ce)∗1+α(c2e)∗)−˜r1˜r2<0, we can obtain that
If (r1−r0(c0)∗)r2≥0, by virtue of (3.3) and (3.4), one can imply that (r1−r0(c0)∗)r2<0, it is contradiction with (r1−r0(c0)∗)r2≥0. Thus, (r1−r0(c0)∗)r2<0 is a necessary condition of Assumption H1 and H2 holding at the same time.
4.
Survival analysis of stochastic population model (1.4)
In order to analysis the long-time behaviors of single-species of system (1.5), first of all, we shall show that system (1.5) has unique global positive solution x(t)=(x1(t),x2(t)).
Lemma 4.1. For any given initial value x(0)∈R2+, there is a unique positive solution x(t) to system (1.5), and the solution will remain R2+ with probability 1.
Proof. Because the coefficients of system (1.5) is locally Lipschitz continuous for any given initial value x(0)∈R2+, there is a unique local solution x(t) in [0,τe), where τe is the explosion time(see [23]). In order to proof the solution is global, we only need to prove τe=+∞,a.s..
For each integer n>n0, defining the stopping time
obviously, τn is increasing when n→∞. Let τ∞=limn→+∞τn, hence, τ∞≤τe,a.s. Next, we just need to proof τ∞=+∞, if the conclusion is not true, there are T>0 and ϵ∈(0,1) such that P{τ∞≤T}>ϵ. Thus, there is a integer n1≥n0, such that P{τn≤T}≥ϵ,n≥n1.
Defining Lyapounov function V:R2+→R+, have
For x∈R2+, applying Itˆo's formula, we get
where
Obviously, there is a positive constant K such that LV(x)≤K.
Integrating both sides of inequality (4.1) from 0 to τn∧T and taking expectation yield
By the definition of τn, xi(τn∧T)=n or 1n for some i=1,2, hence,
It follows from (4.2) that
when n→∞, we have
which is a contradiction.
This completes the proof.
Lemma 4.2. Let x(t) be the solution of system (1.5) with the initial value x(0)∈R2+, for any θ>0, have
Proof. Defining function V(x)=ln(x1+θx2), applying Itˆo′s formula to V(x), we have
where r=max{(r1+θd12(1+λ1√α)),(r2+d21θ)} and ˆa=0.5min{a1,a2θ}. Thus
Integrating the two sides of the above inequality in the interval [0,t], we get
where M(t)=∫t0esσ1x1(s)dB1(s)+σ2θx2(s)dB2(s)x1(s)+θx2(s)ds.
The quadratic variation of M(t) is ⟨M(t),M(t)⟩=∫t0σ21x21(s)+σ22θ2x22(s)(x1(s)+θx2(s))2ds. According to the exponential martingale inequality, for all positive constants μ,ν and T0, we can obtain that
we choose μ=e−k, β=γeklnk, T0=k and γ>1,
since +∞∑k=1k−γ<∞, according to Borel-Cantalli Lemma, there exists Ω∈F and positive integer k1=k1(ω) satisfy P(Ω)=1, for all ω∈Ω, and k>k1, have
It follows from ln(x1+θx2)+r−ˆa(x1+θx2) that there is a positive constant K, such that ln(x1+θx2)+r−ˆa(x1+θx2)≤K. by (4.3) and (4.4), for all k>k1, we have
for k−1≤t≤k, we get
Let t→+∞, we can observe that lim supt→+∞lnlnxi(t)t≤lim supt→+∞lnln(x1(t)+θx2(t))t≤0,a.s.,i=1,2.
This completes the proof.
4.1. Extinction
Let (θ,ρ) be the solution of the following equations
where b>0 and d>0. By virtue of (4.5), it easily observe that θ=dρ−c, where ρ is the solution of equation
Because a and c are the solutions of equation ρ2−(a+c)ρ+ac=0, obviously, Eq (4.6) has two solutions, and there must be a solution
which is greater than c, thus θ>0.
Remark 4.3. We next come to analyze the following possible cases of the solution of Eq (4.6).
(a) If a and c are negative constants, when bd−ac<0, all solutions of Eq (4.6) are negative. However, when bd−ac≥0, there must be a nonnegative solution of Eq (4.6).
(b) If a or c aren't both negative, we can imply that there must be a positive solution of Eq (4.6).
Theorem 4.4. Let (x1(t),x2(t)) be the solution of system (1.5) with initial value (x1(0),x2(0))∈R2+. If
the single-species population x of system (1.5) will die out, that is, limt→+∞xi(t)=0,a.s.,i=1,2.
Proof. Let θ>0, it follows from (1.5) that
Then for all ϵ>0, there is a positive constant t1, for all t≥t1, it follows from (4.7) that
We can imply that there must be a
and θ=Du21+ϵρ−Ru2−ϵ>0, such that
Applying Itˆo′s formula to ln(x1+θx2), we have
By (4.9), we can obtain that
where N(t)=∫tt1σ1x1(s)dB1(s)+σ2θx2(s)dB2(s)(x1(s)+θx2(s)).
The quadratic variation of N(t), have
It follows from the Theorem 2.2, we can get that limt→+∞N(t)t−t1=0,a.s.
If (Ru1+Ru2)+√(Ru1−Ru2)2+4Du12Du21<σ2, let ϵ be sufficient small such that ρ<0.5σ2. Because limt→+∞N(t)t−t1=0,a.s., it follows from (4.10) that
which yields
This completes the proof of Theorem 4.4.
According to Theorem 4.4 and Lemma 2.4, we can obtain the following corollary.
Corollary 4.5. If limt→+∞ue(t)=¯ue, when the coefficients ¯R1+¯R2+√(¯R1−¯R2)2+4¯D12¯D21<σ2, the single-species population x will be extinct.
Remark 4.6. It follows from the proof of Theorem 4.4 and the results of Remark 4.3, if Ru1<0, Ru2<0 and Du12Du21−Ru1Ru2<0 hold, the single-species population will be extinct.
Remark 4.7. From Theorem 4.4, if ~r1<0,~r2<0 and d12d21(1+λ1c∗e1+α(c2e)∗)(1−λ2(ce)∗1+α(c2e)∗)−~r1~r2<0, it follows from the proof of the Theorem 4.4 and the results of the Remark 4.3(a), we find that the single-species population x of stochastic model (1.4) will die out, and it is also extinction in deterministic model (1.3). When Assumption H3 or H4 aren't true, the single-species x of deterministic model (1.3) is strongly persistent, but Theorem 4.4 shows that the single-species x of stochastic model will die out when white noises large enough, which means that the white noises in the environment will affect the sustainable survival of the species, especially the endangered species.
4.2. Persistence in the mean
Theorem 4.8. Let (x1(t),x2(t)) be the solution of system (1.5) with initial value (x1(0),x2(0))∈R2+, if
the single-species population x is strongly persistent in the mean.
Proof. Let ϵ>0 be large enough that
By
and (4.7), for all ϵ>0, there exists a positive constant t1, when t≥t1, we can obtain that
In view of the proof of Theorem 4.4, by virtue of (4.11), we can imply that there are positive constants θ and ρ such that
where θ and ρ satisfy the following equations:
Thus ρ=(Rl1+Rl2−2ϵ)+√(R1−R2)2+4(D12−ϵ)(D21−ϵ)2. By virtue of (4.12), we get
Integrating from t1 to t and dividing by t on above inequality, have
where N(t)=∫tt1σ1x1(s)dB1(s)+σ2θx2(s)dB2(s)x1(s)+θx2(s).
Taking the inferior limit on both sides of (4.13), we obtain that
Because limt→+∞N1(t)t=0,limt→+∞ln(ϕ1(0)+θϕ2(0))t=0,a.s. and ρ−0.5ˇσ2>0, by virtue of (4.14) and Lemma 2.3, we get lim inft→+∞⟨x1(t)+θx2(t)⟩≥ρ−0.5ˇσ2max{a1,a2θ}.
The proof of Theorem 4.8 is completes.
It follows from Theorem 4.8 and Lemma 2.4, we can get the following corollary.
Corollary 4.9. If limt→+∞ue(t)=¯ue, when the coefficients ¯R1+¯R2+√(¯R1−¯R2)2+4¯D12¯D21>˘σ2, the single-species population x is strongly persistent in the mean.
We next discuss the persistence in the mean of the population of each patch.
Theorem 4.10. Let (x1(t),x2(t)) be the solution of system (1.5) with initial value (x1(0),x2(0))∈R2+, if
the population xi in the patch i is strongly persistent in the mean, and
Proof. It follows from (1.5) that
Integrating both sides of above inequalities (4.15) and (4.16) from 0 to t,
For sufficiently small ϵ>0, such that ⟨Ri(t)⟩l−ϵ>0,i=1,2. It follows from (4.17) and (4.18) that
by virtue of Lemma 2.1, (4.19), (4.20) and the arbitrariness of ϵ, we can obtain that
The proof of Theorem 4.10 is completes.
5.
Examples and numerical simulations
In this section, we will show the numerical simulation results to illustrate the accuracy of analytical results in above section by using the famous Milstein's method [30]. It is very hard to choose parameters of the model from realistic estimation, which needs to apply many methods of statistical, therefore, we will only use some hypothetical parameters to simulate the theoretical effects in this section.
Example1. In deterministic system (1.3), we choose the parameters as:
r1=0.2,r2=0.2,r0=0.8,a1=0.5,a2=0.6,g=0.3,m=0.2,h=0.5,f=0.4,d12=0.5,d21=0.7,ue=0.4, with initial value (x1(0),x2(0),c0(0),ce(0))=(0.5,0.5,0.5,0.4).
In order to simulate the influence of chemotaxis on the survival of single-species, we change the values of λ1,λ2, and α. We firstly adopt λ1=0.5, λ2=0.2,α=1.5, by simple calculation, we know that it satisfy Assumption H1 and H2, by virtue of the Theorem 4.4, one can see that the single-species population x will die out, see Figure 1(a). If λ1=0.5, λ2=0.5,α=0.1, by computing, Assumption H4 is not true, by virtue of the Theorem 4.4's (2), we can observe that the single-species x is strongly persistent, see Figure 1(b).
Example2. In stochastic system (1.4), Chooses the parameters as:
r1=0.2,r2=0.3,r0=0.8,a1=0.5,a2=0.6,g=0.3,m=0.12,h=0.5, f=0.4,d12=0.3, d21=0.4,ue=0.4−0.1e−0.2t, λ1=0.6,λ2=0.4,α=1, with initial value (x1(0),x2(0),c0(0),ce(0))= (0.5,0.5,0.1,0.3).
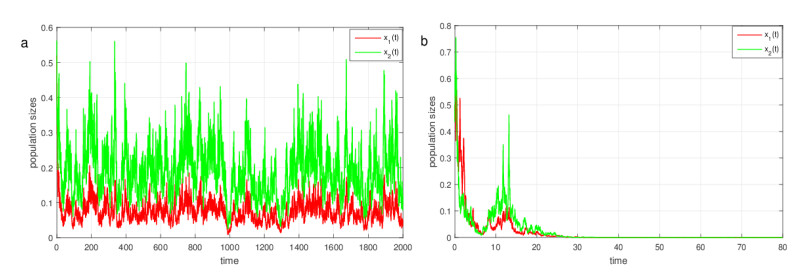
We next focus on the effect of the intensity of white noises on the survival of population x. we adopt σ1=0.2, σ2=0.2, computing shows that ¯R1+¯R2+√(¯R1−¯R2)2+4¯D12¯D21−ˇσ2=0.2298−0.04=0.1898>0, it follows from the Corollary 4.9 that the population x is strongly persistent in the mean, see Figure 2(a). Suppose σ1=0.7, σ2=0.8, and other parameters are the same as Figure 2(a), by computing, one can know that ¯R1+¯R2+√(¯R1−¯R2)2+4¯D12¯D21−ˇσ2=0.2298−0.2775=−0.0477<0, according to Corollary 4.9, one can find that the population x will die out (see Figure 2(b)). Therefore, from Figure 2, we can observe that the single-species x will be extinct when the densities of white noises larger enough.
Example3. In stochastic system (1.4), we choose the parameters as:
r1=0.2,r2=0.3,r0=0.8,a1=0.5,a2=0.6,g=0.3, m=0.2,h=0.5,f=0.4,ue=0.4−0.1e−0.2t,σ1=0.4, σ2=0.4, with initial value (x1(0),x2(0), c0(0),ce(0))=(0.5,0.5,0.1,0.3).
Case a: Suppose that d12=0,d21=0, the population x live in two independent patches. Simple calculation shows that r1−r0¯⟨c0(t)⟩<0.5σ21 and r2>0.5σ22. According to the Remark 3 in [22] and Lemma 2.3, we can get that the population x1 goes to extinction, and the population x2 is strongly persistent in the mean, see Figure 3(a).
Case b: If d12=0.3,d21=0.5,λ1=0,λ2=0, thus, system (1.4) is a single-species stochastic diffusion system. By computing, ¯R1+¯R2+√(¯R1−¯R2)2+4¯D12¯D21−σ2=0.0654−0.08=−0.0146<0, by Theorem 4.4, population x will die out (see Figure 3(b)).
Case c: If d12=0.3,d21=0.2,λ1=0,λ2=0, by computing, we have ¯R1+¯R2+√(¯R1−¯R2)2+4¯D12¯D21−ˇσ2=0.3523−0.16=0.1923>0, by Theorem 4.8, we know that the population x is strongly persistent in the mean (see Figure 3(c)).
Case d: If d12=0.3,d21=0.5,λ1=0.3,λ2=0.4,α=0.2, by simple computing shows that ¯R1+¯R2+√(¯R1−¯R2)2+4¯D12¯D21−ˇσ2=0.2161−0.16=0.0561>0, by Theorem 4.8, the population x is strongly persistent in the mean (see Figure 3(d)).
Figure 3 shows that the properties of chemotaxis have an influence on persistence in the mean and extinction of the population.
6.
Conclusion
It is a pretty active topic to consider spatial information affects population dynamics, when the habitat of species is polluted, the species will be stimulated by the toxins in the habitat and increase diffusion to other patch. Thus, single-species population diffusion models with chemotaxis in polluted environment are proposed and studied. For the deterministic model, sufficient conditions for persistent and extinction of population are obtain. And then, considering the influence of environmental noise, a single-species population diffusion model with chemotaxis in polluted environment is proposed. Firstly, we discussed that the model (1.4) has unique global positive solution. Secondly, we investigated the persistence in the mean and extinction of system (1.4), if Ru1+Ru2+√(Ru1−Ru2)2+4Du12Du21<σ2, the single-species population will extinction; if Rl1+Rl2+√(Rl1−Rl2)2+4Dl12Dl21>ˇσ2, the single-species population is strongly persistent in the mean. Finally, numerical simulations are used to confirm the efficiency of the main results.
Figure 2(a) and (b) show that the single-species x will die out when the densities of white noises large enough, therefore, it is significance to consider the effect of stochastic perturbation.
If we set d12=d21=0, that is to say, the single-species population live in two independent environments, respectively. Literature [22] shows that, when r1−r0¯ce<0.5σ21, the population x1 will tend to extinct, when r1−r0¯ce>0.5σ21, the population x1 is persistent in the mean, see Figure 3(a). However, by virtue of Theorem 4.4 and Theorem 4.8, we can obtain that population diffusion would affect the survival of the population x, see Figure 3(a) and (c).
Acknowledgments
This paper is supported by the Youth Science and technology talent growth project of Guizhou Provincial Department of Education(KY[2018]341, KY[2015]456), the Project for Innovative Research Groups of Guizhou Province of China (KY[2016]051), China Postdoctoral Science Foundation funded project(2017M623074), Science and Technology Foundatiaon of Guizhou Province of China (Project No. LKT[2012]23, [2018]1162).
Conflict of interest
The authors declare that they have no competing interests.










 DownLoad:
DownLoad: