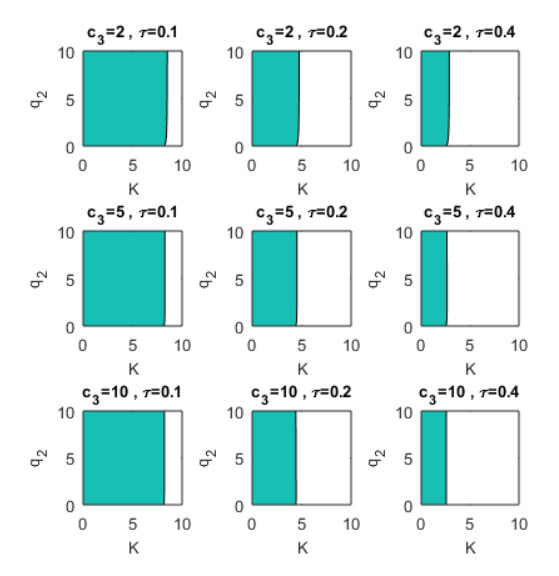
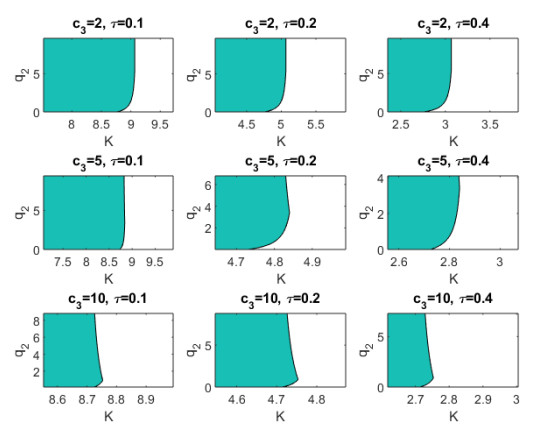
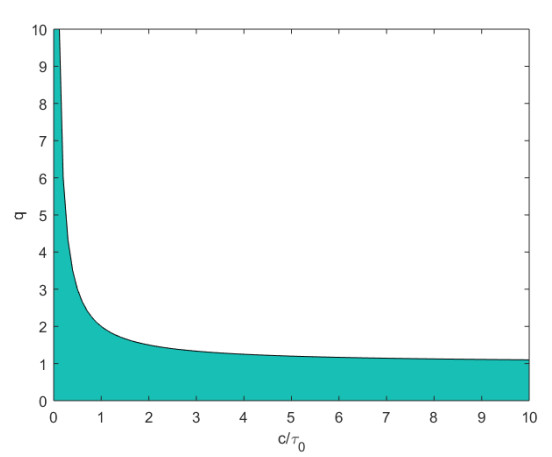
Recently, the notion of cryptocurrencies has come to the fore of public interest. These assets that exist only in electronic form, with no underlying value, offer the owners some protection from tracking or seizure by government or creditors. We model these assets from the perspective of asset flow equations developed by Caginalp and Balenovich, and investigate their stability under various parameters, as classical finance methodology is inapplicable. By utilizing the concept of liquidity price and analyzing stability of the resulting system of ordinary differential equations, we obtain conditions under which the system is linearly stable. We find that trend-based motivations and additional liquidity arising from an uptrend are destabilizing forces, while anchoring through value assumed to be fairly recent price history tends to be stabilizing.
1.
Introduction
Proteolytic enzymes, also called proteases, catalyze degradation of proteins and peptides by hydrolytic cleavage of peptide bonds [1]. Being essential for cell growth and differentiation, the proteolytic enzymes are ubiquitous in biological systems [2]. Microorganisms produce a vast diversity of intracellular and extracellular proteases. While the intracellular proteases play essential functions in cellular biochemistry, physiology, and regulatory aspects, the extracellular proteases provide carbon and nitrogen sources to cells by degrading extracellular proteins into small peptides and amino acids that can be transported into the cells [3]. Aside from their importance from biological point of view, the proteolytic activity is sought in numerous industrial processes, for example, in the detergent, leather, fabric and food industries, in pharmacology and drug manufacture, waste management, animal feed preparations etc. [4],[5]. Furthermore, proteases are commonly used as basic research tools in many biochemical investigations. For example, in protein identification, unknown proteins are subjected to trypsin digestion into small peptides for their subsequent analysis by mass spectrometry [6]. Other important applications in research include peptide synthesis, peptide sequencing, digestion of unwanted proteins in purified samples as in the DNA and RNA purifications, Klenow fragment production and so on [7]–[10]. With the total annual sales of about 1.5–1.8 billion USD, proteases, therefore, account for about 60% of the global enzyme sales constituting the largest product-segment of industrial enzymes [11]. Although the proteolytic enzymes can be obtained from many of the organisms, those derived from microbes especially bacteria are preferred for the large-scale production since bacteria are the easiest, cheapest and fastest to grow in a relatively small and simple set-up and are also suitable to genetic manipulation for increased production. Microbial proteases were also found more active and stable at extreme conditions than those of the plant or animal origin [12]. Therefore, the microbial enzymes can be obtained in abundant quantities on a regular basis and with a uniform quality [13]. Hence, many commercially important enzymes including proteases are generally obtained from a variety of bacterial species.
Recently, use of the protease producing bacteria is gaining increasing acceptance in aquaculture industry, world's fastest growing food production sector [14]. The proteolytic bacteria if included in aquaculture may serve multiple purposes such as (1) improved digestion of protein-rich substances present in the host's natural diet and in commercial feed resulting in an increased growth of the host [15]; (2) enhancement of nonspecific immune response in the host [16]; (3) reduction of organic pollutants produced in aquaculture from the undigested feed [17] etc. Besides, as compared to exogenous proteases, use of the protease producing microbes are more ecofriendly and easy in the application in aquaculture [18].
Nile tilapia is the third most important aquaculture species by volume having an enormous economic value [19]. For its high popularity among consumers and its easy and inexpensive method of farming, tilapia has become the most widely cultivated fish worldwide [20],[21]. The fish has a versatile eating habit and consumes phytoplankton, zooplanktons, macrophytes, insects, detritus, nematodes etc. in its diet [22]. Being a herbivorous-omnivorous fish without a true stomach, and with phytoplankton and plant debris comprising a major portion of its diet, Nile tilapia generally lacks pepsin and the role of pepsin is taken over by alkaline proteases which are more active in an alkaline environment [23]. Supplementing its feed with bacteria secreting extracellular proteases, therefore, appears highly beneficial to its cultivation.
To address the increasing demand of protease producing bacteria in industry, research and aquaculture, we focused on obtaining proteolytic strains from the gastrointestinal tract (GIT) of Nile tilapia. Fish GIT has been recognized as an excellent source of bacteria producing extracellular hydrolytic enzymes [15], and there is also a general consensus that the bacteria to be included in the animal feed should be isolated from GIT of the animals where they will be applied [18]. Consequently, we have isolated cultivable GI bacteria from Nile tilapia and screened them for protease production. The positive isolates were all identified and extensively characterized based on their genetic and biochemical properties and sugar fermentation abilities. Moreover, their substrate preferences as well as depolymerization capacities on various protein substrates were also studied.
2.
Materials and methods
2.1. Preparation of intestinal sample
For isolation of bacteria, intestinal sample was prepared from two healthy fish of 21.5 and 17.1 cm in length and 193.8 g and 170.2 g in weight respectively, purchased from a local market near Chittagong University campus, Bangladesh. Entire digestive tract of each fish was removed by aseptic surgery and its external surface was thoroughly washed with autoclaved distilled water and then sterilized using 70% v/v ethanol. Internal contents of the digestive tract were squeezed out and collected in a beaker. Inside of the digestive tract was then rinsed well with sterile water which was also added to the internal contents.
2.2. Isolation of bacteria
Bacteria present in the intestinal sample were isolated as previously described with minor modifications [15]. Briefly, 100 µL of the intestinal sample and its 10-fold serial dilutions (100 through 10−6) were spread on nutrient agar (NA; 5 g/L peptone, 3 g/L yeast extract, 5 g/L NaCl, 18 g/L agar; pH 7) and Luria-Bertani (LB) agar (10 g/L tryptone, 5 g/L yeast extract, 10 g/L NaCl, 18 g/L agar; pH 7) [24] plates and incubated at 30 °C for 24 to 48 h. All morphologically distinct colonies were selected and streaked on fresh NA and LB agar plates to obtain pure cultures [25].
2.3. Preparation of stock culture
Cells from the colony of pure culture was inoculated to nutrient and LB broth and incubated at 37 °C. After 24 h of growth, 500 µL of the culture was transferred to a cryo-vial, sterile glycerol was added to the final concentration of 20% v/v and preserved at −80 °C for further analysis.
2.4. Culture conditions
The isolates were routinely maintained in LB media at 30 °C, unless otherwise noted. Each isolate was revived from its glycerol stock by transferring cells to 2 to 5 mL LB broth by a sterile loop and grown overnight in an orbital shaker at 180 rpm at 30 °C. 1% v/v of this activated overnight culture was transferred to 10 mL fresh broth, incubated at 30 °C for 24 h and used for subsequent analysis.
2.5. Screening for proteolytic activity
To detect presence of extracellular proteolytic activity, 10 µl of a 0.8 OD600 culture of each isolate was spot-inoculated on the surface of skim milk agar media (5 g/L peptone, 2.5 g/L yeast extract, 1 g/L dextrose, 28 g/L skim milk powder, 18 g/L agar; pH 7) as well as NA and LB media each supplemented with 1% (w/v) skim milk powder and incubated at 30 °C for 24 to 48 h. Isolates that showed zones of clear halo surrounding the colonies were considered positive for protease production.
2.6. Morphological, cultural and biochemical characterization
Determination of morphological, cultural and biochemical properties of the isolates and their fermentation of various carbohydrates were carried out by methods described previously [25],[26].
2.7. 16S rRNA gene amplification and sequencing
16S rRNA gene of each isolate was amplified from its genomic DNA using GoTaq G2 Hot Start Master Mix (Promega) and the purified PCR products were sequenced using BigDye Terminator v3.1 Cycle Sequencing Kit according to a previous report [15].
2.8. Sequence deposition
The 16S rRNA genes sequenced in the present study have been deposited in the GenBank database under the accession numbers OK287066 to OK287072.
2.9. Taxonomic analysis
Taxonomic annotation of the proteolytic isolates was carried out by analysis of their 16S rRNA gene sequences with nucleotide BLAST of NCBI [27], RDP classifier and seqmatch [28] and Silva ACT: Alignment, Classification and Tree Service [29]. All parameters were set to default values with the only exceptions made in BLAST searches where the ‘Max target sequences’ was set to 1000. Phylotypes in the BLAST searches were determined by considering the query coverage, percent identity, maximum score, total score, and the total number of hits obtained for the query sequence against a particular genus or species. Organisms with an ambiguous taxonomic description such as enrichment culture clones, uncultured bacteria or unclassified bacteria were not taken into consideration [30]. NCBI taxonomy browser was followed to obtained taxonomic hierarchy of the isolates [31].
2.10. Phylogenetic analysis
Phylogenetic analysis of the isolates was performed essentially as previously described [32]. 16S rRNA gene sequence of the isolates, and 700 bp of their nearest type strains, and the top hit strains in BLAST results were aligned by Muscle [33] algorithm in Molecular Evolutionary Genetics Analysis (MEGA) software, version X [34]. The closest type strain for each isolate was found by using EzBioCloud's 16S-based ID [35], and their sequences were collected from the EzBioCloud database having the accession numbers CP001628, LASD01000006, FLYB01000015, JJMH01000057, HQ888847, BAMA01000316, LDJN01000038. Two additional strains used in the alignment for each isolate were selected from the top hits in BLAST search results and their sequences were obtained from GenBank database with the accession numbers MW198159.1, MT509874.1, MT509997.1, MK033338.1, MN420979.1, MH341969.1, MT533939.1, MT033093.1, MK571729.1, MK640708.1, KY913809.1, EU307934.1, MT555731.1, MT649753.1. A phylogenetic tree of the aligned sequences was built by maximum likelihood (ML) method [36] using Tamura-Nei model [37] with 1000 bootstrap replications in MEGA as described in [30].
2.11. Determination of substrate specificity
Ability of the proteolytic isolates to hydrolyze casein, gelatin and bovine serum albumin (BSA) was examined based on the formation of clear halos around colonies streaked on NA and LB media supplemented with 1% (w/v) of each substrate as described above.
2.12. Estimation of relative activity
To determine relative proteolytic activity, the isolates were grown on media containing 1% (w/v) of casein, gelatin or BSA at 30 °C for 48 h. The diameter of the zone of clearance and that of the colonies were measured. Relative activity (RA) was then calculated using the formula, RA = (colony diameter + halo zone diameter)/colony diameter [15].
2.13. Statistical analysis
All experiments were performed at least three times separately, averaged and the standard deviation was generated. The data were presented as the mean ± standard deviation displayed as error bars.
3.
Results
3.1. Proteolytic activity of the gut associated bacteria
In this study, we aim to isolate and characterize proteolytic strains in the gut flora of nilotica. To this end, 26 of its gut associated culture-dependent strains were isolated and screened for their ability to produce extracellular proteases on skim milk agar plates. Only 7 of the isolates (designated as TGB1 to TGB7) showed proteolytic activity as indicated by the formation of clear halos on media due to the depolymerization of casein in skim milk (Figure S1). To further evaluate their proteolytic aptitude, enzyme activity was assessed on three different protein substrates including casein, gelatin and BSA. All the seven isolates were found capable of degrading the three substrates which indicate relatively broad specificity of their secreted proteases.
3.2. Taxonomic and phylogenetic characteristics
Molecular taxonomy of the protease producing strains was determined by homology and phylogeny analysis of their 16S rRNA gene sequences to those in various databases. The sequences were subjected to a battery of 16S rRNA gene based methods for their identification. Results of the sequence analysis and subsequent phylotype assignments are presented in Table 1. Nucleotide blast of the sequences against those in GenBank and EzBioCloud databases showed a high sequence-similarity, with the percent identities higher than 99% to the respective sequences of Priestia, Citrobacter, Pseudomonas, Stenotrophomonas, Burkholderia, Providencia and Micrococcus (Table 1). The taxonomic assignment was also supported by other classification platforms such as RDP classifier, EzBioCloud 16S-based ID and Silva ACT (Table 1) confirming the taxonomic annotations to at least genus level. Phylotypes of the isolates each belonging to a separate genus indicates a very high diversity among the isolates without a single genus found predominant over the others. Considering their phylotypes along the taxonomic hierarchy, it was observed that the isolates belong to the phyla Firmicutes, Proteobacteria and Actinobacteria, with Proteobacteria being highly dominant (~72%).
3.3. Morphological, cellular and biochemical properties
Morphological, cultural and cellular characteristics of the proteolytic isolates and their biochemical properties are summarized in Tables 2, 3 and 4. Cell morphology showed that most of the isolates were Gram negative rods although TGB1 and TGB7 appeared Gram positive and TGB7 was found to be a coccus (Table 3). All isolates could produce catalase and most of them also produced H2S. The isolates were found negative in the MR-VP tests. Extracellular amylase activity was detected in three of the isolates including the Priestia (TGB1), Citrobacter (TGB2) and Stenotrophomonas (TGB4) strains. Fermentation tests with carbohydrates including various mono, di, tri and polysaccharides showed that the isolates had a rather limited metabolic capacity. Glucose was the sugar fermented by most (5/7) isolates. A maximum of five sugars could be fermented by the Burkholderia (TGB5) isolate. Overall, the cultural and biochemical properties of the isolates largely complied to their phylogenetic affiliations as described in the Bergey's manual of systematic bacteriology [38].
3.4. Protease producing capacity and substrate preferences
To evaluate protease producing capacity of the isolates on different substrates, a general estimate of their protease production was performed based on diffusion of the secreted proteases across culture medium and presented as relative activity (RA) [18] in Figure 2. Three different isolates, Pseudomonas (TGB3), Stenotrophomonas (TGB4) and Micrococcus (TGB7), were found producing the maximum amount of protease on the casein, gelatin and albumin media respectively. A relatively higher production on casein media was also exhibited by the Providencia (TGB6) and Micrococcus (TGB7) isolates, and on gelatin media by the Pseudomonas (TGB3), Burkholderia (TGB5) and Micrococcus (TGB7) isolates. The Micrococcus (TGB7) strain, therefore, appeared to be the only isolate efficient in degrading any of the three substrates. In general, most of the isolates showed substrate degrading capacity in the order of gelatin > casein > BSA; exceptions were the Pseudomonas (TGB3) and Providencia (TGB6) isolates in which the order was casein > gelatin > BSA. Such a pattern suggests that protease released by the bacteria might have relatively higher preferences for casein and gelatin over albumin.
4.
Discussion
We carried out this study to obtain proteolytic bacteria from the GIT of Nile tilapia since bacteria producing extracellular proteases are demonstrated to have the potential to be used as probiotic agents in fish feed; moreover they also comprise a valuable source of the enzymes for research and industrial use. We have discussed importance of the protease producing bacteria in research, aquaculture and industries in the introduction section. The beneficial gastrointestinal flora has been recognized in recent research as the most suitable candidates intended for probiotic use [39]. Accordingly, we have isolated and studied gut bacteria of tilapia and detected proteolytic activity in about 27% of the isolates. The fact that the major fraction (73%) of the isolates lacked protease producing ability is not unusual considering that Nile tilapia has a herbivorous-omnivorous feeding habit. In our previous research on microbial hydrolytic enzymes, we found proteolytic activity in 50% of the intestinal bacteria in Bombay duck which, however, is a carnivore [15]. The diet of a carnivore is supposed to be rich in protein substances and largely devoid of plant based materials. As a result the proteolytic strains are expected to be dominant among the GI flora of a carnivorous fish. Consistent with this perception, Bairagi et al. reported relatively high densities of cellulolytic and amylolytic strains in tilapia although proteolytic isolates were also found in considerable numbers [40]. Similarly, Kar and Ghosh found higher populations of proteolytic bacteria in the carnivorous fish Channa punctatus than that in the herbivore Labeo rohita [41]. Although all these previous studies including ours arrived at the same conclusion suggesting it to be a general phenomenon, to fully confirm if it is indeed the case for Nile tilapia to have relatively lower proportion of proteolytic strains, an extensive study should be performed with large number of samples analyzed individually by both culture-dependent and metagenomic methods. But the primary objective of this work being obtaining proteolytic strains for downstream applications, it was outside of the scope.
The protease producing isolates of the present study were all identified genetically from their 16S rRNA gene analysis which was further supported from their morphological and biochemical properties. The isolates appeared taxonomically diverse at the genus level each belonging to a separate phylotype. Few of the similar phylotypes have been previously documented in the GIT of Nile tilapia. For example, species of Bacillus (B. megaterium; reclassified as Priestia megaterium), Citrobacter, and Burkholderia were commonly isolated from Nile tilapia [39],[42]–[45], and therefore seems to be autochthonous to this fish. Moreover, these three species which were recovered from tilapia intestine had also been reported to possess extracellular protease activity and other beneficial properties, and are, therefore, considered as probiotic candidates for Nile tilapia [39],[46],[47]. Although not frequently, but the other four genera identified in our analysis, Pseudomonas, Stenotrophomonas, Micrococcus and Providencia, have also been described among the intestinal bacteria of Nile tilapia [44],[48]–[50]. Whatever the source of their isolation is, species of all the seven genera were reported producing extracellular protease enzymes [51]–[58]. At the phylum level, Proteobacteria were found dominant over the other two phyla, Firmicutes and Actinobacteria in our study. Species of Proteobacteria have also been described among the most common gut microbiota of other freshwater fish such as common carp (Cyprinus carpio), grass carp (Ctenopharyngodon idella), goldfish (Carassius auratus), bluegill (Lepomis macrochirus), largemouth bass (Micropterus salmoides) etc. [59]–[61], and also in marine fish such as shorthorn sculpin (Myoxocephalus scorpius), lumpfish (Cyclopterus lumpus) arctic flounder (Liopsetta glacialis), Atlantic salmon (Salmo salar L.), cod (Gadus morhua), herring (Clupea pallasii) etc. [62],[63]. Generally, all the three phyla i.e. Proteobacteria, Firmicutes and Actinobacteria identified in our study have been commonly reported among the gut flora of Nile tilapia. For example, similar to our findings, Wu et al. also identified species which belonged only to the above three phyla where species of Firmicutes were found more dominant in the gut of Nile tilapia fed with woody forages [44]. In a culture-independent study using metagenomic approach Bereded et al. reported that the gut microbiota of Nile tilapia were dominated by two more phyla Cyanobacteria, Fusobacteria in addition to the above three [64].
The gut flora of Nile tilapia had also been demonstrated being influenced by the environment and diet. Even the dietary supplementation of exogenous proteases was found to have a significant influence on the gut bacteria. Recently, Hassaan et al. showed that the gut microbiota of Nile tilapia could be qualitatively improved by the addition of probiotics and/or exogenous protease in its diet [65]. They reported that inclusion of B. pumilus and exogenous protease in the diet inhibited nitrogenous hydrocarbon degrading bacteria which was otherwise present in its gut when fed with the control diet. This suggests that the probiotic and protease supplements might be sufficient for the complete digestion of dietary proteins and peptides present in the feed. They also showed that the probiotic diet without the exogenous protease could also inhibit the pathogenic bacterium Citrobacter koseri. Findings of Wang et al. also indicated that probiotic microbes could improve the gut microflora of Nile tilapia [66]. They found that addition of B. cereus to the tank water resulted in the stimulation of potentially beneficial bacteria including Acetobacterium and Bacillus spp. In another study on the effects of dietary probiotic supplementation, Xia et al. showed that feed supplemented with two probiotic species, B. cereus and B. subtilis, resulted in a significant improvement of autochthonous bacterial communities in the gut of the juvenile tilapia and also had a stimulatory effect on a variety of potential probiotics after 6 weeks of feeding [67]. Zeng et al. studied the effect of various woody-forage diets on Nile tilapia and observed a positive impact of 30% Moringa oleifera Lam on its growth, feed utilization as well as microbiota composition [44]. The gut microbiota of Nile tilapia can be affected by the rearing environment as well. The optimum temperature of water for rearing Nile tilapia is 27 to 32 °C and the fish cannot survive in temperatures below 8 °C. Previously, a strong correlation was revealed between the bacterial communities of the rearing water and those in the gut [68]. Moreover, seasonal fluctuation of water temperature was also found to change the composition of gut microbiota in Nile tilapia. Bereded et al. in a recent study demonstrated modifications of both the diversity and composition of gut bacteria with seasonal and spatial variation [69].
All the isolates of our study showed ability to degrade three different proteins including casein, gelatin and albumin with different degrees of degradation efficiencies and substrate preferences. However, albumin turned out to be relatively less preferred. Most isolates showed higher affinity for gelatin followed by casein and albumin as also previously reported, for example, in proteases from a Photobacterium sp. and a Brevibacillus brevis isolate [70],[71]. Three of our isolates, on the other hand, showed highest preference for casein which has been commonly observed in previous studies as well [72]–[76].
In summary, we have isolated and identified protease producing bacteria in the gut of Nile tilapia. We revealed morphological, cellular and biochemical properties of the proteolytic isolates and showed that their secreted proteases could hydrolyze casein, gelatin and albumin with different depolymerization capacities. Further investigations on ability of the proteases to digest proteins in aquaculture feed, elucidation of their structural and catalytic properties for industrial exploitations, and occurrence of additional beneficial properties in the proteolytic isolates, are needed.









 DownLoad:
DownLoad: