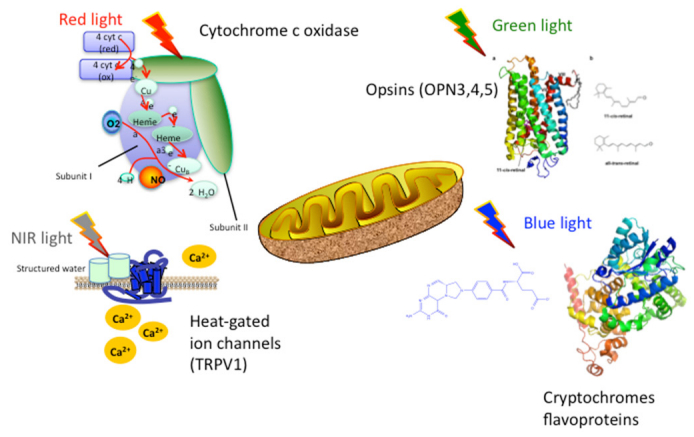
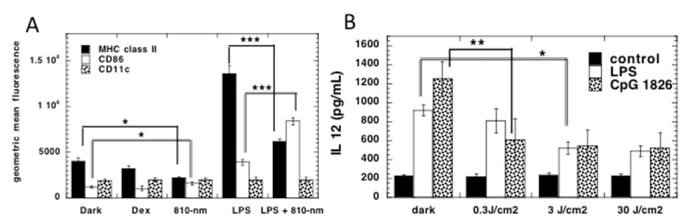
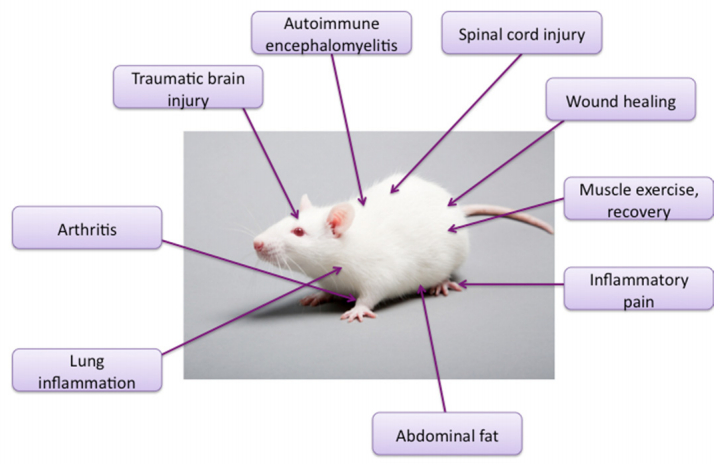
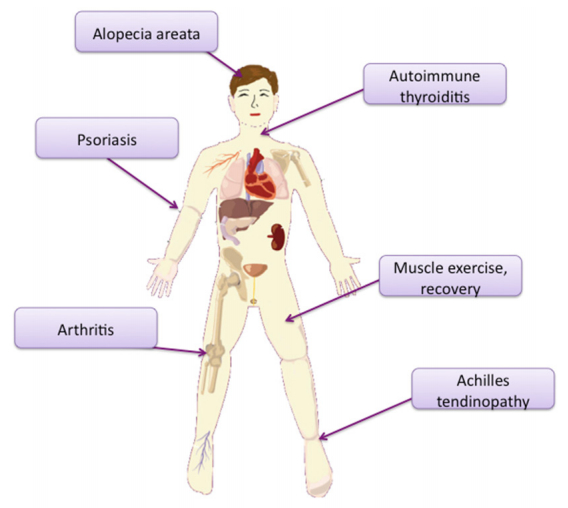
Photobiomodulation (PBM) also known as low-level level laser therapy is the use of red and near-infrared light to stimulate healing, relieve pain, and reduce inflammation. The primary chromophores have been identified as cytochrome c oxidase in mitochondria, and calcium ion channels (possibly mediated by light absorption by opsins). Secondary effects of photon absorption include increases in ATP, a brief burst of reactive oxygen species, an increase in nitric oxide, and modulation of calcium levels. Tertiary effects include activation of a wide range of transcription factors leading to improved cell survival, increased proliferation and migration, and new protein synthesis. There is a pronounced biphasic dose response whereby low levels of light have stimulating effects, while high levels of light have inhibitory effects. It has been found that PBM can produce ROS in normal cells, but when used in oxidatively stressed cells or in animal models of disease, ROS levels are lowered. PBM is able to up-regulate anti-oxidant defenses and reduce oxidative stress. It was shown that PBM can activate NF-kB in normal quiescent cells, however in activated inflammatory cells, inflammatory markers were decreased. One of the most reproducible effects of PBM is an overall reduction in inflammation, which is particularly important for disorders of the joints, traumatic injuries, lung disorders, and in the brain. PBM has been shown to reduce markers of M1 phenotype in activated macrophages. Many reports have shown reductions in reactive nitrogen species and prostaglandins in various animal models. PBM can reduce inflammation in the brain, abdominal fat, wounds, lungs, spinal cord.
Introduction
The algebraic classification (up to isomorphism) of algebras of dimension n from a certain variety defined by a certain family of polynomial identities is a classic problem in the theory of non-associative algebras. There are many results related to the algebraic classification of small-dimensional algebras in many varieties of non-associative algebras [11,12,2,3,4,6,13,9,16]. So, algebraic classifications of 2-dimensional algebras [16,19], 3-dimensional evolution algebras [1], 3-dimensional anticommutative algebras [17], 4-dimensional division algebras [5,7], 4-dimensional nilpotent algebras [13] and 6-dimensional anticommutative nilpotent algebras [12] have been given. In the present paper, we give the algebraic classification of 5-dimensional nilpotent commutative algebras. The variety of commutative algebras is defined by the following identity: xy=yx. It contains commutative CD-algebras, Jordan algebras, mock-Lie algebras and commutative associative algebras as subvarieties. On the other hand, it is a principal part in the varieties of weakly associative algebras and flexible algebras.
The algebraic study of central extensions of associative and non-associative algebras has been an important topic for years (see, for example, [10,20] and references therein). Our method for classifying nilpotent commutative algebras is based on the calculation of central extensions of nilpotent algebras of smaller dimensions from the same variety (first, this method has been developed by Skjelbred and Sund for Lie algebra case in [20]) and the classifications of all complex 5-dimensional nilpotent commutative (non-Jordan) CD-algebras [11]; nilpotent Jordan (non-associative) algebras [9]; and nilpotent associative commutative algebras [18].
1.
The algebraic classification of nilpotent commutative algebras
1.1. Method of classification of nilpotent algebras
Throughout this paper, we use the notations and methods well written in [10], which we have adapted for the commutative case with some modifications. Further in this section we give some important definitions.
Let (A,⋅) be a complex commutative algebra and V be a complex vector space. The C-linear space Z2(A,V) is defined as the set of all bilinear maps θ:A×A⟶V such that θ(x,y)=θ(y,x). These elements will be called cocycles. For a linear map f from A to V, if we define δf:A×A⟶V by δf(x,y)=f(xy), then δf∈Z2(A,V). We define B2(A,V)={θ=δf :f∈Hom(A,V)}. We define the second cohomology space H2(A,V) as the quotient space Z2(A,V)/B2(A,V).
Let Aut(A) be the automorphism group of A and let ϕ∈Aut(A). For θ∈Z2(A,V) define the action of the group Aut(A) on Z2(A,V) by
It is easy to verify that B2(A,V) is invariant under the action of Aut(A). So, we have an induced action of Aut(A) on H2(A,V).
Let A be a commutative algebra of dimension m over C and V be a C-vector space of dimension k. For the bilinear map θ, define on the linear space Aθ=A⊕V the bilinear product " [−,−]Aθ" by [x+x′,y+y′]Aθ=xy+θ(x,y) for all x,y∈A,x′,y′∈V. The algebra Aθ is called a k-dimensional central extension of A by V. One can easily check that Aθ is a commutative algebra if and only if θ∈Z2(A,V).
Call the set Ann(θ)={x∈A:θ(x,A)=0} the annihilator of θ. We recall that the annihilator of an algebra A is defined as the ideal Ann(A)={x∈A:xA=0}. Observe that Ann(Aθ)=(Ann(θ)∩Ann(A))⊕V.
The following result shows that every algebra with a non-zero annihilator is a central extension of a smaller-dimensional algebra.
Lemma 1.1. Let A be an n-dimensional commutative algebra such that
Then there exists, up to isomorphism, a unique (n−m)-dimensional commutative algebra A′ and a bilinear map θ∈Z2(A′,V) with Ann(A′)∩Ann(θ)=0, where V is a vector space of dimension m, such that A≅A′θ and A/Ann(A)≅A′.
Proof. Let A′ be a linear complement of Ann(A) in A. Define a linear map P:A⟶A′ by P(x+v)=x for x∈A′ and v∈Ann(A), and define a multiplication on A′ by [x,y]A′=P(xy) for x,y∈A′. For x,y∈A, we have
Since P is a homomorphism, P(A)=A′ and A′ is a commutative algebra and also A/Ann(A)≅A′, which gives us the uniqueness. Now, define the map θ:A′×A′⟶Ann(A) by θ(x,y)=xy−[x,y]A′. Thus, A′θ is A and therefore θ∈Z2(A′,V) and Ann(A′)∩Ann(θ)=0.
Definition 1.2. Let A be an algebra and I be a subspace of Ann(A). If A=A0⊕I then I is called an annihilator component of A. A central extension of an algebra A without annihilator component is called a non-split central extension.
Our task is to find all central extensions of an algebra A by a space V. In order to solve the isomorphism problem we need to study the action of Aut(A) on H2(A,V). To do that, let us fix a basis e1,…,es of V, and θ∈Z2(A,V). Then θ can be uniquely written as θ(x,y)=s∑i=1θi(x,y)ei, where θi∈Z2(A,C). Moreover, Ann(θ)=Ann(θ1)∩Ann(θ2)∩…∩Ann(θs). Furthermore, θ∈B2(A,V) if and only if all θi∈B2(A,C). It is not difficult to prove (see [10,Lemma 13]) that given a commutative algebra Aθ, if we write as above θ(x,y)=s∑i=1θi(x,y)ei∈Z2(A,V) and Ann(θ)∩Ann(A)=0, then Aθ has an annihilator component if and only if [θ1],[θ2],…,[θs] are linearly dependent in H2(A,C).
Let V be a finite-dimensional vector space over C. The Grassmannian Gk(V) is the set of all k-dimensional linear subspaces of V. Let Gs(H2(A,C)) be the Grassmannian of subspaces of dimension s in H2(A,C). There is a natural action of Aut(A) on Gs(H2(A,C)). Let ϕ∈Aut(A). For W=⟨[θ1],[θ2],…,[θs]⟩∈Gs(H2(A,C)) define ϕW=⟨[ϕθ1],[ϕθ2],…,[ϕθs]⟩. We denote the orbit of W∈Gs(H2(A,C)) under the action of Aut(A) by Orb(W). Given
we easily have that if W1=W2, then s⋂i=1Ann(θi)∩Ann(A)=s⋂i=1Ann(ϑi)∩Ann(A), and therefore we can introduce the set
which is stable under the action of Aut(A).
Now, let V be an s-dimensional linear space and let us denote by E(A,V) the set of all non-split s-dimensional central extensions of A by V. By above, we can write
We also have the following result, which can be proved as in [10,Lemma 17].
Lemma 1.3. Let Aθ,Aϑ∈E(A,V). Suppose that θ(x,y)=s∑i=1θi(x,y)ei and ϑ(x,y)=s∑i=1ϑi(x,y)ei. Then the commutative algebras Aθ and Aϑ are isomorphic if and only if
This shows that there exists a one-to-one correspondence between the set of Aut(A)-orbits on Ts(A) and the set of isomorphism classes of E(A,V). Consequently we have a procedure that allows us, given a commutative algebra A′ of dimension n−s, to construct all non-split central extensions of A′. This procedure is:
1. For a given commutative algebra A′ of dimension n−s, determine H2(A′,C), Ann(A′) and Aut(A′).
2. Determine the set of Aut(A′)-orbits on Ts(A′).
3. For each orbit, construct the commutative algebra associated with a representative of it.
1.2. Reduction to non-CCD-algebras
The idea of the definition of a CD-algebra comes from the following property of Jordan and Lie algebras: the commutator of any pair of multiplication operators is a derivation. This gives three identities of degree four, which reduce to only one identity of degree four in the commutative or anticommutative case. Namely, a commutative algebra is a commutative CD-algebra (CCD-algebra) if it satisfies the following identity:
The above described method gives all commutative (CCD- and non-CCD-) algebras. But we are interested in developing this method in such a way that it only gives non-CCD commutative algebras, because the classification of all CCD-algebras is done in [11]. Clearly, any central extension of a commutative non-CCD-algebra is a non-CCD-algebra. But a CCD-algebra may have extensions which are not CCD-algebras. More precisely, let D be a CCD-algebra and θ∈Z2C(D,C). Then Dθ is a CCD-algebra if and only if
for all x,y,a,b∈D. Define the subspace Z2D(D,C) of Z2C(D,C) by
Observe that B2(D,C)⊆Z2D(D,C). Let H2D(D,C)=Z2D(D,C)/B2(D,C). Then H2D(D,C) is a subspace of H2C(D,C). Define
Then Ts(D)=Rs(D) ⊍ Us(D). The sets Rs(D) and Us(D) are stable under the action of Aut(D). Thus, the commutative algebras corresponding to the representatives of Aut(D) -orbits on Rs(D) are CCD-algebras, while those corresponding to the representatives of Aut(D)-orbits on Us(D) are not CCD-algebras. Hence, we may construct all non-split commutative non-CCD-algebras A of dimension n with s-dimensional annihilator from a given commutative algebra A′ of dimension n−s in the following way:
1. If A′ is non-CCD, then apply the procedure.
2. Otherwise, do the following:
(a) Determine Us(A′) and Aut(A′).
(b) Determine the set of Aut(A′)-orbits on Us(A′).
(c) For each orbit, construct the commutative algebra corresponding to one of its representatives.
1.3. Notations
Let us introduce the following notations. Let A be a nilpotent algebra with a basis e1,e2,…,en. Then by Δij we will denote the bilinear form Δij:A×A⟶C with Δij(el,em)=δilδjm, if i≤j and l≤m. The set {Δij:1≤i≤j≤n} is a basis for the linear space of bilinear forms on A, so every θ∈Z2(A,V) can be uniquely written as θ=∑1≤i≤j≤ncijΔij, where cij∈C. Let us fix complex number ηk (ηkk=−1, ηlk≠1 for 0<l<k). For denote our algebras, we will use the following notations:
Remark 1. All families of algebras from our final list do not have intersections, but inside some families of algebras there are isomorphic algebras. All isomorphisms between algebras from a certain family of algebras constucted from the representative ∇(Σ) are given in the list of distinct orbit representations. The notation ⟨∇(Ξ)⟩O(Ξ1)=O(Ξ2) represents that the elements ⟨∇(Ξ1)⟩ and ⟨∇(Ξ2)⟩ have the same orbit.
1.4. 3- and 4-dimensional commutative algebras.
Thanks to [8] we have the complete classification of complex 4-dimensional nilpotent commutative algebras. It will be re-written by some different way for separating CCD- and non-CCD-algebras.
2.
Central extensions of 3-dimensional nilpotent commutative algebras
2.1. 2-dimensional central extension of N3∗02.
Here we will collect all information about N3∗02:
Let us use the following notations:
Take θ=4∑i=1αi∇i∈H2C(N3∗02). Since
we have
We are interested only in (α3,α4)≠(0,0) and consider the vector space generated by the following two cocycles:
Thus, we have
Consider the following cases.
1. α4≠0, then:
(a) β3=0,β2≠0,β1=0, then by choosing x=2α24, y=−α3α4, z=α23−2α1α4, we have the representatives ⟨∇4,∇2⟩;
(b) β3=0,β2≠0,β1≠0, then by choosing
we have the representatives ⟨∇4,∇1+α∇2⟩α≠0;
(c) β3=0,β2=0,β1≠0, then by choosing y=−xα32α4, we have two representatives ⟨∇4,∇1⟩ and ⟨∇2+∇4,∇1⟩, depending on α23=α2α4 or not. The first representative will be joint with the family from the case (1b);
(d) β3≠0,4α2β23=4β2α3β3−β22α4,β2=4β1, then by choosing
we have the representative ⟨∇4,∇3⟩;
(e) β3≠0,4α2β23=4β2α3β3−β22α4,β2≠4β1, then by choosing
we have the representative ⟨∇4,∇1+∇3⟩;
(f) β3≠0,4α2β23≠4β2α3β3−β22α4, then by choosing
we have the family of representatives ⟨∇2+∇4,α∇1+∇3⟩.
2. α4=0,α3≠0, then we may suppose that β3=0 and
(a) if β1≠0,β2=4β1,α2=4α1, then by choosing x=α3,y=−α1,z=0, we have the representative ⟨∇3,∇1+4∇2⟩;
(b) if β1≠0,β2=4β1,α2≠4α1, then by choosing x=α2−4α1α3,y=4α21−α1α2α23,z=0, we have the representative ⟨−24(∇2+∇3),∇1+4∇2⟩;
(c) if β1≠0,β2≠4β1, then by choosing x=α3(β2−4β1),y=β1α2−α1β2,z=0, we have the family of representatives ⟨∇3,∇1+α∇2⟩α≠4, which will be jointed with the case (2a);
(d) if β1=0, then we have the representative ⟨−3∇3,∇2⟩.
Summarizing, we have the following distinct orbits:
Note that the algebras constructed from the orbits ⟨∇1+4∇2,−24(∇2+∇3)⟩, ⟨∇1+λ∇2,∇3⟩, ⟨∇1+α∇2,∇4⟩, ⟨∇2,−3∇3⟩ and ⟨∇2,∇4⟩ are parts of some families of algebras which found below. Hence, we have the following new algebras:
2.2. 2-dimensional central extension of N3∗04.
Here we will collect all information about N3∗04:
Let us use the following notations:
Take θ=4∑i=1αi∇i∈H2C(N3∗04). Since
we have
Consider the following cases:
1. α4≠0, then consider the vector space generated by the following two cocycles:
Thus, we have
Then we consider the following subcases:
(a) β3=0,α3=0, then we have:
(i) if β1=0,α1=0, then we have the representative ⟨∇4,∇2⟩;
(ii) if β1=0,α1≠0, then by choosing x=5√α1α4−1, we have the representative ⟨∇1+∇4,∇2⟩;
(iii) if β1≠0,β2=0, then by choosing x=1 and z=−α2α−14, we have the representative ⟨∇4,∇1⟩;
(iv) if β1≠0,β2≠0, then by choosing x=√β1β2−1 and z=α1β2−β1α2α4√β1β2, we have the representative ⟨∇4,∇1+∇2⟩.
(b) β3=0,α3≠0, then we have:
(i) if β2=0, then by choosing x=√α3α4−1 and z=−α2√α3α−34, we have the representative ⟨∇3+∇4,∇1⟩;
(ii) if β2≠0,β1=0, then by choosing
we have the representative ⟨∇3+∇4,∇2⟩;
(iii) if β2≠0,β1≠0,β2α3=β1α4, then by choosing x=√α3α4−1 and z=0, we have the family of representatives ⟨α∇1+∇3+∇4,∇1+∇2⟩;
(iv) if β2≠0,β1≠0,β2α3≠β1α4, then by choosing
we have the family of representatives ⟨α∇3+∇4,∇1+∇2⟩α≠0,1, which will be jointed with the case (1(a)iv).
(c) β3≠0,α3=0, then we have:
(i) if β2=0,α1≠0, then by choosing x=5√α1α−14 and z=−α25√α1α−64, we have the family of representatives ⟨∇1+∇4,α∇1+∇3⟩;
(ii) if β2=0,α1=0, then by choosing z=−α2xα4, we have two representatives ⟨∇4,∇3⟩ or ⟨∇4,∇1+∇3⟩ depending on whether β1α4=α2β3 or not;
(iii) if β2≠0, then by choosing x=β2β3 and z=−α2β2β3α4, we have the family of representatives ⟨α∇1+∇4,β∇1+∇2+∇3⟩.
2. α4=0,α3≠0, then we may suppose that β3=0. Thus, we have
and consider the following subcases:
(a) β2=0, then we have two representatives ⟨∇3,∇1⟩ or ⟨∇2+∇3,∇1⟩, depending on whether α2=0 or not;
(b) β2≠0,α2=0, then by choosing z=−α1xα3, we have two representatives ⟨∇3,∇2⟩ or ⟨∇3,∇1+∇2⟩, depending on whether β1=0 or not.
3. α4=0,α3=0,β3=0,β2=0,α2≠0, then we have the representative ⟨∇2,∇1⟩.
Summarizing, we have the following distinct orbits:
Note that, the orbit ⟨∇1,∇2⟩ after a change of the basis of the constructed algebra gives a part of the family Nα79, which will be found below. Hence, we have the following new algebras:
3.
Central extensions of nilpotent CCD-algebras.
3.1. 1-dimensional central extensions of N4∗02.
Here we will collect all information about N4∗02:
Let us use the following notations:
Take θ=8∑i=1αi∇i∈H2C(N4∗02). Since
we have
We interested in (α2,α5,α7,α8)≠(0,0,0,0) and (α4,α6,α7)≠(0,0,0). Let us consider the following cases:
1. α6=0,α7=0, then α4≠0 and we have the following subcases:
(a) α8=0,α2α4−α1α5=0, then we have a split extension;
(b) α8=0,α2α4−α1α5≠0,α3=4α1, then by choosing
we have the representative ⟨∇2+∇4⟩;
(c) α8=0,α2α4−α1α5≠0,α3≠4α1, then by choosing
we have the representative ⟨∇2+∇3+∇4⟩;
(d) α8≠0,α3=4α1, then by choosing
we have the representative ⟨∇4+∇8⟩;
(e) α8≠0,α3≠4α1, then by choosing
we have the representative ⟨∇3+∇4+∇8⟩.
2. α6=0,α7≠0, then we have the following subcases:
(a) α4=0,α3=0, then by choosing
we have the representative ⟨∇7⟩;
(b) α4=0,α3≠0, then by choosing
we have the representative ⟨∇3+∇7⟩;
(c) α4≠0,α3α27−2α4α5α7+α24α8=0, then by choosing
we have the representative ⟨∇4+∇7⟩;
(d) α4≠0,α3α27−2α4α5α7+α24α8≠0, then by choosing
we have the representative ⟨∇4+∇5+∇7⟩.
3. α6≠0, then we have the following subcases:
(a) α6α8−α27=0,α5α6−α4α7=0,α2α6−α1α7=0, then we have a split extension;
(b) α6α8−α27=0,α5α6−α4α7=0,α2α6−α1α7≠0,α3α6−α24=0, then by choosing
we have the representative ⟨∇2+∇6⟩;
(c) α6α8−α27=0,α5α6−α4α7=0,α2α6−α1α7≠0,α3α6−α24≠0, then by choosing
and w=(α24−2α1α6)√α3α6−α242α36, we have the representative ⟨∇2+∇3+∇6⟩;
(d) α6α8−α27=0,α5α6−α4α7≠0,2α6(α2α6−α1α7)=α4(α5α6−α4α7), then by choosing
we have the representatives ⟨∇5+∇6⟩ and ⟨∇3+∇5+∇6⟩ depending on whether α3α6=α24 or not;
(e) α6α8−α27=0,α5α6−α4α7≠0,2α6(α2α6−α1α7)≠α4(α5α6−α4α7), then by choosing x=2α6(α2α6−α1α7)−α4(α5α6−α4α7)2α26(α5α6−α4α7), t=α26α5α6−α4α7x4, q=−α42α6x, r=α6α7α4α7−α5α6x4, e=0, w=α24−2α1α6α6x, we have the representative ⟨∇2+α∇3+∇5+∇6⟩;
(f) α6α8−α27≠0,α5α6−α4α7=0, then by choosing
we have the representatives ⟨∇6+∇8⟩ and ⟨∇3+∇6+∇8⟩ depending on whether α3α6=α24 or not.
(g) α6α8−α27≠0,α5α6−α4α7≠0, then by choosing
we have the representative ⟨α∇3+∇5+∇6+∇8⟩.
Summarizing, we have the following distinct orbits
which gives the following new algebras:
3.2. 1-dimensional central extensions of N4∗04.
Here we will collect all information about N4∗04:
Let us use the following notations:
Take θ=8∑i=1αi∇i∈H2C(N4∗04). Since
we have
We interested in (α3,α5,α7,α8)≠(0,0,0,0) and (α2,α4,α6,α7)≠(0,0,0,0). Let us consider the following cases:
1. α6=0,α7=0,α4=0, then α2≠0 and we have the following cases:
(a) if α8=0,α5=0, then by choosing t=1 and r=−α3α2, we have a split extension;
(b) if α8=0,α5≠0, then by choosing
we have the representative ⟨∇2+∇5⟩;
(c) if α8≠0,α5=0,α1=0, then by choosing
we have the representative ⟨∇2+∇8⟩;
(d) if α8≠0,α5=0,α1≠0, then by choosing
we have the representative ⟨∇1+∇2+∇8⟩;
(e) if α8≠0,α5≠0, then by choosing
we have the representative ⟨∇2+∇5+∇8⟩.
2. α6=0,α7=0,α4≠0, then by choosing r=−α5α4t,y=−α1x+α5zα4, we have α∗1=α∗5=0. Now we can suppose that α1=0,α5=0, and we have the following subcases:
(a) if α8=0,α3=0, then we have a split extension;
(b) if α8=0,α3≠0,α2=0, then by choosing x=α3, y=0, z=0, r=0, t=α43, we have the representative ⟨∇3+∇4⟩;
(c) if α8=0,α3≠0,α2≠0, then by choosing x=α2α4, y=0, z=0, r=0,t=α52α3α44, we have the representative ⟨∇2+∇3+∇4⟩;
(d) if α8≠0,α2=0, then by choosing x=1,y=0,z=−α3α8,r=0,t=√α4α8, we have the representative ⟨∇4+∇8⟩;
(e) if α8≠0,α2≠0, then by choosing x=α3α4,y=0,z=−α2α3α4α8,r=0,t=α32√α54α8, we have the representative ⟨∇2+∇4+∇8⟩.
3. α6=0,α7≠0, then by choosing r=−α8t2α7,y=−(α2α8−α3α7)xα27,z=−α2xα7, we have α∗2=α∗3=α∗8=0. Now we can suppose that α2=0, α3=0, α8=0, and consider the following cases:
(a) if α4=0,α5=0,α1=0, then we have the representative ⟨∇7⟩;
(b) if α4=0,α5=0,α1≠0, then by choosing x=1α7,t=α1,y=0,z=0,r=0, we have the representative ⟨∇1+∇7⟩;
(c) if α4=0,α5≠0,α1=0, then by choosing x=√α5α7,t=1,y=0,z=0,r=0, we have the representative ⟨∇5+∇7⟩;
(d) if α4=0,α5≠0,α1≠0, then by choosing x=√α5α7,t=√α21α5α7,y=0,z=0,r=0, we have the representative ⟨∇1+∇5+∇7⟩;
(e) if α4≠0,α5=0,α1=0, then by choosing x=√α7,t=α4,y=0,z=0,r=0, we have the representative ⟨∇4+∇7⟩;
(f) if α4≠0,α5=0,α1≠0, then by choosing x=3√α1α4,t=3√α1α24α37,y=0,z=0,r=0, we have the representative ⟨∇1+∇4+∇7⟩;
(g) if α4≠0,α5≠0, then by choosing x=√α5α7,t=√α4α5α27,y=0,z=0,r=0, we have the representative ⟨α∇1+∇4+∇5+∇7⟩.
4. α6≠0, then by choosing r=−α7tα6,y=−α2x+α7zα6, we have α∗2=α∗7=0. Now we can suppose that α2=0,α7=0, and we have:
(a) if α8=0,α5=0, then α3≠0 and we have the following subcases:
(i) α4=0,α1=0, then by choosing x=α3,t=α63α6,y=0,z=0,r=0, we have the representative ⟨∇3+∇6⟩;
(ii) α4=0,α1≠0, then by choosing x=5√α1α6,t=5√α71α53α26,y=0,z=0,r=0, we have the representative ⟨∇1+∇3+∇6⟩;
(iii) α4≠0, then by choosing x=√α4α6,t=5√α74α23α56,y=0,z=0,r=0, we have the representative ⟨α∇1+∇3+∇4+∇6⟩.
(b) α8=0,α5≠0, then we have the following subcases:
(i) α4=0,α3=0, then by choosing x=6√α5α6,t=1,z=−α16√α55α6,y=0,r=0, we have the representative ⟨∇5+∇6⟩;
(ii) α4=0,α3≠0, then by choosing x=α3α5,t=α63α−75α6,z=−α1α3α25,y=0,r=0, we have the representative ⟨∇3+∇5+∇6⟩;
(iii) α4≠0, then by choosing x=√α4α6,t=α34α5α26,z=−α1α−15√α4α−16,y=0,r=0, we have the representative ⟨α∇3+∇4+∇5+∇6⟩.
(c) α8≠0, then we have the following subcases:
(i) α5=0,α4=0,α1=0, then by choosing x=1,t=√α6α8,z=−α3α8,y=0,r=0, we have the representative ⟨∇6+∇8⟩;
(ii) α5=0,α4=0,α1≠0, then by choosing
we have the representative ⟨∇1+∇6+∇8⟩;
(iii) α5=0,α4≠0, then by choosing
we have the representative ⟨α∇1+∇4+∇6+∇8⟩;
(iv) α5≠0, then by choosing x=4√α25α6α8, t=α25√α6α38, z=−α3√α54√α6α58, y=0, r=0, we have the representative ⟨α∇1+β∇4+∇5+∇6+∇8⟩.
Summarizing, we have the following distinct orbits:
Hence, we have the following new algebras:
3.3. 1-dimensional central extensions of N4∗08.
Here we will collect all information about N4∗08:
Let us use the following notations:
Take θ=7∑i=1αi∇i∈H2C(N4∗08). Since
we have
We are interested in (α3,α4,α5,α6,α7)≠(0,0,0,0,0), (α2+α3,α4,α6,α7)≠(0,0,0,0) and (α1,α2,α5,α6)≠(0,0,0,0). Let us consider the following cases:
1. α7=0,α6=0,α5=0,α4=0, then α3≠0, α2+α3≠0 and (α1,α2)≠(0,0).
(a) if α2≠−α34, then by choosing x=4α2+α3,y=−α1, we have the representative ⟨α∇2+∇3⟩α≠0,−14,−1;
(b) if α2=−α34, then we have the representatives
depending on α1=0 or not.
2. α7=0,α6=0,α5=0,α4≠0, then by choosing y=−3α2α4x, we have α∗2=0. This we can suppose α2=0, which implies α1≠0 and choosing x=√α1α−14, we have the representative ⟨∇1+α∇3+∇4⟩.
3. α7=0,α6=0,α5≠0.
(a) if α4=0, then α2≠−α3 and choosing
we have the representative ⟨∇2+∇5⟩;
(b) if α4≠0,α4≠α5,2(α2α5−α2α4+α3α5)+α3α4=0, then by choosing
we have the representative ⟨α∇4+∇5⟩α≠0,1;
(c) if α4≠0,α4≠α5,2(α2α5−α2α4+α3α5)+α3α4≠0, then by choosing
we have the family of representatives ⟨∇2+α∇4+∇5⟩α≠0,1;
(d) if α4≠0,α4=α5, then by choosing y=−α2xα5 and z=(α3α5−α1α5+3α22)xα25, we have the representatives ⟨∇4+∇5⟩ and ⟨∇3+∇4+∇5⟩ depending on whether α3=0 or not. Note that ⟨∇4+∇5⟩=⟨∇2+∇4+∇5⟩ and it will be jointed with the family from the case (3c).
4. if α7=0,α6≠0, then by choosing x=1, y=√(α4+2α5)2−36α2α6−α4−2α56α6,
we have α∗1=α∗2=α∗3=0. Now we can suppose that α1=0,α2=0,α3=0, and we have the following cases:
(a) if α4=0,α5=0, then by choosing x=1,y=0,z=0,t=0, we have the representative ⟨∇6⟩;
(b) if α4=0,α5≠0, then by choosing x=−4α53α6,y=8α259α26,z=0,t=0, we have the representative ⟨∇4+14∇5+∇6⟩;
(c) if α4≠0, then by choosing x=α4α6,y=0,z=0,t=0, we have the family of representatives ⟨∇4+α∇5+∇6⟩, which will be jointed with the representative from the case (4b).
5. if α7≠0, then by choosing x=1, y=−α6α7, t=2α36+2(α4−α5)α6α7−3α3α273α37 and z=0, we have α∗3=0,α∗6=0. Now we can suppose that α3=0,α6=0, and we have the following cases:
(a) if α5≠0, then by choosing x=√α5α−17,y=0,z=√α21α−15α−17,t=0, we have the family of representatives ⟨α∇2+β∇4+∇5+∇7⟩;
(b) if α5=0,α2=0, then α1≠0 and we have the family of representatives ⟨∇1+α∇4+∇7⟩;
(c) if α5=0,α2≠0, then by choosing x=3√α2α−17,y=0,z=0,t=0, we have the family of representatives ⟨α∇1+∇2+β∇4+∇7⟩.
Summarizing all cases we have the following distinct orbits
which gives the following new algebras:
3.4. 1-dimensional central extensions of N4∗09.
Here we will collect all information about N4∗09:
Let us use the following notations:
Take θ=8∑i=1αi∇i∈H2C(N4∗09). Since
we have
We are interested in (α3,α5,α7,α8)≠(0,0,0,0). Let us consider the following cases:
1. α8=0,α7=0,α5=0, then α3≠0 and we have
(a) if α1=0,α4=0,α6=0, then by choosing x=1,r=α3,s=−α2,t=0, we have the representative ⟨∇3⟩;
(b) if α1=0,α4=0,α6≠0, then by choosing x=α6,r=α3α26,s=−α2α26,t=0, we have the representative ⟨∇3+∇6⟩;
(c) if α1=0,α4≠0,α6=0, then by choosing x=α23,r=α3α4,s=−α2α4,t=0, we have the representative ⟨∇3+∇4⟩;
(d) if α1=0,α4≠0,α6≠0, then by choosing x=α3−1√α4α6, r=α3−2√α34α6, s=−α2α3−3√α34α6, t=0, we have the representative ⟨∇3+∇4+∇6⟩;
(e) if α1≠0,α4=0,α6=0, then by choosing x=1, r=α1α3−1, s=−α1α2α−23, t=0, we have the representative ⟨∇1+∇3⟩;
(f) if α1≠0,α4=0,α6≠0, then by choosing x=3√α1α6α−23, r=α1α−13, s=−α1α2α−23, t=0, we have the representative ⟨∇1+∇3+∇6⟩;
(g) if α1≠0,α4≠0, then by choosing
we have the family of representatives ⟨∇1+∇3+∇4+α∇6⟩.
2. α8=0,α7=0,α5≠0 and we have
(a) if α3=0,α2=0,α4=0,α6=0, then by choosing r=1,x=α5,t=−α1,s=0, we have the representative ⟨∇5⟩;
(b) if α3=0,α2=0,α4=0,α6≠0, then by choosing x=α5α6, r=α55α36,s=0,t=−α1α6, we have the representative ⟨∇5+∇6⟩;
(c) if α3=0,α2=0,α4≠0,α6=0, then by choosing x=1,r=α4α5−1,t=−α1α5−1,s=0, we have the representative ⟨∇4+∇5⟩;
(d) if α3=0,α2=0,α4≠0,α6≠0, then by choosing
we have the representative ⟨∇4+∇5+∇6⟩;
(e) if α3=0,α2≠0,α4=0,α6=0, then by choosing
we have the representative ⟨∇2+∇5⟩;
(f) if α3=0,α2≠0,α4=0,α6≠0, then by choosing
we have the representative ⟨∇2+∇5+∇6⟩;
(g) if α3=0,α2≠0,α4≠0, then by choosing
we have the family of representatives ⟨∇2+∇4+∇5+α∇6⟩;
(h) if α3≠0,α4=0,α6=0, then by choosing x=α3α5−1,r=α3,s=−α2,t=−α1α3α−25, we have the representative ⟨∇3+∇5⟩;
(i) if α3≠0,α4=0,α6≠0, then by choosing
we have the representative ⟨∇3+∇5+∇6⟩;
(j) if α3≠0,α4≠0, then by choosing x=α3α5−1,r=α4α5−1,s=−α2α4α−13α−15,t=−α1α3α−25, we have the family of representatives ⟨∇3+∇4+∇5+α∇6⟩.
3. α8=0,α7≠0, then by choosing x=2α27, t=α3α6−2α2α7, s=−α6, r=2α7, we have α∗2=α∗6=0. Now we can suppose that α2=0 and α6=0, then for s=0 and t=0, we have:
(a) if α1=0,α3=0,α4=0,α5=0, then by choosing r=1,x=1, we have the representative ⟨∇7⟩;
(b) if α1=0,α3=0,α4=0,α5≠0, then by choosing x=α7,r=α5α7, we have the representative ⟨∇5+∇7⟩;
(c) if α1=0,α3=0,α4≠0,α5=0, then by choosing x=√α7,r=√α4, we have the representative ⟨∇4+∇7⟩;
(d) if α1=0,α3=0,α4≠0,α5≠0, then by choosing x=α4√α7α−35,r=α4α5−1, we have the representative ⟨∇4+∇5+∇7⟩;
(e) if α1=0,α3≠0,α5=0, then by choosing r=α3,x=α5, we have the family of representatives ⟨∇3+α∇4+∇7⟩;
(f) if α1=0,α3≠0,α5≠0, then by choosing x=α3α5−1,r=α23α−15α−17, we have the family of representatives ⟨∇3+α∇4+∇5+∇7⟩;
(g) if α1≠0,α3=0,α4=0,α5=0, then by choosing x=α1α7,r=α1, we have the representative ⟨∇1+∇7⟩;
(h) if α1≠0,α3=0,α4=0,α5≠0, then by choosing x=3√α1α7α−25,r=3√α21α−15α−17, we have the representative ⟨∇1+∇5+∇7⟩;
(i) if α1≠0,α3=0,α4≠0, then by choosing x=α1α4−1,r=α1√α−14α−17, we have the family of representatives ⟨∇1+∇4+α∇5+∇7⟩;
(j) if α1≠0,α3≠0, then by choosing x=α1α7α−23,r=α1α3−1, we have the family of representatives ⟨∇1+∇3+α∇4+β∇5+∇7⟩.
4. α8≠0, then by choosing x=α8,t=−α3,s=−α7,r=α8, we have α∗3=α∗7=0. Now we can suppose that α3=0 and α7=0, then for s=0 and t=0, we have
(a) if α1=0,α2=0,α4=0,α6=0, then we have the representatives ⟨∇8⟩ and ⟨∇5+∇8⟩, depending on whether α5=0 or not;
(b) if α1=0,α2=0,α4=0,α6≠0, then we have the representatives ⟨∇6+∇8⟩ and ⟨∇5+∇6+∇8⟩, depending on whether α5=0 or not;
(c) if α1=0,α2=0,α4≠0,α6=0, then we have the representatives ⟨∇4+∇8⟩ and ⟨∇4+∇5+∇8⟩, depending on whether α5=0 or not;
(d) if α1=0,α2=0,α4≠0,α6≠0, then by choosing x=4√α6α8−1,r=√α4α8−1, we have the representative ⟨∇4+α∇5+∇6+∇8⟩;
(e) if α1=0,α2≠0,α4=0,α5=0, then we have the representatives ⟨∇2+∇8⟩ and ⟨∇2+∇6+∇8⟩, depending on whether α6=0 or not;
(f) if α1=0,α2≠0,α4=0,α5≠0, then by choosing x=3√α2α5−1,r=α5α8−1, we have the representative ⟨∇2+∇5+α∇6+∇8⟩;
(g) if α1=0,α2≠0,α4≠0, then by choosing x=6√α22α−14α−18, r=√α4α8−1, we have the representative ⟨∇2+∇4+α∇5+β∇6+∇8⟩;
(h) if α1≠0,α2=0,α4=0,α5=0, then we have the representatives ⟨∇1+∇8⟩ and ⟨∇1+∇6+∇8⟩ depending on whether α6=0 or not;
(i) if α1≠0,α2=0,α4=0,α5≠0, then by choosing x=α1α8α−25,r=α5α8−1, we have the representative ⟨∇1+∇5+α∇6+∇8⟩;
(j) if α1≠0,α2=0,α4≠0, then by choosing x=α1α4−1,r=√α4α8−1, we have the representative ⟨∇1+∇4+α∇5+β∇6+∇8⟩;
(k) if α1≠0,α2≠0, then by choosing x=5√α22α−11α−18,r=5√α41α−32α−18, we have the representative ⟨∇1+∇2+α∇4+β∇5+γ∇6+∇8⟩.
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
3.5. 1-dimensional central extensions of N4∗10.
Here we will collect all information about N4∗10:
Let us use the following notations:
Take θ=8∑i=1αi∇i∈H2C(N4∗10). Since
we have
We are interested in (α5,α7,α8)≠(0,0,0). Let us consider the following cases:
1. α8=0,α5=0, then α7≠0. Now by choosing
we have α∗1=0,α∗2=0,α∗4=0,α∗6=0. Then we have the representatives ⟨∇7⟩ or ⟨∇3+∇7⟩ depending on whether α3=0 or not.
2. α8=0,α5≠0, then by choosing
we have α∗2=α∗4=α∗6=0 and α∗7=0. Therefore, we can suppose that α2=0,α4=0,α6=0,α7=0, and have the following cases:
(a) if α1=0,α3=0, then we have the representative ⟨∇5⟩;
(b) if α1=0,α3≠0, then by choosing x=α3α5,r2=x3, we have the representative ⟨∇3+∇5⟩;
(c) if α1≠0, then by choosing x=5√α21α25,r2=x3, we have the family of representatives ⟨∇1+α∇3+∇5⟩.
3. α8≠0, then by choosing y=−α5x2+α8z22α8x,s=√x(α5z−α7x)α8,t=−α2x+α5y+α7zα8, we have α∗2=α∗5=α∗7=0. Therefore, we can suppose that α2=0,α5=0,α7=0, and have the following cases:
(a) if α3=0,α4=0,α6=0, then we have the representative ⟨∇8⟩ and ⟨∇1+∇8⟩ depending on whether α1=0 or not.
(b) if α3=0,α4=0,α6≠0, then by choosing x=3√α6α8,r2=x3,z=−α13√α26α8, we have the representative ⟨∇6+∇8⟩;
(c) if α3=0,α4≠0, then by choosing x=5√α24α28,r2=x3,z=α635√α34α28, we have the representative ⟨α∇1+∇4+∇8⟩;
(d) if α3≠0, then by choosing x=√α3α8,r2=x3,z=α4√α3α8, we have the representative ⟨α∇1+∇3+β∇6+∇8⟩.
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
3.6. 1-dimensional central extensions of N4∗11.
Here we will collect all information about N4∗11:
Let us use the following notations:
Take θ=8∑i=1αi∇i∈H2C(N4∗11). Since
we have
We are interested in (α2,α5,α7,α8)≠(0,0,0,0). Let us consider the following cases:
1. α8=0,α7=0,α5=0, then α2≠0 and we have
(a) if α4=0,α6=0, then by choosing x=2α2,z=−α1,s=8α22α3,t=0, we have the representative ⟨∇2⟩;
(b) if α4=0,α6≠0, then by choosing x=α2α6,z=−α12α6,s=α1α2α6+2α22α32α36,t=0, we have the representative ⟨∇2+∇6⟩;
(c) if α4≠0,α4=−2α2,α1≠0, then by choosing
we have the family of representatives \langle \nabla_1+\nabla_2-2\nabla_4+\alpha\nabla_6 \rangle;
{\rm{(d)}}\ if \alpha_4\neq0, \alpha_4 = -2\alpha_2, \alpha_1 = 0, \alpha_6\neq 0, then by choosing x = \alpha_2\alpha_6^{-1}, z = 0, s = \alpha_2^2\alpha_3\alpha_6^{-3}, t = 0, we have the representative \langle \nabla_2-2\nabla_4 +\nabla_6 \rangle;
{\rm{(e)}}\ if \alpha_4\neq0, \alpha_4 = -2\alpha_2, \alpha_1 = 0,\alpha_6 = 0, then by choosing x = \alpha_2, z = 0, s = \alpha_2^2\alpha_3, t = 0, we have the representative \langle \nabla_2-2\nabla_4 \rangle;
{\rm{(f)}}\ if \alpha_4\neq0, \alpha_4\neq-2\alpha_2, \alpha_6 = 0, then by choosing
we have the the family of representatives \langle \nabla_2+\alpha\nabla_4 \rangle_{\alpha\neq0,-2}, which will be jointed with the cases (1a) and (1b);
{\rm{(g)}}\ if \alpha_4\neq0, \alpha_4\neq-2\alpha_2, \alpha_6\neq0, then by choosing
we have the family of representatives \langle \nabla_2+\alpha\nabla_4+\nabla_6 \rangle_{\alpha\neq0,-2}, which will be jointed with the cases (1b) and (1d).
2. \alpha_8 = 0, \alpha_7 = 0, \alpha_5\neq0 , then we have
{\rm{(a)}}\ if \alpha_6 = 0, \alpha_2 = 0, then by choosing
we have the representative \langle \nabla_5 \rangle;
{\rm{(b)}}\ if \alpha_6 = 0, \alpha_2\neq0, then by choosing
we have the representative \langle \nabla_2+\nabla_5 \rangle;
{\rm{(c)}}\ if \alpha_6\neq0, \alpha_6 = 4\alpha_5, \alpha_3 = 0, \alpha_2 = 0, then by choosing x = \alpha_5, z = 0, s = -\alpha_4\alpha_5^2, t = -\alpha_1, we have the representative \langle \nabla_5+4\nabla_6 \rangle;
{\rm{(d)}}\ if \alpha_6\neq0, \alpha_6 = 4\alpha_5, \alpha_2\alpha_4+\alpha_3\alpha_5 = 0, \alpha_2\neq0, then by choosing
we have the representative \langle \nabla_2+\nabla_5+4\nabla_6 \rangle;
{\rm{(e)}}\ if \alpha_6\neq0, \alpha_6 = 4\alpha_5, \alpha_2\alpha_4+\alpha_3\alpha_5\neq0, then by choosing
we have the family of representatives \langle \alpha\nabla_2+\nabla_3+\nabla_5+4\nabla_6 \rangle;
{\rm{(f)}}\ if \alpha_6\neq0, \alpha_6\neq4\alpha_5, \alpha_2 = 0, then by choosing
we have the family of representatives \langle \nabla_5+\alpha\nabla_6 \rangle_{\alpha\neq0,4}, which will be jointed with the cases (2a) and (2c);
{\rm{(g)}}\ if \alpha_6\neq0, \alpha_6\neq4\alpha_5, \alpha_2\neq0, then by choosing
we have the family of representatives \langle \nabla_2+\nabla_5+\alpha\nabla_6 \rangle_{(\alpha\neq0,4)}, which will be jointed with the cases (2a) and (2c).
3. \alpha_8 = 0, \alpha_7\neq0 , then by choosing z = -\frac{\alpha_2}{\alpha_7}x, s = -\frac{\alpha_6}{2\alpha_7}x^3, t = \frac{ \alpha_2 (\alpha_6-4 \alpha_5)+\alpha_3 \alpha_7}{\alpha^2_7}x, we have \alpha_2^* = \alpha_3^* = \alpha_6^* = 0 . Now we can suppose that \alpha_2 = 0, \alpha_3 = 0, \alpha_6 = 0 and have the following subcases:
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_4 = 0, \alpha_5 = 0, then we have the representative \langle \nabla_7 \rangle;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_4 = 0, \alpha_5\neq0, then by choosing x = \frac{\alpha_5}{\alpha_7}, z = 0, s = 0, t = 0, we have the representative \langle \nabla_5+\nabla_7 \rangle;
{\rm{(c)}}\ if \alpha_1 = 0, \alpha_4\neq0, then by choosing x = \sqrt{\frac{\alpha_4}{\alpha_7}}, z = 0, s = 0, t = 0, we have the family of representatives \langle \nabla_4+\alpha\nabla_5+\nabla_7 \rangle;
{\rm{(d)}}\ if \alpha_1\neq0, then by choosing x = \sqrt[4]{\frac{\alpha_1}{\alpha_7}}, z = 0, s = 0, t = 0, we have the family of representatives \langle \nabla_1+\alpha\nabla_4+\beta\nabla_5+\nabla_7 \rangle.
4. \alpha_8\neq0 , then by choosing z = -\frac{\alpha_5}{2\alpha_8}x, s = -\frac{\alpha_7}{\alpha_8}x^3, t = \frac{\alpha_5\alpha_7-2\alpha_2\alpha_8}{2\alpha^2_8}x, we have \alpha_2^* = \alpha_5^* = \alpha_7^* = 0 . Now we can suppose that \alpha_2 = 0, \alpha_5 = 0, \alpha_7 = 0 and have the following subcases:
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_3 = 0, \alpha_4 = 0, \alpha_6 = 0, then we have the representative \langle \nabla_8 \rangle;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_3 = 0, \alpha_4 = 0, \alpha_6\neq0, then by choosing x = \sqrt{\frac{\alpha_6}{\alpha_8}}, z = 0, s = 0, t = 0, we have the representative \langle \nabla_6+\nabla_8 \rangle;
{\rm{(c)}}\ if \alpha_1 = 0, \alpha_3 = 0, \alpha_4\neq0, then by choosing x = \sqrt[3]{\frac{\alpha_4}{\alpha_8}}, z = 0, s = 0, t = 0, we have the family of representatives \langle \nabla_4+\alpha\nabla_6+\nabla_8 \rangle;
{\rm{(d)}}\ if \alpha_1 = 0, \alpha_3\neq0, then by choosing x = \sqrt[4]{\frac{\alpha_3}{\alpha_8}}, z = 0, s = 0, t = 0, we have the family of representatives \langle \nabla_3+\alpha\nabla_4+\beta\nabla_6+\nabla_8 \rangle;
{\rm{(e)}}\ if \alpha_1\neq0, then by choosing x = \sqrt[5]{\frac{\alpha_1}{\alpha_8}}, z = 0, s = 0, t = 0, we have the family of representatives \langle \nabla_1+\alpha\nabla_3+\beta\nabla_4+\gamma\nabla_6+\nabla_8 \rangle.
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
3.7. 1 -dimensional central extensions of {\mathbf N}_{12}^{4*} .
Here we will collect all information about {\mathbf N}_{12}^{4*}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{8}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4*}_{12}) . Since
we have
We will consider only the action of \phi_+ for find representatives and after that we will see that the set of our representatives gives distinct orbits under action of \phi_+ and \phi_-. We are interested in (\alpha_3,\alpha_5,\alpha_7,\alpha_8)\neq(0,0,0,0) . Let us consider the following cases:
1.\ \alpha_8 = 0, \alpha_5 = 0, \alpha_7 = 0, then \alpha_3\neq0 and we have the following subcases:
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_4 = 0, \alpha_6 = 0, then by choosing x = \alpha_3, s = -\alpha_2\alpha_3, t = 0, we have the representative \langle \nabla_3 \rangle;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_4 = 0, \alpha_6\neq0, then by choosing x = \frac{\alpha_6}{\alpha_3}, s = -\frac{\alpha_2\alpha_6^2}{\alpha_3^3}, t = 0, we have the representative \langle \nabla_3+\nabla_6 \rangle;
{\rm{(c)}}\ if \alpha_1 = 0, \alpha_4\neq0, then by choosing x = \frac{\alpha_4}{\alpha_3}, s = -\frac{\alpha_2\alpha^2_4}{\alpha_3^3}, t = 0, we have the representative \langle \nabla_3+\nabla_4+\alpha\nabla_6 \rangle;
{\rm{(d)}}\ if \alpha_1\neq0, then by choosing x = \sqrt{\frac{\alpha_1}{\alpha_3}}, s = -\frac{\alpha_1\alpha_2}{\alpha_3^2}, t = 0, we have the representative \langle \nabla_1+\nabla_3+\alpha\nabla_4+\beta\nabla_6 \rangle.
2. \alpha_8 = 0, \alpha_5 = 0, \alpha_7\neq0, then we have the following subcases:
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_3 = 0, \alpha_4 = 0, then by choosing x = 1, s = -\frac{\alpha_6}{2\alpha_7}, t = \frac{\alpha_3\alpha_6-2\alpha_2\alpha_7}{2\alpha_7^2}, we have the representative \langle \nabla_7 \rangle;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_3 = 0, \alpha_4\neq0, then by choosing x = \sqrt{\frac{\alpha_4}{\alpha_7}}, s = -\frac{\alpha_4\alpha_6}{2\alpha^2_7}, t = \frac{\sqrt{\alpha_4}(\alpha_3\alpha_6-2\alpha_2\alpha_4)}{2\alpha_7^2\sqrt{\alpha_7}}, we have the representative \langle \nabla_4+\nabla_7 \rangle;
{\rm{(c)}}\ if \alpha_1 = 0, \alpha_3\neq0, then by choosing x = \frac{\alpha_3}{\alpha_7}, s = -\frac{\alpha^2_3\alpha_6}{2\alpha^3_7}, t = \frac{\alpha_3^2\alpha_6-2\alpha_2\alpha_3\alpha_7}{2\alpha_7^3}, we have the representative \langle \nabla_3+\alpha\nabla_4+\nabla_7 \rangle;
{\rm{(d)}}\ if \alpha_1\neq0, then by choosing x = \sqrt[3]{\frac{\alpha_1}{\alpha_7}}, s = -\sqrt[3]{\frac{\alpha_1^2\alpha^3_6}{8\alpha^5_7}}, t = \frac{\sqrt[3]{\alpha_1}(\alpha_3\alpha_6-2\alpha_2\alpha_7)}{2\alpha_7^2\sqrt[3]{\alpha_7}}, we have the representative \langle \nabla_1+\alpha\nabla_3+\beta\nabla_4+\nabla_7 \rangle.
3. \alpha_8 = 0, \alpha_5\neq0, then by choosing t = -\frac{\alpha_1}{\alpha_5}x, s = -\frac{\alpha_4}{\alpha_5}x^2, we have \alpha_1^* = \alpha_4^* = 0. Now we can suppose that \alpha_1 = 0, \alpha_4 = 0 and have the following subcases:
{\rm{(a)}}\ if \alpha_2 = 0, \alpha_3 = 0, \alpha_6 = 0, then we have the family of representatives \langle \nabla_5+\alpha\nabla_7 \rangle;
{\rm{(b)}}\ if \alpha_2 = 0, \alpha_3 = 0, \alpha_6\neq0, then by choosing x = \sqrt{\frac{\alpha_6}{\alpha_5}}, s = 0, t = 0, we have the family of representatives \langle \nabla_5+\nabla_6+\alpha\nabla_7 \rangle;
{\rm{(c)}}\ if \alpha_2 = 0, \alpha_3\neq0, then by choosing x = \frac{\alpha_3}{\alpha_5}, s = 0, t = 0, we have the family of representatives \langle \nabla_3+\nabla_5+\alpha\nabla_6+\beta\nabla_7 \rangle;
{\rm{(d)}}\ if \alpha_2\neq0, then by choosing x = \sqrt[3]{\frac{\alpha_2}{\alpha_5}}, s = 0, t = 0, we have the family of representatives \langle \nabla_2+\alpha\nabla_3+\nabla_5+\beta\nabla_6+\gamma\nabla_7 \rangle.
4. \alpha_8\neq0, then by choosing t = -\frac{\alpha_3}{\alpha_8}x, s = -\frac{\alpha_7}{\alpha_8}x^2, we have \alpha_3^* = \alpha_7^* = 0. Now we can suppose that \alpha_3 = 0, \alpha_7 = 0 and have the following subcases:
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_2 = 0, \alpha_4 = 0, \alpha_5 = 0, \alpha_6 = 0, then we have the representative \langle \nabla_8 \rangle;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_2 = 0, \alpha_4 = 0, \alpha_5 = 0, \alpha_6\neq0, then by choosing x = \sqrt[4]{\frac{\alpha_6}{\alpha_8}}, s = 0, t = 0, we have the representative \langle \nabla_6+\nabla_8 \rangle;
{\rm{(c)}}\ if \alpha_1 = 0, \alpha_2 = 0, \alpha_4 = 0, \alpha_5\neq0, then by choosing x = \sqrt{\frac{\alpha_5}{\alpha_8}}, s = 0, t = 0, we have the family of representatives \langle \nabla_5+\alpha\nabla_6+\nabla_8 \rangle;
{\rm{(d)}}\ if \alpha_1 = 0, \alpha_2 = 0, \alpha_4\neq0, then by choosing x = \sqrt[4]{\frac{\alpha_4}{\alpha_8}}, s = 0, t = 0, we have the family of representatives \langle \nabla_4+\alpha\nabla_5+\beta\nabla_6+\nabla_8 \rangle;
{\rm{(e)}}\ if \alpha_1 = 0, \alpha_2\neq0, then by choosing x = \sqrt[5]{\frac{\alpha_2}{\alpha_8}}, s = 0, t = 0, we have the family of representatives
{\rm{(f)}}\ if \alpha_1\neq0, then by choosing x = \sqrt[5]{\frac{\alpha_1}{\alpha_8}}, s = 0, t = 0, we have the family of representatives
Summarizing all cases we have the following distinct orbits:
which gives the following new algebras:
3.8. 1 -dimensional central extensions of {\mathbf N}_{13}^{4*}(\lambda) .
Here we will collect all information about {\mathbf N}_{13}^{4*}(\lambda):
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{7}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}({\mathbf N}_{13}^{4*}(\lambda)) . Since
we have
We are interested in
Let us consider the following cases:
1.\ \alpha_7 = 0, \alpha_6 = 0, \alpha_5 = 0, \alpha_4 = 0, then \alpha_1\neq0, \alpha_3\neq0 and
{\rm{(a)}}\ if \lambda\notin \{ 1,2,4\}, (\lambda-4)\alpha_3\neq4(1-\lambda)(\lambda-2)\alpha_1, then by choosing y = \frac{\alpha_2x}{(\lambda-4)\alpha_3+4(\lambda-1)(\lambda-2)\alpha_1}, we have the family of representatives
{\rm{(b)}}\ if \lambda\notin \{ 1,2,4\}, (\lambda-4)\alpha_3 = 4(1-\lambda)(\lambda-2)\alpha_1, \alpha_2 = 0, then we have the family of representatives \langle \frac{\lambda-4}{4(1-\lambda)(\lambda-2)}\nabla_1+\nabla_3 \rangle_{\lambda\neq 1,2,4}, which we will be jointed with the family from the case (1a);
{\rm{(c)}}\ if \lambda\notin \{ 1,2,4\}, (\lambda-4)\alpha_3 = 4(1-\lambda)(\lambda-2)\alpha_1, \alpha_2\neq0, then by choosing x = \frac{\alpha_2}{\alpha_3}, y = 0, z = 0, t = 0, we have the family of representatives \langle (\lambda-4)\nabla_1+4(1-\lambda)(\lambda-2) (\nabla_2+\nabla_3) \rangle_{\lambda\neq 1,2,4};
{\rm{(d)}}\ if \lambda\in \{ 1,2,4\}, then by choosing some suitable x and y we have the family of representatives \langle \alpha\nabla_1+\nabla_3 \rangle_{\alpha\neq 0, \lambda\in \{1,2,4\}}, which will be jointed with the family from the case (1a).
2. \alpha_7 = 0, \alpha_6 = 0, \alpha_5 = 0, \alpha_4\neq0, then we have
{\rm{(a)}}\ if \alpha_3 = 2(2-\lambda)\alpha_1 , then by choosing
we have the representative \langle \nabla_4 \rangle;
{\rm{(b)}}\ if \alpha_3\neq2(2-\lambda)\alpha_1 , then by choosing
we have the representative \langle \nabla_3+\nabla_4 \rangle .
3. \alpha_7 = 0, \alpha_6 = 0, \alpha_5\neq0, then
{\rm{(a)}}\ if \alpha_4 = 0, then \alpha_1\neq0 and
{\rm{(i)}}\ if \lambda\neq0, then by choosing
we have the family of representatives \langle \nabla_1+\nabla_5 \rangle_{\lambda\neq0};
{\rm{(ii)}}\ if \lambda = 0, then by choosing x = \frac{\alpha_1}{\alpha_5}, y = -\frac{\alpha_1\alpha_3}{2\alpha_5^2}, z = 0, t = 0, we have the family representative \langle \nabla_1+\alpha\nabla_2+\nabla_5 \rangle_{\alpha\neq0,\lambda = 0} and the representative \langle \nabla_1+\nabla_5 \rangle_{\lambda = 0}, which will be jointed with the family from the case (3(a)i).
(b) if \alpha_4\neq0 and \lambda = 0, then we have the followings:
{\rm{(i)}}\ if \alpha_3\alpha_4 = 2\alpha_1(2\alpha_4+\alpha_5), then by choosing
we have the family of representatives \langle \alpha\nabla_4+\nabla_5 \rangle_{\alpha\neq0,\lambda = 0};
{\rm{(ii)}}\ if \alpha_3\alpha_4\neq 2\alpha_1(2\alpha_4+\alpha_5), then by choosing
we have the family of representatives \langle \nabla_3+\alpha\nabla_4+\nabla_5 \rangle_{\alpha\neq0, \lambda = 0} ;
(c) if \alpha_4\neq0 and \lambda\neq 0, then we have the followings:
{\rm{(i)}}\ if 4\alpha_4 = \lambda\alpha_5, 4\lambda(\lambda-4)\alpha_1\alpha_3+\lambda^2\alpha_2\alpha_5+4(3\lambda^3-12\lambda^2+8\lambda+16)\alpha_1^2 = 0, \lambda\alpha_3+2(\lambda^2-2\lambda-4)\alpha_1 = 0, then by choosing x = 1, y = -\frac{\alpha_1}{\alpha_4}, z = 0, t = 0, we have the family of representatives \langle \frac{\lambda}{4}\nabla_4+\nabla_5 \rangle_{\lambda\neq0} ;
{\rm{(ii)}}\ if 4\alpha_4 = \lambda\alpha_5, 4\lambda(\lambda-4)\alpha_1\alpha_3+\lambda^2\alpha_2\alpha_5+4(3\lambda^3-12\lambda^2+8\lambda+16)\alpha_1^2 = 0, \lambda\alpha_3+2(\lambda^2-2\lambda-4)\alpha_1\neq0, then by choosing
we have the family of representatives \langle \nabla_3+\frac{\lambda}{4}\nabla_4+\nabla_5 \rangle_{\lambda\neq0} ;
{\rm{(iii)}}\ if 4\alpha_4 = \lambda\alpha_5, 4\lambda(\lambda-4)\alpha_1\alpha_3+\lambda^2\alpha_2\alpha_5+4(3\lambda^3-12\lambda^2+8\lambda+16)\alpha_1^2\neq0, then by choosing
we have the family of representatives \langle \nabla_2+\alpha\nabla_3+\frac{\lambda}{4}\nabla_4+\nabla_5 \rangle_{\lambda\neq0} ;
{\rm{(iv)}}\ if \lambda\neq0, 4\alpha_4\neq\lambda\alpha_5 , then by choosing
we have two families of representatives
depending on \alpha_3\alpha_4-2\alpha_1\alpha_5+2(\lambda-2)\alpha_1\alpha_4 = 0 or not. These families will be jointed with representatives from cases (3(c)i) and (3(c)ii).
4. \alpha_7 = 0, \alpha_6\neq0, then by choosing y = -\frac{\alpha_4}{2\alpha_6}x, z = -\frac{\alpha_4^2-2\alpha_1\alpha_6}{2\alpha^2_6}x, we have \alpha_1^* = 0, \alpha_4^* = 0. Since we can suppose that \alpha_1 = 0, \alpha_4 = 0 and
{\rm{(a)}}\ if \lambda\neq0, \alpha_3 = 0, then by choosing t = {\frac{\alpha_2}{\lambda\alpha_6}}x, we have the representatives \langle \nabla_6 \rangle _{\lambda\neq0} and \langle \nabla_5+\nabla_6 \rangle _{\lambda\neq0} depending on \alpha_5 = 0 or not;
{\rm{(b)}}\ if \lambda\neq0, \alpha_3\neq0, then by choosing x = \sqrt{\frac{\alpha_3}{\alpha_6}}, t = {\frac{\alpha_2\sqrt{\alpha_3}}{\lambda\alpha_6\sqrt{\alpha_6}}}, we have the family of representatives \langle \nabla_3+\alpha\nabla_5+\nabla_6 \rangle _{\lambda\neq0};
{\rm{(c)}}\ if \lambda = 0, \alpha_2 = 0, \alpha_3 = 0, then we have the representatives \langle \nabla_6 \rangle _{\lambda = 0} and \langle \nabla_5+\nabla_6 \rangle _{\lambda = 0} depending on \alpha_5 = 0 or not, which will be jointed with representatives from the case (4a);
{\rm{(d)}}\ if \lambda = 0, \alpha_2 = 0, \alpha_3\neq0, then by choosing x = \sqrt{\frac{\alpha_3}{\alpha_6}}, t = 0 , we have the family of representatives \langle \nabla_3+\alpha\nabla_5+\nabla_6 \rangle _{(\lambda = 0)}, which will be jointed with the family of representatives from the case (4b);
{\rm{(e)}}\ if \lambda = 0, \alpha_2\neq0, then by choosing x = \sqrt[3]{\frac{\alpha_2}{\alpha_6}}, t = 0, we have the family of representatives \langle \nabla_2+\alpha\nabla_3+\beta\nabla_5+\nabla_6 \rangle _{\lambda = 0}.
5. \alpha_7\neq0, \lambda\neq-2, then by choosing
we have \alpha_1^* = 0, \alpha_4^* = 0, \alpha_6^* = 0. Now we can suppose that \alpha_1 = 0, \alpha_4 = 0, \alpha_6 = 0 then we have
{\rm{(a)}}\ if \alpha_3 = 0, \alpha_5 = 0, \alpha_2 = 0, then we have the representative \langle \nabla_7 \rangle_{\lambda\neq-2} ;
{\rm{(b)}}\ if \alpha_3 = 0, \alpha_5 = 0, \alpha_2\neq0, then by choosing x = \sqrt[4]{\alpha_2\alpha_7^{-1}}, we have the representative \langle \nabla_2+\nabla_7 \rangle_{\lambda\neq-2} ;
{\rm{(c)}}\ if \alpha_5\neq0, then by choosing x = \sqrt{\alpha_5\alpha_7^{-1}}, y = -\frac{\alpha_3}{2 \sqrt{\alpha_5 \alpha_7}}, z = \frac{\alpha_3^2}{ 4 \sqrt{\alpha_5^3\alpha_7}}, t = 0, we have the family of representatives \langle \alpha\nabla_2+\nabla_5+\nabla_7 \rangle_{\lambda\neq-2} .
{\rm{(d)}}\ if \alpha_3\neq0, \alpha_5 = 0 then by choosing x = \sqrt[3]{\alpha_3\alpha_7^{-1}}, we have the family of representatives \langle \alpha\nabla_2+\nabla_3+ \nabla_7 \rangle_{\lambda\neq-2} .
6. \alpha_7\neq0, \lambda = -2, then
{\rm{(a)}}\ if \alpha_6 = 0, \alpha_5 = 0, then by choosing z = \frac{y^2}{x}-\frac{\alpha_4x}{2\alpha_7}, t = -\frac{x \alpha_1+y \alpha_4}{\alpha_7}, we have \alpha_1^* = 0 and \alpha_4^* = 0. Now consider the followings:
{\rm{(i)}}\ if \alpha_3 = 8\alpha_1, \alpha_2\alpha_7-\alpha_4^2 = 0, then we have the representative \langle \nabla_7 \rangle_{\lambda = -2}, which will be jointed with the representative from the case (5a);
{\rm{(ii)}}\ if \alpha_3 = 8\alpha_1, \alpha_2\alpha_7-\alpha_4^2\neq0, then by choosing x = \sqrt[4]{\frac{\alpha_2\alpha_7-\alpha_4^2}{\alpha_7^2}}, y = 0, we have the representative \langle \nabla_2+\nabla_7 \rangle_{\lambda = -2}, which will be jointed with the representative from the case (5b);
{\rm{(iii)}}\ if \alpha_3\neq8\alpha_1, then by choosing x = \sqrt[3]{\frac{\alpha_3-8\alpha_1}{\alpha_7}}, y = \frac{\alpha_2\alpha_7-\alpha_4^2}{48\alpha_1\alpha_7-6\alpha_3\alpha_7}x, we have the representative \langle \nabla_3+\nabla_7 \rangle_{\lambda = -2} .
(b) if \alpha_6 = 0, \alpha_5\neq0, then by choosing
we have the family of representatives \langle \alpha\nabla_2+\nabla_5+\nabla_7 \rangle_{\lambda = -2}, which will be jointed with the representative from the case (5c).
(c) if \alpha_6\neq0, then we have the following cases:
{\rm{(i)}}\ if \alpha_5\alpha_7 = \alpha_6^2, 8\alpha_1\alpha_7+\alpha_4\alpha_6 = \alpha_3\alpha_7, then by choosing
we have the family of representatives \langle \alpha\nabla_2+\nabla_5+\nabla_6+\nabla_7 \rangle_{\lambda = -2};
{\rm{(ii)}}\ if \alpha_5\alpha_7 = \alpha_6^2, 8\alpha_1\alpha_7+\alpha_4\alpha_6\neq \alpha_3\alpha_7, then by choosing
we have the family of representatives
{\rm{(iii)}}\ if \alpha_5\alpha_7-\alpha_6^2\neq0, then by choosing
we have the family of representatives
which will be jointed with the family from the case (6(c)i).
Summarizing all cases we have the following distinct orbits:
Now we have the following new algebras
3.9. 1 -dimensional central extensions of {\mathbf N}_{14}^{4*} .
Here we will collect all information about {\mathbf N}_{14}^{4*}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{8}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4*}_{14}) . Since
we have
We are interested in (\alpha_2,\alpha_5,\alpha_7,\alpha_8)\neq(0,0,0,0) . Let us consider the following cases:
1.\ \alpha_8 = 0, \alpha_7 = 0, \alpha_5 = 0, then \alpha_2\neq0 and we have
{\rm{(a)}}\ if \alpha_6 = 0, \alpha_4 = 0, \alpha_3 = 0, then by choosing x = 2\alpha_2, q = 1, r = 0, s = 0, t = -\alpha_1, we have the representative \langle \nabla_2 \rangle;
{\rm{(b)}}\ if \alpha_6 = 0, \alpha_4 = 0, \alpha_3\neq0, then by choosing x = \alpha_3, q = \alpha_2\alpha_3^2, r = 0, s = 0, t = -\frac{\alpha_1\alpha_3}{2\alpha_2}, we have the representative \langle \nabla_2+\nabla_3 \rangle;
{\rm{(c)}}\ if \alpha_6 = 0, \alpha_4\neq0, then by choosing x = \alpha_4, q = \alpha_2\alpha_4, r = -\frac{\alpha_2\alpha_3}{2}, s = 0, t = -\frac{\alpha_1\alpha_4}{2\alpha_2}, we have the representative \langle \nabla_2+\nabla_4 \rangle;
{\rm{(d)}}\ if \alpha_6\neq0, \alpha_3\alpha_6-\alpha_4^2 = 0, then by choosing x = \alpha_6, q = \alpha_2, r = -\frac{\alpha_2\alpha_4}{\alpha_6}, s = 0, t = -\frac{\alpha_1\alpha_6}{2\alpha_2}, we have the representative \langle \nabla_2+\nabla_6 \rangle;
{\rm{(e)}}\ if \alpha_6\neq0, \alpha_3\alpha_6-\alpha_4^2\neq0, then by choosing
we have the representative \langle \nabla_2+\nabla_3+\nabla_6 \rangle.
2. \alpha_8 = 0, \alpha_7 = 0, \alpha_5\neq0, then we have
{\rm{(a)}}\ if \alpha_6 = 0, \alpha_2 = 0, then by choosing
we have the representatives \langle \nabla_5 \rangle and \langle \nabla_1+\nabla_5 \rangle depending on whether \alpha_1 = 0 or not;
{\rm{(b)}}\ if \alpha_6 = 0, \alpha_2\neq0, then by choosing
we have the representatives \langle \nabla_2+\nabla_5 \rangle;
{\rm{(c)}}\ if \alpha_6\neq0, \alpha_5 = -\alpha_6, then we have the following subcases:
{\rm{(i)}}\ if \alpha_2 = 0, \alpha_4 = 0, \alpha_1 = 0, then we have the representative \langle \nabla_5-\nabla_6 \rangle;
{\rm{(ii)}}\ if \alpha_2 = 0, \alpha_4 = 0, \alpha_1\neq0, then by choosing
we have the representative \langle \nabla_1+\nabla_5-\nabla_6 \rangle;
{\rm{(iii)}}\ if \alpha_2 = 0, \alpha_4\neq0, \alpha_1 = 0, then by choosing
we have the representative \langle \nabla_4+\nabla_5-\nabla_6 \rangle;
{\rm{(iv)}}\ if \alpha_2 = 0, \alpha_4\neq0, \alpha_1\neq0, then by choosing
we have the representative \langle \nabla_1+\nabla_4+\nabla_5-\nabla_6 \rangle;
{\rm{(v)}}\ if \alpha_2\neq0, \alpha_4 = 0, then by choosing
we have the representative \langle \nabla_2+\nabla_5-\nabla_6 \rangle;
{\rm{(vi)}}\ if \alpha_2\neq0, \alpha_4\neq0, then by choosing
we have the representative \langle \nabla_2+\nabla_4+\nabla_5-\nabla_6 \rangle.
(d) if \alpha_6\neq0, \alpha_5\neq-\alpha_6, then we have the following subcases:
{\rm{(i)}}\ if \alpha_2 = 0, \alpha_1 = 0, then by choosing
we have the family of representatives \langle \nabla_5+\alpha\nabla_6 \rangle_{\alpha\neq0,-1}, which will be jointed with the cases (2a) and (2(c)i);
{\rm{(ii)}}\ if \alpha_2 = 0, \alpha_1\neq0, then by choosing
we have the family of representatives \langle \nabla_1+\nabla_5+\alpha\nabla_6 \rangle_{\alpha\neq0,-1}, which will be jointed with the cases (2a) and (2(c)ii);
{\rm{(iii)}}\ if \alpha_2\neq0, then by choosing
we have the family of representatives \langle \nabla_2+\nabla_5+\alpha\nabla_6 \rangle_{\alpha\neq0,-1}, which will be jointed with the cases (2b) and (2(c)v).
3. \alpha_8 = 0, \alpha_7\neq0 then by choosing r = -\frac{\alpha_5}{\alpha_7}q, s = \frac{\alpha_5\alpha_6-\alpha_4\alpha_7}{\alpha_7^2}q, we have \alpha_4^* = \alpha_5^* = 0. Therefore, we can suppose that \alpha_4 = 0, \alpha_5 = 0, thus we have
{\rm{(a)}}\ if \alpha_2 = 0, \alpha_1 = 0, \alpha_3 = 0, then we have the representatives \langle \nabla_7 \rangle and \langle \nabla_6+\nabla_7\rangle depending on whether \alpha_6 = 0 or not;
{\rm{(b)}}\ if \alpha_2 = 0, \alpha_1 = 0, \alpha_3\neq0, then by choosing x = \sqrt[3]{\frac{\alpha_3}{\alpha_7}}, q = 1, r = 0, s = 0, t = 0, we have the family of representatives \langle \nabla_3+\alpha\nabla_6+\nabla_7\rangle;
{\rm{(c)}}\ if \alpha_2 = 0, \alpha_1\neq0, \alpha_3 = 0, then we have the representatives \langle \nabla_1+\nabla_7\rangle and \langle \nabla_1+\nabla_6+\nabla_7\rangle depending on whether \alpha_6 = 0 or not;
{\rm{(d)}}\ if \alpha_2 = 0, \alpha_1\neq0, \alpha_3\neq0, then by choosing
we have the family of representatives \langle \nabla_1+\nabla_3+\alpha\nabla_6+\nabla_7\rangle;
{\rm{(e)}}\ if \alpha_2\neq0, \alpha_3 = 0, then by choosing q = \frac{\alpha_2}{\alpha_7}, r = 0, s = 0, t = -\frac{\alpha_1}{2\alpha_2}x, we have the representatives \langle \nabla_2+\nabla_7 \rangle and \langle \nabla_2+\nabla_6+\nabla_7\rangle depending on whether \alpha_6 = 0 or not;
{\rm{(f)}}\ if \alpha_2\neq0, \alpha_3\neq0, then by choosing x = \sqrt[3]{\frac{\alpha_3}{\alpha_7}}, q = \frac{\alpha_2}{\alpha_7}, r = 0, s = 0, t = -\frac{\alpha_1\sqrt[3]{\alpha_3}}{2\alpha_2\sqrt[3]{\alpha_7}} we have the family of representatives \langle \nabla_2+\nabla_3+\alpha\nabla_6+\nabla_7\rangle.
4. \alpha_8\neq0 then by choosing r = -\frac{\alpha_7}{\alpha_8}q, s = \frac{\alpha_7^2-\alpha_5\alpha_8}{\alpha_8^2}q, t = -\frac{\alpha_2}{\alpha_8}x, we have \alpha_2^* = \alpha_5^* = \alpha_7^* = 0. Therefore, we can suppose that \alpha_2 = 0, \alpha_5 = 0, \alpha_7 = 0, then we have
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_3 = 0, \alpha_4 = 0, then we have the representatives \langle \nabla_8 \rangle and \langle \nabla_6+\nabla_8 \rangle depending on whether \alpha_6 = 0 or not;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_3 = 0, \alpha_4\neq0, then by choosing x = \sqrt[3]{\frac{\alpha_4}{\alpha_8}}, q = 1, r = 0, s = 0, t = 0, we have the family of representatives \langle \nabla_4+\alpha\nabla_6+\nabla_8 \rangle;
{\rm{(c)}}\ if \alpha_1 = 0, \alpha_3\neq0, then by choosing x = \sqrt[4]{\frac{\alpha_3}{\alpha_8}}, q = 1, r = 0, s = 0, t = 0, we have the family of representatives \langle \nabla_3+\alpha\nabla_4+\beta\nabla_6+\nabla_8 \rangle;
{\rm{(d)}}\ if \alpha_1\neq0, \alpha_3 = 0, \alpha_4 = 0, then we have the representatives \langle \nabla_1+\nabla_8 \rangle and \langle \nabla_1+\nabla_6+\nabla_8 \rangle depending on whether \alpha_6 = 0 or not;
{\rm{(e)}}\ if \alpha_1\neq0, \alpha_3 = 0, \alpha_4\neq0, then by choosing x = \sqrt[3]{\frac{\alpha_4}{\alpha_8}}, q = \sqrt[6]{\frac{\alpha_1^3}{\alpha_4^2\alpha_8}}, r = 0, s = 0, t = 0, we have the family of representative \langle \nabla_1+\nabla_4+\alpha\nabla_6+\nabla_8 \rangle;
{\rm{(f)}}\ if \alpha_1\neq0, \alpha_3\neq0, then by choosing x = \sqrt[4]{\frac{\alpha_3}{\alpha_8}}, q = \frac{\sqrt{\alpha_1}}{\sqrt[4]{\alpha_3\alpha_8}}, r = 0, s = 0, t = 0, we have the family of representative \langle \nabla_1+\nabla_3+\alpha\nabla_4+\beta\nabla_6+\nabla_8 \rangle.
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
3.10. 1 -dimensional central extensions of {\mathbf N}_{15}^{4*} .
Here we will collect all information about {\mathbf N}_{15}^{4*}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{8}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4*}_{15}) . Since
we have
We are interested in (\alpha_2,\alpha_5,\alpha_7,\alpha_8)\neq(0,0,0,0) . Let us consider the following cases:
1.\ \alpha_8 = 0, \alpha_7 = 0, \alpha_5 = 0, then \alpha_2\neq0 and we have
{\rm{(a)}}\ if \alpha_6 = 0, \alpha_4 = 0, then by choosing x = 2\alpha_2, r = 4\alpha_2\alpha_3, s = 0, t = -\alpha_1, we have the representative \langle \nabla_2 \rangle;
{\rm{(b)}}\ if \alpha_6 = 0, \alpha_4\neq0, \alpha_2 = 2\alpha_4, then we have the representatives \langle 2\nabla_2+\nabla_4 \rangle and \langle 2\nabla_2+\nabla_3+\nabla_4 \rangle depending on whether \alpha_3 = 0 or not;
{\rm{(c)}}\ if \alpha_6 = 0, \alpha_4\neq0, \alpha_2\neq2\alpha_4, then by choosing x = \alpha_2-2\alpha_4, r = \alpha_3(\alpha_2-2\alpha_4), s = 0, t = \frac{\alpha_1(2\alpha_4-\alpha_2)}{2\alpha_2}, we have the family of representatives \langle \nabla_2+\alpha\nabla_4 \rangle_{\alpha\neq0,\frac{1}{2}}, which will be jointed with representatives from the cases (1a) and (1b);
{\rm{(d)}}\ if \alpha_6\neq0, then by choosing x = \frac{\alpha_2}{\alpha_6}, r = -\frac{\alpha_2^2\alpha_4}{\alpha_6^3}, s = 0, t = -\frac{\alpha_1}{2\alpha_6}, we have the representative \langle \nabla_2+\alpha\nabla_3+\nabla_6 \rangle.
2. \alpha_8 = 0, \alpha_7 = 0, \alpha_5\neq0 then we have
{\rm{(a)}}\ if \alpha_5\neq-\alpha_6, then we have the following subcases:
{\rm{(i)}}\ if \alpha_2 = 0, \alpha_1 = 0, then by choosing
we have the family of representatives \langle \nabla_5+\alpha\nabla_6 \rangle_{\alpha\neq-1} ;
{\rm{(ii)}}\ if \alpha_2 = 0, \alpha_1\neq0, then by choosing
we have the family of representatives \langle \nabla_1+\nabla_5+\alpha\nabla_6 \rangle_{\alpha\neq-1} ;
{\rm{(iii)}}\ if \alpha_2\neq0, then by choosing
we have the family of representatives \langle \nabla_2+\nabla_5+\alpha\nabla_6 \rangle_{\alpha\neq-1} .
(b) if \alpha_6 = -\alpha_5, then we have the following subcases:
{\rm{(i)}}\ if \alpha_4 = 0, \alpha_2 = 0, \alpha_1 = 0, then we have the representative \langle \nabla_5-\nabla_6 \rangle, which will be jointed with the family from the case (2(a)i);
{\rm{(ii)}}\ if \alpha_4 = 0, \alpha_2 = 0, \alpha_1\neq0, then by choosing x = \sqrt[4]{\frac{\alpha_1}{\alpha_5}}, r = 0, s = -\frac{\alpha_3\sqrt{\alpha_1}}{2\alpha_5\sqrt{\alpha_4}}, t = 0, we have the representative \langle \nabla_1+\nabla_5-\nabla_6 \rangle, which will be jointed with the family from the case (2(a)ii);
{\rm{(iii)}}\ if \alpha_4 = 0, \alpha_2\neq0, then by choosing x = \frac{\alpha_2}{\alpha_5}, r = 0, s = -\frac{\alpha^2_2\alpha_3}{2\alpha^3_5}, t = -\frac{\alpha_1}{2\alpha_5}, we have the representative \langle \nabla_2+\nabla_5-\nabla_6 \rangle, which will be jointed with the family from the case (2(a)iii);
{\rm{(iv)}}\ if \alpha_4\neq0, then by choosing x = \frac{\alpha_4}{\alpha_5}, s = -\frac{\alpha_3 \alpha_4^2}{ 2 \alpha_5^3}, r = 0, we have the families of representatives
depending on \alpha_2 = 0 or not.
3. \alpha_8 = 0, \alpha_7\neq0, then by choosing
we have \alpha_3^* = \alpha_4^* = \alpha_5^* = 0. Therefore, we can suppose that \alpha_3 = 0, \alpha_4 = 0, \alpha_5 = 0, and we have
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_2 = 0, then we have the representatives \langle \nabla_7 \rangle and \langle \nabla_6+\nabla_7 \rangle depending on whether \alpha_6 = 0 or not;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_2\neq0, then by choosing x = \sqrt{\alpha_2\alpha_7^{-1}}, r = 0, s = 0, t = 0, we have the family of representative \langle \nabla_2+\alpha\nabla_6+\nabla_7 \rangle;
{\rm{(c)}}\ if \alpha_1\neq0, then by choosing x = \sqrt[5]{\frac{\alpha_1}{\alpha_7}}, r = 0, s = 0, t = 0, we have the family of representative \langle \nabla_1+\alpha\nabla_2+\beta\nabla_6+\nabla_7 \rangle.
4. \alpha_8\neq0, then by choosing r = -\frac{\alpha_7}{\alpha_8}x^2, t = -\frac{\alpha_2}{\alpha_8}x, s = -\frac{\alpha_5x^2+\alpha_7r}{\alpha_8} we have \alpha_2^* = \alpha_5^* = \alpha_7^* = 0. Therefore, we can suppose that \alpha_2 = 0, \alpha_5 = 0, \alpha_7 = 0, then we have
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_3 = 0, \alpha_4 = 0, then we have the representatives \langle \nabla_8 \rangle and \langle \nabla_6+\nabla_8 \rangle depending on whether \alpha_6 = 0 or not;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_3 = 0, \alpha_4\neq0, then by choosing x = \sqrt[3]{{\alpha_4}{\alpha_8^{-1}}}, r = 0, s = 0, t = 0, we have the family of representative \langle \nabla_4+\alpha\nabla_6+\nabla_8 \rangle;
{\rm{(c)}}\ if \alpha_1 = 0, \alpha_3\neq0, then by choosing x = \sqrt[4]{{\alpha_3}{\alpha_8^{-1}}}, r = 0, s = 0, t = 0, we have the family of representative \langle \nabla_3+\alpha\nabla_4+\beta\nabla_6+\nabla_8 \rangle;
{\rm{(d)}}\ if \alpha_1\neq0, then by choosing x = \sqrt[6]{{\alpha_1}{\alpha_8^{-1}}}, r = 0, s = 0, t = 0, we have the family of representative \langle \nabla_1+\alpha\nabla_3+\beta\nabla_4+\gamma\nabla_6+\nabla_8 \rangle.
Summarizing all cases we have the following distinct orbits:
which gives the following new algebras:
3.11. 1 -dimensional central extensions of {\mathbf N}_{16}^{4*} .
Here we will collect all information about {\mathbf N}_{16}^{4*}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{8}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4*}_{16}) . Since
in the case \phi = \phi_1 , we have
and on the opposite case, for \phi = \phi_2, we have
We are interested in (\alpha_2,\alpha_5,\alpha_7,\alpha_8)\neq(0,0,0,0) . Let us consider the following cases:
1.\ \alpha_8 = 0, \alpha_7 = 0, \alpha_5 = 0, then \alpha_2\neq0 and
{\rm{(a)}}\ if \alpha_4\neq 0, then by choosing \phi = \phi_1, x = \alpha_4 \alpha_2^{-1}, t = -\frac{ \alpha_1 \alpha_4 }{2 \alpha_2^{2}}, we have the family of representatives \langle \nabla_2+\alpha\nabla_3+\nabla_4 +\beta \nabla_6 \rangle;
{\rm{(b)}}\ if \alpha_4 = 0,\alpha_3\neq0, then by choosing \phi = \phi_1, x = \sqrt{ \alpha_3 \alpha_2^{-1}}, t = -\frac{ \alpha_1 \sqrt{ \alpha_3}}{2 \sqrt{\alpha_2^3}}, we have the family of representatives \langle \nabla_2+\nabla_3+\alpha \nabla_6 \rangle;
{\rm{(c)}}\ if \alpha_4 = 0,\alpha_3 = 0, then by choosing \phi = \phi_1, x = 2 \alpha_2, t = - \alpha_1, s = 0, we have the family of representatives \langle \nabla_2+ \alpha \nabla_6 \rangle.
2. \alpha_8 = 0, \alpha_7 = 0, \alpha_5\neq0 and
{\rm{(a)}}\ if \alpha_2\neq 0,\alpha_4\neq 0, then by choosing
we have the following family of representatives
{\rm{(b)}}\ if \alpha_2\neq 0,\alpha_4 = 0, then by choosing
we have the following family of representatives \langle \alpha \nabla_2+ \nabla_5+ \beta \nabla_6 \rangle_{\alpha\neq0};
{\rm{(c)}}\ if \alpha_2 = 0, then by choosing \phi = \phi_2, y = 1, t = 0, s = 0, we have the representative with \alpha_5^* = 0 and \alpha_2^*\neq0, which was considered above.
3. \alpha_8 = 0, \alpha_7\neq0, then we have
{\rm{(a)}}\ if \alpha_2 = 0, \alpha_5 = 0, \alpha_1 = 0, \alpha_3 = 0, then we have the representatives \langle \nabla_7 \rangle and \langle \nabla_6+\nabla_7\rangle depending on whether \alpha_6 = 0 or not;
{\rm{(b)}}\ if \alpha_2 = 0, \alpha_5 = 0, \alpha_1\neq0, then by choosing
we have the family of representatives \langle \nabla_1+\alpha\nabla_3+\beta\nabla_6+\nabla_7\rangle;
{\rm{(c)}}\ if \alpha_2\neq0, then by choosing
we have the family of representatives \langle \nabla_2+\alpha\nabla_3+\beta\nabla_5+\gamma\nabla_6+\nabla_7\rangle.
4. \alpha_8\neq0, then by choosing \phi = \phi_1, t = -\frac{\alpha_2}{\alpha_8}x, s = -\frac{\alpha_5}{\alpha_8}x, we have \alpha_2^* = \alpha_5^* = 0. Now we can suppose that \alpha_2 = 0, \alpha_5 = 0 and we have
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_3 = 0, \alpha_4 = 0, \alpha_6 = 0, then we have the representatives \langle \nabla_8\rangle and \langle \nabla_7+\nabla_8\rangle depending on whether \alpha_7 = 0 or not;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_3 = 0, \alpha_4 = 0, \alpha_6\neq0, then by choosing \phi = \phi_1, x = \sqrt{{\alpha_6}{\alpha_8^{-1}}}, s = 0, t = 0, we have the family of representative \langle \nabla_6+\alpha\nabla_7+\nabla_8\rangle;
{\rm{(c)}}\ if \alpha_1 = 0, \alpha_3 = 0, \alpha_4\neq0, then by choosing \phi = \phi_1, x = \sqrt[3]{{\alpha_4}{\alpha_8^{-1}}}, s = 0, t = 0, we have the family of representatives \langle \nabla_4+\alpha\nabla_6+\beta\nabla_7+\nabla_8\rangle;
{\rm{(d)}}\ if \alpha_1\neq0, then by choosing \phi = \phi_1, x = \sqrt[4]{{\alpha_1}{\alpha_8^{-1}}}, s = 0, t = 0, we have the family of representatives \langle \nabla_1+\alpha\nabla_3+\beta\nabla_4+\gamma\nabla_6+\mu\nabla_7+\nabla_8\rangle.
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
3.12. 1 -dimensional central extensions of {\mathbf N}_{17}^{4*} .
Here we will collect all information about {\mathbf N}_{17}^{4*}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{8}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4*}_{17}) . Since
then in the case \phi = \phi_1, we have
and in the opposite case \phi = \phi_2, we have
We are interested in (\alpha_3,\alpha_6,\alpha_7,\alpha_8)\neq(0,0,0,0) . Let us consider the following cases:
1.\ \alpha_8 = 0, \alpha_7 = 0, \alpha_6 = 0, then \alpha_3\neq0 and choosing \phi = \phi_1, t = -\frac{\alpha_1}{2\alpha_3}x, we get \alpha_1^* = 0. Now consider the following subcases:
{\rm{(a)}}\ if \alpha_2 = 0, \alpha_4 = 0, \alpha_5 = 0, then we have the representative \langle \nabla_3 \rangle;
{\rm{(b)}}\ if \alpha_2 = 0, \alpha_4 = 0, \alpha_5\neq0, then by choosing \phi = \phi_1, x = \sqrt{\frac{\alpha_5}{\alpha_3}}, q = 1, s = 0, t = -\frac{\alpha_1\sqrt{\alpha_5}}{2\alpha_3\sqrt{\alpha_3}}, we have the representative \langle \nabla_3+\nabla_5 \rangle;
{\rm{(c)}}\ if \alpha_2 = 0, \alpha_4\neq0, then by choosing \phi = \phi_1, x = \sqrt[3]{\frac{\alpha_4}{\alpha_3}}, q = 1, s = 0, t = -\frac{\alpha_1\sqrt[3]{\alpha_5}}{2\alpha_3\sqrt[3]{\alpha_3}}, we have the representative \langle \nabla_3+\nabla_4+\alpha\nabla_5 \rangle;
{\rm{(d)}}\ if \alpha_2\neq0, \alpha_4 = 0, \alpha_5 = 0, then by choosing \phi = \phi_1, x = \alpha_2, q = \frac{1}{\alpha_3}, s = 0, t = -\frac{\alpha_1\alpha_2}{2\alpha_3}, we have the representative \langle \nabla_2+\nabla_3 \rangle;
{\rm{(e)}}\ if \alpha_2\neq0, \alpha_4 = 0, \alpha_5\neq0, then by choosing \phi = \phi_1, x = \sqrt{\frac{\alpha_5}{\alpha_3}}, q = \sqrt{\frac{\alpha_2^2}{\alpha_3\alpha_5}}, s = 0, t = -\frac{\alpha_1\sqrt{\alpha_5}}{2\alpha_3\sqrt{\alpha_3}}, we have the representative \langle \nabla_2+\nabla_3+\nabla_5 \rangle;
{\rm{(f)}}\ if \alpha_2\neq0, \alpha_4\neq0, then by choosing \phi = \phi_1, x = \sqrt[3]{{\alpha_4}{\alpha_3^{-3}}}, q = \sqrt[3]{{\alpha_2^3}{\alpha^{-2}_3\alpha_4^{-1}}}, we have the family of representative \langle \nabla_2+\nabla_3+\nabla_4+\alpha\nabla_5 \rangle.
2. \alpha_8 = 0, \alpha_7 = 0, \alpha_6\neq0, and \alpha_3 = 0 , then by choosing some suitable automorphism \phi_2 we have \alpha_3^*\neq0 which is the case considered above. Now we can suppose that \alpha_3\neq0, and choosing t = -\frac{\alpha_1}{2\alpha_3}x, s = -\frac{\alpha_4}{2\alpha_6}x, we have \alpha_1^* = 0, \alpha_4^* = 0. Therefore, we can suppose that \alpha_1 = 0, \alpha_4 = 0. Consider the following subcases:
{\rm{(a)}}\ \alpha_2 = 0, \alpha_5 = 0, then by choosing \phi = \phi_1, x = \alpha_6, q = \alpha_3, s = 0, t = 0, we have the representative \langle \nabla_3+\nabla_6 \rangle;
{\rm{(b)}}\ \alpha_2\neq0, then by choosing \phi = \phi_1, x = {\alpha_3^{-1}}{\sqrt{\alpha_2\alpha_6}}, q = \sqrt{\alpha_2\alpha_6^{-1}}, s = 0, t = 0, we have the family of representatives \langle \nabla_2+\nabla_3+\alpha\nabla_5+\nabla_6 \rangle.
3. \alpha_8 = 0, \alpha_7\neq0, then by choosing \phi = \phi_1, t = -{\alpha_2}{\alpha_7^{-1}}x, s = -{\alpha_5}{\alpha_7^{-1}}q, we have \alpha_2^* = 0, \alpha_5^* = 0. Therefore, we can suppose that \alpha_2 = 0, \alpha_5 = 0. Consider the following subcases:
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_4 = 0, \alpha_3 = 0, \alpha_6 = 0, then we have the representative \langle \nabla_7 \rangle ;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_4 = 0, \alpha_3 = 0, \alpha_6\neq0, then by choosing \phi = \phi_1, x = {\alpha_6}{\alpha_7^{-1}}, q = 1, s = 0, t = 0, we have the representative \langle \nabla_6+\nabla_7 \rangle ;
{\rm{(c)}}\ if \alpha_1 = 0, \alpha_4 = 0, \alpha_3\neq0, and \alpha_6 = 0, then by choosing some suitable automorphism \phi_2, we have \alpha_6^*\neq0 . Thus we can consider the case \alpha_6\neq0 and choosing \phi = \phi_1, x = {\alpha_6}{\alpha_7^{-1}}, q = {\alpha_3}{\alpha_7^{-1}}, s = 0, t = 0, we have the representative \langle \nabla_3+\nabla_6+\nabla_7 \rangle ;
{\rm{(d)}}\ if \alpha_1 = 0, \alpha_4\neq0, \alpha_3 = 0, \alpha_6 = 0, then by choosing \phi = \phi_1, x = 1, q = {\alpha_4}{\alpha_7^{-1}}, s = 0, t = 0, we have the representative \langle \nabla_4+\nabla_7 \rangle;
{\rm{(e)}}\ if \alpha_1 = 0, \alpha_4\neq0, \alpha_3 = 0, \alpha_6\neq0, then by choosing \phi = \phi_1, x = {\alpha_6}{\alpha_7}^{-1}, q = {\alpha_4\alpha_7^2}{\alpha_6^{-3}}, we have the representative \langle \nabla_4+\nabla_6+\nabla_7 \rangle;
{\rm{(f)}}\ if \alpha_1 = 0, \alpha_4\neq0, \alpha_3\neq0, then by choosing
we have the family of representatives \langle \nabla_3+\nabla_4+\alpha\nabla_6+\nabla_7 \rangle ;
{\rm{(g)}}\ if \alpha_1\neq0. In case of \alpha_4 = 0, choosing some suitable automorphism \phi_2, we have \alpha_4^*\neq0. Thus, we can suppose \alpha_4\neq0 , and choosing
we have the family of representatives \langle \nabla_1+\alpha\nabla_3+\nabla_4+\beta\nabla_6+\nabla_7 \rangle .
4. \alpha_8\neq0, then by choosing \phi = \phi_1, t = -\frac{\alpha_3}{\alpha_8}x, s = -\frac{\alpha_6}{\alpha_8}q, we have \alpha_3^* = 0, \alpha_6^* = 0. Consider the following cases:
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_4 = 0, \alpha_2 = 0, \alpha_5 = 0, then we have the representatives \langle \nabla_8 \rangle and \langle \nabla_7+\nabla_8 \rangle depending on whether \alpha_7 = 0 or not;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_4 = 0, \alpha_2 = 0, \alpha_5\neq0, then we have the representatives \langle \nabla_5+\nabla_8 \rangle and \langle \nabla_5+\nabla_7+\nabla_8 \rangle depending on whether \alpha_7 = 0 or not;
{\rm{(c)}}\ if \alpha_1 = 0, \alpha_4 = 0, \alpha_2\neq0. In case of \alpha_5 = 0, choosing some suitable automorphism \phi_2, we have \alpha_5^*\neq0 . Thus, we can suppose \alpha_5\neq0 , and choosing
we have the family of representatives \langle \nabla_2+\nabla_5+\alpha\nabla_7+\nabla_8 \rangle ;
{\rm{(d)}}\ if \alpha_1 = 0, \alpha_4\neq0, \alpha_2 = 0, \alpha_5 = 0, then we have the representatives \langle \nabla_4+\nabla_8 \rangle and \langle \nabla_4+\nabla_7+\nabla_8 \rangle depending on whether \alpha_7 = 0 or not;
{\rm{(e)}}\ if \alpha_1 = 0, \alpha_4\neq0, \alpha_2 = 0, \alpha_5\neq0 , then by choosing
we have the family of representatives \langle \nabla_4+\nabla_5+\alpha\nabla_7+\nabla_8 \rangle ;
{\rm{(f)}}\ if \alpha_1 = 0, \alpha_4\neq0, \alpha_2\neq0 , then by choosing
we have the family of representatives \langle \nabla_2+\nabla_4+\alpha\nabla_5+\beta\nabla_7+\nabla_8 \rangle ;
{\rm{(g)}}\ if \alpha_1\neq0 then by choosing some suitable automorphism \phi_2, we have \alpha_4^*\neq0 . Thus, we can suppose \alpha_4\neq0 , and choosing
we have the family of representatives
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
3.13. 1 -dimensional central extensions of {\mathbf N}_{18}^{4*} .
Here we will collect all information about {\mathbf N}_{18}^{4*}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{8}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4*}_{18}) . Since
we have
We are interested in (\alpha_3,\alpha_6,\alpha_7,\alpha_8)\neq(0,0,0,0) . Let us consider \phi = \phi_+ and the following cases:
1.\ \alpha_8 = 0, \alpha_7 = 0, \alpha_6 = 0, then \alpha_3\neq0 and choosing t = -\frac{\alpha_1}{2\alpha_3}x, we get \alpha_1^* = 0. Now consider the following subcases:
{\rm{(a)}}\ if \alpha_2 = 0, \alpha_4 = 0, \alpha_5 = 0, then we have the representative \langle \nabla_3 \rangle ;
{\rm{(b)}}\ if \alpha_2 = 0, \alpha_4 = 0, \alpha_5\neq0, then by choosing x = \sqrt{\frac{\alpha_5}{\alpha_3}}, s = 0, t = -\frac{\alpha_1\sqrt{\alpha_5}}{2\alpha_3\sqrt{\alpha_3}}, we have the representative \langle \nabla_3+\nabla_5 \rangle;
{\rm{(c)}}\ if \alpha_2 = 0, \alpha_4\neq0, then by choosing x = \sqrt[3]{\frac{\alpha_4}{\alpha_3}}, s = 0, t = -\frac{\alpha_1\sqrt[3]{\alpha_4}}{2\alpha_3\sqrt[3]{\alpha_3}}, we have the family of representatives \langle \nabla_3+\nabla_4+\alpha\nabla_5 \rangle;
{\rm{(d)}}\ if \alpha_2\neq0, then by choosing x = {\alpha_2}{\alpha_3^{-1}}, s = 0, t = -\frac{\alpha_1\alpha_2}{2\alpha^2_3}, we have the family of representatives \langle \nabla_2+\nabla_3+\alpha\nabla_4+\beta\nabla_5 \rangle.
2. \alpha_8 = 0, \alpha_7 = 0, \alpha_6\neq0, then by choosing s = -\frac{\alpha_4}{2\alpha_6}x, we have \alpha_4^* = 0. Consider the following cases:
{\rm{(a)}}\ \alpha_3 = 0, then we have two families of representatives \langle \alpha\nabla_1+\beta\nabla_2+\nabla_6 \rangle and \langle \alpha\nabla_1+\beta\nabla_2+\nabla_5+\nabla_6 \rangle depending on whether \alpha_5 = 0 or not;
{\rm{(b)}}\ \alpha_3\neq0 then by choosing x = \frac{\alpha_6}{\alpha_3}, s = -\frac{\alpha_4}{2\alpha_6}, t = -\frac{\alpha_1\alpha_6}{2\alpha_3^2}, we have the family of representatives \langle \alpha\nabla_2+\nabla_3+\beta\nabla_5+\nabla_6 \rangle.
3. \alpha_8 = 0, \alpha_7\neq0, then by choosing t = -{\alpha_2}{\alpha_7^{-1}}x, s = -{\alpha_5}{\alpha_7^{-1}}, we have \alpha_2^* = 0, \alpha_5^* = 0. Thus, we can suppose that \alpha_2 = 0, \alpha_5 = 0 and now consider the following cases:
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_4 = 0, \alpha_6 = 0, then we have the family of representatives \langle \alpha\nabla_3+\nabla_7 \rangle;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_4 = 0, \alpha_6\neq0, then by choosing x = {\alpha_6}{\alpha_7^{-1}}, s = 0, t = 0, we have the family of representatives \langle \alpha\nabla_3+\nabla_6+\nabla_7 \rangle;
{\rm{(c)}}\ if \alpha_1 = 0, \alpha_4\neq0, then by choosing x = \sqrt[3]{{\alpha_4}{\alpha_7^{-1}}}, s = 0, t = 0, we have the family of representatives \langle \alpha\nabla_3+\nabla_4+\beta\nabla_6+\nabla_7 \rangle;
{\rm{(d)}}\ if \alpha_1\neq0, then by choosing x = {\alpha_1}{\alpha_7^{-1}}, s = 0, t = 0, we have the family of representatives \langle \nabla_1+\alpha\nabla_3+\beta\nabla_4+\gamma\nabla_6+\nabla_7 \rangle.
4. \alpha_8\neq0, then by choosing t = -\frac{\alpha_3}{\alpha_8}x, s = -\frac{\alpha_6}{\alpha_8}, we have \alpha_3^* = 0, \alpha_6^* = 0. Thus, we can suppose that \alpha_3 = 0, \alpha_6 = 0. Consider the following cases:
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_2 = 0, \alpha_4 = 0, \alpha_5 = 0, then we have the representatives \langle \nabla_8 \rangle and \langle \nabla_7+\nabla_8 \rangle depending on whether \alpha_7 = 0 or not;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_2 = 0, \alpha_4 = 0, \alpha_5\neq0, then by choosing x = \sqrt[3]{{\alpha_5}{\alpha_8^{-1}}}, s = 0, t = 0, we have the family of representatives \langle \nabla_5+\alpha\nabla_7+\nabla_8 \rangle;
{\rm{(c)}}\ if \alpha_1 = 0, \alpha_2 = 0, \alpha_4\neq0 then by choosing x = \sqrt[4]{{\alpha_4}{\alpha_8^{-1}}}, s = 0, t = 0, we have the family of representatives \langle \nabla_4+\alpha\nabla_5+\beta\nabla_7+\nabla_8 \rangle;
{\rm{(d)}}\ if \alpha_1 = 0, \alpha_2\neq0, then by choosing x = \sqrt{{\alpha_2}{\alpha_8^{-1}}}, s = 0, t = 0, we have the family of representatives \langle \nabla_2+\alpha\nabla_4+\beta\nabla_5+\gamma\nabla_7+\nabla_8 \rangle;
{\rm{(e)}}\ if \alpha_1\neq0, then by choosing x = \sqrt{{\alpha_1}{\alpha_8}^{-1}}, s = 0, t = 0, we have the family of representatives \langle \nabla_1+\alpha\nabla_2+\beta\nabla_4+\gamma\nabla_5+\mu\nabla_7+\nabla_8 \rangle .
Summarizing all cases, we have the following distinct orbits:
which gives the following new algebras:
3.14. 1 -dimensional central extensions of {\mathbf N}_{19}^{4*} .
Here we will collect all information about {\mathbf N}_{19}^{4*}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{8}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4*}_{19}) . Since
then, in the case \phi = \phi_1^{x = 1,q = 1}, we have
For define the main families of representatives, we will use \phi = \phi_1^{x = 1,q = 1} and for find equal orbits we will use other automorphisms. We are interested in
Let us consider the following cases:
1.\ if \alpha_8 = 0, \alpha_7 = 0, \alpha_6 = 0, then \alpha_3\neq0 and choosing t = -\frac{\alpha_1}{2\alpha_3}, we have the family of representatives \langle \alpha\nabla_2+\nabla_3+\beta\nabla_4+\gamma\nabla_5 \rangle;
2.\ if \alpha_8 = 0, \alpha_7 = 0, \alpha_6\neq0 and \alpha_3 = 0, then by choosing some suitable automorphism \phi_2 we have \alpha_3^*\neq0 , \alpha_6^* = 0, which is the case considered above;
3.\ if \alpha_8 = 0, \alpha_7 = 0, \alpha_6\neq0, \alpha_3\neq0, then by choosing t = -\frac{\alpha_1}{2\alpha_3}, s = \frac{\alpha_4}{2\alpha_6}, we have the family of representatives \langle \alpha\nabla_2+\beta\nabla_3+\gamma\nabla_5+\nabla_6 \rangle_{\beta\neq0} ;
4.\ if \alpha_8 = 0, \alpha_7\neq0, then by choosing t = -{\alpha_2}{\alpha_7}^{-1}, s = -{\alpha_5}{\alpha_7}^{-1}, we have the family of representatives \langle \alpha\nabla_1+\beta\nabla_3+\gamma\nabla_4+\mu\nabla_6+\nabla_7 \rangle;
5.\ if \alpha_8\neq0, then by choosing t = -{\alpha_3}{\alpha_8}^{-1}, s = -{\alpha_6}{\alpha_8}^{-1}, we have the family of representatives \langle \alpha\nabla_1+\beta\nabla_2+\gamma\nabla_4+\mu\nabla_5+\nu\nabla_7+\nabla_8 \rangle.
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
4.
Central extensions of nilpotent non- \mathfrak{CCD} -algebras.
4.1. 1 -dimensional central extensions of {\mathbf N}_{01}^{4} .
Here we will collect all information about {\mathbf N}_{01}^{4}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{7}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4}_{01}) . Since
we have
We are interested in (\alpha_2,\alpha_4,\alpha_6,\alpha_7)\neq(0,0,0,0) and consider following cases:
1.\ \alpha_7 = \alpha_6 = \alpha_4 = 0, then \alpha_2\neq0, and we have the following subcases:
{\rm{(a)}}\ if \alpha_5 = -\alpha_2, then we have
{\rm{(i)}}\ if \alpha_1 = 0, \alpha_3 = 0, then we have the representative \langle \nabla_2-\nabla_5 \rangle;
{\rm{(ii)}}\ if \alpha_1 = 0, \alpha_3\neq0, then by choosing x = \sqrt{{\alpha_3}{\alpha_2}^{-1}}, z = 0, t = 0, we have the representative \langle \nabla_2+\nabla_3-\nabla_5 \rangle;
{\rm{(iii)}}\ if \alpha_1\neq0, then by choosing x = \sqrt{{\alpha_1}{\alpha_2}^{-1}}, z = 0, t = 0, we have the family of representatives \langle \nabla_1+\nabla_2+\alpha\nabla_3-\nabla_5 \rangle;
(b) if \alpha_5\neq-\alpha_2, then by choosing z = -\frac{\alpha_1}{\alpha_5+\alpha_2}x, t = 0, we have the family of representatives \langle \nabla_2+\alpha\nabla_5 \rangle_{\alpha\neq-1} and \langle \nabla_2+\nabla_3+\alpha\nabla_5 \rangle_{\alpha\neq-1} depending on whether \alpha_3 = 0 or not, which will be jointed with the cases (1(a)i) and (1(a)ii).
2. \alpha_7 = 0, \alpha_6 = 0, \alpha_4\neq0, then we have the following subcases:
{\rm{(a)}}\ if \alpha_5 = -\alpha_2, \alpha_1 = 0,
{\rm{(i)}}\ if \alpha_3 = 0, then we have the representatives \langle \nabla_4 \rangle and \langle \nabla_2+\nabla_4-\nabla_5 \rangle depending on whether \alpha_2 = 0 or not;
{\rm{(ii)}}\ if \alpha_3\neq0, then by choosing x = \sqrt[3]{{\alpha_3}{\alpha_4}^{-1} }, we have the family of representatives \langle \alpha\nabla_2+\nabla_3+ \nabla_4-\alpha\nabla_5 \rangle;
(b) if \alpha_5 = -\alpha_2, \alpha_1\neq0, then by choosing x = \sqrt[3]{{\alpha_1}{\alpha_4}^{-1} }, we have the family of representatives \langle \nabla_1+\alpha\nabla_2+\beta\nabla_3+ \nabla_4-\alpha\nabla_5 \rangle;
(c) if \alpha_5\neq-\alpha_2, then we have
{\rm{(i)}}\ if \alpha_3 = 0, \alpha_2 = 0, then by choosing x = \frac{\alpha_5}{\alpha_4}, z = -\frac{\alpha_1\alpha_5}{\alpha_4(\alpha_2+\alpha_5)}, t = 0, we have the representative \langle \nabla_4+\nabla_5 \rangle;
{\rm{(ii)}}\ if \alpha_3 = 0, \alpha_2\neq0, then by choosing x = \frac{\alpha_2}{\alpha_4}, z = -\frac{\alpha_1\alpha_2}{\alpha_4(\alpha_2+\alpha_5)}, t = 0, we have the family of representatives \langle \nabla_2+\nabla_4+\alpha\nabla_5 \rangle_{\alpha\neq-1}, which will be jointed with a representative from the case (2(a)i);
{\rm{(iii)}}\ if \alpha_3\neq0, then by choosing x = \sqrt[3]{{\alpha_3}{\alpha_4}^{-1}}, z = -\frac{\alpha_1\sqrt[3]{\alpha_3}}{(\alpha_2+\alpha_5)\sqrt[3]{\alpha_4}}, t = 0, we have the family of representatives \langle \alpha\nabla_2+\nabla_3+\nabla_4+\beta\nabla_5 \rangle_{\beta\neq-\alpha}, which will be jointed with the family from the case (2(a)i).
3. \alpha_7 = 0, \alpha_6\neq0, then we consider the following subcases:
{\rm{(a)}}\ if \alpha_3 = 0, \alpha_4 = 0, then choosing z = -\frac{\alpha_2}{\alpha_6}x, t = -\frac{\alpha_1x+\alpha_5z}{\alpha_6}, we have representatives \langle \nabla_6 \rangle and \langle \nabla_5+\nabla_6 \rangle depending on whether \alpha_5 = 2\alpha_2 or not;
{\rm{(b)}}\ if \alpha_3 = 0, \alpha_4\neq0, then by choosing x = \frac{\alpha_4}{\alpha_6}, z = -\frac{\alpha_2\alpha_4}{\alpha^2_6}, t = \frac{\alpha_4(\alpha_2\alpha_5-\alpha_1\alpha_6)}{\alpha^3_6}, we have the family of representatives \langle \nabla_4+\alpha\nabla_5+\nabla_6 \rangle;
{\rm{(c)}}\ if \alpha_3\neq0, then by choosing x = \sqrt[4]{\frac{\alpha_3}{\alpha_6}}, z = -\frac{\alpha_2\sqrt[4]{\alpha_3}}{\alpha_6\sqrt[4]{\alpha_6}}, t = \frac{(\alpha_2\alpha_5-\alpha_1\alpha_6)\sqrt[4]{\alpha_3}}{\alpha_6^2\sqrt[4]{\alpha_6}}, we have the family of representatives \langle \nabla_3+\alpha\nabla_4+\beta\nabla_5+\nabla_6 \rangle.
4. \alpha_7\neq0, then by choosing z = -\frac{\alpha_6}{\alpha_7}x, t = \frac{\alpha_6^2+\alpha_2\alpha_7}{\alpha^2_7}x, we have \alpha_2^* = 0, \alpha_6^* = 0. Thus, we can suppose that \alpha_2 = 0, \alpha_6 = 0 and now consider following subcases:
{\rm{(a)}}\ \alpha_1 = 0, \alpha_3 = 0, \alpha_4 = 0, then we have representatives \langle \nabla_7 \rangle and \langle \nabla_5+\nabla_7 \rangle depending on whether \alpha_5\alpha_7-\alpha^2_6 = 0 or not;
{\rm{(b)}}\ \alpha_1 = 0, \alpha_3 = 0, \alpha_4\neq0, then by choosing x = \sqrt[3]{{\alpha_4}{\alpha_7}^{-1}}, we have the family of representatives \langle \nabla_4+\alpha\nabla_5+\nabla_7 \rangle;
{\rm{(c)}}\ \alpha_1 = 0, \alpha_3\neq0, then by choosing x = \sqrt[6]{{\alpha_3}{\alpha_7}^{-1}}, we have the family of representatives \langle \nabla_3+\alpha\nabla_4+\beta\nabla_5+\nabla_7 \rangle;
{\rm{(d)}}\ \alpha_1\neq0, then by choosing x = \sqrt[6]{{\alpha_1}{\alpha_7^{-3}}}, we have the family of representatives \langle \nabla_1+\alpha\nabla_3+\beta\nabla_4+\gamma\nabla_5+\nabla_7 \rangle.
Summarizing all cases, we have the following distinct orbits:
Hence, we have the following new algebras:
4.2. 1 -dimensional central extensions of {\mathbf N}_{02}^{4} .
Here we will collect all information about {\mathbf N}_{02}^{4}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{7}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4}_{02}) . Since
we have
We are interested in (\alpha_1,\alpha_4,\alpha_6,\alpha_7)\neq(0,0,0,0) and consider following cases:
1.\ if \alpha_7 = \alpha_6 = \alpha_4 = 0, then \alpha_1\neq0, and we have
{\rm{(a)}}\ if \alpha_5 = -\alpha_1 , then we have the family of representatives
{\rm{(b)}}\ if \alpha_5\neq-\alpha_1 , then by choosing z = -\frac{\alpha_3}{\alpha_1+\alpha_5}, t = 0, we have the family of representatives \langle \nabla_1+\alpha\nabla_2+\beta\nabla_5 \rangle_{\beta\neq-1};
2. if \alpha_7 = 0, \alpha_6 = 0, \alpha_4\neq0, then by choosing z = -\frac{\alpha_2}{4\alpha_4}, t = 0, we have the family of representatives \langle \alpha\nabla_1+\beta\nabla_3+\nabla_4+\gamma\nabla_5 \rangle;
3. if \alpha_7 = 0, \alpha_6\neq0, then by choosing
we have the family of representatives \langle \alpha\nabla_2+\beta\nabla_4+\gamma\nabla_5+\nabla_6 \rangle;
4. if \alpha_7\neq0, then by choosing z = -{\alpha_6}{\alpha_7}^{-1}, t = ({\alpha^2_6-\alpha_1\alpha_7}){\alpha^{-2}_7}, we have the family of representatives \langle \alpha\nabla_2+\beta\nabla_3+\gamma\nabla_4+\mu\nabla_5+\nabla_7 \rangle.
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
4.3. 1 -dimensional central extensions of {\mathbf N}_{03}^{4} .
Here we will collect all information about {\mathbf N}_{03}^{4}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{7}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4}_{03}) . Since
we have
We are interested in (\alpha_2,\alpha_5,\alpha_6,\alpha_7)\neq(0,0,0,0) and consider following cases:
1.\ \alpha_7 = \alpha_6 = \alpha_5 = 0, then \alpha_2\neq0, and we have the following subcases:
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_3 = 0, then we have the representatives \langle \nabla_2 \rangle and \langle \nabla_2+\nabla_4 \rangle depending on whether \alpha_4 = 0 or not;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_3\neq0, then by choosing x = \sqrt[3]{{\alpha_3}{\alpha_2}^{-1}}, t = 0, we have the family of representatives \langle \nabla_2+\nabla_3+\alpha\nabla_4 \rangle;
{\rm{(c)}}\ if \alpha_1\neq0, then by choosing x = \sqrt[3]{{\alpha_1}{\alpha_2^{-1}}}, t = 0, we have the family of representatives \langle \nabla_1+\nabla_2+\alpha\nabla_3+\beta\nabla_4 \rangle.
2. \alpha_7 = 0, \alpha_6 = 0, \alpha_5\neq0, then we have the following subcases:
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_2 = 0, \alpha_3 = 0, then we have the representatives \langle \nabla_5 \rangle and \langle \nabla_4+\nabla_5 \rangle depending on whether \alpha_5 = 0 or not;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_2 = 0, \alpha_3\neq0, then by choosing x = \sqrt[4]{{\alpha_3}{\alpha_5^{-1}}}, t = 0, we have the family of representatives \langle \nabla_3+\alpha\nabla_4+\nabla_5 \rangle;
{\rm{(c)}}\ if \alpha_1 = 0, \alpha_2\neq0, then by choosing x = {\alpha_2}{\alpha_5^{-1}}, t = 0, we have the family of representatives \langle \nabla_2+\alpha\nabla_3+\beta\nabla_4+\nabla_5 \rangle;
{\rm{(d)}}\ if \alpha_1\neq0, then by choosing x = \sqrt[4]{{\alpha_1}{\alpha_5^{-1}}}, t = 0, we have the family of representatives \langle \nabla_1+\alpha\nabla_2+\beta\nabla_3+\gamma\nabla_4+\nabla_5 \rangle.
3. \alpha_7 = 0, \alpha_6\neq0, then we have the following subcases:
{\rm{(a)}}\ if \alpha_2 = 0, \alpha_3 = 0, \alpha_4 = 0, then we have representatives \langle \nabla_6 \rangle and \langle \nabla_5+\nabla_6 \rangle depending on whether \alpha_5 = 0 or not;
{\rm{(b)}}\ if \alpha_2 = 0, \alpha_3 = 0, \alpha_4\neq0, then by choosing x = \sqrt[4]{{\alpha_4}{\alpha_6}^{-1}}, t = -\alpha_1 \sqrt[4]{\alpha_4 \alpha_6^{-5}} , we have the family of representatives \langle \nabla_4+\alpha\nabla_5+\nabla_6 \rangle;
{\rm{(c)}}\ if \alpha_2 = 0, \alpha_3\neq0, then by choosing x = \sqrt[5]{{\alpha_3}{\alpha_6^{-1}}}, t = - \alpha_1\sqrt[5]{\alpha_3 \alpha_6^{-6}}, we have the family of representatives \langle \nabla_3+\alpha\nabla_4+\beta\nabla_5+\nabla_6 \rangle;
{\rm{(d)}}\ if \alpha_2\neq0, then by choosing x = {{\alpha_2}{\alpha_6^{-1}}}, t = -{\alpha_1\sqrt{\alpha_2 \alpha_6^{-3}}}, we have the family of representatives \langle \nabla_2+\alpha\nabla_3+\beta\nabla_4+\gamma\nabla_5+\nabla_6 \rangle.
4. \alpha_7\neq0, then we have the following subcases:
{\rm{(a)}}\ \alpha_1\alpha_7-\alpha_2\alpha_6 = 0, \alpha_3 = 0, \alpha_4 = 0, \alpha_5 = 0, then we have representatives \langle \nabla_7 \rangle and \langle \nabla_6+\nabla_7 \rangle depending on whether \alpha_6 = 0 or not;
{\rm{(b)}}\ \alpha_1\alpha_7-\alpha_2\alpha_6 = 0, \alpha_3 = 0, \alpha_4 = 0, \alpha_5\neq0, then by choosing x = \sqrt[4]{{\alpha_5}{\alpha_7^{-1}}}, t = -{\alpha_2\sqrt[4]{\alpha_5 \alpha_7^{-5}}}, we have the family of representatives \langle \nabla_5+\alpha\nabla_6+\nabla_7 \rangle;
{\rm{(c)}}\ \alpha_1\alpha_7-\alpha_2\alpha_6 = 0, \alpha_3 = 0, \alpha_4\neq0, then by choosing x = \sqrt[7]{{\alpha_4\alpha_7^{-1}}}, t = -{\alpha_2\sqrt[7]{\alpha_4 \alpha_7^{-8}}}, we have the family of representatives \langle \nabla_4+\alpha\nabla_5+\beta\nabla_6+\nabla_7 \rangle;
{\rm{(d)}}\ \alpha_1\alpha_7-\alpha_2\alpha_6 = 0, \alpha_3\neq0, then by choosing x = \sqrt[8]{{\alpha_3}{\alpha_7^{-1}}}, t = -{\alpha_2\sqrt[8]{\alpha_3 \alpha_7^{-9}}}, we have the family of representatives \langle \nabla_3+\alpha\nabla_4+\beta\nabla_5+\gamma\nabla_6+\nabla_7 \rangle;
{\rm{(e)}}\ \alpha_1\alpha_7-\alpha_2\alpha_6\neq0, then by choosing
we have the family of representatives \langle \nabla_1+\alpha\nabla_3+\beta\nabla_4+\gamma\nabla_5+\mu\nabla_6+\nabla_7 \rangle.
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
4.4. 1 -dimensional central extensions of {\mathbf N}_{04}^{4} .
Here we will collect all information about {\mathbf N}_{04}^{4}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{7}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4}_{04}) . Since
we have
We are interested in (\alpha_2,\alpha_5,\alpha_6,\alpha_7)\neq(0,0,0,0) and consider following cases:
1.\ if \alpha_7 = \alpha_6 = \alpha_5 = 0, then \alpha_2\neq0, and we have the family of representatives
2.\ if \alpha_7 = 0, \alpha_6 = 0, \alpha_5\neq0, then we have the family of representatives
3.\ if \alpha_7 = 0, \alpha_6\neq0, then by choosing \phi = \phi_+, t = -{\alpha_1}{\alpha_6^{-1}}, we have the family of representatives
4.\ if \alpha_7\neq0, then by choosing \phi = \phi_+, t = -{\alpha_2}{\alpha_7^{-1}} we have the family of representatives
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
4.5. 1 -dimensional central extensions of {\mathbf N}_{05}^{4} .
Here we will collect all information about {\mathbf N}_{05}^{4}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{7}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4}_{05}) . Since
we have
We are interested in (\alpha_2,\alpha_4,\alpha_6,\alpha_7)\neq(0,0,0,0) and consider following cases:
1.\ \alpha_7 = \alpha_6 = \alpha_4 = 0, then \alpha_2\neq0 and we have the following subcases:
{\rm{(a)}}\ \alpha_3 = -2\alpha_2,
{\rm{(i)}}\ if \alpha_1 = 0, \alpha_5 = 0, then we have the representative \langle \nabla_2-2\nabla_3 \rangle;
{\rm{(ii)}}\ if \alpha_1 = 0, \alpha_5\neq0, then by choosing x = \sqrt{{\alpha_2}{\alpha_5^{-1}}}, z = 0, t = 0, we have the representative \langle \nabla_2-2\nabla_3+\nabla_5 \rangle;
{\rm{(iii)}}\ if \alpha_1\neq0, then by choosing x = \sqrt[3]{{\alpha_1}{\alpha_2^{-1}}}, z = 0, t = 0, we have the family of representatives \langle \nabla_1+\nabla_2-2\nabla_3+\alpha\nabla_5 \rangle.
(b) \alpha_3\neq -2\alpha_2,
{\rm{(i)}}\ if \alpha_5 = 0, then choosing x = 1, z = -\frac{\alpha_1}{\alpha_3+2\alpha_2}, we have the family of representatives \langle \nabla_2+\alpha\nabla_3 \rangle_{\alpha\neq-2}, which will be jointed with the case (1(a)i);
{\rm{(ii)}}\ if \alpha_5\neq0, then by choosing x = \sqrt{\frac{\alpha_2}{\alpha_5}}, z = -\frac{\alpha_1\sqrt{\alpha_2}}{(\alpha_3+2\alpha_2)\sqrt{\alpha_5}}, we have the family of representatives \langle\nabla_2+\alpha\nabla_3+\nabla_5 \rangle_{\alpha\neq-2}, which will be jointed with the case (1(a)ii).
2. \alpha_7 = \alpha_6 = 0, \alpha_4\neq0, then we have the following subcases:
{\rm{(a)}}\ if \alpha_2 = 0, \alpha_3 = 0, then we have representatives \langle \nabla_4 \rangle and \langle \nabla_4+\nabla_5 \rangle depending on whether \alpha_5 = 0 or not;
{\rm{(b)}}\ if \alpha_2 = 0, \alpha_3\neq0 , then by choosing x = {\alpha_3}{\alpha_4^{-1}}, z = 0, t = -{\alpha_1\alpha_3}{\alpha_4^{-2}}, we have the family of representatives \langle \nabla_3+ \nabla_4+\alpha\nabla_5 \rangle;
{\rm{(c)}}\ if \alpha_2\neq0 , then by choosing x = {\alpha_2}{\alpha_4^{-1}}, z = 0, t = -{\alpha_1\alpha_2}{\alpha_4^{-2}}, we have the family of representatives \langle \nabla_2+\alpha\nabla_3+ \nabla_4+\beta\nabla_5 \rangle.
3. \alpha_7 = 0, \alpha_6\neq0, then by choosing z = -{\alpha_2}x{\alpha_6^{-1}} , we have \alpha_2^* = 0. Thus, we can suppose that \alpha_2 = 0 and consider following subcases:
{\rm{(a)}}\ \alpha_4 = 0,
{\rm{(i)}}\ if \alpha_1 = 0, \alpha_3 = 0, then we have representatives \langle \nabla_6 \rangle and \langle \nabla_5+\nabla_6 \rangle depending on whether \alpha_5 = 0 or not;
{\rm{(ii)}}\ if \alpha_1 = 0, \alpha_3\neq0 then by choosing x = \sqrt[3]{{\alpha_3}{\alpha_6^{-1}}}, z = 0, t = 0, we have the family of representatives \langle \nabla_3+\alpha\nabla_5+\nabla_6 \rangle;
{\rm{(iii)}}\ if \alpha_1\neq0, then by choosing x = \sqrt[6]{{\alpha_1}{\alpha_6^{-1}}}, z = 0, t = 0, we have the family of representatives \langle \nabla_1+\alpha\nabla_3+\beta\nabla_5+\nabla_6 \rangle.
(b) \alpha_4\neq0 , then by choosing x = \sqrt{{\alpha_4}{\alpha_6^{-1}}}, t = -{\alpha_1\sqrt{\alpha_4 \alpha_6^{-3}}}, we have the family of representatives \langle \alpha\nabla_3+\nabla_4+\beta\nabla_5+\nabla_6 \rangle.
4. \alpha_7\neq0,
{\rm{(a)}}\ if \alpha_1\alpha_7-\alpha_2\alpha_4 = 0, \alpha_3 = 0, \alpha_6 = 0, then then by choosing z = -\frac{\alpha_4}{2\alpha_7}x, t = \frac{\alpha_4\alpha_6-2\alpha_2\alpha_7}{2\alpha^2_7}x, we have representatives \langle \nabla_7 \rangle and \langle \nabla_5+\nabla_7 \rangle depending on whether \alpha_5 = 0 or not;
{\rm{(b)}}\ if \alpha_1\alpha_7-\alpha_2\alpha_4 = 0, \alpha_3\alpha_7-\alpha_4\alpha_6 = 0, \alpha_6\neq0, then by choosing
we have the family of representatives \langle \alpha\nabla_5+\nabla_6+\nabla_7 \rangle;
{\rm{(c)}}\ if 2\alpha_1\alpha_7^2-\alpha_3\alpha_4\alpha_7+\alpha_4^2\alpha_6-2\alpha_2\alpha_4\alpha_7 = 0, \alpha_3\alpha_7-\alpha_4\alpha_6\neq0, then by choosing
we have the family of representatives \langle \nabla_3+\alpha\nabla_5+\beta\nabla_6+\nabla_7 \rangle;
{\rm{(d)}}\ if 2\alpha_1\alpha_7^2-\alpha_3\alpha_4\alpha_7+\alpha_4^2\alpha_6-2\alpha_2\alpha_4\alpha_7\neq0, then by choosing
we have the family of representatives \langle \nabla_1+\alpha\nabla_3+\beta\nabla_5+\gamma\nabla_6+\nabla_7 \rangle.
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
4.6. 1 -dimensional central extensions of {\mathbf N}_{06}^{4} .
Here we will collect all information about {\mathbf N}_{06}^{4}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{7}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4}_{06}). Since
we have
Since (\alpha_2,\alpha_4,\alpha_6,\alpha_7)\neq(0,0,0,0) and for \phi = \phi_+, we have the following cases:
1.\ if \alpha_7 = \alpha_6 = \alpha_4 = 0, then \alpha_2\neq0, and we have the following subcase:
{\rm{(a)}}\ if \alpha_5 = \alpha_3+2\alpha_2, then we have the family of representatives
{\rm{(b)}}\ if \alpha_5\neq\alpha_3+2\alpha_2, then by choosing z = 0, t = \frac{\alpha_1}{\alpha_3+2\alpha_2-\alpha_5} , we have the family of representative \langle \nabla_2+\alpha\nabla_3+\beta\nabla_5 \rangle_{\beta\neq\alpha+2};
2. if \alpha_7 = 0, \alpha_6 = 0, \alpha_4\neq0, then by choosing z = 0, t = \frac{\alpha_1}{\alpha_4}, we have the family of representatives \langle \alpha\nabla_2+\beta\nabla_3+\nabla_4+\gamma\nabla_5 \rangle;
3. if \alpha_7 = 0, \alpha_6\neq0, then we have the following subcases:
{\rm{(a)}}\ if \alpha_6 = \alpha_4, then by choosing z = -{\alpha_2}{\alpha_6^{-1}}, t = 0, we have the family of representatives \langle \alpha\nabla_1+\beta\nabla_3+\nabla_4+\gamma\nabla_5+\nabla_6 \rangle;
{\rm{(b)}}\ if \alpha_6\neq\alpha_4, then by choosing z = -\frac{\alpha_2}{\alpha_6}, t = \frac{\alpha_1\alpha_6-\alpha_2\alpha_5+\alpha_2\alpha_3}{\alpha_6(\alpha_4-\alpha_6)}, we have the family of representatives \langle \alpha\nabla_3+\beta\nabla_4+\gamma\nabla_5+\nabla_6 \rangle_{\beta\neq1};
4. if \alpha_7\neq0, then by choosing z = -\frac{\alpha_4}{2\alpha_7}, t = \frac{\alpha_4\alpha_6-2\alpha_2\alpha_7}{2\alpha^2_7}, we have the family of representatives
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
4.7. 1 -dimensional central extensions of {\mathbf N}_{07}^{4} .
Here we will collect all information about {\mathbf N}_{07}^{4}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{7}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4}_{07}) . Since
we have
We are interested in (\alpha_3,\alpha_4,\alpha_6,\alpha_7)\neq(0,0,0,0) and consider following cases:
1.\ \alpha_7 = \alpha_6 = \alpha_4 = 0, then \alpha_3\neq0, and we have the following subcases:
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_2 = 0, then we have representatives \langle \nabla_3 \rangle and \langle \nabla_3+\nabla_5 \rangle depending on whether \alpha_5 = 0 or not;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_2\neq0, then by choosing x = \sqrt{{\alpha_2}{\alpha_3^{-1}}}, t = 0, we have the family of representatives \langle \nabla_2+\nabla_3+\alpha\nabla_5 \rangle;
{\rm{(c)}}\ if \alpha_1\neq0, then by choosing x = \sqrt[4]{{\alpha_1}{\alpha_3^{-1}}}, t = 0, we have the family of representatives \langle \nabla_1+\alpha\nabla_2+\nabla_3+\beta\nabla_5 \rangle.
2. \alpha_7 = 0, \alpha_6 = 0, \alpha_4\neq0, then we have the following subcases:
{\rm{(a)}}\ if \alpha_2 = 0, \alpha_3 = 0 , then we have the familty of representative \langle \nabla_4+\alpha\nabla_5 \rangle;
{\rm{(b)}}\ if \alpha_2 = 0, \alpha_3\neq0 , then by choosing x = {\alpha_3}{\alpha_4^{-1}}, t = -{\alpha_1\alpha_3}{\alpha_4^{-2}}, we have the family of representatives \langle \nabla_3+ \nabla_4+\alpha\nabla_5 \rangle;
{\rm{(c)}}\ if \alpha_2\neq0, then by choosing x = \sqrt[3]{{\alpha_2}{\alpha_4^{-1}}}, t = -{\alpha_1\sqrt[3]{\alpha_2 \alpha_4^{-4}}}, we have the family of representatives \langle \nabla_2+\alpha\nabla_3+ \nabla_4+\beta\nabla_5 \rangle.
3. \alpha_7 = 0, \alpha_6\neq0, then we have the following subcases:
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_3 = 0, \alpha_4 = 0, then we have representatives \langle \nabla_6 \rangle and \langle \nabla_5+\nabla_6 \rangle depending on whether \alpha_5 = 0 or not;
{\rm{(b)}}\ if \alpha_1\alpha_6 = \alpha_2\alpha_4, \alpha_3 = 0, \alpha_4\neq0, then choosing x = \sqrt{{\alpha_4}{\alpha_6^{-1}}}, t = -{\alpha_2\sqrt{\alpha_4} \alpha_6^{-3}}, we have the family of representatives \langle \nabla_4+\alpha\nabla_5+\nabla_6 \rangle;
{\rm{(c)}}\ if \alpha_1\alpha_6 = \alpha_2\alpha_4, \alpha_3\neq0, then by choosing x = \sqrt[3]{\frac{\alpha_3}{\alpha_6}}, t = -\frac{\alpha_2\sqrt[3]{\alpha_3}}{\alpha_6\sqrt[3]{\alpha_6}}, we have the family of representatives \langle \nabla_3+\alpha\nabla_4+\beta\nabla_5+\nabla_6 \rangle;
{\rm{(d)}}\ if \alpha_1\alpha_6\neq\alpha_2\alpha_4 , then choosing x = \sqrt[7]{\frac{\alpha_1\alpha_6-\alpha_2\alpha_4}{\alpha^2_6}}, t = -\frac{\alpha_2\sqrt[7]{\alpha_1\alpha_6-\alpha_2\alpha_4}}{\alpha_6\sqrt[7]{\alpha^2_6}}, we have the family of representatives \langle \nabla_1+\alpha\nabla_3+\beta\nabla_4+\gamma\nabla_5+\nabla_6 \rangle.
4. \alpha_7\neq0, then we have the following subcases:
{\rm{(a)}}\ \alpha_1 = 0, \alpha_2\alpha_7 = \alpha_3\alpha_6, \alpha_4 = 0, \alpha_5 = 0, then we have representatives \langle \nabla_7 \rangle and \langle \nabla_6+\nabla_7 \rangle depending on whether \alpha_6 = 0 or not;
{\rm{(b)}}\ \alpha_1 = 0, \alpha_2\alpha_7 = \alpha_3\alpha_6, \alpha_4 = 0, \alpha_5\neq0, then by choosing x = \sqrt[4]{{\alpha_5}{\alpha_7^{-1}}}, t = -{\alpha_3\sqrt[4]{\alpha_5 \alpha_7^{-5}}}, we have family of representatives \langle \nabla_5+\alpha\nabla_6+\nabla_7 \rangle;
{\rm{(c)}}\ \alpha_1\alpha_7 = \alpha_3\alpha_4, \alpha_2\alpha_7 = \alpha_3\alpha_6, \alpha_4\neq0, then by choosing x = \sqrt[4]{{\alpha_4}{\alpha_7^{-1}}}, t = -{\alpha_3\sqrt[4]{\alpha_4 \alpha_7^{-5}}}, we have family of representatives \langle \nabla_4+\alpha\nabla_5+\beta\nabla_6+\nabla_7 \rangle;
{\rm{(d)}}\ \alpha_1\alpha_7 = \alpha_3\alpha_4, \alpha_2\alpha_7\neq\alpha_3\alpha_6, then by choosing x = \sqrt[7]{(\alpha_2\alpha_7-\alpha_3\alpha_6)\alpha^{-2}_7}, t = -{\alpha_3\sqrt[7]{(\alpha_2\alpha_7-\alpha_3\alpha_6)\alpha_7^{-9}}}, we have the family of representatives
{\rm{(e)}}\ \alpha_1\alpha_7\neq \alpha_3\alpha_4, then by choosing
we have family of representatives \langle \nabla_1+\alpha\nabla_2+\beta\nabla_4+\gamma\nabla_5+\mu\nabla_6+\nabla_7 \rangle.
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
4.8. 1 -dimensional central extensions of {\mathbf N}_{08}^{4} .
Here we will collect all information about {\mathbf N}_{08}^{4}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{7}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4}_{08}) . Since
we have
Since (\alpha_2,\alpha_4,\alpha_6,\alpha_7)\neq(0,0,0,0), we have the following cases:
1.\ if \alpha_7 = \alpha_6 = \alpha_4 = 0, then \alpha_2\neq0, and we have the family of representatives
2.\ if \alpha_7 = 0, \alpha_6 = 0, \alpha_4\neq0, then by choosing t = -{\alpha_1}{\alpha_4^{-1}}, we have the family of representatives
3.\ if \alpha_7 = 0, \alpha_6\neq0, then by choosing t = {\alpha_3}{\alpha_6^{-1}}, we have the family of representatives
4.\ if \alpha_7\neq0, then by choosing t = -{\alpha_2}{\alpha_7^{-1}}, we have the family of representatives
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
4.9. 1 -dimensional central extensions of {\mathbf N}_{09}^{4} .
Here we will collect all information about {\mathbf N}_{09}^{4}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{7}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4}_{09}) . Since
we have
Since (\alpha_3,\alpha_5,\alpha_6,\alpha_7)\neq(0,0,0,0), we have the following cases:
1.\ \alpha_7 = \alpha_6 = \alpha_5 = 0, then \alpha_3\neq0, and we have the following subcases:
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_2 = 0, then we have representatives \langle \nabla_3 \rangle and \langle \nabla_3+\nabla_4 \rangle depending on whether \alpha_4 = 0 or not;
{\rm{(b)}}\ if \alpha_1 = 0, \alpha_2\neq0, then by choosing x = \sqrt[4]{{\alpha_2}{\alpha_3^{-1}}}, t = 0, we have the family of representatives \langle \nabla_2+\nabla_3+\alpha\nabla_4 \rangle;
{\rm{(c)}}\ if \alpha_1\neq0, then by choosing x = \sqrt[6]{{\alpha_1}{\alpha_3^{-1}}}, t = 0, we have the family of representatives \langle \nabla_1+\alpha\nabla_2+\nabla_3+\beta\nabla_4 \rangle.
2. \alpha_7 = 0, \alpha_6 = 0, \alpha_5\neq0, then we have the following cases:
{\rm{(a)}}\ if \alpha_2 = 0, \alpha_3 = 0, then we have representatives \langle \nabla_5 \rangle and \langle \nabla_4+\nabla_5 \rangle depending on whether \alpha_4 = 0 or not;
{\rm{(b)}}\ if \alpha_2 = 0, \alpha_3\neq0, then choosing x = {\alpha_3}{\alpha_5^{-1}}, t = -{\alpha_1\alpha_3}{\alpha_5^{-2}}, we have the family of representatives \langle \nabla_3+ \alpha\nabla_4+\nabla_5 \rangle;
{\rm{(c)}}\ if \alpha_2\neq0, then by choosing x = \sqrt[5]{{\alpha_2}{\alpha_5^{-1}}}, t = -{\alpha_1\sqrt[5]{\alpha_2 \alpha_5^{-6} }}, we have the family of representatives \langle \nabla_2+\alpha\nabla_3+ \beta\nabla_4+\nabla_5 \rangle.
3. \alpha_7 = 0, \alpha_6\neq0, then we have the following cases:
{\rm{(a)}}\ if \alpha_1\alpha_6 = \alpha_2\alpha_5, \alpha_3 = 0, \alpha_4 = 0, then we have representatives \langle \nabla_6 \rangle and \langle \nabla_5+\nabla_6 \rangle depending on whether \alpha_5 = 0 or not;
{\rm{(b)}}\ if \alpha_1\alpha_6 = \alpha_2\alpha_5, \alpha_3 = 0, \alpha_4\neq0, then by choosing x = \sqrt[6]{{\alpha_4}{\alpha_6^{-1}}}, t = -{\alpha_2\sqrt[6]{\alpha_4 \alpha_6^{-7} }}, we have the family of representatives \langle \nabla_4+\alpha\nabla_5+\nabla_6 \rangle;
{\rm{(c)}}\ if \alpha_1\alpha_6 = \alpha_2\alpha_5, \alpha_3\neq0, then by choosing x = \sqrt[3]{{\alpha_3}{\alpha_6^{-1}}}, t = -{\alpha_2\sqrt[3]{\alpha_3 \alpha_6^{-4}}}, we have the family of representatives \langle \nabla_3+\alpha\nabla_4+\beta\nabla_5+\nabla_6 \rangle;
{\rm{(d)}}\ if \alpha_1\alpha_6\neq \alpha_2\alpha_5 , then by choosing
we have the family of representatives
4. \alpha_7\neq0, then we have the following cases:
{\rm{(a)}}\ if \alpha_1 = 0, \alpha_2\alpha_7 = \alpha_3\alpha_6, \alpha_4 = 0, \alpha_5 = 0, then choosing x = 1, t = -\frac{\alpha_3}{\alpha_7}, we have representatives \langle \nabla_7 \rangle and \langle \nabla_6+\nabla_7 \rangle depending on whether \alpha_6 = 0 or not;
{\rm{(b)}}\ if \alpha_1\alpha_7 = \alpha_3\alpha_5, \alpha_2\alpha_7 = \alpha_3\alpha_6, \alpha_4 = 0, \alpha_5\neq0, then by choosing x = \sqrt[6]{{\alpha_5}{\alpha_7^{-1}}}, t = -{\alpha_3\sqrt[6]{\alpha_5 \alpha_7^{-7} }}, we have the family of representatives \langle \nabla_5+\alpha\nabla_6+\nabla_7 \rangle;
{\rm{(c)}}\ if \alpha_1\alpha_7 = \alpha_3\alpha_5, \alpha_2\alpha_7 = \alpha_3\alpha_6, \alpha_4\neq0, then by choosing
we have the family of representatives \langle \nabla_4+\alpha\nabla_5+\beta\nabla_6+\nabla_7 \rangle;
{\rm{(d)}}\ \alpha_1\alpha_7 = \alpha_3\alpha_5, \alpha_2\alpha_7\neq\alpha_3\alpha_6, then by choosing
we have the family of representatives
{\rm{(e)}}\ \alpha_1\alpha_7\neq\alpha_3\alpha_5, then by choosing
we have the family of representatives
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
4.10. 1 -dimensional central extensions of {\mathbf N}_{10}^{4} .
Here we will collect all information about {\mathbf N}_{10}^{4}:
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{7}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4}_{10}) . Since
we have
Since (\alpha_2,\alpha_4,\alpha_6,\alpha_7)\neq(0,0,0,0), we have the following cases:
1.\ if \alpha_7 = 0, \alpha_6 = 0, \alpha_4 = 0, then \alpha_2\neq0, and we have the family of representatives
2.\ if \alpha_7 = 0, \alpha_6 = 0, \alpha_4\neq0, then we have the family of representatives
3.\ if \alpha_7 = 0, \alpha_6\neq0, then we have the family of representatives
4.\ if \alpha_7\neq0, then we have the family of representatives
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
4.11. 1 -dimensional central extensions of {\mathbf N}_{11}^{4}(\lambda) .
Here we will collect all information about {\mathbf N}_{11}^{4}(\lambda):
Let us use the following notations:
Take \theta = \sum\limits_{i = 1}^{7}\alpha_i\nabla_i\in\mathrm{H}^2_{\mathfrak{C}}(\mathbf{N}^{4}_{11}(\lambda)) . Since
we have
Since (\alpha_3,\alpha_5,\alpha_6,\alpha_7)\neq(0,0,0,0), we have the following cases:
1.\ if \alpha_7 = 0, \alpha_6 = 0, \alpha_5 = 0 , then \alpha_3\neq0 and we have the family of representatives
2.\ if \alpha_7 = 0, \alpha_6 = 0, \alpha_5\neq0 then by choosing t = -{\alpha_1}{\alpha_5^{-1}}, we have the family of representatives
3.\ if \alpha_7 = 0, \alpha_6\neq0 then by choosing t = -{\alpha_2}{\alpha_6^{-1}}, we have the family of representatives
4.\ if \alpha_7\neq0 then by choosing t = -{\alpha_3}{\alpha_7^{-1}}, we have the family of representatives
Summarizing, we have the following distinct orbits:
which gives the following new algebras:
Remark 2. Note that the algebras \mathbf{N}^{4}_{11}(\lambda) and \mathbf{N}^{4}_{11}(-\lambda) are isomorphic. Hence, there are some additional isomorphism relations for algebras from the present subsection \mathbf{N}^{\lambda, \Xi} \cong \mathbf{N}^{-\lambda, \Xi} .
5.
Classification theorem.
Theorem 5.1. Let \mathbf N be a complex 5 -dimensional nilpotent commutative algebra. Then we have one of the following situations.
{\mathit{1.}} \ If \mathbf N is associative, then \mathbf N is isomorphic to one algebra listed in [15].
{\mathit{2.}} \ If \mathbf N is a non-associative Jordan algebra, then \mathbf N is isomorphic to one algebra listed in [9].
{\mathit{3.}} \ If \mathbf N is a non-Jordan \mathfrak{CD} -algebra, then \mathbf N is isomorphic to one algebra listed in [11].
{\mathit{4.}}\ If \mathbf N is a non- \mathfrak{CD} -algebra, then \mathbf N is isomorphic to one algebra listed in the following list.









 DownLoad:
DownLoad: