1. The Role of Short-term Consolidation in Memory Persistence
Much research has been devoted to understanding how information enters short-term memory. There is considerable agreement that perceptual and long-term representations are automatically activated when information is perceived [1,2,3,4,5], resulting in a transient sensory memory for the stimulus. These sensory memory representations are lost in at most a matter of seconds, with the time of persistence being dependent on the presentation modality [6,7,8]. Some encoding time is needed in order to transition the sensory memory information into a short-term memory trace [9,10,11,12,13]. If short-term encoding is not completed before loss of the sensory memory trace, the memory will not be maintained for further processing. After encoding is complete, it is generally assumed that automatic processing in the immediate second or two following item presentation cannot continue to improve the representation within short-term memory. Intentional strategic processing using long-term memory, such as elaborative encoding, may still improve memory performance [14,15,16], but this process takes more than a second or two to occur and is generally a function of improved long-term connections.
The following pages challenge the assumption that encoding is the only short-term memory formation process occurring following item presentation. Emerging evidence strongly supports a critical period immediately following item presentation. During this period, short-term memory performance can be improved by directing attention toward the internal representation of the just-presented item, even after encoding processes are no longer possible [17,18]. This process has been called short-term consolidation [18,19], and results in better memory performance by attenuating short-term forgetting [18]. The mechanism through which short-term consolidation functions is unknown at present, but some informed guesswork leads to several potential cognitive and neurobiological processes. The relevant sections below describe the evidence for short-term consolidation as a separate process from encoding, what is known about the consolidation process itself, and the potential cognitive and neurobiological processes through which consolidation may function.
Throughout this work I primarily discuss short-term consolidation in the context of memory models including a short-term memory state. This often implies a division between a long-term memory and a short-term memory state. Although I subscribe to this distinction, it is not an argument that this work is intended to address. By talking about short-term memory I simply mean memory used for immediate processing with specific empirical qualities over the short term. Models of memory with only a long-term memory state could be made to conform to the empirical findings and theoretical processes related to short-term consolidation. The findings and processes described in the following pages are equally applicable to single-process models of memory and should not be read as only addressing memory models emphasizing a distinction between short-term and long-term memory.
2. Encoding, Consolidation, and Immediate Memory States
Whether one conceptualizes short-term memory as a memory system distinct from long-term memory [1,20,21,22,23] or as the most accessible portion of long-term memory [24,25,26] stimuli must enter the memory system. Encoding and consolidation processes are the manner in which this occurs. At this point, it is important to provide further clarity on the differences between sensory and short-term memory, and between the short-term encoding and short-term consolidation processes.
Sensory memory is the brief storage of sensory information brought in by the senses [6,27]. Sensory memory is often referred to as iconic memory when it is in the visual modality and echoic memory within the auditory modality. The information held within sensory memory is transient and is constantly updated based upon incoming information from the senses. Sensory memory cannot be actively maintained or cognitively manipulated. As such, it is extremely time-limited and vulnerable to disruption from newly perceived sensory stimuli. On the other hand, short-term memory is a state of memory that is created through the encoding process described below [11,12]. Short-term memory is composed of activated sensory and long-term features that can be actively maintained and manipulated. Although activation of a short-term memory trace can still be lost, it is more robust against forgetting processes than sensory memory due to the function of short-term memory maintenance [1,28] and the availability of other cognitive processes such as short-term consolidation [18]. These cognitive operations are not available for use with a sensory memory trace.
In order for encoding to occur, a sensory memory trace is needed. When sensory stimuli are first perceived a sensory memory trace is created [6,7,8]. This trace is only active for a brief period of time and is extremely vulnerable to disruption from interfering stimuli. If no further processing occurs during this time, no short-term or long-term memory trace will be created and no memory will exist. A similar process can be conceptualized for internally generated stimuli, mimicking what happens with physical perception [29]. Stored concepts in long-term memory can be retrieved and brought to mind, creating an internally generated stimulus similar to a sensory memory trace. If attention dwells on this internally generated stimulus long enough it can be encoded into short-term memory. If attention is quickly shifted away from the internally generated stimulus before encoding is complete, then no short-term memory will be formed and the stimulus will fade.
Directing attention toward a sensory memory trace begins the process of encoding the trace into short-term memory. Encoding occurs so long as attention is directed toward the trace or the physical stimulus in the environment and proceeds until completed or until attention is shifted away from the stimulus [13,30,31]. Encoding refers to a process where attention is directed toward a sensory memory trace to establish a stable stimulus identity and feature values which can then be stored and manipulated by short-term/working memory mechanisms. Encoding of a particular item ends once a representation has been successfully established within short-term memory. Encoding fails if a representation has not been established by the time the sensory memory trace has been abolished whether through decay [1,6] or perceptual interference such as that produced by post-perceptual masking [7,10,11].
Within different theoretical approaches to short-term memory the encoding process can be thought of in slightly different terms. The cognitive processes thought to underlie encoding can be seen as equivalent to Massaro’s [9,32] perceptual processing to extract short-term memory units. In this theoretical approach the extraction of units from sensory information occurs if a meaningful pattern can be identified, at which point the unit enters short-term memory for later use in conscious processing. In the multicomponent models of Baddeley [20,33] and Logie [34], encoding can be thought of as the entry of items into modality specific short-term memory buffers. In the embedded process model of Cowan [1,28] or within a single-state view such as that of Farrell [25] encoding is equivalent to the use of sensory memory to activate stimulus object traces within long-term memory, as well as the individual features of which the stimulus is composed.
In contrast to encoding, consolidation of a memory trace does not involve the creation of the short-term trace itself. Consolidation is a process that is carried out after a trace is encoded and exists in a short-term memory state [18,19]. Through consolidation the trace becomes more resistant to forgetting processes which will occur later during memory retention [18]. The consolidation process itself is brief, finishing within a second or two after item presentation, and results in increased resistance to forgetting over the life of the short-term memory trace. Consolidation requires attention to be directed toward the short-term memory trace and as such can occur even after the physical stimulus and related sensory memory traces no longer exist. As sensory memory traces are not necessary for consolidation to occur, the process may continue even after post-perceptual masking or the presentation of otherwise interfering stimuli, so long as attention can dwell on the internal short-term memory trace.
The lack of research on the nature of consolidation makes it difficult to confidently identify a single cognitive process that is accountable for the consolidation effect in short-term memory. Despite this, some existing models do have concepts that would naturally fit what we know about short-term consolidation at this point. For example, in the information processing framework of Massaro [32], consolidation could represent the secondary recognition processes in which perceptually meaningful memory units are transformed into generalized abstract memories by identifying meaningful relationships beyond the perceptual stage. In the Multi-Component Framework of Baddeley [20,33] or Logie [34], consolidation could represent the initial processing needed to register items already within the phonological store or visual-spatial sketchpad as items to be maintained by the phonological loop or inner scribe. The Embedded-Process Model of Cowan [1,28] could incorporate consolidation as the entry of activated memory traces into the focus attention and the enhancement of these memory traces that accompanies residence within the focus of attention. Single-process views of memory [25,26] could potentially include short-term consolidation as the leading edge of long-term consolidation. This last approach is discussed in greater detail in the relevant section below.
In the literature on short-term memory, the terms encoding and consolidation are often used interchangeably, leading to much confusion when trying to differentiate the two processes. Studies by some authors use the terms encoding and consolidation as I do [17,18,19], others refer to the period during which the short-term memory is being created as the consolidation period [11,12,13]. Many others conflate encoding and consolidation, using the terms as synonyms. While terminology is in some sense arbitrary, formalized meaning across studies is needed and I argue for the following usage of terminology.
Here, short-term encoding refers to a process through which the short-term memory state is created. During the encoding process attention is directed toward sensory memory traces. Successfully encoded short-term memories can be stored and manipulated by short-term/working memory mechanisms. On the other hand, short-term consolidation refers to a process which improves the durability of an established short-term memory against forgetting processes that occur later. During the consolidation process attention is directed toward short-term memory traces. Short-term consolidation improves the durability and persistence of the trace in the face of forgetting processes that occur at a later point in time.
3. Masking to Differentiate Consolidation from Encoding
Differentiating the effects of encoding and consolidation in short-term memory can be difficult. Both processes occur immediately following item presentation and proceed until completion. Both processes are complete in, at most, a total of only a second or two. Both processes lead to better memory performance when longer periods of time are allowed for their completion. This similarity presents a strong challenge to orthogonal manipulation of encoding and consolidation in an experimental context. In most circumstances attempts to lengthen or constrain the encoding period necessarily manipulate the time available for consolidation. Similarly, increasing the time available for consolidation necessarily increases the time available to encode stimuli in most circumstances.
One method to experimentally deconfound encoding and consolidation is to present a post-perceptual pattern mask after the presentation of each memory item (see Figure 1). Encoding of memory items begins at the time the memory item is presented and may continue until the stimulus is successfully encoded into short-term memory, until the sensory memory decays, or until onset of the post-perceptual pattern mask. Post-perceptual pattern masks prevent further encoding by overwriting the sensory afterimage and sensory memory traces that would otherwise allow continued encoding of the stimulus even after the item is removed from the environment [11,35,36], so long as the sensory memory trace is still present within the brain. When a longer period of time is available for encoding there will be a higher probability of successful encoding. In contrast, short-term consolidation does not require a sensory image to proceed, allowing this process to continue even after post-perceptual masking. This means that the period immediately following the pattern mask can be used for consolidation, but not encoding of the stimulus, so long as attention is allowed to dwell on the representation in short-term memory.
Onset of a post-perceptual mask ends encoding processes, but this should not be taken to mean that encoding always continues until the masking stimulus is presented. Encoding is a fast process and can sometimes be completed before a masking stimulus is presented given that enough stimulus presentation and encoding time is provided. For example, if two colored squares are presented on screen for a total of 4000 ms, encoding is likely to be complete before the presentation ends and any masking stimulus is presented. In this case consolidation could occur before the masking stimulus is presented. While masking ends encoding that is not already completed, it is not necessary to start the consolidation process. Consolidation can occur once a short-term memory representation is created; another way of saying at any point after encoding is complete. Thus, any time before a mask could be used for only encoding or for encoding and consolidation, but time after a mask can only be used for the consolidation process.
Stimulus masking procedures allow for the clear differentiation of consolidation from encoding processes in short-term memory. Unfortunately they are rarely used. In most experiments attempting to investigate short-term consolidation of visual stimuli it is assumed that the sensory memory image decays within about 250 ms of the stimulus being removed from the screen. This means that encoding cannot proceed beyond this time period. The logic that follows is that any manipulation of available free time that occurs after 250 ms from memory item offset is a manipulation of the consolidation period. This would be a sound argument if not for findings that post-perceptual masking effects occur after longer delays than the standard 250 msec. Sligte, Scholte, and Lamme [37] show large masking effects 750 msecs after memory array offset while Pinto, Sligte, Shapiro, & Lamme [38] find large masking effects 1300 msec after memory array offset, implying continued encoding throughout this period. Cowan [28] discusses a number of earlier studies with bearing on sensory memory persistence and argues that some visual sensory memory might persist for as long as 15 seconds.
If the arguments by Cowan [28] or the findings by Sligte et al. [37] and Pinto et al. [38] are broadly applicable then studies of short-term consolidation not utilizing a pattern-masking procedure are confounding encoding and consolidation effects. The lack of pattern masking is likely to be a strong driver of the confusion between consolidation and encoding effects in the short-term memory literature. It also means that many potentially exciting findings on the nature of short-term consolidation effects may in fact reflect encoding processes. In future work, I strongly advocate for the inclusion of pattern masks in all procedures investigating short-term consolidation.
Not only does the exclusion of pattern masks from common memory procedures mean that we often cannot attribute an effect to either encoding or consolidation processes, it also means that sensory memory for stimuli may linger into the retention interval or memory test periods [36]. Studies manipulating other retention and testing phenomenon such as interference effects, timing effects, or measures of memory precision could in fact be measuring changes in sensory memory. In any study where the existence of sensory memory could potentially be a confound pattern masks should be used. This applies not only to change detection tasks, but also to simple span, complex span, and memory production tasks. Without masking, it is often difficult or impossible to tell whether changes in sensory memory or the existence of sensory memory play a role in short-term memory findings.
4. Consolidation: Preventing Forgetting with Attention
Perhaps the first work to clearly demonstrate short-term consolidation as a process independent of encoding was by Jolicoeur & Dell’Acqua [19]. In this study one or three memory items, either letters or symbols, were presented and masked on each trial. Following item masking, there was a variable period of free time allowed for memory consolidation, after which a speeded two-choice task was required. The two-choice task was to identify whether a presented tone was high or low in pitch, with participants instructed to respond immediately upon hearing the tone. Participants then recalled the memory items. When more time was allowed for memory consolidation between offset of the masking stimulus and onset of the tone presentation, response times to the tone task were faster. This indicates that attention was more readily available for use in the secondary task when more time for consolidation was given. Mask presentation had ended encoding, yet attention was still occupied in consolidating the memory trace, or so Jolicoeur and Dell’Acqua argue.
Although Jolicoeur & Dell’Acqua [19] found delays in secondary task execution with shorter memory consolidation times, they did not find increased memory performance with longer consolidation times as would be expected for a process working to improve the durability of memory traces. This hole in the logical argument in favor of the short-term consolidation process was remedied by both Nieuwenstein & Wyble [17], who demonstrate that longer consolidation time before a secondary two-choice reaction time task improves performance on a memory task, and by Ricker & Cowan [18], who demonstrate that longer consolidation time between presentations of each memory item improves memory performance. Perhaps these studies succeeded in finding direct effects of short-term consolidation on memory where Jolicoeur & Dell’Acqua did not because they used a higher memory load and involved a more demanding use of attention to disrupt consolidation. Together these studies indicate that attention is needed for short-term memory consolidation. More specifically, they demonstrate that other tasks requiring attention are delayed when consolidation is ongoing [19] and that increased consolidation time leads to better memory performance up until the point that attention is removed from the memory trace [17,18].
Nieuwenstein & Wyble [17] demonstrated short-term consolidation effects on memory performance in all five of their experiments using a similar task to Jolicoeur & Dell’Acqua [19] but with some important differences. English-speaking participants had to remember two English letters, four English letters, or one complex Kanji character. This was a higher memory load than the one or three English Letters and one or three simple symbols used by Jolicoeur & Dell’Acqua. The secondary task of Nieuwenstein & Wyble was also more demanding, involving a two-choice number or color discrimination rather than the pitch identification of Jolicoeur & Dell’Acqua. The time between mask offset and secondary task onset was varied to manipulate the total time available for consolidation before attention was captured by the secondary task. In all experiments longer consolidation times lead to better performance on the memory task.
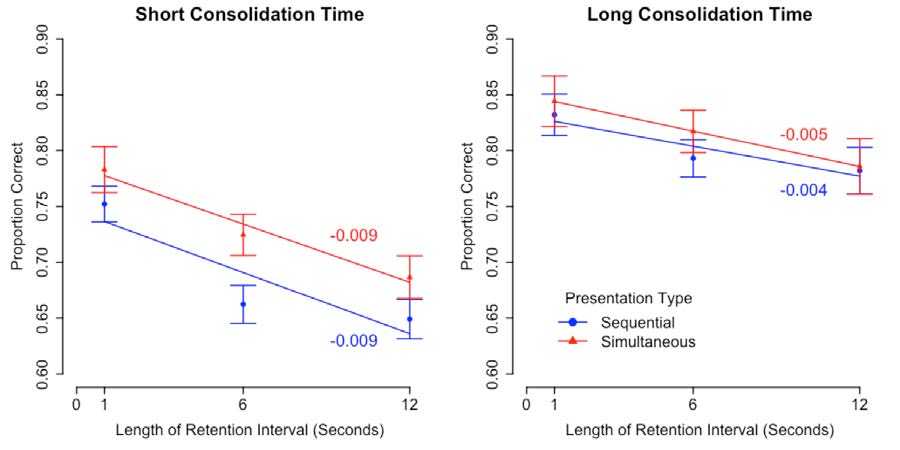
The investigation by Ricker & Cowan [18] is particularly helpful in uncovering how short-term consolidation improves memory performance. This study demonstrates that consolidation improves memory performance by slowing the rate of trace decay (i.e. time-based forgetting). Ricker and Cowan presented unfamiliar visual characters that are difficult to name, masked them to remove sensory memory, and then required participants to retain the memory traces over a variable retention interval of one to twelve seconds. Retention interval duration was measured as the amount of time from offset of the final masking stimulus to onset of the memory test. Performance declined with an increasing retention interval, in line with the large amount of recent evidence in favor of trace decay as a mechanism of short-term forgetting [39,40,41,42,43,44,45,46,47]. In the critical manipulation of consolidation timea variable amount of free time was given between item presentations. This free time occurred after masking of each memory item, but before the presentation of the next item, to allow for more memory consolidation. In this way consolidation time and retention interval duration were manipulated orthogonally. Consolidation time referred to the total free time after each item presentation while retention interval duration referred to the total amount of time between the memory-set presentation sequence and memory test. If consolidation did not reduce the rate of trace decay, then one would expect poorer performance with longer pauses between items. This would be expected due to the increased time for decay-based forgetting with longer pauses. Instead, longer consolidation times resulted in better performance. In particular the rate of loss across the retention interval was smaller when more consolidation time was allowed (see Figure 2). In other words, trace decay was slower when more consolidation was possible, indicating that consolidation improves short-term memory performance by slowing later forgetting.
While Ricker & Cowan [18] found that the short-term consolidation process decreased decay-based forgetting specifically, it may be that consolidation actually reduces future forgetting more generally. Researchers investigating short-term/working memory have found evidence for forgetting based upon interference from other attended stimuli [48,49] or based upon the cognitive load of a secondary task [21,50,51]. In particular, forgetting due to cognitive load, defined as the proportion of maintenance time during which attention is occupied by a non-maintenance task, is likely to be decreased by consolidation of a memory item. This is because trace decay in combination with attention-based maintenance is thought to drive cognitive load effects [21,42]. However, some claim this effect to be independent of decay [52,53]. Future work will be needed to establish whether the effect of short-term consolidation is only to decrease trace decay across a retention interval irrespective of other task demands, or a more general effect that strengthens the memory trace against diverse sources of forgetting.
While the finding that more time for consolidation of memory items leads to slower rates of forgetting is exciting, there is another theoretical approach that seeks to explain results such as that of Ricker & Cowan [18] without recourse to consolidation or decay. Temporal Distinctiveness Theory states that increasing the amount of free time before or after item presentation will increase memory accuracy by decreasing the temporal confusability of the memory item with other similar items [24,54]. Less confusable items are easier to recall, leading to better memory performance. According to this theory, increasing the time that I and others argue is used for consolidation [17,18,19] actually improves performance because the memory item becomes more temporally distinct from other memory items or distracters, not because the memory item becomes better consolidated. In order to be sure that short-term consolidation is at work temporal distinctiveness effects needed to be ruled out.
To investigate whether temporal distinctiveness is at work in visual-array change-detection tasks Ricker et al. [43] performed a series of four experiments using the same basic paradigm as Ricker & Cowan [18], but manipulated inter-trial interval length, the amount of time between memory trials, instead of consolidation times. Under the assumptions of Temporal Distinctiveness Theory manipulation of inter-trial interval duration and consolidation period duration should have the same effect on memory performance. If temporal distinctiveness effects are causing the consolidation effect then the theory predicts that increases in the duration of the inter-trial intervals should result in increases in memory performance just as increases in the duration of consolidation do. Ricker et al. did not find this result. Longer inter-trial intervals did not lead to slower rates of forgetting despite leading to conditions which should be more temporally distinct. This violates the core tenants of Temporal Distinctiveness Theory, ruling out temporal distinctiveness as an explanation of decay and consolidation effects in visual change detection. This finding of a null effect is not based upon a lack of evidence to reject the null. Bayes factor analysis was performed and demonstrated positive evidence in favor of a null effect in critical comparisons for all four experiments.
This work illuminates the possibility that consolidation and decay may explain many of the phenomena that are currently accounted for using temporal distinctiveness models in other contexts as well. In very few cases in the literature are consolidation effects considered as alternative explanations to temporal distinctiveness effects. A second look at many traditional findings is certainly warranted with an eye for whether consolidation may be at work.
5. Consolidation Happens Quickly
A particular difficulty in investigating short-term consolidation is that the process happens very quickly. In their early work, Jolicoeur & Dell’Acqua [19] found diminishing reaction time benefits for secondary task performance as total time available for consolidation approached two seconds. However, the major consolidation benefits seemed to occur within the first second. Clearly, a very fast sequence of events is needed to study short-term consolidation.
Despite the detailed study of the time course of short-term consolidation by Jolicoeur & Dell’Acqua [19], they did not observe any effect of consolidation time on memory performance. In order to directly test the time course of memory consolidation, Ricker [in preparation] examined memory for sets of randomly generated angles and observed how increases in consolidation time affected the error when reproducing these angles at memory test. In this study four random angle stimuli were presented in serial and 200 to 2200 milliseconds were given for consolidation after each item was presented and masked. This consolidation period was a brief period of free time after the mask of the current memory item and before the next memory item was presented. After all items were presented the participant reproduced the angle of each memory item in the order in which they were presented. If short-term consolidation improves memory performance by protecting memory traces against later forgetting, then the longer consolidation periods in this experiment should result in smaller errors when reproducing the presented angles at memory test.
In Experiment 1 of Ricker [in preparation] the time from memory item onset to mask offset was 600 ms, resulting in a minimum time available for consolidation from stimulus onset of 800 ms per item, and a maximum consolidation time from stimulus onset of 2600 ms per item. No effect of consolidation time was observed. Note that consolidation can begin at any point after encoding is completed, even when encoding is completed during stimulus presentation or before the masking period has ended. With the length of presentation time in Experiment 1 it is possible that consolidation was completed at some point before the item masking period ended. In Experiment 2, presentation and mask time was shortened to a combined 200 ms while the minimum additional time for consolidation before the next item onset was 200 ms and maximum additional consolidation time was 2000 ms. This results in a minimum time to complete consolidation from stimulus onset of 400 ms per item and a maximum time to complete consolidation from stimulus onset of 2200 ms per item. In this second experiment a benefit of increased consolidation time was observed. Changing the minimum available consolidation time from 800 ms to 400 ms per item was crucial. It appears that most of the consolidation of a single angle stimulus is completed by 800ms after stimulus onset, but still ongoing at 400 ms after stimulus onset.
The completion of consolidation in under a second makes it a difficult process to study, but well within the realm of other cognitive processes frequently investigated by cognitive psychologists. The real difficulty is in differentiating item presentation and encoding from a period during which only item consolidation occurs. With careful control of visual stimuli short-term consolidation can be brought under experimental control and studied. Research involving long presentation times or lacking post-perceptual masks is likely to miss consolidation effects entirely and declare them nonexistent despite the strong evidence in their favor found elsewhere.
Several important questions remain about the time course of short-term consolidation. For example, the reason that consolidation time is limited in duration is currently unknown. It could be that the consolidation period is short because short-term consolidation is completed very quickly or because the consolidation process can only function for a brief period of time immediately after item presentation. My own work has demonstrated consolidation periods using sequential presentation of items. If item consolidation is limited by a critical period, it is likely that simultaneous presentation of items is particularly vulnerable to deficits in short-term consolidation. This is because multiple items cannot be consolidated before the shared critical period ends.
6. Is Consolidation Distinct from Short-term Maintenance?
Short-term consolidation of memory items occurs at the leading edge of any maintenance period imposed upon memory. Unfortunately, we do not presently have a road marker that can tell us when consolidation has ended and when maintenance begins. Ideally we would have a procedure for this purpose that is similar in function to how post-perceptual masks differentiate consolidation from encoding processes. Without this procedure it is difficult to test if consolidation and maintenance processes are different from one another at all. It may be the case that short-term consolidation processes are the same as those that make up memory maintenance. For example, the consolidation process could be the early use of an attentional refreshing mechanism such as that proposed by Barrouillet & Camos [21]. According to these authors attentional refreshing is the focusing of attention on a memory trace in order to refresh it’s otherwise decaying activation. Following this theory, if the activation of any given trace decays lower than some threshold the item is forgotten.
Alternatively, short-term consolidation could be the initial steps of setting up an articulatory rehearsal loop. Naveh-Benjamin & Jonides [55] demonstrated that the first few seconds of articulatory rehearsal require attention, presumably because initiation of rehearsal is not attention free. Later repetitions within any given articulation loop appear largely attention free. It could be that short-term consolidation is this initial attention demanding period of articulatory rehearsal. At this point there is no definitive evidence to tell us whether short-term consolidation is the same as maintenance processing, but circumstantial evidence suggests that short-term consolidation is a process independent of any maintenance activities.
Vergauwe, Ricker, and Cowan [submitted] provide indirect evidence that suggests short-term consolidation and attentional refreshing occur through different mechanisms. In this study, participants remembered a series of sequentially presented letters for immediate recall. After presentation and masking of each individual item, participants were presented with a processing task that either required only the just-presented item to be kept in the focus of attention, as would be done during item consolidation, or mimicked attentional refreshing mechanisms by requiring participants to cycle all of the items in the memory list through the focus of attention. Performance was worse in the condition requiring participants to cycle all items through memory immediately after memory item presentation, mimicking attentional refreshing, as compared to when participants only needed to keep the just-presented item in the focus of attention, mimicking consolidation. If consolidation and attentional refreshing rely on the same cognitive process, then the refreshing-like processing task should not reduce performance relative to the consolidation-like task that allowed continual focus on the just-presented item. This is not what was found.
Unfortunately, this evidence is indirect. What Vergauwe et al. [submitted] tested was performance after a secondary task requiring either focal attention or refreshing-like memory scanning. This study did not test performance after a direct manipulation of focal-attention or attentional refreshing on memory independent of secondary task processing. It is possible that the secondary task itself disrupts consolidation regardless of the requirements and that the memory scanning task resulted in lower performance due to increased binding [49] or context based interference [25]. One could also argue that this task did not mimic attentional refreshing maintenance mechanisms because some authors conceive of attentional refreshing as a slower more deliberate process [56,57] and not a rapid cycling of items through the focus of attention. However several studies have shown that attentional refreshing must involve very fast cycling of memory items in order to be a plausible maintenance mechanism [52,58,59]. Slow attentional focusing as a mechanism of short-term maintenance just doesn’t fit the data.
More evidence in favor of the separation of a consolidation mechanism from either attentional refreshing or articulatory rehearsal is given by Bayliss, Bogdanovs, Jarrold [60]. This study explicitly tested whether consolidation and attentional refreshing are the same process. In the Experiments 1 and 2 the amount of attentional refreshing allowed and secondary task performance required were held constant across the retention interval. A free period of time was provided either at the beginning of the maintenance period, allowing short-term consolidation, or at the end of the maintenance period, preventing short-term consolidation. If short-term consolidation is the same process as attentional refreshing, then the location of the free period should not influence memory performance in this context. Improved memory performance was observed when consolidation was allowed even when the amount of attentional refreshing possible was unchanged and when secondary task difficulty was held constant. This was true across several levels of attentional refreshing availability and secondary task difficulty. If attentional refreshing and consolidation are the same cognitive process, then there should have been no consolidation benefit when there was no change in the amount of attentional refreshing possible.
The dichotomy between short-term consolidation and articulatory rehearsal has been more thoroughly established than the potential dichotomy between short-term consolidation and attentional refreshing. The main evidence is that the benefit to memory performance when given time for consolidation is unaffected by the presence or absence of articulatory suppression. This is a technique used to prevent articulatory rehearsal by requiring the participant to constantly repeat a single irrelevant word or phrase such as “one, two three, one, two, three…” If preventing articulatory rehearsal does not remove the benefit of providing time for consolidation, then it is hard to imagine how short-term consolidation could occur through articulatory mechanisms. Both Stevandowski & Jolicoeur [61], using a masking procedure, and Bayliss et al. [60], without use a masking procedure, found that the presence of articulatory suppression did not negate the benefits of providing more time for short-term consolidation.
Although the study of Bayliss et al. [60] seems to provide strong evidence in favor of consolidation as a unique cognitive process different from attentional refreshing and articulatory rehearsal, it should be noted that this series of experiments did not include post-perceptual masking of the memory stimuli. Without the masking stimuli it is possible that encoding processes were still underway during the period that was assumed to be used for short-term consolidation. If this is the case then the manipulations by Bayliss et al. that were to manipulate consolidation may actually have been manipulating encoding, or a mixture of encoding and consolidation processes.
The research thus far seems to indicate that maintenance mechanisms are not responsible for consolidation processes. While we have strong evidence against the consolidation process being a part of articulatory rehearsal, thanks to the masking procedure of Stevandowski & Jolicoeur [61], the same is not true for the relationship between consolidation and attentional refreshing. Studies investigating the relationship between consolidation and refreshing indicate that the two are different processes, but have either been indirect or have not used masking to differentiate encoding and consolidation processes. Future work following up on the existing literature is needed to confirm that attentional refreshing during the critical period immediately following encoding is not responsible for the short-term consolidation process.
7. Potential Neural Mechanisms of Short-term Consolidation
The previous sections of this work describe the impact of short-term consolidation on memory performance. This final section switches focus and speculates about plausible neurobiological bases for the short-term consolidation process. While the accuracy of these neural accounts of short-term consolidation is not necessary in order to demonstrate the existence of a short-term consolidation process, it would be helpful to link the cognitive process to a neural mechanism. This could help to inform us about when consolidation is likely to be effective and potentially allow researchers and clinicians to monitor and measure short-term consolidation without recourse to memory performance scores. This section outlines three potential neurobiological mechanisms through which short-term consolidation may be instantiated. These theoretical mechanisms are not necessarily exclusive of one another. Several may work together or function at the same time in order to reach the short-term consolidation benefits observed in the behavioral literature.
The most intuitively simple approach to understanding short-term consolidation may be through the long-term consolidation process. Long-term consolidation occurs through mechanisms involving the hippocampus and it’s interaction with the cortex [62,63,64]. Although changes to the cortex resulting from long-term consolidation are too slow to account for the short-term consolidation effects observed here, long-term potentiation within the hippocampus occurs on the order of milliseconds [65,66]. The resulting changes in hippocampal organization are needed before long-term consolidation can begin and occur on a timescale which could support short-term consolidation. If hippocampal traces contribute to performance in short-term memory tasks as some have argued [67,68,69,70], they may represent the best candidate for the neurobiological basis of short-term consolidation.
A second promising way in which short-term consolidation may function within the brain is by linking neural activity within the intraparietal sulcus to brain activity occurring within sensory and association areas. Activity within the intraparietal sulcus is thought to reflect the use of attention to track short-term memory representations that are present in other areas of the brain [71,72,73]. Limits on short-term memory capacity are thought to occur because the circuitry within the intraparietal sulcus is only able to track a maximum of 3-5 memory representations at one time [71,73,74]. It could be that short-term consolidation is the process of creating functional connectivity links between individual pointers in the intraparietal sulcus and the widely distributed memory traces.
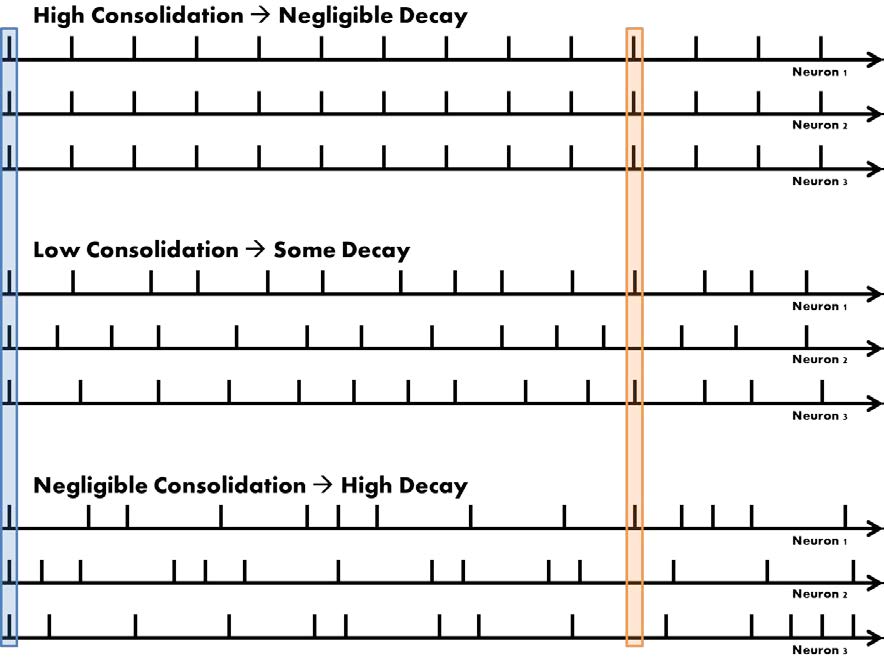
A third approach to understanding the neural correlates of short-term consolidation could be related to its function in reducing the impact of trace decay. Following theories of object binding which argue that disparate neural populations function as a single unit by firing in temporal synchronicity [75,76,77,78], trace decay may be a process through which neural populations gradually desynchronize their firing (see Figure 3). This could occur through the gradual accumulation of random noise in firing rates or because of internally generated interference from irrelevant thoughts and processing. In this approach to understanding decay, short-term consolidation would be a process which reduces the rate of desynchronization either by somehow strengthening the signal itself, relative to the accumulating noise, or by reducing the accumulation of noise.
8. Concluding Remarks
In the pages above I have provided a basic outline of what is known about short-term consolidation of memory. In brief, short-term consolidation improves memory performance by reducing decay-based forgetting and possibly forgetting from other sources as well. It is a separate process from encoding and likely to be a separate process from short-term maintenance. Short-term consolidation occurs very quickly, immediately after item presentation, and requires attention to function. It does not require the presence of the stimulus itself or the existence of a sensory memory trace in order to occur. The existence of a short-term memory trace is enough.
As with any expanding area of research, there is much more we don’t yet know about short-term consolidation than there is that we do know. In addition to the questions posed in the preceding sections, there are many further interesting unknowns. For example, we don’t yet know whether short-term consolidation is a process unique to short-term memory or if it also affects long-term memory. If short-term consolidation is the first stage of long-term consolidation, it could be that short-term consolidation is an improvement in long-term memory retrieval rates observed in short-term memory paradigms. This would be very much in line with the primary/secondary memory approach to working memory performance [23,79].
As investigation into short-term consolidation increases over the coming years we will learn more and fill in our knowledge of what the process is, what it is not, and how it functions on a neural level. Confusion over the meaning of the term consolidation by short-term memory researchers has often led to confounding encoding and short-term consolidation into a single process. This continues to be a problem which slows the accumulation of knowledge. The present work clarifies how short-term consolidation is different from encoding and serves as a guide to what is currently known about short-term consolidation.
Conflict of Interest
The author declares no conflict of interest.










 DownLoad:
DownLoad: