In this note, a regularity criterion of weak solutions to the 3D-Boussinesq equations with respect to Serrin type condition under the framework of Besov space ˙Br∞,∞.. It is shown that the weak solution (u,θ) is regular on if u satisfies
T∫0‖u(⋅,t)‖21+r˙Br∞,∞.dt<∞,
for 0<r<1. This result improves some previous works.
1.
Introduction
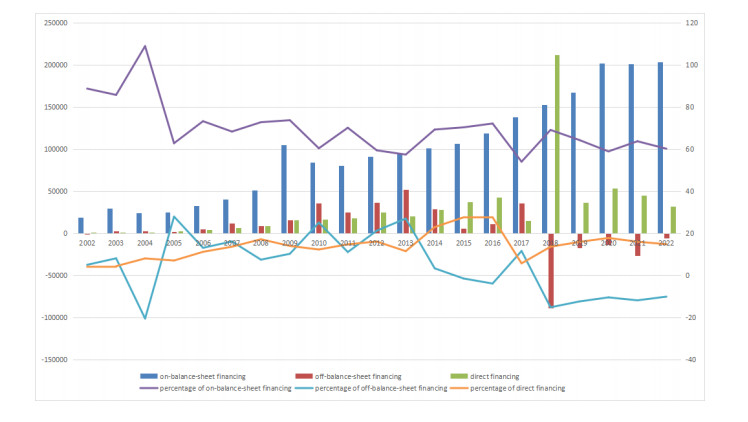
The development of the financial system plays a crucial role in economic growth. The social financing structure is based on the actual demand for funds and provides a comprehensive view of the financial system from a financing perspective. It demonstrates good representativeness and holds practical significance. As China's capital market continues to grow and finance undergoes continuous innovation, along with the ongoing progress of interest rate market-oriented reforms, various financing tools and methods have emerged, leading to a diverse and complex trend in the social financing structure, as illustrated in Figure 1.
According to Figure 1, the percentage of on-balance sheet financing in Aggregate Financing to the Real Economy has decreased from 88.73% in 2002 to 60.10% in 2022. It has maintained at around 60%. This indicates that despite policy changes leading to a decline in the proportion of RMB loans and foreign currency loans in Aggregate Financing to the Real Economy, they remain unbalanced and constitute a significant share. On the other hand, the proportion of off-balance sheet financing has fluctuated greatly and has been negative this year. This could be attributed to the introduction of various policies and measures by regulatory authorities to regulate traditional shadow banking and interbank business, which has impacted entrusted and trust loans to some extent. Direct financing in Aggregate Financing to the Real Economy has shown a gradual increase from 4.06% in 2002 to 14.79% in 2022. It has surpassed 20% at certain points during this period. In recent years, China's policy aims to increase the proportion of direct financing and enhance its role in promoting the real economy. As China's capital market develops, the scale of direct financing is steadily growing. Consequently, the financial system is transitioning from a bank-led structure to a market-oriented structure, resulting in a declining role of banks as the main channel of social financing and a continuous rise in the proportion of direct financing in Aggregate Financing to the Real Economy.
China's financing channel structure primarily relies on indirect financing. Yi [1] suggests that developing direct financing, particularly equity financing, is crucial in reducing excessive dependence on bank debt financing and ensuring continued financial support for the real economy. Therefore, it is essential to maintain a reasonable range for the social financing structure to avoid hindering economic development. For instance, if bank credit accounts for a significant proportion, it can lead to increased investment risks, destabilize investment, and impede economic growth. Guru and Yadav [2] highlighted the interdependent relationship between the banking industry and the stock market in stimulating economic growth. Zhang et al. [3] emphasize that banks continue to play a pivotal role in supporting economic growth and facilitating industry transformation, while He and Wei [4] focus on the emerging role of non-bank credit as new market-oriented financing channel. Consequently, investigating the precise influence of the social financing structure on economic growth is imperative. Although existing literature has examined the impact of social financing structure on overall economic growth, limited attention has been given to its effects on specific industry sectors, particularly across multiple sectors. For instance, Ning et al. [5] highlight that bank loans are the primary source of funding for energy efficiency projects, but they have proven to be insufficient. They suggest that green bonds (direct financing) can also contribute to enhancing energy development. Zeng et al. [6] identified credit financing and equity financing as critical determinants for strategic emerging industries. Notably, the financing preferences of various industry sectors can vary, with some industries favoring bank loans while others opting for equity financing. Therefore, this study aims to explore the influence of social financing structure on diverse industry sectors, seeking to elucidate the distinct impact of different financing structures on sectoral development.
Thus, we aim to provide new insights into the impact of social financing structures on the economic growth of various sectors from two perspectives. First, we classify the social financing structure at the macro level and explores its impact on multiple industry sectors. While researchers have focused on a single sector or the real economy, we examine all industries and offers new ideas to understand the impact mechanism of social financing structure on economic growth. Second, we investigate the impact of financing structure on multiple industry sectors using a large TVP-VAR model, which complements previous research. Due to the limitations of model dimension and the difficulty of modeling and limited explanatory capacity of conventional economic theory models when applied to high-dimensional big data [7,8], previous studies have often employed smaller models with fewer variables. These studies have focused on TVP-VAR models, threshold regression models, GMM estimation methods, and similar approaches. To address these limitations, we adopt a new perspective by utilizing high-dimensional methods. Specifically, the large TVP-VAR model is employed for empirical analysis to systematically investigate the transmission mechanisms of different financing methods to various industry sectors in China. This approach enables a more comprehensive and in-depth analysis of the impact mechanism of financing structure on different industries. It is worth noting that we can help evaluate the impact of different financing structures on the risk tolerance of industry sectors by considering time-varying spillover effects. More importantly, before constructing the large TVP-VAR model, this research carefully selected relevant time points using the time-varying spillover index model to provide empirical evidence for further analysis. The time-varying spillover index model utilized in this study is advantageous as it is resilient to outliers due to the implementation of multivariate Kalman filtering methods. Additionally, it eliminates the need for arbitrarily chosen rolling window sizes, preventing the loss of valuable observations [9,10].
The paper is structured as follows: Section 2 presents a literature review, Section 3 introduces time-varying spillover index model and large TVP-VAR model methods, Section 4 covers preliminary analysis and data processing, Section 5 showcases the empirical discoveries, and Section 6 concludes the paper with recommendations.
2.
Literature review
The study by Gurley and Shaw [11] introduced the concepts of 'direct financing' and 'indirect financing'. They explained that stock financing and bond financing are typical forms of direct financing, while bank credit financing is a typical form of indirect financing. Bank lending is a primary source of corporate financing in many developing countries [12,13]. Mishkin [14] emphasizes that indirect financing is more significant than direct financing for most countries. Hence, a high proportion of indirect financing is a common phenomenon. However, it is important to maintain the proportion of indirect financing within a reasonable range rather than pursuing a 'bigger is better' approach. Allen et al. [15] and He and Wei [4] highlight that China's financial system is largely dominated by an inefficient banking sector, that is, RMB lending being predominant. Zhao et al. [16] also note that China's financing channel structure primarily relies on indirect financing. Therefore, it is necessary to promote direct financing and develop multi-level capital markets to better serve the real economy [17].
Different scholars have expressed varying opinions on the relationship between different financing methods. Feng et al. [18] argue that the growth of direct financing, particularly the bond market, has adversely affected traditional credit business. On the other hand, Levine [19] and Beck and Levine [20] propose a contrasting viewpoint, suggesting that direct and indirect financing are complementary rather than substitutes, offering similar financial services. Nizam et al. [21] discovered that financing channels have a notable positive effect on bank financial performance. In a related study, Guru and Yadav [2] demonstrated that the development of the banking industry and stock market are mutually supportive in fostering economic growth. In a study by Bansal et al. [22], it was discovered that all types of financing funds, except for Istisna financing, had a positive impact on economic growth. Qian and Yeung [23] and Zhang et al. [3] emphasize that banks continue to play a pivotal role in supporting economic growth and facilitating industry transformation.
Through a review of the literature, scholars have examined the influence of financing structure and proposed reforms using textual analysis, such as Zhao et al. [16] and Yi [1]. Empirical research in this area primarily focuses on the relationship between financing structure and the economy. For instance, Benczúr et al. [24] conducted a study that empirically demonstrated the nonlinearity of financing on economic growth. Additionally, there have been empirical investigations into the impact of various financing methods on specific industries. For example, He et al. [25] have investigated the influence of bank credit on investment in the renewable energy sector.
Through empirical research, scholars have found that different financing methods have varying impacts on economic growth. Deng et al. [26] demonstrated the significant contribution of direct financing to economic fluctuations using a TVP-VAR model, while the development of indirect financing remains an important driver of economic prosperity. Cheng and Degryse [27] and Zhang et al. [28] empirically concluded that bank credit, or on-balance sheet financing, can promote economic growth. However, Mishra and Narayan [29] suggested that the banking sector exerts a noteworthy and adverse influence on the progression of the economy. Additionally, Hsu et al. [30] found that the development of credit markets may hinder innovation in industries that rely on external financing and are highly high-tech-intensive. Law and Singh [31] highlight the nonlinear relationship between bank credit (indirect financing) and economic growth. The literature presented above demonstrates the significance of indirect financing in economic growth. However, several empirical studies conducted by scholars have highlighted the influence of direct financing on economic growth. For instance, Tian [32] empirically established a positive relationship between direct financing and GDP in the long and short run. Xiang et al. [33] highlighted that developed countries rely on equity financing and debt financing as the primary sources of funds for corporate innovation. They emphasized that corporate innovation serves as a key driver for economic growth, drawing insights from the experiences of these countries. Fanta and Makina [34] discovered that bond market financing influenced the development of the South African economy. Zhang et al. [35] point out that the issuance of green bonds (direct financing), which provides direct financing, not only expands financing channels but also fosters green development, and green development plays a significant role in driving national economic activities [36]. Additionally, Pradhan et al. [37] found that bond market development may have a long-term impact on economic growth. Beck and Levine [12] observed a positive influence of stock markets and banks on economic growth. Conversely, Singh [38] argued that a fragile stock market, such as an immature one, can undermine economic growth. Holmström and Tirole [39] demonstrated that the expansion of stock markets and increased liquidity contribute to firm production and economic growth. Jin et al. [40] highlighted the critical role of the bond market in financing for direct financing, surpassing the stock market.
In studies on financing structure and inter-industry, Anton and Nucu [41] discovered that bank credit (indirect financing) and bond issuance (direct financing) have a beneficial effect on renewable energy sources. Wang et al. [42] found that corporate governance mechanisms mainly influence the investment behavior of energy-intensive enterprises by adjusting long-term credit supply (indirect financing, proportion of bank credit), thereby affecting the energy economy. Ouyang and Li [43] argue that total credit plays a negative role in both China's economic growth and energy consumption. In contrast, Ning et al. [5] highlight that bank loans are the primary source of funding for energy efficiency projects, but they have proven to be insufficient. They suggest that green bonds (direct financing) can also contribute to enhancing energy development.
The existing literature primarily focuses on the relationship between specific aspects of financing structure and economic growth, such as bank credit in indirect financing and the relationship between the stock market and economic growth in direct financing. However, there are limited studies that consider the entire financing system and its impact on the economy, and even fewer studies that explore the impact of different components within the social financing structure on industry-specific economic growth. We aim to provide new insights into the impact of social financing structures on the economic growth of various sectors from two perspectives. First, we classify the social financing structure at the macro level and explores its impact on multiple industry sectors. While previous research has mainly focused on a single sector or the real economy, we examine all industries and offer new ideas to understand the impact mechanism of social financing structure on economic growth. Second, we investigate the impact of financing structure on multiple industry sectors using a large TVP-VAR model, which complements previous research. Due to the limitations of model dimension and the difficulty of modeling and limited explanatory capacity of conventional economic theory models when applied to high-dimensional big data [7,8], previous studies have often employed smaller models with fewer variables. These studies have primarily focused on TVP-VAR models, threshold regression models, GMM estimation methods, and similar approaches. To address these limitations, we adopt a new perspective by utilizing high-dimensional methods. Specifically, the large TVP-VAR model is employed for empirical analysis to systematically investigate the transmission mechanisms of different financing methods to various industry sectors in China. This approach enables a more comprehensive and in-depth analysis of the impact mechanism of financing structure on different industries.
3.
Models and methods
3.1. Time-varying spillover index
Various scholars have employed different spillover index methods to assess the dynamic connectivity among variables in financial markets (see [44,45,46,47,48,49,50,51,52,53]). In this article, we will utilize the time-varying spillover index method, and the construction method of the time-varying fluctuation spillover index is an extension of the fluctuation connectivity method proposed by Diebold and Yilmaz [54]. Antonakakis et al. [9] builds on the idea of Koop and Korobilis [55], allowing the variance and covariance matrix to pass the Kalman filter with a forgetting factor to estimate changes in the construction of the time-varying volatility spillover index. This method offers two advantages over the rolling window spillover effect. First, it overcomes the challenge of selecting an arbitrarily sized rolling window. Second, it avoids the loss of valuable observations. Based on the Wold representation theorem, the vector moving average (VMA) representation is obtained by transforming the TVP-VAR model:
where Mt=(At0Im(p−1)0), ηt=(εt,0,⋯,0)′, J=(I,0,⋯,0)′, zt−1=(yt−1,yt−2,⋯,yt−p)′, At=(A1t,A2t,⋯,Apt)′. Mt is a mp×mp matrix, ηt is an mp×1 vector, J is an mp×m vector. When k approaches infinity, it is equivalent to:
where Bjt is a m×m matrix, GIRFs(Ψij,t(H)) represents the response of all variables j after the impact of variable i. The difference in the forecast of H-step forward is calculated as follows:
where ej is a m×1 vector of dimensional columns, where the m-th position element is 1 and the rest are 0. The calculation GFEVD(ϕij,t(H)) reflects the connectivity of the two directions from j to i and illustrates the effect of variable j on i in terms of the variance share of the prediction error. We normalize all variances so that they sum to 1. The formula is as follows:
The following formula shows how to calculate the spillover index in different cases:
(1) The total spillover index
(2) The directional spillover index
The directional spillover from market i to all other markets j:
The directional spillover imparted by all other variables j to variable i:
(3) The net total directional connectedness:
3.2. Large TVP-VAR model
Here, we can write TVP-SVAR as:
where B0,i,⋯,Bp,t are n×n matrix, yt,t=1,⋯p is an n×1 vector, B0,t is an n×n lower triangular matrix with diagonal elements of ones, and ∑t=diag(exp(h1,t),⋯,exp(hn,t)). If we set Bt=1−B0,t, matrix Bt has zeros on the diagonal. The TVP-SVAR can now be written as:
where b1,t=vec(B1,t),l=1,⋯,p and bt=vec(Bt), where bt contains all the n(n−1)2 non-zero elements of Bt in a vector and D is an n2×n(n−1)2 selection matrix. If we define an n×k matrix:
where k=(np+1+n−12)n. Let αt=(μ′tb′tb′1,t⋯b′p,t)′, We can rewrite Eq (13) as:
According to Chan et al. [56], the speed of model estimation can be improved by applying a rank Qa reduction to the covariance matrix for the state equation. If we set the rank Qa to ra=rank(Qa)£k, and use recentering and parameter expansion [57], the model can be written as follows in Eqs (15) and (16):
where Aα is a k×rα matrix, fα,t and zα,t are rα×1 matrix, and εt and zt are independent, and since rα is usually much smaller than k, the Eqs (17) and (18) are referred to as the reduced sources of error model.
4.
Data
4.1. Data description
In this study, we examine the influence of social financing structures on various industry sectors. Utilizing a time-varying spillover effect model and a large TVP-VAR model, we conduct detailed analysis to determine the effects of different financing structures on the growth of diverse industry sectors. The variables chosen for this study include:
(1) Indicators of financing structure: The scale of social financing can effectively monitor cross-institutional, cross-market, and cross-industry capital flows. Zhao et al. [16] pointed out that the composition ratio of the social financing scale can provide insights into the changes in China's financing structure. Following the approach of Deng et al. [26] and He and Wei [4], we consider the indicators of on-balance-sheet financing (OBS), off-balance-sheet financing (OBSF), and direct financing (DF) as a proportion of the total social financing scale. This selection of indicators allows for a more accurate reflection of the structural changes in the financing system.
(2) Indices of different industries: We aim to investigate the influence of changes in the social financing structure on multiple industry sectors. Industry indices are indicators that reflect the development of various industries in the market, offering insights into overall trends and market conditions within each industry. To select appropriate industry indices for analysis, previous scholars have commonly utilized indices such as the Shanghai and Shenzhen 300 Index [58], the Shanghai Stock Exchange Sector Indices [59,60], and the Wind Industry Index [61] to investigate the effects of different indicators on market fluctuations. However, due to significant missing data in both the CSI 300 and SSE 500 indices over the chosen time span, we will select 11 industry indexes in the Wind industry index, according to the global industry classification standard, namely Energy Index (ENERGY), Material Index (MATERIAL), Industrial Index (INDUSTRY), Optional Consumption Index (OPT CONS), Daily Consumption Index (CONS), Health Care Index (HEALTH), Financial Index (FINANCIAL), Real Estate Index (REAL ESTATE), Information Technology Index (INFO TECHN), Telecom Services Index (TELECOM), and Utilities Index (PUBLIC). Subsequently, logarithmic difference processing will be applied to each index to analyze and measure the information on the development of different industries.
The monthly data indicator on the scale of social financing was first published in 2012, and its monthly data since 2002 was published in September 2012. In order to make the best use of the information in the data, the sample data range selected is from January 2002 to March 2023.
4.2. Descriptive statistics
A preliminary analysis of the data was conducted, and Table 1 displays the descriptive statistics on yields and financing structure indicators for eleven industry indices. The analysis reveals the following insights on the different industries: the average yield is positive for all industries, with CONS having the highest average yield of 0.42%, while REAL ESTATE has the lowest yield of 0.15%. Notably, CONS and HEALTH experienced their maximum yields during the pandemic, indicating varying impacts of the pandemic on different industries. The standard deviation highlights that INFO TECHN exhibits high volatility, while PUBLIC has minimal volatility. The skewness coefficient indicates that ENERGY, FINANCIAL, and REAL ESTATE have left-biased yields, with negative returns outweighing positive returns. On the other hand, the earnings distribution in other industries is right-skewed, indicating that positive returns outweigh negative returns. Furthermore, the kurtosis coefficient reveals that all industry yields have large kurtosis values and exhibit a typical peak shape.
The descriptive analysis of the financing structure reveals that on-balance sheet financing constitutes an average of 69.70% of social financing, while off-balance sheet financing accounts for 4.77%. This implies that indirect financing makes up 74.47% of social financing, whereas direct financing also accounts for 14%. This proportion aligns with the financing structure of my country. In the context of China's development, indirect financing has traditionally played a dominant role. Scholars have also explained that the long-term dominance of indirect financing has a negative impact on the economy. Furthermore, China's policies reflect the need to enhance the proportion of direct financing in order to effectively drive economic development. Notably, the standard deviation of off-balance sheet financing is the highest, indicating greater volatility compared to other financing proportions. Direct financing exhibits the lowest volatility.
Second, we generated a heat map displaying the correlation coefficients between various financing methods and industries, as shown in Figure 2. Our analysis revealed that, within a linear relationship, on-balance sheet financing significantly influences the development of industries, with the exception of ENERGY and PUBLIC. Conversely, direct financing did not exhibit statistically significant impacts on industries.
4.3. Data validation
To ensure the avoidance of spurious regression, it is essential to perform stationarity examinations on every dataset, given that the primary data series utilized in the investigation are time series. we employ ADF and PP to ascertain the stationarity of the aforementioned series.
As shown in Table 2, the ADF and PP unit root tests were performed on the original sequences. The results indicated that the sequences passed the test at a significance level of 1%, rejecting the null hypothesis of the unit root. This suggests that the data were stationary and satisfied the homogeneous single integer condition, allowing for the establishment of the model.
In this study, we aim to determine the optimal lag lengths for constructing a large TVP-VAR model. By applying the minimum AIC and FPE principles, we find that the optimal lag length is set to 1. The findings of model selections for both rα (number of states of the mean equation) and rh (number of states driving volatility) are presented in Table 3. To compare different models, we utilize the Deviation Information Criterion (DIC) as a model comparison criterion, following the method proposed by Chan and Eisenstat (2018). The DIC values are shown in Table 4. For a model consisting of 14 variables, DIC suggests choosing a model with 9 states, where 3 states drive the mean equation coefficient in αt and 6 states drive the volatility in th.
5.
Empirical results and analysis
In this section, we aim to analyze the impact of financing structure on various industries using two approaches. First, we will examine the relationship between them through the time-varying spillover index. Second, we will utilize the time-varying spillover index to identify a suitable time point for the subsequent large TVP-VAR model, which serves as an empirical foundation. Last, the large TVP-VAR model is employed to enhance our analysis of the time-varying spillover effects.
5.1. Time-varying spillover conclusions
The average volatility spillover index alone is insufficient in capturing the dynamic evolution and cyclical fluctuation of systemic risk. Therefore, this study employs the R language to calculate the spillover index for conducting a detailed analysis of numerical fluctuation transmission. It also compares the fluctuation spillover between different industries and financing structures, considering both time and direction. To identify the periods when the impact of the financing structure is more pronounced, we first plot the TOTAL spillover index. Figure 3 provides a visual representation of the time periods with high spillover index in the entire system. Further analysis of these periods can be done using the NET spillover index.
Since 2002, the beginning of the sampling period, it has been observed that the entire system has experienced a high level of spillover, with noticeable spillover effects between different industries and financing structures. The results indicate a strong risk spillover effect and linkage between various industries and financing structures in China, reaching a maximum of 77.75%. Moreover, the overall spillover index of different industries has shown cyclical fluctuations due to the impact of events such as the subprime mortgage crisis, stock market crash, and the US epidemic. These fluctuations reflect the ability of different industries to respond to external macroeconomic changes. Following 2012, my country adopted a proactive fiscal policy and a prudent monetary policy. In light of the new normal of economic development, China's economy has achieved relative stability. However, it is important to note that certain risks exist. The 'stock market crash' outbreak in 2015 led to the spread of systemic risks across industries, resulting in a sharp increase in the risk spillover index. In 2017, the government emphasized the need to strengthen the supervision of financial institutions, which yielded some positive results in the transformation of China's financial system from virtual to real, gradually reducing the risk spillover value. From 2018 onwards, the financial sector has been directly affected by the trade dispute between China and the United States, leading to an overall increase in the risk spillover index. In 2020, in response to the COVID-19, the level of spillover across the system decreased significantly. The highest value of the total spillover index was recorded in December 2017, with a value of 77.75%.
In the TOTAL spillover index chart, three significant time periods are identified for further analysis. These periods include the 'financial crisis' stage starting in June 2008, the 'stock market crash' stage starting in June 2015, and the 'new crown epidemic' stage starting in January 2020. These stages exhibit high spillover levels and rapid increases in spillover. During this period, there may be significant interactions among various industry sectors and financing structures.
Figure 4 utilizes the NET spillover index to accurately assess the impact of various industries and financing structures. The NET spillover index provides insights into the direction of fluctuations. Negative (positive) values indicate that the risk spillover received (transmitted) by a particular indicator is greater than the risk spillover it transmits. This helps to evaluate the risk of receiving or transmitting spillover.
The results presented in Figure 4 demonstrate that the NET spillover index of various industries and financing structures exhibits significant time-varying characteristics. Moreover, it is observed that major emergencies have a more pronounced impact. Specifically, when a major emergency occurs, the risk spillover index of each industry shows a similar trend to the spillover risk index of the corresponding structure. Moreover, during the entire time period, the ENERGY, CONS, HEALTH, FINANCIAL, REAL ESTATE, INFO TECHN, and TELECOM industries were the recipients of the entire spillover index. OBS and OBSF were the transmitters of the entire spillover index, with absolute risk dominance. Dogan et al. [50] highlighted the significant role of renewable energy as a net receiver and its increased negative connectivity during the epidemic. In the 'stock crash' phase, the absolute value of the NET spillover index of most indicators increased. Although most industries did not change their role direction during this stage, they did increase their influence. The MATERIAL, INDUSTRY, OPT CONS, and PUBLIC industries became recipients of risk during the 2015 'stock crash', and during the COVID-19, MATERIAL, INDUSTRY, and OPT CONS have shifted roles and now serve as vectors for transmitting risks. This was because during the epidemic, everyone's consumption did not decrease. On the contrary, due to hoarding of materials, consumption was promoted. The degree of spillover fluctuations in DF is not stable compared to other indicators. It is noteworthy that DF consistently receives the highest spillover index. Upon analysis of the time-varying graph of the net spillover index, it is evident that the majority of industries act as the primary recipients of risk, whereas OBS and OBSF primarily function as transmitters of risk.
Table 4 presents the average volatility spillover index for the entire time period, providing a summary of the situation across all time periods. First, upon comparing the diagonal elements of the spillover matrix, it becomes evident that the spillover within different industries is higher than the spillover effect between industries. Additionally, all industries are primarily influenced by their own lagging effects. Notably, the TELECOM industry exhibits the highest internal risk spillover intensity, reaching up to 18.2%. This can be attributed to the telecommunications service industry's strategic, foundational, and leading nature within China's economic system. It possesses strong penetration and plays a significant driving role, resulting in high-risk characteristics for enterprises operating in this industry. Conversely, the internal risk spillovers for MATERIAL, INDUSTRY, OPT CONS, and PUBLIC are relatively small, at approximately 11%. The spillover effect of the financing structure, influenced by itself, possibly due to the fact that the regulation of the financing structure is not limited to specific industries and is instead regulated based on economic development.
Second, the 'FROM' column represents the sum of accepted fluctuation spillovers. Among the industry sectors listed in this column, MATERIAL have the highest value at 88.9%, while TELECOM have the lowest value at 81.8%. The MATERIAL, INDUSTRY, OPT CONS, and PUBLIC are the major recipients of volatility shocks, whereas the TELECOM is the least affected by spillover effects from other industries. This could be attributed to the late emergence and relatively less interconnectedness of my country's trust industry with other sectors. OBS, OBSF, and DF serve as receivers of spillover effects in the entire system. The total receiving spillover index is even lower than the total spillover index of the financial system, standing at only 47%. OBS and OBSF are the major senders of spillover effects in the entire system. This indicates that these types of financing are the primary recipients of volatility impacts. The 'TO' row represents the sum of the share of fluctuation spillovers from different industries and monetary policies to other objects. The maximum value in this row is 97.6% for the INDUSTRY, signifying that it is the main sender of fluctuations, and other industries should be particularly mindful of its spillover effects. From the analysis of mutual spillover effects between different industries, it can be observed that MATERIAL, INDUSTRY, and OPT CONS are not only highly affected by external shocks, but also major contributors to fluctuations. On the other hand, TELECOM is the least affected by external shocks and exhibit the least volatility. Direct financing, similarly, experiences minimal impact from external shocks and shows the least exposure to fluctuations. The 'NET' row in the table represents the disparity between the column sum of different industries and financing structures and its own row sum. This value indicates the share of net volatility shock contributed by all industries and financing structures. Among these, OBS has the highest value at 35.8%, while the TELECOM industry index has the lowest value at −25.3%. In terms of net spillover index across various industries and financing structures, INDUSTRY emerges as the primary sender of fluctuations, whereas the TELECOM serves as the main receiver. Analyzing the net spillover index of different industries and financing structures, it is evident that ENERGY, CONS, HEALTH, FINANCIAL, REAL ESTATE, INFO TECHN, TELECOM, PUBLIC, and DF are net recipients of risks. Conversely, materials, industry, consumer discretionary, OBSF, and OBS financing are net spillovers of external risks.
Finally, the 'TCI' represents the total spillover index of the system, which is 76% in this table. This indicates that, on average, 76% of the volatility impact received by different industries and financing structures originates from other sources. This highlights the interconnectedness of various industries and financing structures in my country, and the significant degree of risk fluctuation spillover.
The construction of the spillover index confirms the presence of significant spillover effects between financing structures and various industries. Building upon this, we utilize the large TVP-VAR model in MATLAB to construct a three-dimensional impulse response and time-point impulse response in order to analyze the influence mechanism of financing structure on multiple industries. The impact effect not only examines the long-term influence of different financing methods on the industry at a specific point in time, but also indicates the positive and negative directions of the impact, whether it enhances or inhibits the effect. This analysis will effectively address the issue of insufficient analysis of the lag effect of spillover effects. Consequently, the next step involves establishing and analyzing the impulse response function.
5.2. Large TVP-VAR model
5.2.1. Stereo impulse response analysis
To examine the dynamic effects that change over time, we employ a large TVP-VAR model in MATLAB to construct a three-dimensional impulse response function. This function allows us to analyze the impact mechanism of financing structure on various industry sectors. The lag order of the impulse response is set to 12th order, which corresponds to the response of different industry sectors in the next year after being impacted by one unit.
First, the three-dimensional time-varying impulse response of on-balance sheet financing is depicted in Figure 5. It is evident that different industry sectors exhibit non-smooth responses over time, indicating strong time variability in their responses to OBS across all 12 periods. The figure reveals that there was significant turbulence in different industry sectors during the stock market crash. Although the shape of the response to OBS is similar across different industry sectors, the intensity varies. The intensity of responses in industries such as HEALTH, FINANCIAL, REAL ESTATE, and INFO TECHN appears to be notably higher compared to other sectors, aligning with our findings on the time-varying spillover index. Specifically, on-balance sheet financing exerts a short-term suppressive impact on the ENERGY. This finding is in line with Uddin et al. [62], who suggest that the banking sector hinders energy consumption, consequently impeding industry growth.
Second, the three-dimensional time-varying impulse response of off-balance sheet financing is illustrated in Figure 6. This figure demonstrates that the impact of the proportion of OBSF on different industries exhibits significant time variability across all 12 periods. The response of various industry sectors during the financial crisis was not particularly dramatic, possibly due to the relatively low level of development of China's OBSF. Consequently, the impact on different industry sectors was not substantial. Conversely, during the stock market crash, the response from different industries was relatively small but predominantly negative, indicating that OBSF hindered economic development. Moreover, during the COVID-19, the negative response from different industry sectors was exceptionally strong. This could be attributed to the fact that companies delayed the resumption of work and production after the outbreak due to the need for epidemic prevention and control, thereby affecting various industries.
Finally, the three-dimensional time-varying impulse response of direct financing is analyzed in Figure 7. The figure demonstrates that the impact of DF on different industries exhibits strong time variability across all 12 periods. Notably, there has been a significant change in the impact of DF on different industries since 2015, particularly during the 'stock market crash' period. This suggests that China's financing structure, which is transitioning from banks (indirect financing) to the stock market and bond market (direct financing), has played a role in influencing different industry stocks. The opening of the 'Shanghai-Hong Kong Stock Connect' at the end of 2014 further supports the stock market and direct financing. Starting from 2015, DF has had a positive impact on most industries, aligning with the findings of Tian [32]. Xiang et al. [33] highlighted that both debt financing and equity financing (direct financing) can incentivize corporate green innovation, ultimately driving progress in industries, particularly those dependent on technological advancements. During the COVID-19, DF has promoted the HEALTH, INFO TECHN, and TELECOM industries, while inhibiting others. This can be attributed to the effective promotion of the healthcare industry due to the COVID-19, as well as national control measures restricting people's outdoor activities. Consequently, companies have resorted to delayed work starts and online offices, leading to an increased demand for information technology. Furthermore, it is observed that DF has a negative impact on OBS, consistent with the findings of Feng et al. [18].
5.2.2. Time-point impulse response analysis
Based on the conclusion of the time-varying spillover effect during the sample period, we conducted a time-point impulse response analysis on three specific time points. The findings are presented in Figures 8−10. It was observed that the various financing structures in China have distinct impacts on different industry sectors.
For on-balance sheet financing, during the financial crisis, OBS had a significant negative impact on various industries, but stabilized in the 5th period. Particularly, the HEALTH, FINANCIAL, REAL ESTATE, INFO TECHN, TELECOM, and PUBLIC were greatly affected. This could be attributed to the fact that on-balance sheet financing constituted a significant portion of social financing during that period, and its economic influence on the industry was of greater significance. The impact on industries during the 2015 'stock market crash' also varied over time. With the exception of the CONS and FINANCIAL, most industries experienced negative impacts followed by positive impacts and eventually reached a stable state, although the degree of response varied. The CONS industries consistently showed negative responses, but the degree of response initially increased, then decreased, and finally leveled off in the 5th period. On the other hand, the FINANCIAL consistently showed positive responses, indicating that OBS had a promoting effect on this industry during the 'stock market crash'. It is worth noting that the INDUSTRY sector exhibited a stronger inhibitory effect during the 'stock market crash' compared to the financial crisis. Under the impact of the COVID-19, all industries have been negatively affected, but the HEALTH has experienced the least inhibitory effect. This may be attributed to the fact that the main challenges posed by the COVID-19 are primarily concentrated on the supply side. Despite the existing market demand for products, there are several issues hindering the procurement of raw materials and impeding the smooth execution of the company's production and operational activities. Consequently, this situation has a detrimental impact on other industries. The major challenges faced by the epidemic demand are primarily concentrated on the supply side. The disruptions to the supply chain have caused difficulties for companies in obtaining materials and goods necessary for their operations. This has also hindered their ability to effectively carry out normal production and operational activities (Khan et al. [63]), thereby impacting other industries. Throughout the epidemic, the country has continuously strengthened financial services, increased support for the real economy, and provided assistance in epidemic prevention and control. This also demonstrates the positive impact of the central bank's actions on the real economy (Ahmed et al. [64]).
For off-balance sheet financing, the impact on the industry varies at different points in time and is heterogeneous. During the financial crisis, OBSF had a significant dampening effect on various industries, but stabilized in Phase 3. In particular, it had the greatest inhibitory effect on the ENERGY, MATERIAL, INFO TECHN, and PUBLIC sectors. In response to the 'stock market crash' in 2015, all industries showed a positive response, with the FINANCIAL sector being the most strongly promoted by OBSF. Except for the ENERGY, OPT CONS, HEALTH, and TELECOM sectors, other sectors were negatively affected by the COVID-19 pandemic. OBSF facilitated the HEALTH sector the most, while the TELECOM sector was facilitated the least.
For direct financing, the response of different industries to DF varies over time. During the financial crisis, the impact of DF on different industries was minimal and stabilized in the third period. Particularly, the promotion effect on the HEALTH and REAL ESTATE was negligible. In the aftermath of the 2015 'stock market crash', all industries experienced negative responses, but the trends and degrees of impact differed. The FINANCIAL was most severely affected by DF. This can be attributed to the higher proportion of DF in the financial industry and the suppression of financing methods during that period. Amidst the impact of the epidemic, industries responded differently to direct financing. REAL ESTATE and TELECOM experienced positive effects, while MATERIAL and FINANCIAL responded negatively. The HEALTH primarily showed negative response, possibly due to the impact of the epidemic and the decline in consumer spending, which in turn raised concerns in the capital market about the country's economy and its impact on investments.
In summary, the impact of various financing methods on the economic development of different industry sectors is influenced by external events, and the extent of their impact varies. In terms of numerical analysis, direct financing has a weaker impact on different industries compared to on-balance sheet and off-balance sheet financing. These findings align with our results using the time-varying spillover index method. On-balance sheet and off-balance sheet financing are considered risky, with different industry sectors being more affected as senders of risk, while direct financing, as the recipient, has a less significant impact on other industry sectors. However, it plays a role in the economic development of various industries. This could be attributed to the incomplete development of China's stock market.
5.3. Discussion
In this article, we present two innovative aspects that offer a fresh perspective on the relationship between social financing structure and economic growth across various industry sectors. First, we classify the social financing structure at the macro level and explores its impact on multiple industry sectors. While previous research has mainly focused on a single sector or the real economy, this paper examines all industries and offers new ideas to understand the impact mechanism of social financing structure on economic growth. Second, it investigates the impact of financing structure on multiple industry sectors using a large TVP-VAR model, which complements previous research. The impulse response analysis yielded several key findings. First, there is variability in how the financing structure affects different industries. Second, the influence of various industries on the proportion of direct financing shifted notably after 2015, suggesting that during the 'stock market crash', direct financing began to have a significant and largely positive impact on various industries. Last, the effects of different financing mechanisms on the economic advancement of diverse industry sectors are subject to external events, with varying degrees of impact.
Different financing methods have varying effects on industry development due to the unique characteristics and needs of each industry. For instance, the FINANCIAL may rely more on debt financing, as supported by our findings. In a three-dimensional analysis comparing the impact of different financing methods on finance, off-balance sheet financing emerges as having a stronger influence. This is because shadow banking offers enterprises alternative financing options like debt financing and trust financing, impacting their financing channels and cost structures, consequently affecting financial industry development. Moreover, HEALTH may lean towards traditional bank loans, a stable and reliable financing option suitable for larger medical institutions or projects. As depicted in the three-dimensional diagram, on-balance sheet financing has the most significant impact on HEALTH. On-balance sheet financing exerts a short-term suppressive impact on the ENERGY. This finding is in line with Uddin et al. [61], who suggest that the banking sector hinders energy consumption, consequently impeding industry growth. This study emphasizes the changing impact of different industries on the proportion of direct financing since 2015, showing an increasing importance of direct financing within industries post the 'stock market crash'. Furthermore, the promotional effect of direct financing on most industries aligns with Tian's earlier findings [32]. It is noteworthy that the impact of financing structure on industry development fluctuates due to various external events, highlighting the susceptibility of industry sectors to external factors.
5.4. Robustness analysis
To ensure the reliability of our research findings, we conducted a robustness test by replacing the original data on financing structure and re-establishing the time-varying spillover index model and large TVP-VAR model. We replaced the financing structure proportion data with specific components' original values from the social financing scale for a robustness analysis. As the original values were found to be non-stationary, they were first differenced. The results post-replacement displayed similarities to the conclusions drawn from the initial analysis, confirming the robustness of the model setting. Due to space constraints, only plotting the TOTAL spillover index (Figure 11) and three-dimensional time-varying impulse response diagram of DF for the entire time period (Figure 12) is presented here for clarification.
Figure 11 plots obtained from the robustness tests indicate that the trends are consistent with the Figure 3. They all demonstrate more pronounced spillover fluctuations at the time points examined, with no significant differences observed.
Based on the three-dimensional impulse response diagram (Figure 12) created using data on financing scale from various financing methods, it was observed that the results were consistent with previous findings. The impact of direct financing scale on various industry sectors became increasingly evident around 2015, with noticeable time-varying characteristics. However, the response intensity and direction varied among different industries. This variation could be attributed to the different sensitivities of industry sectors to financing scale data, with the possibility of some information being overlooked during the data processing phase. Additionally, both direct financing scale, on-balance sheet financing scale, and off-balance sheet financing scale exhibited strong time-varying characteristics.
6.
Conclusions
We utilize the time-varying spillover index model and the large TVP-VAR model to analyze various financing methods from a new perspective, employing high-dimensional and time-varying techniques with monthly data spanning from January 2002 to March 2023. We aim to employ for empirical analysis to systematically investigate the transmission mechanisms of different financing methods to various industry sectors in China. This approach enables a more comprehensive and in-depth analysis of the impact mechanism of financing structure on different industries. We draw the following conclusions:First, the spillover effect within industries is higher than the spillover effect between industries, and each industry is most affected by its own lagging effects. The industries of ENERGY, CONS, HEALTH, FINANCIAL, REAL ESTATE, INFO TECHN, TELECOM, PUBLIC, and DF are net recipients of risk, while MATERIAL, INDUSTRY, OPT CONS, OBS, and OBSF are net spillovers of external risk. Moreover, the impact of different financing methods on industries varies. On-balance sheet financing is most susceptible to risk spillovers from other industries, while both on-balance-sheet and off-balance-sheet financing pose higher risks to other industries. Direct financing, on the other hand, has the least impact on industry development within the financing structure. Additionally, according to the impulse response analysis, there is mutual influence between different financing methods, but there are variations. Third, the response of different industries on the proportion of direct financing has significantly changed since 2015. This indicates that during the 'stock market crash', the influence of direct financing on various industries started to become apparent, with most of them experiencing positive effects. Finally, the impact of different financing methods on the economic development of various industrial sectors is influenced by external events, and the extent of this impact varies.
6.1. Policy implications
This analysis underscores the importance of policymakers focusing on the effects of changes in financing methods on industry development. It also highlights the need for corporate decision-makers to consider the development of different industry sectors and the financing methods chosen by each sector, as well as their impact on industry development to mitigate potential risks. Additionally, it emphasizes the necessity for our country to establish a financing structure that aligns with the financial service demands of the real economy. Only when the financing structure meets the needs of the real economy's development can it effectively support and drive real economic growth. Policymakers should also harness the positive impacts of various financing methods on industry development and foster a social system that supports China's economic progress. In addition, in line with the financial development path of finance serving the real economy, it is imperative to actively promote direct financing, enhance the multi-level capital market system, and cater to diverse financing needs. Emphasizing the importance of direct financing across society can help mitigate risks associated with excessive indirect financing through banks and reduce corporate financing expenses. Wang et al. [65] emphasized that a robust stock market and increased financing options can boost corporate innovation and foster economic growth. Therefore, expediting the establishment of a multi-level stock market financing system and leveraging equity financing advantages is crucial. Furthermore, fostering the bond market, expanding bond financing, and aligning with the needs of the real economy are essential. In times of external events, policymakers should carefully assess their impact on industries, provide tailored financing options, regulate accordingly, and facilitate positive industry growth.
6.2. Limitations and future recommendations
The current research has certain limitations. For future studies, it is recommended to explore industry data at the company level to better capture financing method differences. Following Ding et al. [66], analyzing specific commodity prices within an industry can provide more focused insights. Additionally, considering the method used by Dogan et al. [50] to study the net directional pairwise spillovers between financing structures and industries in different time periods can enhance understanding. Exploring causal relationships using tests like nonlinear Granger causality or multivariate non-linearity, as suggested by Ye et al. [67], could be beneficial. Furthermore, investigating the impact of financing method structures on industry development, such as the role of the bond market in direct financing highlighted by Jin et al. [40], can offer valuable insights for different industries.
Use of AI tools declaration
The authors declare that they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
This work was supported by the National Natural Science Foundation of China (71963008) and the Joint Cultivation Project of Guangxi Natural Science Foundation (No.2018GXNSFAA294131).
Conflict of interest
The authors declare no conflicts of interest.









 DownLoad:
DownLoad:













