1.
Introduction
Separable algebras in tensor categories are a natural generalization of finite-dimensional (associative unital) semisimple algebras over C. Let C be a tensor category, see e.g., [19,51]. If C happens to be in addition unitary i.e., C∗, see e.g., [6,52], the main result of this note, Theorem 4.13, states that every separable algebra is "unitarizable" i.e., it is isomorphic to a "unitarily" separable algebra, and the converse holds trivially. For the precise notions, see Definitions 3.3, 4.1 and 4.2. By Theorem 4.13, every statement involving separable algebras living in a tensor or multitensor C*-category has a "unitary" counterpart.
On the one hand, unitarily separable algebras also appear in the literature under the name of special C∗-Frobenius algebras [6] or Q-systems [45,46,48]. Their study was initially motivated by the applications to operator algebras, in particular to the construction and classification of finite-index subfactors [37,38,55,57,58]. See [20] for an introduction to the subject, [31] for an overview, and [4], and references therein, for recent classification results. Since [47], Q-systems also play a pivotal role in the construction and classification of finite-index extensions of algebraic quantum field theories [33] in arbitrary spacetime dimensions, and of one-dimensional conformal field theories in the (completely) unitary vertex operator algebra framework [12,39] as well, since [30]. Recently, Q-systems have been employed in the study of "quantum symmetries" (tensor category actions, generalizing ordinary group symmetries) of C∗-algebras [10,11,14,21].
On the other hand, separable algebras have a priori no inbuilt unitarity. Together with an additional commutativity assumption with respect to a given braiding, since [15], they are also often called étale algebras. These objects, typically assuming connectedness, are studied in relation to Ocneanu's quantum subgroups [54]. See [26] for recent results and a detailed account on their classification program. As for (commutative irreducible) Q-systems in the algebraic quantum field theory framework, connected étale algebras can be used to describe (local irreducible) extensions of vertex operator algebras [35], see also [13,40]. Notably, they describe all rational 2D conformal field theories maximally extending a given tensor product of (isomorphic) chiral subtheories. See [22,23,24,25,60] in the Euclidean setting, [34,41] in the full vertex operator algebra setting, [5,7] for the algebraic quantum field theory setting, and [3] for the Wightman quantum field theory setting. See also [42] for a proof of functoriality of the [22] construction when varying the given chiral subtheory.
The proof of our main result, Theorem 4.13, strongly relies on Theorem 3.2 in [8]. In the connected (i.e., haploid) case, the notions of separable algebra, Frobenius algebra, and isomorphic to unitarily separable algebra (i.e., isomorphic to special C∗-Frobenius algebra = Q-system) all coincide by Lemma 4.10 below and by Theorem 3.2, see also Remark 3.3, in [8]. In the non-connected case, we first decompose a separable algebra A in C into indecomposable ones, Lemma 4.8, then unitarize the category of right A-modules in C, Lemma 4.11. Last, we show that the unitarized category is equivalent to the modules over a unitarily separable algebra in C to which A is isomorphic, Proposition 4.12. This leads to Theorem 4.13.
We point out that the semisimplicity of C (or of the tensor or multitensor subcategory generated by A) is implicitly used in Theorem 3.2 in [8]. Here, we need it to exploit the separability of A via Proposition 4.3. Thus, a possible generalization of Theorem 4.13 to the case of non-semisimple monoidal C∗-categories C should require a different idea, possibly "internal" to the C∗-algebra C(A,A), on how to show directly that a separable algebra is isomorphic in C to a unitarily separable one.
2.
Preliminaries
A C∗-category is a generalization of a C∗-algebra of operators acting between different Hilbert spaces instead of one. The objects X,Y,Z,… of C can be thought of as the Hilbert spaces, the morphisms f,g,h,… of C as the bounded linear operators. Formally, it is a C-linear category C ([19,49]) equipped with an involutive contravariant anti-linear endofunctor ∗:C→C (sometimes called dagger or adjoint) and a family of norms ‖⋅‖ on morphisms such that
● the endofunctor ∗ is the identity on objects (we use f∗∈C(Y,X) to denote the image of the morphism f∈C(X,Y)),
● the hom space C(X,Y) is a Banach space for every X,Y∈C,
● ‖gf‖≤‖g‖‖f‖, ‖f∗f‖=‖f‖2, f∗f≥0, for every f∈C(X,Y), g∈C(Y,Z).
In particular, a C∗-category with one object is a unital C∗-algebra (see [28]).
In the following, we use 1X to denote the identity morphism in C(X,X). For a morphism f∈C(X,Y) we will occasionally write f:X→Y if the environment category C is clear from the context.
A morphism f in a C∗-category is called unitary (resp. self-adjoint) if f∗=f−1 (resp. f∗=f). Let C and D be two C∗-categories. A ∗-functor from C to D is a linear functor such that F(f∗)=F(f)∗ for every morphism f.
A multitensor C∗-category is an abelian rigid ([17,48]) monoidal category (C,⊗:C×C→C,1) equipped a C∗-category structure satisfying the following conditions:
● the tensor unit 1 of C is semisimple, i.e., C(1,1) is finite-dimensional,
● ⊗ is a bilinear functor and (f⊗g)∗=f∗⊗g∗ for every morphisms f,g,
● the associator and the left/right unitor constraints are unitary.
If C(1,1)≃C, i.e., if 1 is simple, then C is called a tensor C∗-category. By Proposition 8.16 in [27], every multitensor C∗-category C is semisimple and locally finite. Moreover, by Mac Lane's coherence theorem, C is equivalent to a strict multitensor C∗-category, i.e., where the associator and the left/right unitors are identities (see [6,19]). From now on, unless otherwise specified, we use C to denote a (strict) multitensor C∗-category.
Remark 2.1. The tensor unit 1 of C is a direct sum of simple objects ⊕ni=11i. Note that C≃⊕ijCij, where Cij:=1i⊗C⊗1j (see Remark 4.3.4 in [19]). Let τ be the linear functional on C(1,1) defined by
Let X∈C. We have X≃⊕ijXij and ¯X≃⊕ij¯Xji, where Xij:=1i⊗X⊗1j and ¯X, ¯Xij denote the dual (or conjugate) objects of X, Xij respectively. Namely, for every i,j∈{1,…,n}, there exists (see below) a solution (γij∈C(1j,¯Xij⊗Xij),¯γij∈C(1i,Xij⊗¯Xij)) of the conjugate equations
which is unique up to unitaries, and such that
for every f∈C(Xij,Xij). The scalar dimension of Xij ([27,48]) is then dXij=τ(γ∗ijγij)=τ(¯γ∗ij¯γij).
For the convenience of the reader, we sketch proof of this well-known fact when i≠j (the case where i=j can be proved similarly). Let {Zs}s be a set of representatives of simple objects in Cij. Since dimC(1j,¯Zs⊗Zs)=dimC(1i,Zs⊗¯Zs)=1, we can choose a solution of the conjugate equations (γs,¯γs) such that τ(γ∗sγs)=τ(¯γ∗s¯γs), i.e., ‖γs‖=‖¯γs‖ (as in Definition 3.4 in [48]). For non-simple Xij∈Cij, let {us,k}k (resp. {¯us,k}k) be a basis of C(Zs,Xij) (resp. C(¯Zs,¯Xij)) such that u∗s,lus,k=δk,l1Zs (resp. ¯u∗s,l¯us,k=δk,l1¯Zs). Let
as before Lemma 3.7 in [48], or before Lemma 8.23 in [27], then (γij,¯γij) is a solution of the conjugate equations that satisfies the Eq (2.1). Indeed,
Let (ω∈C(1,¯Xij⊗Xij),¯ω∈C(1,Xij⊗¯Xij)) be a solution of the conjugate equations that satisfies the Eq (2.1). Then, there exists an invertible morphism h∈C(Xij,Xij) such that ω=(1¯Xij⊗h)γij and ¯ω=((h∗)−1⊗1¯Xij)¯γij. By choosing a different basis of C(Zs,Xij), we may assume that h=∑s∑kas,kus,ku∗s,k, where as,k>0. Then, the condition that (ω,¯ω) fulfills the Eq (2.1) implies that h=1Xij. In other words, the solution of the conjugate equations that satisfies the Eq (2.1) is unique up to unitaries (see Lemmas 3.3 and 3.7 in [48], and cf. Lemma 8.35 in [27], for more details).
Let γX:=⊕ijγij and ¯γX:=⊕ij¯γij. Note that these are not the standard solutions of the conjugate equations defined in [27], where the Perron-Frobenius data of the matrix dimension enter as numerical prefactors for each i,j (see Definitions 8.25 and 8.29 therein), unless the tensor unit is simple (as in Section 3 of [48]) and they coincide with the standard solutions of [48]. In particular, the "loop" or "bubble" morphisms γ∗XγX and ¯γ∗X¯γX will neither be scalar in C(1,1), nor equal, nor will (γX,¯γX) be spherical (resp. minimal) in the sense of Theorem 8.39 (resp. Theorem 8.44) in [27].
With the (γX,¯γX) defined above, we have
and
for every g∈C(X,Y), h∈C(Y,X), and X,Y∈C. Moreover, if a solution of the conjugate equations (ω∈C(1,¯X⊗X),¯ω∈C(1,X⊗¯X)) fulfills
then there exists a unitary u∈C(X,X) (or ¯u∈C(¯X,¯X)) such that ω=(1¯X⊗u)γX and ¯ω=(u⊗1¯X)¯γX (or ω=(¯u⊗1X)γX and ¯ω=(1X⊗¯u)¯γX).
Based on these observations, it is not hard to check that C endowed with the pivotal duality {(¯X,γX,¯γX)}X∈C is a pivotal category (see, e.g., Section 1.7 in [61] for the definition of pivotal category).
3.
Algebras and modules in multitensor C∗-categories
We recall below the natural generalization of the notion of finite-dimensional unital associative algebra (in the tensor category of finite-dimensional complex vector spaces Vecf.d.,C). Let C be a strict multitensor C∗-category.
Definition 3.1. An algebra in C is a triple (A,m,ι), where A is an object in C, m∈C(A⊗A,A) is the "multiplication" morphism, ι∈C(1,A) is the "unit" morphism, fulfilling the associativity and unit laws
Definition 3.2. Two algebras (A,m,ι) and (A′,m′,ι′) in C are said to be isomorphic if there is an invertible (not necessarily unitary) morphism t∈C(A,A′) such that tm=m′(t⊗t) and tι=ι′.
Definition 3.3. An algebra (A,m,ι) in C is called a C∗-Frobenius algebra if m∗ is a left (or equivalently right) A-module morphism such that
An algebra (A,m,ι) in C is called special if the multiplication is a coisometry*
*or, in a different convention, a scalar multiple of a coisometry, cf. [2,6,29,50,53]. Also, note that we do neither ask ι∗ι to be 11, nor a multiple of 11, and that the latter condition is automatic if the tensor unit 1 is simple.
Definition 3.4. Forgetting the C∗ structure, an algebra (A,m,ι) in C endowed with a coalgebra structure (A,Δ∈C(A,A⊗A),ε∈C(A,1)) (not necessarily Δ=m∗, ε=ι∗) fulfilling the coassociativity and counit laws, is called a Frobenius algebra if the analogue of (3.1) holds with m∗ replaced by Δ (see [1,22,62]).
The following crucial results proven in [6,22,48] assuming C(1,1)≃C, see in particular Chapter 3 in [6], also hold for multitensor C∗-categories, cf. Section 2.2 in [32].
Proposition 3.5. Let (A,m,ι) be an algebra in C.
● If (A,m,ι) is special, then it is a C∗-Frobenius algebra.
● If (A,m,ι) is a C∗-Frobenius algebra, then it is isomorphic to a special one.
Example 3.6. Recall, e.g., from Section 2 in [1] and Section 2.1 in [53], that a C∗-Frobenius algebra in Hilbf.d.,C, the tensor C∗-category of finite-dimensional Hilbert spaces, is just an ordinary finite-dimensional C∗-algebra with a Frobenius structure. Forgetting the C∗ structure, a Frobenius algebra in the tensor category Vecf.d.,C of finite-dimensional vector spaces is a finite-dimensional Frobenius algebra.
We shall use module categories (and their unitary version, C∗-module categories recalled below) over multitensor C∗-categories. See [56] or Chapter 7 in [19] for the definitions of module category over a monoidal category C and module functor.
Definition 3.7. A left C∗-module category over a multitensor C∗-category C is a left C-module category (M,⊙:C×M→M) which is also a C∗-category, such that
● ⊙ is bilinear and (f⊙g)∗=f∗⊙g∗ for every morphisms f∈C, g∈M,
● the associator and the unitor constraints are unitary.
Right C∗-module categories and C∗-bimodule categories are defined similarly.
Typical examples of left (resp. right) C-module categories (not necessarily C∗) come from considering right (resp. left) modules over an algebra (A,m,ι) in C. We use RModC(A) (resp. LModC(A)) to denote the category of right (resp. left) A-modules in C.
Definition 3.8. Let (A,m,ι) be a special C∗-Frobenius algebra in C. As for algebras, a right A-module (X,r∈C(X⊗A,X)) in C is called special if
We denote by sRModC(A) the category of special right A-modules in C. The definition for left A-modules is analogous.
By the arguments of Chapter 3 in [6], cf. Section 2.2 in [32], we have
Proposition 3.9. Let (A,m,ι) be a special C∗-Frobenius algebra in C. Then sRModC(A) is a left C∗-module category over C, where the involution and norms are inherited from C.
More generally, given a right A-module (X,r∈C(X⊗A,X)), then (X,r′:=h−1r(h⊗1A)) is a special right A-module, where h:=√rr∗, and h−1 is a right A-module isomorphism from (X,r) to (X,r′). Moreover, RModC(A) is a left C∗-module category over C with the following C∗-structure
● f∈RModC(A)(X,Y)↦h2Xf∗h−2Y∈RModC(A)(Y,X),
● |||f|||:=‖h−1YfhX‖, f∈RModC(A)(X,Y),
where hX:=√rXr∗X and hY:=√rYr∗Y are defined respectively from the right A-module actions of X and Y. The embedding sRModC(A)↪RModC(A) is an equivalence of left C∗-module categories.
4.
Separable algebras are unitarizable
In this section, we prove our main theorem.
Definition 4.1. An algebra (A,m,ι) in C is called separable if the multiplication m∈C(A⊗A,A) splits as a morphism of A-A-bimodules in C, i.e., if there is an A-A-bimodule morphism f∈C(A,A⊗A) such that mf=1A.
Clearly, every (not necessarily special) C∗-Frobenius algebra in C is separable. Indeed, by Proposition 3.5, it is isomorphic to a special algebra in C (Definition 3.3), namely mm∗=1A holds up to isomorphism of algebras, hence it is separable.
Moreover, a special C∗-Frobenius algebra, which is also called a Q-system after [46] (see also [6,8,11,48,50] and references therein), can be viewed as a "unitarily" separable algebra. The following definition is motivated by this fact.
Definition 4.2. A (Frobenius) algebra in C is unitarizable if it is (not necessarily unitarily) isomorphic to a special C∗-Frobenius algebra in C.
Our main result (Theorem 4.13) states that every separable algebra in C is unitarizable.
By the proof of Proposition 7.8.30 in [19], cf. Section 3 in [56], Section 2.3 in [15], Section 2.4 in [36], Section 4 in [43], the following characterization of separability for algebras in (not necessarily C∗) multitensor categories holds.
Proposition 4.3. Let (A,mA,ιA), (B,mB,ιB) be separable algebras in C. Then the categories RModC(A), LModC(A), and BiModC(A|B) (A-B-bimodules in C) are semisimple.
In particular, an algebra (C,mC,ιC) in C is separable if and only if BiModC(C|C) is semisimple.
Let (A,m,ι) be an algebra in C, (X,r)∈RModC(A), and (Y,l)∈LModC(A). We recall, e.g. from Section 7.8 in [19] tensor product of X and Y over A is the object X⊗AY∈C defined as the co-equalizer of the diagram
The following result follows from Proposition 7.11.1 in [19].
Proposition 4.4. Let (A,mA,ιA), (B,mB,ιB) be algebras in C such that RModC(A), RModC(B) are semisimple. Then, the category FunC|(RModC(A),RModC(B)) of left C-module functors is equivalent to BiModC(A|B).
The equivalence is given by
Definition 4.5. A separable algebra (A,mA,ιA) in C is called indecomposable if RModC(A) is an indecomposable left C-module category, i.e., if it is not equivalent to a direct sum of non-zero left C-module categories.
Definition 4.6. An algebra (A,mA,ιA) is called connected (or haploid) if dim(C(1,A))=1, i.e., if A is a simple object in RModC(A).
Lemma 4.7. Let C≃⊕ijCij be the decomposition as in Remark 2.1. Then (A,mA,ιA) is a connected algebra in C if and only if there exists exactly one j∈{1,…,n} such that A=Ajj is a connected algebra contained in the tensor C∗-category Cjj with tensor unit 1j.
Proof. Recall 1=⊕ni=11i. By connectedness, there is only one j such that C(1j,A)≠0, and dim(C(1j,A))=1. Moreover, every Akl must be zero unless k=l=j. □
The following result is well-known, we sketch the proof for the reader's convenience.
Lemma 4.8. Let (A,m,ι) be a separable algebra in C. Then A is a direct sum of indecomposable separable algebras.
Proof. Note that RModC(A) is indecomposable if and only if the identity functor id=−⊗AA associated with the trivial bimodule A is a simple object in FunC|(RModC(A),RModC(A)). By Proposition 4.4,
Assume that dim(BiModC(A|A)(A,A))>1. Recall from Proposition 4.3 that BiModC(A|A) is semisimple. Let p be a non-trivial idempotent in BiModC(A|A)(A,A), i.e., 1A−p≠0, p2=p, and let B be the image of p. Then B is a separable algebra with multiplication and unit given by vm(w⊗w) and vι, where v:A→B and w:B→A are A-A-bimodule morphisms such that vw=1B and wv=p. Note that f:B→B is a B-B-bimodule morphism with the previous algebra structure on B if and only if wfv:A→A is an A-A-bimodule morphism. Thus dim(BiModC(B|B)(B,B))<dim(BiModC(A|A)(A,A)). This implies that A is a direct sum of indecomposable separable algebras. □
Remark 4.9. If, in addition, the category C is braided and the separable algebra (A,m,ι) is commutative in the sense of Definition 1.1 in [40], cf. Definition 4.20 in [6], then BiModC(A|A) and RModC(A) can be identified. Hence, by the previous proof, A is a direct sum of connected separable algebras, cf. Remark 3.2 in [15].
Lemma 4.10. Let (A,m,ι) be a connected separable algebra in C. Then A can be promoted to a Frobenius algebra.
Proof. By Lemma 4.7, we may assume that C is a tensor C∗-category. Recall the conventions in Remark 2.1. ¯A is a right A-module with right A-action given by
Let f:A→¯A be the non-zero right A-module morphism defined by
Since RModC(A) is semisimple by Proposition 4.3, A is a simple right A-module by connectedness, and dA=d¯A (where dA is the scalar dimension [27] of A in C, or equivalently the dimension [48] in Cjj, cf. Lemma 4.7), f is invertible in C. Hence, by Lemma 3.7 in [22], A can be promoted to a Frobenius algebra. □
Let (M,⊙) be a left C-module category. Then M is said to be enriched in C if the functor C↦M(C⊙X,Y):C→Vecf.d.,C is representable for every X,Y∈M, i.e., there exists an object [X,Y]∈C such that
The object [X,Y] is called the internal hom from X to Y. In particular, [X,−]:M→C is the right adjoint of the functor −⊙X:C→M.
If M=RModC(A), where A is a separable algebra in C, then M is enriched in C. More explicitly, the internal hom [X,Y] is given by ¯X⊗A¯Y. We refer the reader to Section 7 in [19] or Section 2 in [44] for basic facts about internal homs.
Lemma 4.11. Let (A,mA,ιA) be an indecomposable separable algebra in C. Then there exists a connected special C∗-Frobenius algebra (B,mB,ιB) in C such that RModC(A) and RModC(B) are equivalent as left C-module categories.
In particular, RModC(A) is equivalent to a left C∗-module category over C.
Proof. Let X be a non-zero simple object in RModC(A). By Proposition 4.3 and by the proof of Theorem 3.1 in [56] (cf. Theorem 2.1.7 in [44]), the internal hom [X,X] in RModC(A) is a connected (by the simplicity of X) algebra in C such that RModC(A) and RModC([X,X]) are equivalent. Note that RModC(A) and RModC([X,X]) are both semisimple. Since
from Propositions 4.3 and 4.4 it follows that A separable implies that [X,X] is separable. By Lemma 4.10, [X,X] can be promoted to a connected Frobenius algebra. Then, [X,X] is isomorphic to a special C∗-Frobenius algebra B in C by Lemma 4.7 and by Theorem 3.2, cf. Remark 3.3, in [8]. We conclude that RModC(A) is equivalent to RModC(B). The latter is a left C∗-module category over C by Proposition 3.9. □
The following result is of independent interest and it should be compared with Lemma 2.18 in [29] for M=RModC(A), and Theorem A.1 in [53].
Proposition 4.12. Let (M,⊙) be an indecomposable left C∗-module over C which is enriched in C. For every non-zero object X in M, the internal hom [X,X] is isomorphic (up to rescaling) to a special C∗-Frobenius algebra in C.
Proof. By Proposition 2.3 in [59], we may choose the right adjoint [X,−]:M→C of the ∗-functor −⊙X:C→M to be a ∗-functor. For every C∈C and Y∈M, we treat C(C,[X,Y]) as the Hilbert space with inner product given by
where γC and τ are defined in Remark 2.1. Fix a faithful tracial state Tr on M(X,X). We treat M(C⊙X,Y) as the Hilbert space with inner product defined by
By the enrichment assumption, C(−,[X,−]) and M(−⊙X,−) are equivalent bilinear ∗-functors Cop×M→Hilbf.d.,C, i.e., C(f,[1X,g])∗=C(f∗,[1X,g∗]) and M(f⊙1X,g)∗=M(f∗⊙1X,g∗) for every f∈C(C2,C1) and g∈M(Y1,Y2). By considering the polar decomposition of natural isomorphisms, we may assume that the natural isomorphism C(−,[X,−])≃M(−⊙X,−) is componentwise unitary, i.e., C(C,[X,Y])≃M(C⊙X,Y) is unitary for every C∈C and Y∈M.
Note that [X,−] is a left C-module functor with the C-module structure αC,Y:C⊗[X,Y]∼→[X,C⊙Y] defined by the following natural isomorphism
where the first and fourth morphisms are induced by the solution of conjugate equation (γC,¯γC) and the third morphism is induced by the module structure of M (see Section 7.12 in [19]). By the fact that the natural isomorphism C(−,[X,−])≃M(−⊙X,−) is componentwise unitary, it is not hard to check the the natural isomorphism (4.1) is unitary. Thus, αC,Y is unitary.
The evaluation evY:[X,Y]⊙X→Y is obtained as the image of 1[X,Y] under the natural isomorphism C([X,Y],[X,Y])≃M([X,Y]⊙X,Y). Let evY=hYuY be the polar decomposition of evY, where hY:=√evYev∗Y. Since αC,Y is the unique morphism such that the following diagram commutes
by the uniqueness of the polar decomposition, we have 1C⊙hY=hC⊙Y. In particular, hY:Y→Y is a left C-module natural isomorphism of the identity functor IdM to itself. Since M is indecomposable, there exist λ>0 such that hY=λ1Y for every Y. Since the multiplication of m:[X,X]⊗[X,X]→[X,X] is defined by
(see Section 3.2 in [56]) we have mm∗=λ21[X,X]. Hence [X,X] can be rescaled to a special C∗-Frobenius algebra. □
Summing up, we can state and prove our main result.
Theorem 4.13. An algebra in a multitensor C∗-category C is isomorphic to a special C∗-Frobenius algebra if and only if it is separable.
Proof. By Lemma 4.8, we only need to show that every indecomposable separable algebra (A,mA,ιA) in C is isomorphic to a special C∗-Frobenius algebra. Recall that RModC(A) is equivalent to a left C∗-module category over C, denoted by M, by Lemma 4.11. Let F:RModC(A)→M be the equivalence of left C-module categories. The algebra A seen as an object of RModC(A) equals [A,A], see e.g., Remark 3.5 in [56], hence it is isomorphic to [F(A),F(A)]. The latter is isomorphic to a special C∗-Frobenius algebra by Proposition 4.12, hence A is, and the proof is complete. □
For fusion C∗-categories C, the following is stated as Corollary 3.8 in [8], as a consequence of Theorem 3.2 therein.
Corollary 4.14. Let M be a finite semisimple left module category over a multi-fusion C∗-category C. Then M is equivalent to RModC(A) for a special C∗-Frobenius algebra A.
Therefore, every finite semisimple left module category M over a multi-fusion C∗-category C admits a unique unitary structure (up to unitary module equivalence).
Proof. By Corollary 7.10.5 in [19], M is equivalent to RModC(B), where B is an algebra in C. Since M is semisimple, BiModC(B|B)≃FunC|(RModC(B),RModC(B)) is semisimple by Theorem 2.18 in [18]. Then B is separable by Proposition 4.3, and RModC(B) is equivalent to RModC(A) for a special C∗-Frobenius algebra A by Theorem 4.13. The uniqueness statement follows from Corollary 9 in [59], see also Theorem 1 and Remark 4 therein. □
We conclude with an application of Theorem 4.13 which justifies Remark 4.2 in [32]. The idempotent completion of a locally idempotent complete bicategory B, introduced in Definition A.5.1 in [16], is the bicategory whose objects are separable algebras in B, whose 1-morphisms are bimodules, and whose 2-morphisms are bimodule maps. By Proposition A.5.4 in [16], there exists a canonical fully faithful bifunctor from B into its idempotent completion. B is called idempotent complete if this bifunctor is a biequivalence. By combining the straightforward generalization of Theorem 4.13 to algebras in (rigid) semisimple C∗-bicategories and Lemma 4.1 in [32], we have the following result.
Corollary 4.15. The rigid C∗-bicategory of finite direct sums of II1 factors, finite Connes' bimodules and intertwiners is idempotent complete.
This result is also stated with a different but equivalent terminology in [11]. By Theorem 4.13, at least for (rigid) semisimple C∗-bicategories, the terminology of Q-system completion used in Definition 3.34 in [11] coincides with the previously mentioned idempotent completion of [16].
Use of AI tools declaration
The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.
Acknowledgments
We thank Zheng Hao for insightful comments and Dave Penneys for informing us about a proof of Theorem 4.13 in the multifusion case that is to appear in [9]. We also thank the referees for their suggestions and comments.
Research by the first author (L.G.) is supported by "MIUR Excellence Department Project MatMod@TOV" awarded to the Department of Mathematics, University of Rome Tor Vergata, CUP E83C23000330006, and by the University of Rome Tor Vergata funding OAQM, CUP E83C22001800005, by progetto GNAMPA 2023 "Metodi di Algebre di Operatori in Probabilità non Commutativa" CUP E53C22001930001, and by progetto GNAMPA 2024 "Probabilità Quantistica e Applicazioni" CUP E53C23001670001. Research by the second author (W.Y.) is supported by the NSFC under grant numbers 11971463, 11871303, 11871127.
Conflict of interest
All authors declare no conflicts of interest in this paper.









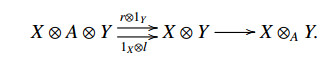
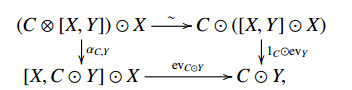




 DownLoad:
DownLoad: