1.
Introduction
Polysaccharide hydrogels have been widely investigated for their use as colon-targeted delivery systems. Notably, the use of AX gels represents an exciting alternative for such application as they exhibit biocompatibility with human colon cells [1] and can be degraded by colonic microbiota [2]–[4]. A recent study documented the potential use of AX microspheres as insulin carriers targeted to the colon, showing a hypoglycemic effect in diabetic-induced rats [5]. Moreover, the administration of AX gels to obese-induced rats showed a protective effect on microbiota by promoting the increase of beneficial bacteria such as Bifidobacteria [6]. AX are the main non-starch polysaccharides from cereal grains with a basic structure consisting of a linear β(1-4)-linked D-xylopyranosyl backbone chain with some α-L-arabinofuranose units attached to O-2 and O-3 position of xylose units [7]. Besides, some ferulic acid (FA) residues can be esterified to the arabinose side-chains through O-5 [8]. AX form three-dimensional networks through the oxidative coupling of FA residues by free radical generating agents such as laccase or peroxidase/H2O2 [9]. The resulting dimers (di-FA) and trimer (tri-FA) covalent cross-linking structures finally lead to the formation of gels [10],[11]. These gels exhibit stability to pH and electrolyte and can absorb large amounts of water [7]. Several studies have demonstrated the ability of AX gels as controlled-release systems of proteins, molecules, and cells [4],[12]–[15]. AX reach the colonic region and are fermented by gut microbiota resulting in the production of short-chain fatty acids (SCFA) as end products [16]. SCFA are well-known to exert positive physiological effects on host health. Particularly butyric acid plays a role in the prevention of colon cancer as it is the primary substrate for colonocytes and promotes the health of the gut barrier [17]. Previous studies have documented the antiproliferative effect of SCFA, butyrate, and acetate on colorectal cancer cells through different mechanisms such as gene expression inhibition and via apoptosis [18],[19]. Moreover, Glei et al. [20] reported that the fermentation supernatants of water-soluble and alkali extracted AX fermented by human feces exhibited an antiproliferative effect on the human colon cancer cells HT29. The protective effect of phenolic acids, to which FA belongs, on colon carcinogenesis has been also reported by several authors [21]–[24]. The FA has demonstrated to inhibit the proliferation of the human colon cancer cell line Caco-2 by affecting the cell cycle, specifically inducing a delay in the S phase and dysregulating genes implied in cell cycle control [22],[23]. AX are mainly degraded by Bacteroides and Bifidobacterium as they produce the enzymes required for its degradation [2],[25]. A cross-synergy effect between Bifidobacterium longum and Bifidobacterium adolescentis in arabinoxylo-oligosaccharides (AXOS) degradation has been suggested [26]. Previous studies have documented that AX fermentation promotes the growth of probiotics such as Lactobacillus and Bifidobacterium [25],[26], while certain strains such as Bacteroides are not favored in the presence of cross-linked AX [25]. The administration of gelled AX to obesity-induced rats fed with high fat diets had a protective effect on the stability of the taxonomic profile of microbiota by increasing Bifidobacterium population, while favoring an adequate balance of Bacteroidetes/Firmicutes [6]. Thus, AX fermentation could evidently exert positive effects on the host health by producing beneficial metabolites (SCFA, FA) and modulating the microbiota composition by increasing probiotic bacteria population. It has been reported that the presence of FA esterified to AX inhibits its degradation and subsequent fermentation [27]. Interestingly, cross-linking of AX reduces the rate of fermentation because the polymeric network structure restricts xylanolytic activity, and therefore arabinose moieties are preferentially used [2]. Previous work indicated that fermentation of AX gels by Bifidobacteria decreases as the cross-linking density increases due to the limited accessibility of bacterial enzymes to their target sites. It is also suggested that a more compact microstructure of the gel and a high content of covalent cross-linking structures (di-FA and tri-FA) may slow down bacterial enzymes penetration to their hydrolysis sites causing slow removing of arabinoses moieties [3]. AX gels produced in the form of particles or microspheres are considered excellent materials for biomedical applications. AX microspheres and AX particles can be fabricated by electrospraying method [4],[12],[28]. Electrospraying is an attractive technique widely used for the fabrication of particles based on synthetic and natural polymers that permits to control the size dispersion and to avoid the formation of agammaegations [29]. AX-based particles with sizes ranging from nanometers to millimeters have been successfully prepared using this technique and demonstrated promising application as encapsulating agents [4],[12],[28]. Previous studies have evaluated the degradation of AX gels by a mixture of Bifidobacteria strains (Bifidobacterium longum and Bifidobacterium adolescentis) [3] and also by a combination of Bacteroides ovatus and Bifidobacterium longum [30]. However, the impact of highly cross-linked AX gels on fermentation by a mixture of the three strains has not been investigated so far. Moreover, to date, there are not reports about the effect of the fermentation supernatants of highly cross-linked AX particles (AXP) on the proliferation of human colon cancer cells. Therefore, this study aimed first to investigate the in vitro fermentation of AXP by a representative colonic bacterial mixture of three strains (Bifidobacterium longum, Bifidobacterium adolescentis, and Bacteroides ovatus) and secondly, to evaluate for the first time the effect of AXP fermentation supernatants on the proliferation of the human colon cell line Caco-2.
2.
Materials and methods
2.1. Materials
AX were extracted from a maize by-product (distiller's dried grains with solubles) and characterized as reported elsewhere [31]. AX presented an arabinose to xylose (A/X) ratio of 1.1, a molecular weight of 209 kDa and a ferulic acid (FA) content of 7.53 µg/mg polysaccharide. Laccase (benzenediol: oxygen oxidoreductase, E.C.1.10.3.2) from Trametes versicolor, and all other chemical products were purchased from Sigma Chemical Co. (St. Louis, MO, USA).
2.2. Methods
2.2.1. AXP preparation and morphology
AXP were prepared using the electrospraying method, as described by Mendez-Encinas et al. [1]. A coaxial electrospray system (The Spraybase® electrospray device, Profector Life Sciences, Kildare, Ireland) with a stainless-steel coaxial nozzle (Spraybase®) was used. AX solution (2% w/v) in 0.05 M citrate-phosphate buffer at pH 5.5 and laccase (6.7 nkat/µL) were employed. The flow rates used were 4.0 mL/h and 0.6 mL/h for AX (inner nozzle) and laccase (outer nozzle) solutions, respectively. The distance between the needle tip and the collector was ~15 cm and the voltage supplied was 11–13 kV. AXP were collected into mineral oil. The AXP were washed with ethanol:water (30:70, 50:50, 70:30 v/v) to remove mineral oil. The AXP form and size were analyzed by optical microscopy (inverted optical microscope Zeiss Axio Vert. A1, Carl Zeiss Microscopy, Jena, Germany) equipped with a digital camera (Axio Cam ERC 5s, Jena, Germany) using an image analysis software (Zen 2.3 lite). The AXP mean diameter was calculated by measuring the diameter of 120 AXP.
2.2.2. Bacteria inoculum
The bacterial strains used were Bifidobacterium longum (ATCC® 15708™), Bifidobacterium adolescentis (ATCC® 15703™), and Bacteroides ovatus (ATCC® 8483™), purchased from the American Type Culture Collection (Mannasas, USA). The bacteria were preserved in 10% glycerol stock solutions at −80 °C. Propagation of bacteria was performed according to Van Laere et al. [32] and Crittenden et al. [33] with some modifications. For bacteria propagation, Bifidobacteria were cultivated once in fresh de Man, Rogose and Sharp (MRS) medium and twice in Bifidobacterium Selective Medium (BSM), and Bacteroides ovatus was cultivated three times in Tryptic Soy Broth (TSB) by inoculating 1 mL of the bacterial solution into the next solution. Afterward, the bacteria were grown in basal media (culture media with no added carbon source), as described by Hughes et al. [25]. The bacteria were first inoculated (0.5 mL) into glass tubes with 5 mL of basal media containing 1% v/w arabinose/xylose (A/X, 1:1) as a sole carbon source and incubated under anaerobic conditions at 37 °C for 24 h, and then inoculated (0.5 mL) in 5 mL of 1% AX w/v basal media. The overnight full-grown cultures were used as an inoculum for AXP in vitro fermentation.
2.2.3. AXP fermentation
The AXP in vitro fermentation experiments were conducted as previously described by Martínez-López et al. [3] with some modifications. AXP were autoclaved at 121 °C for 15 min and then mixed with sterile basal media to obtain a final concentration of 5 g/L (w/v) AX as a sole carbon source. Oxyrase (Oxyrase Inc., Mansfield, OH, USA) was added to the media in order to remove the dissolved oxygen from the microenvironment. The media were inoculated with 4.5% v/v of a bacterial mixture (Bifidobacterium longum, Bifidobacterium adolescentis, and Bacteroides ovatus, ratio 1:1:1, 1 × 106 CFU/mL) and incubated under anaerobic conditions for 48 h at 37 °C. The fermentations were performed in duplicate, and culture media with no added carbon source was used as control. The bacterial growth was measured by monitoring the optical density (OD) of samples immediately after inoculation and after 18, 24, 42, and 48 h of fermentation. Aliquots of 200 µL of culture were placed in 96-well microplates, and the OD was measured at 600 nm using a microplate reader (Thermo Scientific MultiSkan Go, Madrid, Spain). Also, the pH of the culture medium was monitored during the experiment, and the strains were checked microscopically to exclude contamination in the culture medium before and after the fermentation. At the end of fermentation, the culture media was centrifuged at 16,000 × g for 15 min at 4 °C. Then, supernatant was sterilized by filtration (0.22 µm) to give AXP fermentation supernatants (AXP-fs), which were stored at −80 °C for further analysis.
2.2.4. Fourier-Transform Infrared (FT-IR) Spectroscopy
Infrared spectra of lyophilized AXP, AXP-fs and culture media were recorded on a Nicolet iS50 FT-IR spectrometer (Thermo Fisher Scientific Inc., Waltham, MA, USA). The samples were analyzed in absorbance mode from 400 and 4000 cm−1 range at 4 cm−1 resolution and 20 °C. Thirty-two scans were averaged and referenced against air.
2.2.5. Molecular weight distribution
AXP and AXP-fs molecular weight distributions were determined by size-exclusion high performance liquid chromatography (SE-HPLC) at 38 °C according to the protocol described by Martínez-Lopez et al. [3]. A TSK gel (Polymer Laboratories, Shropshire, United Kingdom) G5000 PWXL column (7.8 × 300 mm) was used. An isocratic elution was performed at 0.6 mL/min with 0.1 M LiNO3 (filtered 0.22 µm). 20 µL of the sample (0.1 % w/v in 0.1 M LiNO3 filtered through 0.22 µm pore size filter) were injected. Detection was done using a Waters 2414 refractive index detector, and pullulan standards (P50 to P800) calibration curve was used for the estimation of molecular weights. The elution profile of the culture media was also analyzed in order to identify and compare the observed peaks.
2.2.6. Short-chain fatty acids (SCFA) content
SCFA content was determined in AXP-fs and control (no-carbon source) as previously described by Zhao et al. [34] with modifications. The pH of the samples was adjusted to 2 with 5 M HCl and maintained at 25 °C for 10 min with occasional shaking. The samples were centrifuged at 5000 rpm for 20 min, and the supernatants were recovered and centrifuged again at 13,000 × g for 10 min. 4-methyl valeric acid (12% v/v in formic acid) was added to the fs as an internal standard to give a final concentration of 1 mM and injected for analysis. The SCFA analysis was carried out using GC (Clarus 580, PerkinElmer, Waltham, MA, USA) with a flame ionization detector and a capillarity column (Elite-FFAP 30 m × 0.50 mm I.D.; film thickness, 1 µm). The SCFA concentration was determined using calibration curves of acetic, propionic, and butyric acids. Results were expressed in mM.
2.2.7. Ferulic acid
FA, di-FA, and tri-FA were quantified by Reversed Phase-HPLC, as previously described [33],[34] after a de-esterification step. An Alltima C18 column (250 mm × 4.6 mm; Alltech Associates, Inc., Deerfield, IL, USA) and a photodiode array detector (Waters 996; Waters Corporation, Milford, MA, USA) were used. Detection was followed by UV absorbance at 320 nm. For free FA, di-FA, and tri-FA determination, the same procedure was followed with no de-esterification step.
2.2.8. Scanning electron microscopy
The surface microstructure of lyophilized AXP and residual AXP after the fermentation process was studied by scanning electron microscopy (JEOL 5410LV, JEOL, Peabody, MA, USA) at low voltage (20 kV). A secondary electron image mode was used to obtain the images.
2.3. AXP fermentation supernatant antiproliferative activity
2.3.1. Cell lines and culture conditions
The human colon cancer cell line, Caco-2 [Caco2] (ATCC® HTB-37), purchased from the American Type Culture Collection (ATCC, Manassas, VA, USA) was grown in Dulbecco's Modified Eagle Medium (DMEM) supplemented with 10% fetal bovine serum (FBS), 1% non-essential amino acids, 100 U/mL penicillin, and 100 mg/mL streptomycin. The cells were maintained at 37 °C and 5% CO2 in a humidified incubator (Thermo Fischer Scientific, San Jose, CA, USA) [35] and culture medium was renewed twice a week. The cultured cells were trypsinized (0.25% [w/v] Trypsin-0.53 mM EDTA solution) for a maximum of 7 min when they reached about 80% confluence and sub-cultivated (1 × 104 cells/cm2) in fresh DMEM supplemented with 10% FBS. For the experiments, cells were seeded and reached 80–90% confluence after 48 h experimental time.
2.3.2. Determination of cell proliferation
The effect of AXP-fs on the proliferation of the colon cancer cell line Caco-2 was determined following the standard 3-(4,5-dimethylthiazol-2-yl)-2,5-diphenyltetrazolium bromide (MTT) assay [35] with some modifications [36]. Cells (1 × 104 cells/50 µL) were seeded in 96-well microplates and incubated for 24 h at 37 °C in an atmosphere of 5% CO2 to allow cell adhesion. After incubation, an aliquot of fermentation supernatants (fs) in DMEM (50 µL, 250 µg/mL) was added to the cells to obtain a final concentration of 2.5% v/v and incubated for 24 h at 37 °C. Culture media alone was used as control and the cytotoxic drug 5-fluorouracil (5-FU) was used as positive control. In the last 4 h of the incubation period, 10 µL of MTT solution (5 mg/mL) were pipetted into each well. The cell viability was measured by the ability of metabolically active cells to reduce tetrazolium salt to purple formazan crystals. The resulting colored precipitates formed were dissolved with acidic (0.4%) isopropyl alcohol. The absorbance of the samples was measured at a test wavelength of 570 nm and a reference wavelength of 650 nm using an ELISA plate reader (Thermo Scientific MultiSkan Go, Madrid, Spain). Results were expressed as the number of viable cells exposed to treatments compared with the number of cells of control (medium alone).
2.4. Statistical analysis
Results of chemical analysis are expressed as means ± standard deviation (S.D) from triplicates. Data were analyzed using a one-way analysis of variance (ANOVA), and differences among means were compared with Tukey Kramer test (p ≤ 0.05) (NCSS, 2007, NCSS, LLC, Kaysville, TN, USA).
3.
Results and discussion
3.1. AXP characteristics
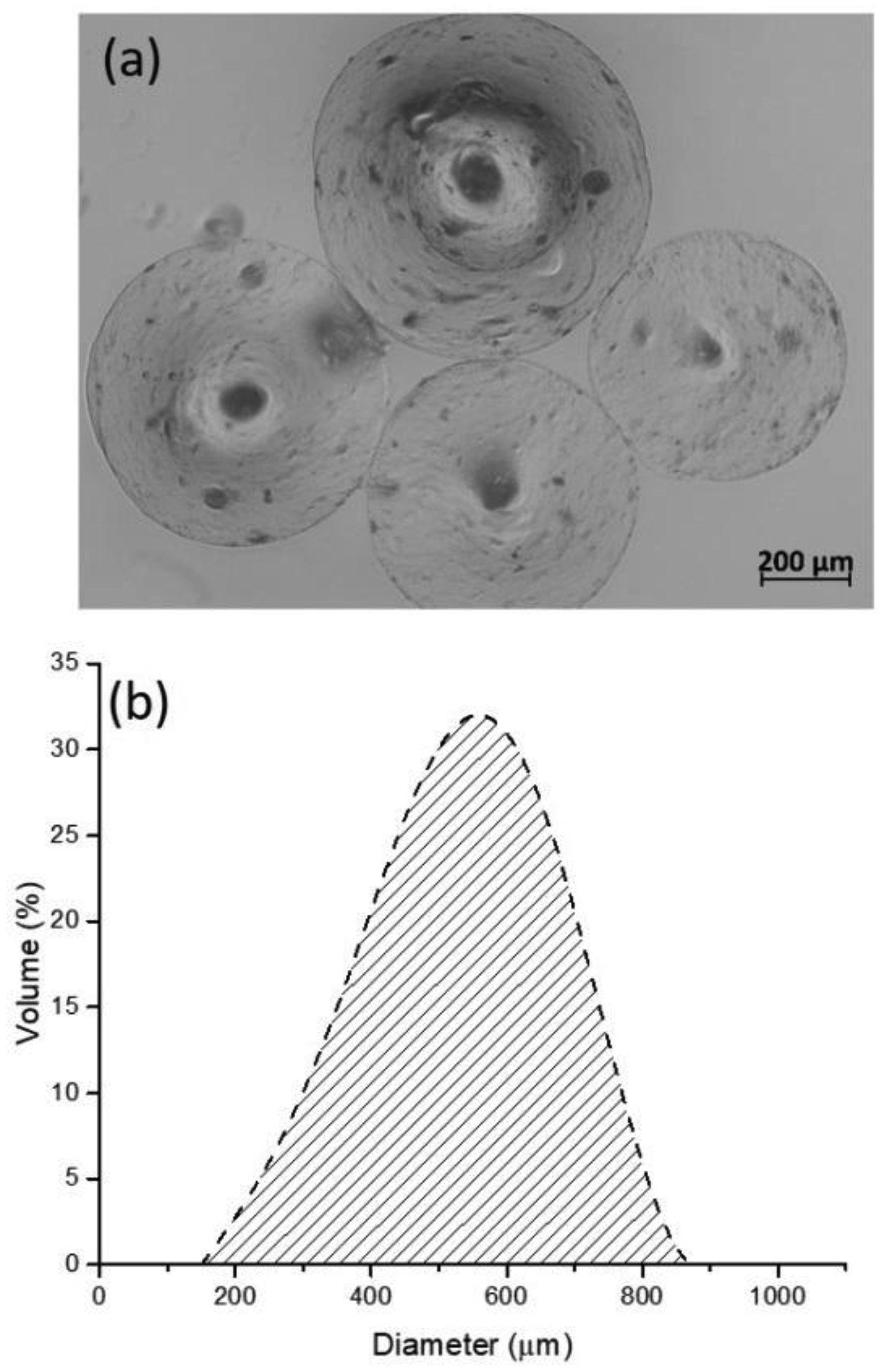
The AXP primary diameter ranged between 450 and 650 µm and presented an average diameter of 533 µm. The optical micrograph of AXP showed a spherical shape and no agammaegation of particles were observed (Figure 1). These characteristics are similar to those previously reported for AX microspheres in the literature with an average diameter between 531 and 533 µm [1],[37].
The cross-linking content (di-FA + tri-FA) in AXP was 1.65 µg/mg polysaccharide, which is high (0.03–0.20 µg/mg AX) in comparison to other AX gels and AX microspheres reported in the literature [3],[5],[28],[37] being therefore considered highly cross-linked AXP [12]. This elevated cross-linking content can be explained by the great FA content in the AX used in the present study (7.53 µg/mg polysaccharide).
3.2. Growth of bacteria in AXP
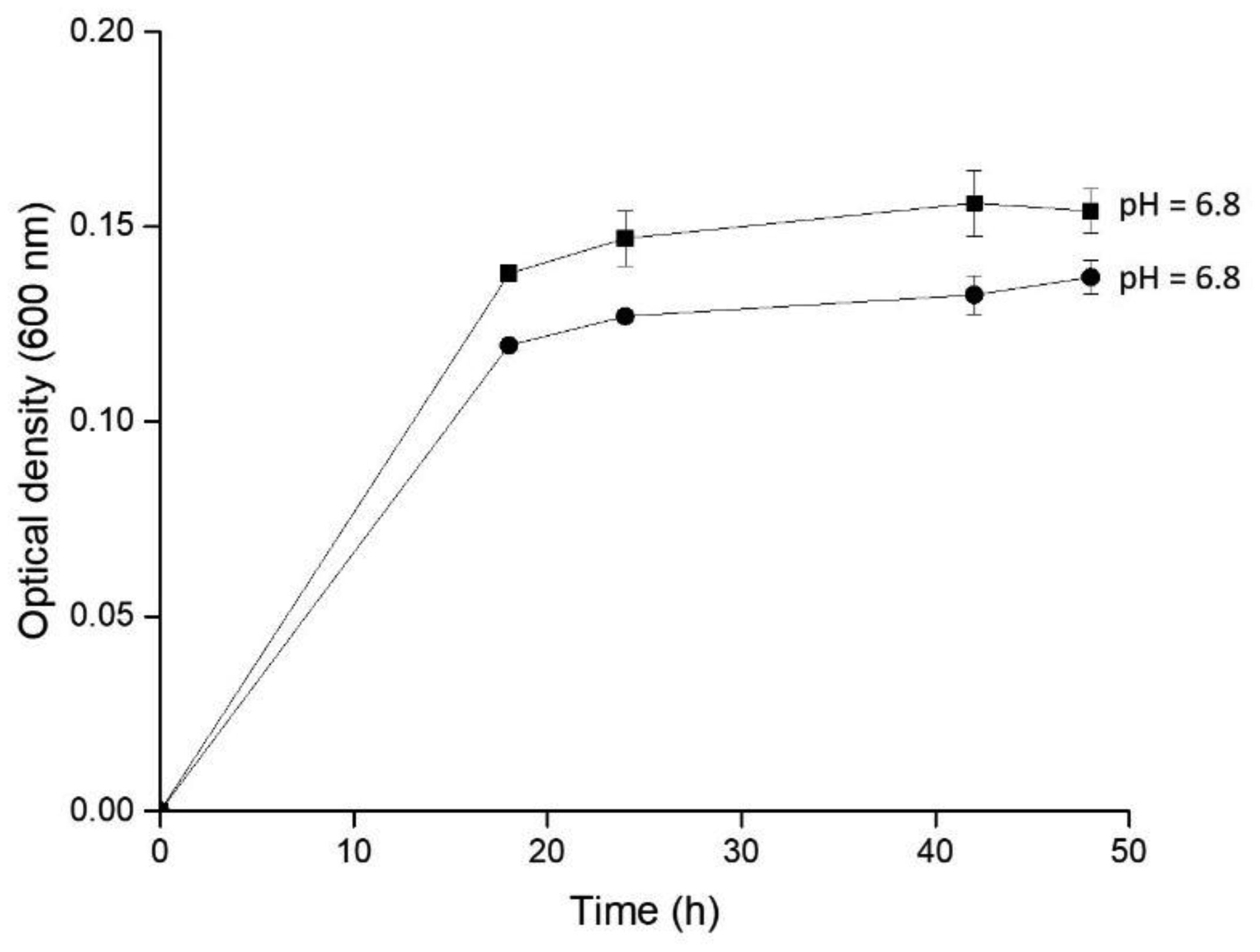
Three strains, Bifidobacterium longum (B. longum), Bifidobacterium adolescentis (B. adolescentis), and Bacteroides ovatus were selected to perform the in vitro fermentation of AXP as they have been reported able to degrade this polysaccharide [2],[3],[16]. Bifidobacteria are well-known probiotics, and these two species (B. longum and B. adolescentis), in particular, have been informed to ferment AX gels [3]. In this work, the ability to ferment AXP as a sole carbon source in the medium by a mixture of Bifidobacterium and Bacteroides strains was investigated. The growth of bacteria on AXP samples was assessed by measuring the optical density (OD) (Figure 2) and pH of the cultures during 48 h incubation.
Control and AXP samples did not show any change in pH after the incubation. However, pH changes should not be used as an exclusive indicator of fermentation, because fermentation is not always accompanied by a drop in pH [38]. Therefore, SE-HPLC method was also used to follow the degradation of polysaccharide by the bacteria. Crittenden et al. [33] found that AX was efficiently and low used as a growth substrate by B. longum and Bacteroides strains, respectively. Also, an evident growth of Bifidobacterium breve 286 on AX from wheat was observed by Paesani et al. [39]. The degradation of AX by Bifidobacteria has been demonstrated to be strain-dependent [40]. The structural features of AX, such as A/X ratio, the average degree of arabinose substitution, and the presence of phenolic acids, play a significant role in the capacity of bacteria to degrade the polysaccharide [27],[41]. Lower A/X ratio were more efficiently fermented than those highly substituted because xylanases prefer unsubstituted xylose regions, and most arabinofuranosidases act on monosubstituted xylose residues [41]. Feng et al. [42] also reported that AX with a low di-substituted xylan backbone are fermented faster by a porcine fecal microbiota. Moreover, the presence of FA esterified to arabinose can decrease the fermentability of AXOS as it limits the arabinofuranosidases activity [27]. The AXP formed in the present study, contained high amounts of di-FA and tri-FA in comparison to fermentable AX gels reported in the literature [3]. This raised cross-linking content in AXP could explain the weak growth of bacteria on these particles and the partial degradation of the polymeric network. Indeed, Hopkins et al. [2] reported that FA cross-links in AX restricted the access of xylanolytic enzymes from children microbiota resulting in a decrease in the polysaccharide fermentation rate. Martínez-López et al. [3] also found that AX gels were degraded more slowly (even entirely degraded) than uncross-linked AX by a Bifidobacteria mixture, suggesting restricted access of bacteria enzymes to their target sites due to the presence of di-FA. Similarly, Rascón-Chu et al. [30] reported the complete degradation of AX gels with a low content of di-FA, by a mixture of Bacteroides ovatus and B. longum. At the opposite to our results, these previous studies reported the complete degradation of AX gels by bacterial mixtures, probably due to their low cross-linking contents (0.3–0.4 µg/mg AX) compared with the values found for AXP (1.65 µg/mg polysaccharide) which could limit the accessibility of bacterial enzymes to their hydrolysis sites.
3.3. AXP-fs characterization
3.3.1. FT-IR spectroscopy
AXP and AXP-fs showed a spectral pattern similar to those found for AX and AX gels [31],[43] (Figure 3). An increase at 1414 cm−1 could be associated with the CO asymmetric stretch suggesting the presence of ester groups due to the cross-linking process [43]. The spectral pattern in the region 1200–900 cm−1 is typical for polysaccharides such as AX, which is indicated by the presence of signals at 1045 and 898 cm−1 attributed to the antisymmetric C-O-C stretching mode of the β-(1-4) linkage between the xyloses units of AX backbone [44],[45]. The absorbance region from 3500 to 1800 cm−1 corresponds to the fingerprint region of polysaccharides related to AX, with two bands at 3400 cm−1 and 2900 cm−1 associated with the OH stretching and CH2 groups, respectively [46],[47]. The spectra also showed the absorption band of protein at 1548 cm−1 (amide II) [44]. These results indicate the presence of AX in AXP-s after fermentation. The presence of unknown peaks observed in the spectra could be related to some culture media components present in the samples.
3.3.2. Molecular weight distribution
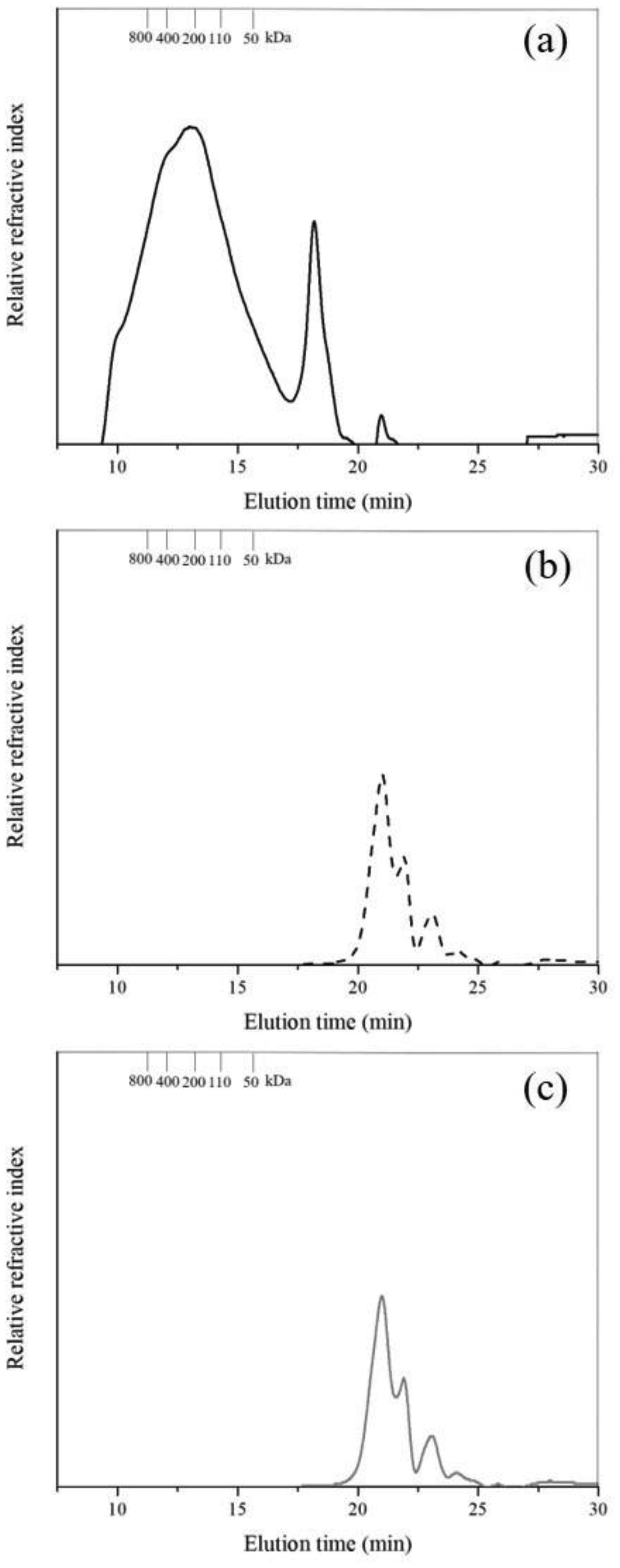
The fermentation of AXP was investigated by analyzing the molecular weight (Mw) distribution pattern of their hydrolysis products by SE-HPLC after 48 h incubation (Figure 4). This analysis gives information related to the degradation degree of the polysaccharide by bacteria. Figure 4a corresponds to the Mw distribution pattern of the polysaccharide in AXP before degradation. The elution profile of AXP-fs (Figure 4b) was similar to that registered for culture media (Figure 4c).
It is possible that only some sugars and polysaccharide chains of low Mw were released during AXP fermentation and therefore not detected in AXP-fs. These findings agree with an incomplete degradation of AXP by bacteria, which could result in the release of only a few polymer chains with low Mw from the AXP surface. The degradation of AX gels by Bifidobacteria mixture has been reported previously by Martinez-Lopez et al. [3]. In this study, the authors found that the presence of two Bifidobacterium strains (B. longum and B. adolescentis) are needed for the degradation of the gel structure. Bifidobacteria species can produce several enzymes that are required for the degradation of AX, such as xylosidases, arabinofuranosidases, and ferulate esterases [48]. Snelders et al. [49] found that the presence of esterified FA in AXOS inhibited arabinose fermentation. Notably, the FA esterified to arabinose decreases arabinofuranosidase action by a steric hindrance [50]. Thus, the high content of FA and cross-linking structures (di-FA and tri-FA) present in AXP may have limited the access and action of specific bacterial enzymes such as arabinofuranosidases and decrease the degree of degradation and its subsequent fermentation.
3.3.3. SCFA production
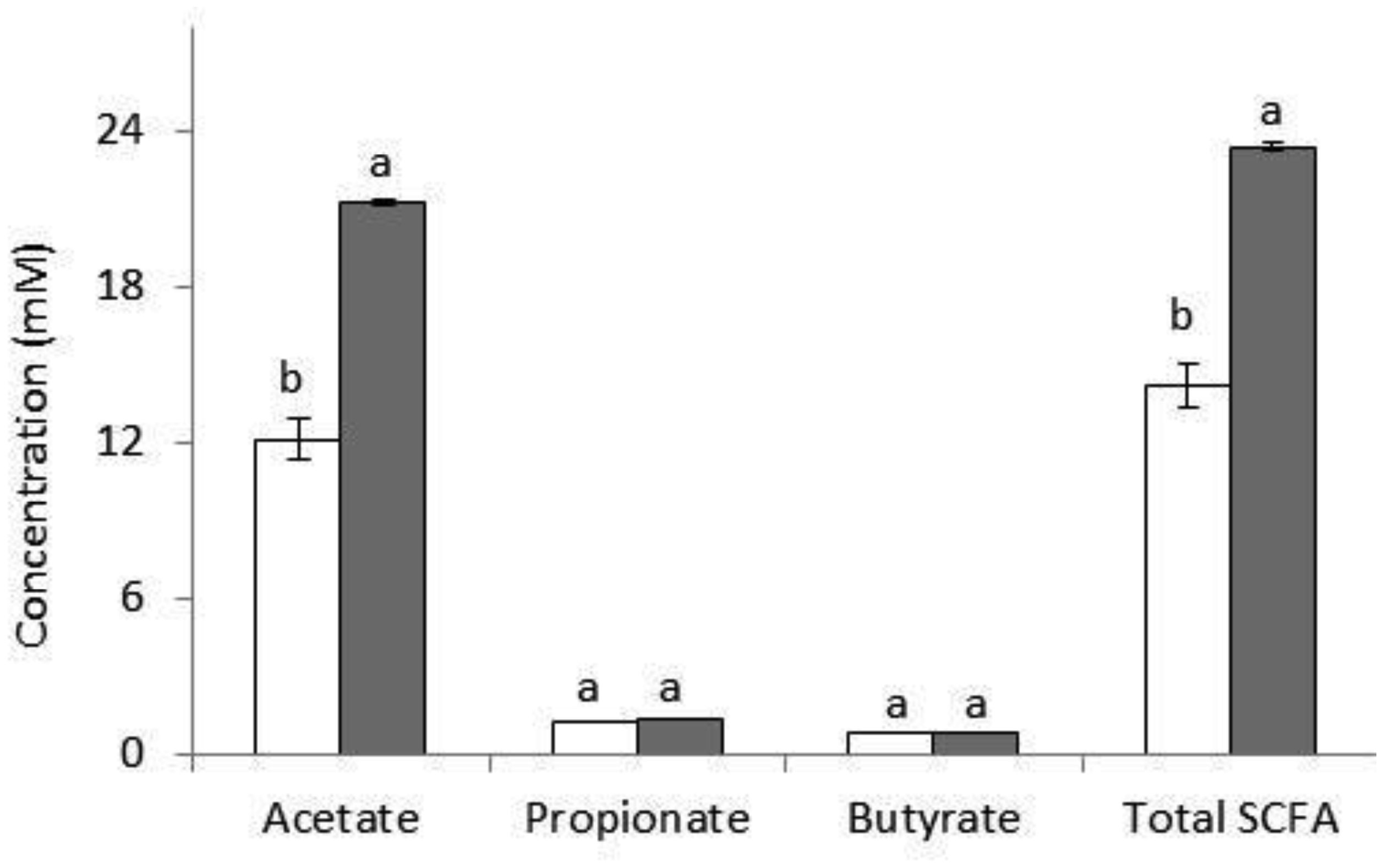
The production of SCFA after the in vitro fermentation of AXP by the bacterial mixture is shown in Figure 5. These results are consistent with the findings reported above. AXP produced a higher amount of total SCFA in comparison with the control (no-carbon source), indicating that they were fermented by bacteria to some extent. Pastell et al. [26] found that the growth of Bifidobacteria in AXOS correlated with an increase in acetate concentration and low pH. On the other hand, a previous study stated that the production of SCFA decreases as the cross-linking increases in AX gels [3]. Therefore, the low SCFA concentration detected in AXP could be mostly related to its high cross-linking content. In general, the SCFA concentration in the samples follows the order acetate > propionate > butyrate.
Acetic acid has been reported as the dominant SCFA produced during the in vitro fermentation of AX gels by Bifidobacteria, followed by propionic and butyric acids as the less abundant metabolites [3]. The high concentrations of acetate could be related to the stimulation of Bifidobacteria species by AX since it is the primary fermentation product of these microorganisms [25]. Production and amount of propionic acid have been related to an increase in Bacteroides population [51]. According to these results, AXP could be used for the development of colon-targeted delivery carriers with slow-fermentable properties.
3.3.4. Covalent cross-linking content after fermentation
The changes in FA, di-FA, and tri-FA contents of AXP, and AXP-fs are compared in Table 1. Besides, quantification of free FA, di-FA, and tri-FA in AXP-fs was performed to quantify the phenolic acids released during fermentation by bacteria. Only a small amount of FA (0.02 µg/mg AX) was detected in the AXP-s, while no free FA was detected in any sample. Probiotic bacteria like Lactobacillus and Bifidobacterium produce ferulate esterases, enzymes required to cleave the FA esterified to arabinose in AX [2],[48]. Hopkins et al. [2] reported that AX was fermented by human fecal microbiota more rapidly than cross-linked AX, probably due to limited enzymes access to the xylose chain. Indeed, the inhibition of endoxylanase activity by phenolic acids has been previously reported [27]. It has been informed that the presence of high content of bound FA in AXOS slowed down fermentation by decreasing arabinofuranosidase activity [27]. A previous study using similar concentration of AX gels (5 g/L w/v) than that used in the present study reported that a more compact microstructure and higher crosslinking density result in slower degradation of the three-dimensional gel structure by fermentation with a mixture of Bifidobacterium [3]. The results found in the present study indicate that the high cross-linking content in AXP could have limited the access of bacterial enzymes to target cleavage sites in AX, therefore limiting the release of FA and subsequent polysaccharide fermentation.
3.3.5. Surface morphology of AXP after degradation by bacteria
The surface morphology of lyophilized AXP before and after fermentation was studied using scanning electron microscopy (Figure 6). AXP shows a spherical shape with a porous and rough surface. Previous studies have also observed wrinkled, rugged, and assorted coatings for AX particles and microspheres [1],[12]. Although some pores can be observed on the surface, the particle exhibits a complete and well-defined structure. Nevertheless, after 48 h incubation, the residual AXP reveals a collapsed and disintegrated structure. Several sheet-form structures were observed in the remaining sample after incubation, which evidences the partial degradation of AXP by bacteria.
Martínez-López et al. [3] reported that the degradation of AX gels by Bifidobacteria resulted in the loss of their structure and development of some cavities, which increased in number and size with incubation time. Moreover, these authors suggested that AX gels with a more compact structure and a higher content of cross-linking structures (di-FA) would limit the accessibility of the gel to bacterial enzymes resulting in a slow degradation. When AX are cross-linked by di-FA bonds, arabinose moieties are preferentially utilized by bacterial enzymes because the gel-like structure restricts the access of xylanolytic enzymes to the xylan backbone [2]. Thus, it is possible that the compact microstructure of AXP due to its high content of cross-linking structures (Table 1) hindered the access of bacterial enzymes to the internal sites of the gel and only permitted the degradation of external areas resulting in a partial degradation.
3.4. Effect of AXP-fs on the inhibition of Caco-2 cells proliferation
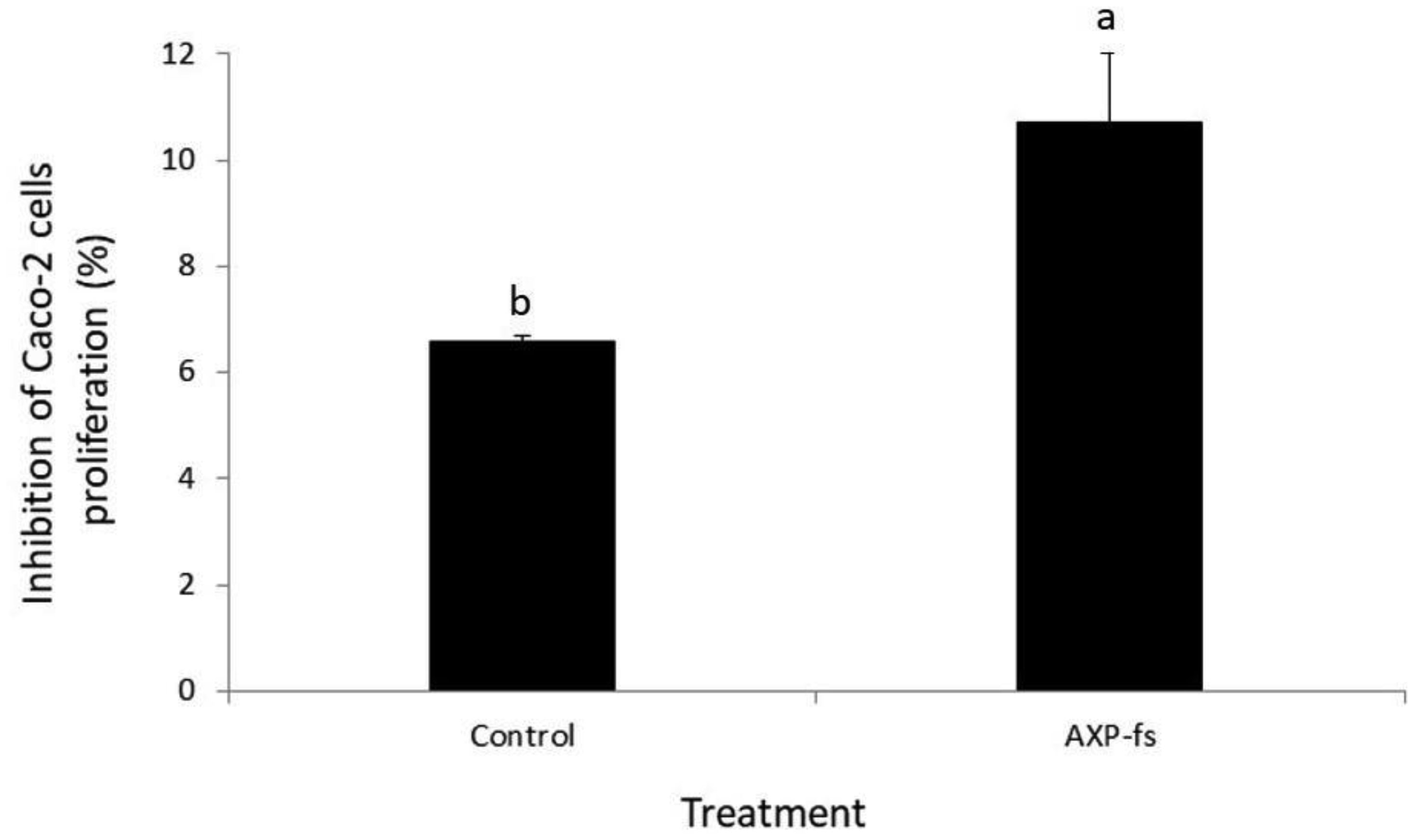
Only one study has evaluated the effect of AX fermentation supernatant on the proliferation of colon cancer cells [20], and to the best of our knowledge, the effect of AXP-fs on colon cancer cell proliferation has not been reported so far. Figure 7 presents the inhibition of proliferation of Caco-2 cells exposed to AXP-fs. The use of dissolvent in the cell cultures caused no cell damage. AXP-fs exerted slight inhibition of Caco-2 cells proliferation by 11%. A slight anti-proliferative effect (<20%) on Caco-2 cells proliferation by chlorogenic acid and its microbial metabolites was previously reported [52]. It has been widely reported the beneficial effects of SCFA, concerning their protective effect against colon carcinogenesis development [53]–[55]. Also, acetate, which was the most abundant SCFA produced during the fermentation of AXP, has anti-inflammatory properties [56]. Recently, Ohara and Mori [18] reported that SCFA, butyrate, and acetate, exerted antiproliferative effect against human colorectal cancer cells (DLD-1 cell line) through a mechanism involving the inhibition of genes encoding proteins involved in DNA replication and cell cycle/proliferation that contribute to major pathways responsible for the suppression of colorectal carcinogenesis pathways. In addition, acetate induces apoptosis in colorectal cancer cells via lysosomal membrane permeabilization and subsequent release of cathepsin D undergoing apoptosis [19]. In this study, the cytotoxic drug 5-FU (positive control) at the concentration of 26 µg/mL inhibited the Caco-2 cell proliferation by 64% (data not shown). 5-FU is a drug frequently used in the treatment of colorectal cancer that works by inhibiting essential biosynthetic processes or by being incorporated into macromolecules such as DNA and RNA and affecting their normal function [57].
Previous research found that fermentation supernatant from wheat AX generated using human feces inhibited the proliferation of the colon cancer cell line HT29 by 75% [20]. This difference in the antiproliferative effect could be related to the polysaccharide structural characteristics (molecular weight, FA, di-FA, and tri-FA content, arabinose substitution degree, among others) and the bacteria population contained in the human feces. A deferulated or uncross-linked AX structure can be degraded more easily by the bacteria, in comparison with a highly ferulated and cross-linked structure. Besides, the AX fermented with human gut microbiota were exposed to a wide variety of bacterial species, while only three pure cultures were used in the present study. AXP were not entirely fermented by the bacterial mixture, as previously evidenced by the SE-HPLC (Figure 4) and SEM (Figure 6) analysis. Thus, the incomplete degradation of AXP could have resulted in a limited production of metabolites responsible for the antiproliferative effect. It is important to notice that AXP were fermented using a bacterial mixture constituted by three pure strains, which could limit their fermentation and subsequent production of metabolites such as SCFA and free FA. Previous studies have demonstrated that cross-linked AX microspheres can be degraded by the intestinal microbiota of diabetic rats [5], which could promote the production of SCFA and favor the antiproliferative activity. However, those AX microspheres contained low amounts of di-FA (0.04 µg/mg AX) and tri-FA (0.009 µg/mg AX), facilitating their fermentation. Although the gut microbiota comprises a wide variety of bacterial species, which can produce different enzymes capable of degrading AX, further studies are needed to investigate the degradation of AXP by gut microbiota. AX gels are mainly fermented in the colon by the microbiota that inhabits this site as has been confirmed by previous in vitro and in vivo studies. Electrosprayed AX particles entrapping Bifidobacteria and insulin were evaluated in a simulator of the human gastrointestinal tract (Simgi) and it was found that only 24% of the insulin entrapped was lost before the particles reach the colon compartments of Simgi, while 76% of this hormone was released in the colon. Moreover, an increase in the Bifidobacteria population which was attributed to the degradation of the particles by these bacteria and also to the bacteria entrapped in the particles was observed [4]. Also, the bioavailability of insulin entrapped in AX microspheres in induced-diabetic rats was evaluated by previous research. An hypoglycemic effect was observed between 6 and at least 24 h which confirms the slowly release of the hormone due to the degradation of AX microspheres by bacteria in the colonic region [5]. Thus, considering that AXP could be slowly fermented in the colon, the production of beneficial metabolites such as SCFA will increase in this area contributing to enhancing the antiproliferative properties. The AXP could be attractive biomaterials with anticancer effect for use in the prevention and/or treatment of colorectal cancer.
4.
Conclusion
Electrosprayed highly cross-linked AX particles (AXP) presenting a mean diameter of 533 µm, a spherical shape, and a cross-linking content of 1.65 µg/mg polysaccharide are partly fermented by the bacterial mixture Bifidobacterium longum, Bifidobacterium adolescentis, and Bacteroides ovatus. AXP partial fermentation generates a moderate short-chain fatty acids production (23 mM). AXP fermentation supernatant (AXP-fs) containing short-chain fatty acids inhibits the proliferation of the human colon cancer cell line Caco-2 cells by 11%. Electrosprayed AXP could be considered as a biomaterial presenting slight antiproliferative properties and potential application in colon cancer prevention. Additional studies regarding the fermentation of AXP by the gut microbiota and the metabolites generated could lead to a better understanding of AXP mechanisms of action in colon cancer prevention.









 DownLoad:
DownLoad: