New stability theorems of uncertain differential equations with time-dependent delay

1.   Introduction

More than half a century ago, when the Itô's ^[1] landmark work on stochastic differential equations (Itô, 1951) comes out, the stochastic differential equations (SDEs), as a new branch of mathematics, have aroused great interest in academic circles. In recent decades, SDEs have been widely applied in many fields such as physical, biological, engineering, medical, social sciences, economics, finance and other models hidden in the observed data. One of the best important works in this research field is to discuss the stability of such systems. For example, in 2017, Zhu and Zhang ^[2] considered the pth moment exponential stability criterion instead of the mean square exponential stability criterion and discrete-time state observations depend on time delays. In 2018, Zhu ^[3] studied the pth moment exponential stability problem for a class of stochastic delay differential equations driven by Lévy processes. In 2019, Zhu ^[4] investigated the stabilization problem of stochastic nonlinear delay systems with exogenous disturbances and the event-triggered feedback control by introducing the notation of input-to-state practical stability and an event-triggered strategy. In 2020, Ma et al. ^[5] focused on the the mean square and almost sure practical exponential stability for a class of stochastic age-dependent capital system with Lévy noise. Zhu and Huang ^[6] investigated the pth moment exponential stability problem for a class of stochastic time-varying delay nonlinear systems driven by G-Brownian motion.

It is well known that all results about SDEs are based on an axiomatic probability theory, and large amounts of sample data is needed to obtain the frequency of their random disturbances. Furthermore, their distribution functions can be obtained. However, in reality, people seem to lack data or the size of sample data applied in practice are less in some cases, such as the emerging infectious disease model, the new stock model and so on. Although sometimes we have a lot of available sample data, the frequency obtained by sample data is, unfortunately, not close enough to the distribution function obtained in some practical problems, and we need to invite some domain experts to evaluate the belief degree that each event may happen in these situations. Human uncertainty with respect to belief degrees can play a crucial role in addressing the issue of indeterminate phenomenon. For describing human uncertainty, a considerable amount of literatures about uncertainty theory (for example, Liu ^[7,8]) have been published on Springer-Verlag. Recently, investigators have examined the effects of uncertainty theory on programming model and application ^[9], risk and reliability analysis ^[10], set theory and control theory ^[11], logic logic for modeling human language ^[12] and currency model ^[13] and the references therein.

In the framework of uncertainty theory, there has been an increasing amount of literature on uncertain differential equations ^{[14,15,16,17]}. In particular, uncertain process and Liu process for such perturbed systems were investigated extensively in ^[14,15]. Existence and uniqueness theorem for uncertain differential equations were derived in ^[16,17]. A broader perspective has been adopted by Liu ^[18] who argued that an analytic method can solve uncertain differential equations. Similarly, Yao ^[19], Liu ^[20], Wang ^[21] found that the nonlinear uncertain differential equation, semi-linear uncertain differential equation, the general uncertain differential equation have their analytic solution. More importantly, numerous studies have attempted to explain solution of an uncertain differential equation (for example, Yao and Chen, ^[22]; Yao, ^[23]). Using these theoretical approaches, researchers have been able to develop the applications of uncertain differential equation successfully. A great deal of previous research into applications has focused on financial systems ^[24,30], game theory ^[25], heat conduction ^[26], uncertain wave equation ^[27,28,29], uncertain differential equations with jump ^[31,32] and the references therein.

Since 2009, after the concept of stability ^[15] for an uncertain differential equation showed firstly, there have been more interest in the stabilities of uncertain differential equation. Being stable in measure ^[33], being stable in $p$-th moment ^[34], stability in mean ^[35], exponential stability ^[36], almost sure stability ^[37] and being stable in inverse distribution ^[38] were successively investigated. Researchers often use uncertain delay differential equations to describe such physical conditions that are related to both the current state and the past state (for example, Barbacioru ^[39]; Ge et al. ^[40]; Wang et al. ^[41]). Following that, Wang et al. ^[42,43] put forward the stability in measure, stability in mean and stability in $p$-th moment for uncertain delay differential equations, and proved the corresponding stability theorems. Jia et al. ^[44] discussed the stability in distribution. The above uncertain delay differential equations can be seen as constant delay. Recently, Wang et al. ^[45] proposed another class of uncertain differential equations with time-dependent delay including stability in measure, stability in mean, which are not applicable for all situations. For completeness, our goal is to provide the almost sure stability, stability in $p$-th moment and stability in distribution of the uncertain differential equations with time-dependent delay as a supplement. The equation is introduced as follows:

where $C_{k}$ is a Liu process with respect to time $k$, and $h$ and $q$ are two continuous functions, $Z_{0}$ is the initial value, $\delta :[0, +\infty)\rightarrow[0, \tau]$ is a delay function. Its equivalent integral form is as follows:

The rest of this article is organized as follows. In Section 2, we define almost sure stability, $p$-th moment stability and stability in distribution for uncertain differential equations with time-dependent delay, In Section 3, firstly, we derive a sufficient condition of almost sure stability and a corollary for uncertain differential equations with time-dependent delay. Secondly, the $p$-th moment stability theorem and corollary of uncertain differential equations with time-dependent delay are presented. Thirdly, we prove a sufficient condition of being stable in distribution for uncertain differential equations with time-dependent delay. Fourthly, we prove some relationships about stabilities for the uncertain differential equation with time-dependent delay. Finally, we make a brief conclusion in Section 4.

2.   Some important concepts

In this section, we will define some concepts of stability.

Definition 1. The uncertain differential equation with time-dependent delay (1.1) is said to be stable almost surely if for any two solutions $Z_{k}$ and $\hat{Z}_{k}$ with different initial states, respectively. We have

where $\mathcal{M}$ is uncertain measure and $\Gamma$ is a nonempty set defined in ^[8].

Definition 2. The uncertain differential equation with time-dependent delay (1.1) is said to be stable in $p$-th moment if

where $E$ is uncertain expected value defined in ^[8].

Definition 3. Let $Z_{k}$ and $\hat{Z}_{k}$ be two solutions of uncertain differential equation with time-dependent delay (1.1) with different initial states. Assume the uncertainty distributions of $Z_{k}$ and $\hat{Z}_{k}$ are $\Phi_{k}(z)$ and $\Psi_{k}(z)$, respectively. Then the uncertain differential equation with time-dependent delay (1.1) is said to be stable in distribution if

$\forall z$ at which $\Phi$ and $\Psi$ are continuous.

3.   Main results

We will give a sufficient condition for (1.1) being stable almost surely.

3.1.   Stable almost surely

Theorem 1. Assume uncertain differential equation with time-dependent delay (1.1) has an unique solution for each given initial state. Then it is stable almost surely if the coefficients $h(k, z, \hat{z})$ and $q(k, z, \hat{z})$ satisfy

where $N_{k}$ is a bounded function satisfying

Proof Suppose that $Z_{k}$ and $\hat{Z}_{k}$ are two solutions of the equation (1.1) with different initial states $\varphi(k)$ and $\psi(k) (k \in[-\tau, 0])$, respectively. Then,

and

Then for a Lipschitz continuous sample $C_{k}(\gamma)$, it holds that

and

where $\gamma\in\Gamma$ in Definition 2.2 ^[45]. By condition (3.1) and Lemma 4.1 in ^[16], we have

where $H(\gamma)$ is the Lipschitz constant of the sample path $C_{k}(\gamma)$.

According to Gronwall inequality ^[45], then we have

Thus we have

Thus, by Theorem 2 in ^[33], we have

Since

We have

Hence, we have $|Z_{k}-\hat{Z}_{k}|\rightarrow0$ as long as $\sup\limits_{r\in[-\tau, 0]}|Z_{r}-\hat{Z}_{r}|\rightarrow0$, which implies that

is stable almost surely according to Definition 1. This completes the proof.

Corollary 1. Supposing that $u_{ik}$, $v_{ik}$, and $\eta_{ik}$ (i = 1, 2) are real-valued functions, then the linear uncertain differential equation with time-dependent delay

is almost surely stable if $u_{ik}$, $v_{ik}$, and $\eta_{ik}$ (i = 1, 2) are bounded, and satisfy

and

Proof Take $h(k, z, \hat{z}) = u_{1k}z+v_{1k}\hat{z}+\eta_{1k}$ and $q(k, z, \hat{z}) = u_{2k}z+v_{2k}\hat{z}+\eta_{2k}$. Let $Q$ denote a common upper bound of $|u_{ik}|$, $|v_{ik}|$, and $|\eta_{ik}|$ (i = 1, 2). The inequalities

and

hold.

According to Theorem 4.1 in ^[45], we have that linear uncertain differential equation with time-dependent delay (3.6) with initial states has a unique solution. Since

we take $N_{k} = u_{1k}\vee u_{2k}$, which is integrable on $[0, +\infty)$. Since, we have

and

By using Theorem 1, the linear uncertain differential equation with time-dependent delay (3.6) is almost surely stable.

Example 1. Consider an uncertain differential equation with time-dependent delay

where $a, b, c\in R.$ It follows from conditions, real-valued functions $\exp(-k)$, $|b \exp(-k)|$, and $|c \exp(-k)|$ are bounded on the interval $[0, +\infty)$, and let $Q$ denote a common upper bound of $\exp(-k)$, $|b \exp(-k)|$, and $|c \exp(-k)|$. The inequalities

and

hold.

According to Theorem 4.1 in ^[45], we have that linear uncertain differential equation with time-dependent delay (3.7) with initial states has a unique solution. Since

According to Corollary 1, linear uncertain differential equation with time-dependent delay (3.7) is stable almost surely.

By (3.7), we get

Because $\Delta C_{k}$ is a normal uncertain variable with expected value 0 and variance $\Delta k^{2}$, the distribution function of $\Delta C_{k}$ is $\Phi(x) = \left(1+\exp\left(\frac{-\pi x}{\sqrt{3} \Delta k}\right)\right)^{-1}, \ \ x\in\Re.$ We may get a sample point $\hat{z}_{k}$ of $\Delta C_{k}$ from $\hat{z}_{k} = \Phi^{-1}(\alpha)$ that $\hat{z}_{k} = \frac{\sqrt{3} \Delta k}{-\pi}\ln(\frac{1}{\alpha}-1)$, $\alpha\in(0, 1),$ where $\alpha$ is belief degree. So $\Delta Z_{k}$ can be given by the following equation.

Thus, $Z_{k}$ can be simulated by the following ordinary differential equation with time-dependent delay.

We take $a = 1$, $b = 2$, $c = 3$, $\alpha = rand(0, 1)$. Figure 1 gives the the value of $Z_{k}$, which illustrates the stability furthermore.

3.2.   Stability in $p$-th moment

This part mainly investigates the stability in $p$-th moment for uncertain differential equation with time-dependent delay (1.1).

Theorem 2. Assume that uncertain differential equation with time-dependent delay (1.1) has an unique solution for each given initial state. Then it is stable in $p$-th moment if the coefficients $h(k, z, \hat{z})$ and $q(k, z, \hat{z})$ satisfy

where $N_{k}$ and $G_{k}$ are two bounded functions satisfying

Proof Suppose that $Z_{k}$ and $\hat{Z}_{k}$ are two solutions of the equation (1.1) with different initial states $\varphi(k)$ and $\psi(k) (k \in[-\tau, 0])$, respectively. Then,

and

Then for a Lipschitz continuous sample $C_{k}(\gamma)$, we have

and

By condition (3.8) and Lemma 4.1 in ^[16], we have

where $H(\gamma)$ is the Lipschitz constant of $C_{k}(\gamma)$.

According to Gronwall inequality ^[45], then we have

Thus we have

where $H$ is a nonnegative uncertain variable. Taking expected value on both sides of expression (3.13), we have

Because

we immediately have

Denote $\kappa = \int_{0}^{+\infty}G_{r}dr < \frac{\pi}{\sqrt{3}p}$. Thus, It follows from the definition of uncertain expected value, Definition 6 in ^[15] and Theorem 2 ^[33] in that

Because $\kappa = \int_{0}^{+\infty}G_{r}dr < \frac{\pi}{\sqrt{3}p}$. Let $\frac{\pi }{\sqrt{3}\kappa p} = a$, so $a > 1$. Then,

Since $\frac{1}{1+{{x}^{a}}} < \frac{1}{{{x}^{a}}}, a > 1,$ while $\int_{1}^{+\infty }{\frac{1}{{{x}^{a}}}}dx, a > 1$ is convergent, so $\int_{1}^{+\infty }{\frac{1}{1+{{x}^{a}}}}dx, a > 1$ is convergent. So $1+2\int_{1}^{+\infty }{{{\left\{ 1+{{x}^{\frac{\pi }{\sqrt{3}\kappa p}}} \right\}}^{-1}}}dx < \mathit{\text{}}+\infty.$ That is,

Thus, we can get

Corollary 2. Supposing that $u_{ik}$, $v_{ik}$, and $\eta_{ik}$ (i = 1, 2) are real-valued functions, then the linear uncertain differential equation with time-dependent delay

is almost surely stable if $u_{ik}$, $v_{ik}$, and $\eta_{ik}$ (i = 1, 2) are bounded,

and

Proof Take $h(k, z, \hat{z}) = u_{1k}z+v_{1k}\hat{z}+\eta_{1k}$ and $q(k, z, \hat{z}) = u_{2k}z+v_{2k}\hat{z}+\eta_{2k}$. Let $Q$ denote a common upper bound of $|u_{ik}|$, $|v_{ik}|$, and $|\eta_{ik}|$ (i = 1, 2). The inequalities

and

hold.

According to Theorem 4.1 in ^[45], we have that linear uncertain differential equation with time-dependent delay (3.15) with initial states has a unique solution. Since

we take $N_{k} = u_{1k}$, $G_{k} = u_{2k}$, which is integrable on $[0, +\infty)$. Since, we have

and

By using Theorem 2, uncertain differential equation with time-dependent delay (3.15) is stable in $p$-th moment.

Example 2. Consider an uncertain differential equation with time-dependent delay

It follows from Theorem 4.1 in ^[45] that uncertain differential equation with time-dependent delay has a unique solution with given initial states. In addition, real-valued functions $\exp(-k)$ is bounded on the interval $[0, +\infty)$, and

and

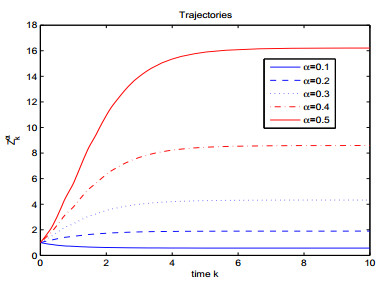
According to Corollary 2, uncertain differential equation with time-dependent delay (3.16) is stable in $p$-th moment. Similar to Example 1, we take $a = 3$ and give the Figure 2, which illustrates the stability furthermore.

Remark 1. When $p = 1$, stability in p-th moment is just stability in mean.

3.3.   Stability in distribution

In this section, we investigate the stability in distribution for uncertain differential equation with time-dependent delay, and prove a sufficient condition for an uncertain differential equation with time-dependent delay being stable in distribution.

Theorem 3. Assume the uncertain differential equation with time-dependent delay (1.1) has an unique solution for each given initial state. Then it is stable in distribution if the coefficients $h(k, z, \hat{z})$ and $q(k, z, \hat{z})$ satisfy

where $N_{k}$ is a bounded function satisfying

Proof Suppose that $Z_{k}$ and $\hat{Z}_{k}$ are two solutions of the Eq (1.1) with different initial states $\varphi(k)$ and $\psi(k) (k \in[-\tau, 0])$, respectively. Then,

and

Then for a Lipschitz continuous sample $C_{k}(\gamma)$, we have

and

By Theorem 4.1 in ^[38], the inverse uncertainty distributions $\Phi_{k}^{-1}(\alpha)$ and $\Psi_{k}^{-1}(\alpha)$ of $Z_{k}$ and $\hat{Z}_{k}$ satisfy the ordinary differential equation with time-dependent delay

respectively, where

It follows that

By the Gronwall's inequality, we have

Since

there exists a real number $Q > 0$ such that

for any $k \geq0$. Thus, for any given $\epsilon > 0$, we set $\delta = \epsilon/Q$ such that

for any $k \geq 0$ provided $\sup\limits_{r\in[-\tau, 0]}|Z_{r}-\hat{Z}_{r}| < \delta$. Then we have

By Theorem 3.1 in ^[38], we obtain

So, the uncertain differential equation with time-dependent delay (1.1) is stable in distribution.

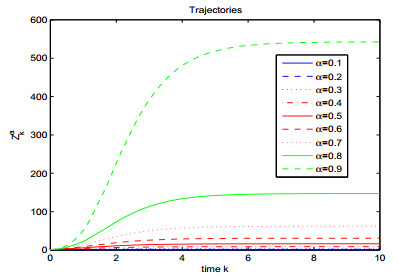
Example 3. Consider (3.16) of Example 2 again, similar to Example 1, Figure 3 gives $Z_{k}^{\alpha}$ of $\alpha = 0.1, \ldots, \alpha = 0.9$. Figure 4 enlarges the Figure 3 at $\alpha = 0.1, \ldots, \alpha = 0.5$. We can see from Figures 3 and 4 that the uncertain differential equation with time-dependent delay (3.16) is stable in distribution.

3.4.   Comparison of stability

Theorem 4. If the uncertain differential equation with time-dependent delay (1.1) is stable in $p$-th moment, then it is stable in measure.

Proof Suppose that $Z_{k}$ and $\hat{Z}_{k}$ are two solutions of the Eq (1.1) with different initial states $\varphi(k)$ and $\psi(k) (k \in[-\tau, 0])$, respectively. Then, it follows from the definition of stability in $p$-th moment that

By Markov inequality, for any given real number $\epsilon > 0$, we have

Therefore, from Definition 5.1 in ^[45], $p$-th moment stability implies the stability in measure.

Theorem 5. For any two real numbers $p_{1}$ and $p_{2} \; (0 < p_{1} < p_{2} < +\infty)$, if the uncertain differential equation with time-dependent delay (1.1) is stable in $p_{2}$-th moment, then it is stable in $p_{1}$-th moment.

Proof Suppose that $Z_{k}$ and $\hat{Z}_{k}$ are two solutions of the Eq (1.1) with different initial states $\varphi(k)$ and $\psi(k) (k \in[-\tau, 0])$, respectively. Then, it follows from the definition of stability in $p_{2}$-th moment that

According to Holder's inequality, we have

Thus, stability in $p_{2}$-th moment implies stability in $p_{1}$-th moment when $p_{1} < p_{2}$.

Theorem 6. If the uncertain differential equation with time-dependent delay (1.1) is stable in measure, then it is stable in distribution.

Proof Suppose that $Z_{k}$ and $\hat{Z}_{k}$ are two solutions of the Eq (1.1) with different initial states $\varphi(k)$ and $\psi(k) (k \in[-\tau, 0])$, respectively. According to Definition 5.1 in ^[45], we have

for any given number $\epsilon > 0$. Suppose $z$ is a given real number. On the one hand, for any $\hat{z} > z$, we can get

By the monotonicity theorem and subadditivity axiom in uncertainty theory ^[8], it holds that

Thus we have

We can get $\mathcal{M}\left\{\sup\limits_{k > 0}|Z_{k}-\hat{Z}_{k}|\geq \hat{z}-z\right\}\rightarrow0$ as $\sup\limits_{r\in[-\tau, 0]}|Z_{r}-\hat{Z}_{r}|\rightarrow0.$ Then we have

for any $\hat{z} > z$. Letting $\hat{z}\rightarrow z^{+}$, we have

On the other hand, for any $\tilde{z} < z$, it is obvious that

By the monotonicity theorem and subadditivity axiom in uncertainty theory ^[7], it holds that

Thus we have

We can get $\mathcal{M}\left\{\sup\limits_{k > 0}|Z_{k}-\hat{Z}_{k}|\geq z-\tilde{z}\right\}\rightarrow0$ as $\sup\limits_{r\in[-\tau, 0]}|Z_{r}-\hat{Z}_{r}|\rightarrow0.$ Then we have

for any $\tilde{z} < z$. Letting $\tilde{z}\rightarrow z^{-}$, we have

By (3.28) and (3.29), we obtain

According to Definition 3, the uncertain differential equation with time-dependent delay (1.1) is stable in distribution.

Theorem 7. If the uncertain differential equation with time-dependent delay (1.1) is stable in p-th moment $(0 < p < +\infty)$, then it is stable in distribution.

Proof If the uncertain differential equation with time-dependent delay (1.1) is stable in p-th moment $(0 < p < +\infty)$, by Theorem 4, the uncertain differential equation with time-dependent delay (1.1) is stable in measure. And by Theorem 6, we obtain the uncertain differential equation with time-dependent delay (1.1) is stable in distribution.

Remark 2. Theorem 4, Theorem 5, Theorem 6, and Theorem 7 give the sufficient condition but not the necessary condition for the comparison of stability.

4.   Conclusions

The main goal of the current study is to propose new stabilities called almost sure stability, stability in $p$-th moment and stability in distribution for uncertain differential equations with time-dependent delay. Meanwhile, the sufficient conditions of these theorems are also provided. Some examples and figures are given to illustrate the stability furthermore. The applications about uncertain differential equations with time-dependent delay will be the focus of our future research.

Acknowledgments

The research was supported by Postgraduate Research & Practice Innovation Program of Jiangsu Province (KYCX20_0170) and National Natural Science Foundation of China (61374183).

Conflict of interest

The authors declare that they have no competing interests.