Dynamic analysis of reaction-diffusion dual carbon model considering economic development in China

1.   Introduction

Since the industrial revolution, the large-scale use of fossil energy, such as coal and oil, has consumed millions of years of stored carbon in an extremely short period of time, and released greenhouse gases into the atmosphere, such as carbon dioxide. It destroys the balance of ecosystem, such as melting glaciers, rising sea levels, forest fires and so on. Since the 1990s, many countries have paid more and more attention to environmental issues in the world, and have started to strongly promote and use all kinds of clean energy. In 2015, the nations of the world reached an agreement on global climate governance at the Paris Climate Conference and signed the Paris Agreement the following year. At the United Nations Climate Conference in 2020, many countries and regions put forward the ambitious target of "carbon peak and carbon neutrality" (in short dual carbon). At the 26th Conference of the Parties (COP26) of the United Nations Framework Convention on Climate Change (UNFCCC) on November 2021, 15 major methane emitters signed the Global Methane Emission Commitment to promote clean fuels and technologies. In April 2021, Mr Biden announced that the United States government would achieve its commitment on energy conservation and emission reduction, and declared that the U. S. would reduce its greenhouse gases emission and achieve carbon neutrality by 2050 in his opening speech at the Leaders' Climate Summit. European Union countries will be carbon neutral by 2050, while Germany and France have set their own target of 2045 ^[1]. Meanwhile, China promised to achieve carbon peak by 2030 and carbon neutrality by 2060 at the UN General Assembly.

Carbon peak and carbon neutrality are defined, as follows: carbon peak, refers to the point at which carbon emission stops growing and reaches a peak, and then gradually declines. The peak value of carbon is an inflection point of carbon emission from increase to decline, marking the decoupling of carbon emission from economic development. Carbon neutrality, refers to the total amount of greenhouse gases emission directly or indirectly, which is produced by enterprises, organizations or individuals for a time, and through afforestation, energy conservation and emission reduction, offsetting the emission of carbon and other greenhouse gases produced by themselves, so as to achieve "net zero emission" of carbon dioxide.

In the study of biological models, some scholars used various kinds of ordinary differential equations ^[2], partial differential equations ^[3], functional partial differential equations ^[4] to describe some actual problems, and gave some practical suggestions through relevant theoretical analysis and researches. For example, in ^[5,6,7], authors proposed a model with diffusion ^[5], spatial diffusion ^[6] and delay ^[7], respectively, and the results were meaningful to some practical question.

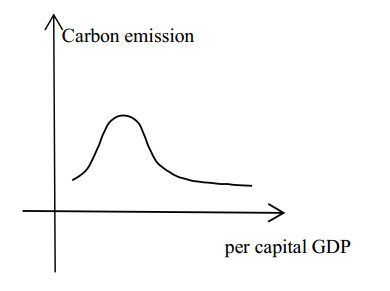
EKC (Environment Kuznets Curve) (see Figure 1) describes a phenomenon that the value of carbon emission is correspondingly light when a country has a low level of economic development. However, with the increasing of per capita income, the value of carbon emission increases from low to high, and the degree of environmental deterioration increases with the growth of economic, when economic development reaches a certain level, that is to say, the value of carbon emission will reach a critical point, or "inflection point". With the further increasing of per capita income, the degree of pollution of the environment gradually decreases and environmental quality is improved gradually.

In addition, many scholars have studied on the influencing factors of carbon emission, calculation of carbon emission, the relationship between economy and carbon emission (i.e., EKC), and the simulations and predictions of carbon peak under different paths. There are many factors affecting carbon emission, such as per capita GDP, number of means of transportation, total population, economic structure, average annual household income and so on, see ^[8,9,10]. However, economic development have a much greater impact on carbon emission in ^[9]. Wang et al. ^[10] concluded that the continuous growth of economic was the leading factor of carbon emission in China. In ^[11,12], scholars have studied the relationship between economic development and carbon emission and pointed out that there is an inverted U-ship curvilinear relationship (i.e., Kuznets inverted U-curve) between regional carbon emission and economic development level to some extent. Further, Apergis ^[13] verified the authenticity of EKC between economic development and carbon emission by using Generalized Method of Moment (GMM) in North America. In ^[14], Wei et al. proposed a carbon emission-absorption model with time delay considering the impact of energy and economy in China. They concluded that China can achieve peak in 2027 and if China wants to achieve carbon neutrality before 2060, it requires to make corresponding policy adjustments. Fei et al. ^[15] used the Analytic Hierarchy Process (in short AHP) to determine the important influencing factor of carbon emission, and established a delayed differential equation model of carbon emission-absorption considering the influence of urbanization in China. Results shown that China can achieve carbon peak by 2030 and carbon neutrality can be achieved by 2060.

The motivation of this paper is as follows. Climate change is an urgent problem. General Secretary Xi Jinping stressed that achieving carbon peak and carbon neutrality is a solemn promise which China has made to the world at the Central Group Learning Session. As the world's largest carbon emitter, China has a long way to achieve carbon neutrality. It is a responsibility, a mission, and an inevitable choice for China to achieve a harmonious coexistence between man and nature, and a green economical and social transformation. This topic has a new research significance. To better describe this fact, we have the following considerations.

1) We select economic development factors as the most important factors affecting carbon emission in China.

2) The gas freely diffuses in a certain space region and remains unchanged on the boundary, so the reaction-diffusion term is added to the ordinary differential model and we select the Dirichlet boundary conditions.

The paper proceeds as follows: in Section 2, we establish a reaction-diffusion dual carbon model with Dirichlet boundary condition under the influence of economic development in China. We analyse the existence and stability of the equilibrium and the existence of bifurcations in Section 3. In Section 4, we analyse data to select appropriate parameters for numerical simulations to support our results. Conclusions are given in Section 5.

2.   Mathematical modeling

To achieve the aim associated with "dual carbon" on schedule, it is necessary to make the total carbon emission less than or equal to carbon absorption. Therefore, we establish a relationship expression which can describe the relationship between carbon emission and carbon absorption under the influence of China's economic development. Since there is a nonlinear relationship between carbon emission and absorption, we establish a differential equation model with reaction-diffusion between carbon emission and carbon absorption, as follows.

where $x\in\Omega = [0, \pi]$, the domain $\Omega$ with smooth boundary $\partial \Omega$, and time $t\geq0$. Among them, $u(x, t)$ and $v(x, t)$ stand for the density of carbon emission and carbon absorption at a certain position $x$ and time $t$, respectively; $d_1$ and $d_2$ represent the diffusion coefficient of carbon dioxide at high and low concentration, respectively; $r_1$ and $r_2$ represent the average growth rate per year of carbon emission and absorption, respectively; $n_1$ and $n_2$ represent the maximum density of carbon that the environment can accommodate and the maximum density of carbon that the environment can absorb (i.e., the environmental capacity) in per unit of area, respectively; $s_1$ and $s_2$ (dimensionless parameters) represent the influence coefficient of carbon emission on carbon absorption and carbon absorption on carbon emission, respectively.

First, we analyse the influencing factors of carbon emission and carbon absorption. Among many influencing factors, economic development is selected as the main influencing factor of carbon emission. Therefore, economic development is mainly considered in this paper ^[9]. Under the influence of economic development, carbon emission presents an inverted U-shape curvilinear (i.e., EKC, see Figure 1), and economic development has a certain threshold. Considering expression:

$f(u)$ is a function of economic development which has an impact on the change rate of carbon emission, where positive constant $a$ describes the threshold value of carbon emission, $u$ stands for carbon emission, and $c_1$ represents the influence coefficient of economic development on carbon emission and it is a dimensionless parameter. Firstly, in certain spare $x\in\Omega = [0, \pi]$, when $u < a$, $f(u) > 0$, $u > a$, $f(u) < 0$. Equation (2.2) satisfies what the EKC describes. Thus, $f(u) = -\frac{c_1}{u-a}$ holds in the first equation of (2.1). Second, we determine the influence factors of carbon absorption. In China more than 960 square kilometers of land, with the farmers of arable land and grassland area of restrictions, a large area of afforestation in a short time to increase carbon sequestration is limited, so we think that carbon absorption is not affected by any factors. To sum up, only the impact of economic development on carbon emission is considered in this model.

After that, considering the diffusion property of the gases, it's reasonable and practical to consider the effect of diffusion in Eq (2.1). As for the selection of Dirichlet boundary condition, the concentration of gases at the boundary can be regarded as a constant and remains unchanged in a fixed area, due to its spatial diffusion property. Finally, a model with reaction-diffusion term and Dirichlet boundary condition is given as follows:

where the meaning of $u(x, t)$, $v(x, t)$ and the above parameters are as described in Eq (2.1).

3.   Existence and stability analysis

In this section, we will analyse the existence and stability of equilibrium and the existence conditions of Turing bifurcation and Hopf bifurcation will be given.

3.1.   Existence and stability of equilibrium

First, we consider the existence and stability of equilibrium. The equilibrium of system (2.3) satisfies the following equations:

In fact, according to the actual meaning of $c_1$, we have $c_1 > 0$, so the first equation of Eq (3.1) has at least one positive solution $u^{*}$, and the second equation of Eq (3.1) also has at least positive solution $v^{*}$ when $1-\frac{s_2}{n_1} u^{*} > 0$. Thereby, the system (2.3) must have one positive equilibrium, if $1-\frac{s_2}{n_1} u^{*} > 0$ is true, and denote $E_{*} = (u^{*}, v^{*})$. Furthermore, we can obtain the following lemma by Cardan's formula:

Lemma 3.1. Assume that $1-\frac{s_2}{n_1} u^{*} > 0$ holds, considering system (2.3),

(1) if $\Delta > 0$, system (2.3) has only one equilibrium: $E_1 = m_1+m_2-\frac{f_2}{3f_1}$;

(2) if $\Delta < 0$, system (2.3) has three equilibria: $E_1 = m_1+m_2-\frac{f_2}{3f_1}$, $E_2 = xm_1+x^{2}m_2-\frac{f_2}{3f_1}$, $E_3 = x^{2}m_1+xm_2-\frac{f_2}{3f_1}$;

(3) if $\Delta = 0$, and $p = q = 0$, system (2.3) has only one equilibrium: $E_1 = -\frac{f_2}{3f_1}$;

(4) if $\Delta = 0$, $p\neq0$ and $q\neq0$, system (2.3) has two equilibria: $E_1 = 2\sqrt[3]{-\frac{q}{2}}-\frac{f_2}{3f_1}$ and $E_2 = x\sqrt[3]{-\frac{q}{2}}+x^{2}\sqrt[3]{-\frac{q}{2}}-\frac{f_2}{3f_1}$;

with

Since we select Dirichlet boundary condition, and suppose that: $\varphi = u^{*}$, $\psi = v^{*}$, the system (2.3) is written as:

For the sake of convenience, by applying the following scalings :

denote $\frac{r_2}{r_1} = r$, and we transform the system (3.2) into $E_0 = (0, 0)$. Let $\tilde{u} = u-u^{*}, \; \tilde{v} = v-v^{*}.$ For the sake of convenience, we still denote $\tilde{u}$ and $\tilde{v}$ by $u$ and $v$, respectively. Thus, the system (3.2) is written as:

among them, $x\in\Omega = [0, \pi]$, that is to say, $\Omega$ is a finite interval in $\mathbb{R}$.

Next, the stability of the equilibrium $E_0 = (0, 0)$ of system (3.3) is analysed. The characteristic equation of the system (3.3) at $E_0 = (0, 0)$ is:

where

Firstly, we analyse the value of $T_n$ (refer to the analysis method of reference ^[16]). Let $T_n = 0$, we have

where $b^{*} = \frac{c_1 (2 u^{*}-a)}{u^{*}(u^{*}-a)^{2}}-u^{*}-r v^{*}.$

Remark 3.1. Assume that $b^{*} > 0$ holds, we have

(1) $\beta_{n}(d_1)$ is decreasing as $d_1$ increases in $(0, +\infty)$;

(2) $\lim_{d_1\rightarrow 0^{+}}\beta_{n}(d_1) = b^{*},$ $\lim_{d_1\rightarrow +\infty}\beta_{n}(d_1) = -\infty,$ and $\beta_{n}(b^{*}) = 0$;

(3) $\beta_{n}(d_1) > \beta_{n+1}(d_1)$ for $d_1 > 0.$

We find that $\beta_{n}(d_1)$ attains its maximum at $n = 1$ by Remark 3.1.

Lemma 3.2. $T_n > 0$ for any $n\in \mathrm{N^{+}}$ if and if only if one of the following conditions are satisfied,

(1) $d_1 > b^{*}$ and $d_2 > 0$;

(2) $0 < d_1 < b^{*}$ and $d_2 > \beta_{1}(d_1)$.

Next, we discuss the value of $D_n$ (refer to analysis method of reference ^[17]). $D_n$ is a quadratic function of $n^{2}$ with symmetry $n_{0}^{2}$, where

If $n_{0}^{2} > 1$, $D_n$ attains its minimum at $n_{0}$,

Denote $\theta = \frac{d_2}{d_1} > 0,$ and $\Lambda(d_1, d_2) = -4 d_1 d_2\cdot minD_n|_{n_{0}}.$ Let $\Lambda(d_1, d_2) = 0$, we obtain that

We consider the symmetry axis of Eq (3.8)

and the discriminant of Eq (3.8)

When $\theta^{*} > 0$ and $\Delta > 0$, there are two positive roots $\theta_1$ and $\theta_2$ in Eq (3.8), where

If $n_0^{2}\leq1$, $D_n$ is increasing in $n = 1, 2, 3, \ldots$, and attains its minimum $D_1$ at $n = 1$, where

By the above analysis, we have the following lemma.

Lemma 3.3. $D_n > 0$ for $n\in {\bf{N^{+}}}$ if and if only if one of the following conditions are satisfied,

(1) $n_0^{2} > 1$, $\theta_2d_1 < d_2 < \theta_1d_1$;

(2) $n_0^{2}\leq1$, $D_1 > 0.$

Theorem 3.1. When Lemma 3.2 and Lemma 3.3 are satisfied at the same time, namely $T_n > 0$ and $D_n > 0$, the characteristic roots of Eq (3.4) have a negative real part, then the equilibrium $E_0 = (0, 0)$ of system (3.3) is locally asymptotically stable, where $\beta_n(d_1)$, $n_0^{2}$, $\theta^{*}$, $\theta_1$ and $\theta_2$, $D_1$ are defined by (3.5), (3.6), (3.9), (3.11), (3.12), respectively.

3.2.   The existence of bifurcation

In this section, we discuss the existence conditions of Turing and Hopf bifurcations. This paper mainly studies the influence of economic development factor on carbon emission and carbon absorption. Therefore, parameter $c_1$ is selected as the bifurcation parameter in the following.

Remark 3.2. Since the equilibrium of system (3.3) is implicit and the existence conditions of bifurcations can not be written directly, thus, we give expressions instead of formula in following analysis.

We analyse Turing bifurcation firstly. If the eigenvalue $\lambda_n = 0$ is a root of Eq (3.4), then Turing bifurcation of system (3.3) will occur, that is to say, $D_n = 0$ always holds, and it is a curve function about $n^{2}$. Denote

then $D_n = 0$ is converted to:

where $h_2 > 0$. Denote $\triangle = h_1^{2}-4h_0h_2,$ under the condition of discriminant $\triangle > 0$, the value of $n^{2}$ is obtained by solving Eq (3.13) as follows:

The discriminant $\triangle = 0$ is the critical condition for Turing bifurcation to occur. Then critical value of $n$ (see Eq (3.15)) is obtained:

Therefore, we have the following theorem about Turing bifurcation.

Theorem 3.2. If $\lambda_n = 0$ is the root of the Eq (3.4) of the system (3.3), then $D_n = 0$ is true, that is to say, $n$ satisfies Eq (3.15), the system (3.3) exists Turing bifurcation. Otherwise, $\lambda_n = 0$ is not the root of the Eq (3.4), there is no Turing bifurcation in the system (3.3).

Now, we start to analyse the existence of Hopf bifurcation. If the eigenvalue $\lambda_n$ is a pair of pure imaginary roots of characteristic Eq (3.4), then system (3.3) generates Hopf bifurcation if and only if:

We might assume $\lambda_n = \pm \mathrm{i}w_n$ $(w_n > 0)$ is a pair of pure imaginary roots. Substituting them into the Eq (3.4), we have

Separating the real and imaginary parts, we obtain

Because $w_n > 0$, the following equations is true.

$T_n$ and $D_n$ see Eq (3.4).

Denote $\lambda_n(c_1) = \alpha(c_1)+\mathrm{i}\beta(c_1)$, where $\alpha(c_1)$ and $\beta(c_1)$ satisfy: $\alpha(c_1^{n_0}) = 0$, $\beta(c_1^{n_0}) = w_{n_0}$, $w_{n_0}$ satisfies Eq (3.15). Taking the derivative of $\lambda_n$ at both ends of Eq (3.4), the transversality condition $\mathrm{Re}(\frac{d\lambda_n}{dc_1}|_{c_1 = c_1^{n_0}})$ can be obtained. And we suppose that

therefore we have the following theorem about Hopf bifurcation.

Theorem 3.3. When $c_1$ satisfies Eq (3.19), and assumption $(H)$ holds, the Eq (3.4) has a pair of pure imaginary roots $\pm \mathrm{i}w_n$, and the system (3.3) undergoes Hopf bifurcation at the equilibrium $E_0 = (0, 0)$.

4.   Numerical simulations

In this section, we will analyse the data and select the appropriate parameters for numerical simulations to verify the stability of equilibrium and obtain some conclusions. Moreover, some suggestions will be given to promote the realization of dual carbon goal.

4.1.   Data analysis

In this section, we select the data of carbon emission and carbon absorption during a period of time in China to forecast and analyse the value of parameters.

1) Coefficient of economic development $a$.

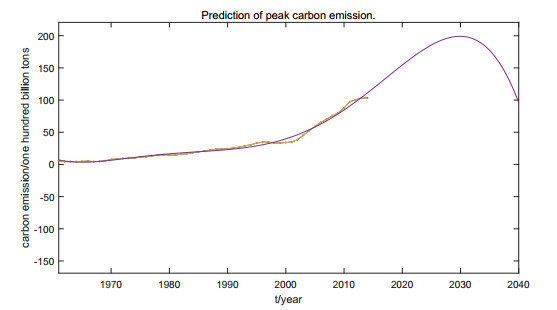
China's economy has a rapid growth currently, in order to select a more reasonable threshold of carbon emission, we select the carbon emission data from 1961 to 2014 in a long period to simulate and predict value of $a$ by Matlab software. According to China's data released by the Carbon Dioxide Information Analysis Center of the Environmental Science Division of oak Ridge National Laboratory in Tennessee (in short ORNL, website: https://www.ornl.gov/), the predictive value of carbon emission between 2014 and 2035 is obtained by polynomial fitting (see Figure 2, where the red scatter is the true value of annual carbon emission, and the purple solid line is the predicted value of carbon emission by polynomial fitting).

Remark 4.1. According to Figure 2, China will achieve carbon peak in 2030 with a peak of 199.1 one hundred million tons. Due to the influence of some factors, such as the policy constraints of energy conservation and emission reduction, industrial structure adjustment and so on, carbon emission will have a downward trend after the peak of carbon emission in 2030, but the overall carbon emission will not be reduced to zero, since the daily production and life of human beings will produce carbon. Therefore, in the fitting result (see purple curve of Figure 2), the part before 2035 is more in line with China's current development situation. For the above reasons, we choose $a = 199.1$ (one hundred million tons) as the threshold of carbon emission.

2) Carbon emission and carbon absorption growth rate per year $r_1$ and $r_2$.

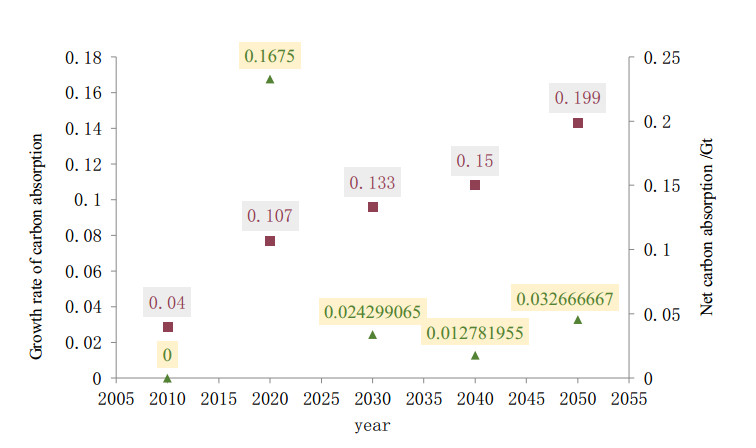
Through analysing the global annual carbon emission and absorption data from official website and calculating growth rate, we obtain Table 1 (from: https://www.icos-cp.eu/science-and-impact/global-carbon-budget/2021) and Figure 3 (from: https://www.docin.com/p-298870009.html). In Table 1, through calculating average value, we obtain the average annual carbon emission is 0.049560279 (see Table 1). In Figure 3, we can obtain that carbon absorption growth rate per year of China is 0.00593 (see the green triangle in Figure 3). Thus, we choose $r_1 = 0.04956$ and $r_2 = 0.00593$, so $r = \frac{r_2}{r_1} = 0.11965$ (The growth rate $x_i$ is calculated as follows: $x_{i+1} = \frac{u_{i+1}-u_{i}}{u_{i}}$, where $x_{i+1}$ stands for growth rate in $i+1$ year, and $u_{i}$ represent carbon emission/ absorption in $i$ year).

3) Other dimensionless parameters.

We select $d_1 = 0.06$, $d_2 = 0.04$. Reason is as follows. Carbon emission will increase the carbon concentration in the region, absorption will decrease the carbon concentration. While the concentration of carbon dioxide and other gases is high, the diffusion rate is high; when the concentration is low, the diffusion rate is low. Therefore, the diffusion coefficient of carbon emission and carbon absorption is $d_1 = 0.06$, $d_2 = 0.04$, respectively. As carbon emission is increasing, carbon absorption will also increase, and the improvement of carbon absorption capacity will directly increase carbon emission, and we generally think $s_1\in[0, 1]$ and $s_2\in[0, 1]$, so we rightly choose $s_1 = 0.2$, $s_2 = 0.22$. In addiction, we generally think dimensionless parameter $c_1\in[0, 1]$.

In summary, we select $d_1 = 0.06$, $d_2 = 0.04$, $s_1 = 0.2$, $s_2 = 0.22$, $r_1 = 0.04956$ and $r_2 = 0.00593$, $a = 199.1$, $c_1 = 0.8$.

4.2.   Numerical simulations

In this section, we will simulate to verify the correctness of theoretical analysis, and give some suggestions to promote the realization of the dual carbon target in China.

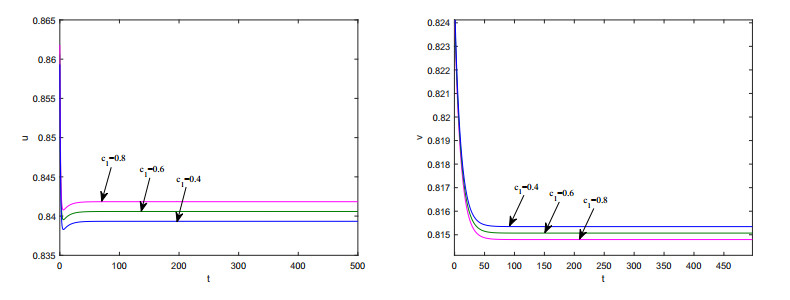
Firstly, we will simulate the system (3.3) without reaction-diffusion, namely $d_1 = d_2 = 0$. Selecting $c_1 = 0.8,$ there is a positive equilibrium $E_* = (0.84183, 0.8148)$ of system (3.2). Let $c_1 = 0.6$ and $0.4$, respectively, positive equilibria are $E_* = (0.84059, 0.81507)$ and $(0.83933, 0.81535)$, respectively. We can find that the equilibrium $E_*$ is locally asymptotically stable by Theorem 3.1 (see Figure 4).

Remark 4.2. Although we can see the change is small in the Figure 4, there is magnitude level, and the multiplied number is huge. Therefore, the impact of economic adjustment on the realization of dual carbon goal can be said to be great, which also verifies that we chose economic factor as the most important factor affecting carbon emission. However, the development of a country cannot be separated from economic development, only regulating economic development is not feasible with the double carbon target. Therefore, we will achieve carbon neutrality through making other adjustment in the long term.

Since $r_1$, $r_2$, $n_1$, $n_2$ and $a$ are derived from predictive analysis of true data and the controllable parameters are only $c_1$ and $s_1$, $s_2$, therefore, we analyze the stability of the equilibrium and the existence of bifurcation of system (3.2) as $c_1$, $s_1$ and $s_2$ change.

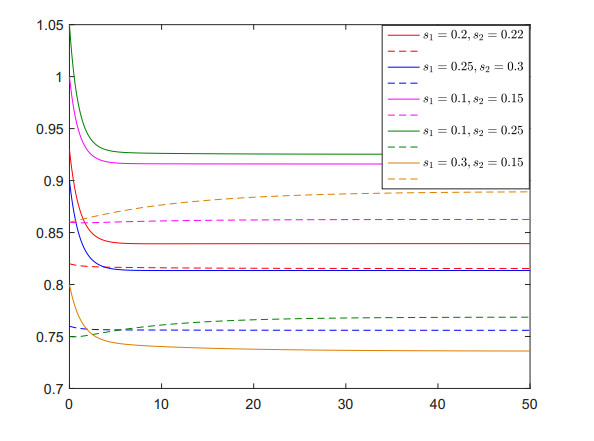
Now, we select four sets of $s_1$, $s_2$ under $c_1 = 0.8$, as follows,

By Theorem 3.1, the equilibrium under four sets of parameters is all locally asymptotically stable, when there is no reaction-diffusion in dual carbon model, see Figure 5, where the solid lines represents the value of carbon emission (namely $u$) and the dotted lines stands for the value of carbon absorption (namely $v$). Similarly, in reaction-diffusion model, the above equilibrium is also locally asymptotically stable and there is no Hopf or Turing bifurcation. The phenomenon is extremely similar, thus, we take the model without reaction-diffusion to analyze the value of equilibrium associated with background in the following analysis.

By comparing the value of solid and dotted lines of different colors in Figure 5, it is found that when $s_1$ is increased and $s_2$ is decreased at the same time, the equilibrium of system (3.2) is locally asymptotically stable and the value of carbon emission is always lower than the value of carbon absorption (see the orange solid and dotted lines in Figure 5), we can achieve carbon neutrality.

Next, we change $c_1 = 0.4$ and $c_1 = 0.6$ under Group $A_1$, Group $A_2$, Group $A_3$ and Group $A_4$, respectively. The equilibrium still is locally asymptotically stable (see Figure 6). By comparing the values of solid and dotted lines (except the orange lines) in Figures 5 and 6 and the value of equilibrium in Table 2, we can see that when the level of economic development is relatively low (namely $c_1 = 0.4$), the value of carbon emission and carbon absorption are at a relatively low level; when the level of economic development is relatively high (namely $c_1 = 0.8$), the value of carbon emission and carbon absorption are at a relatively high level. However, when $c_1$ varies, and the value of carbon emission is always higher than the value of carbon absorption. We cannot achieve carbon neutrality except increasing $s_1$ and decreasing $s_2$.

Remark 4.3. When we give priority to adjusting the factors that can directly affect carbon emission and carbon absorption, for example, afforestation increases forest carbon sink and vigorously promoting clean energy is aim to reduce carbon emission, carbon neutrality can be achieved on schedule and there could even be carbon trading.

Next, we consider system (3.3) with reaction-diffusion. Based on the above parameters: $d_1 = 0.06$, $d_2 = 0.04$; $s_1 = 0.2$, $s_2 = 0.22$; $r = 0.11965$, $c_1 = 0.8$, there is a positive equilibrium $E_* = (0.84183, 0.8148)$ of system (3.2) (i.e., $E_0 = (0, 0)$ of system (3.3)). By calculation, the parameters satisfy Theorem 3.1, the equilibrium $E_* = (0.84183, 0.8148)$ of system (3.2) is locally asymptotically stable (see Figure 7). By calculation, we can attain that there is no $n$ satisfy Eq (3.15). Therefore, there is no Turing bifurcation. By Eq (3.4), we have $T_n = 0.0930\neq0$, which is in contradictory with Eq (3.19). So there is no Hopf bifurcation.

Remark 4.4. Comparing the above analysis, we have the following results.

(1) When $c_1$ is in a certain range, the positive equilibrium $E_*$ of the system (3.2) is always locally asymptotically stable, that is to say, the carbon emission and absorption can remain stable after a period of time. But the carbon emission is still greater than the carbon absorption. There is a certain distance to achieve carbon neutrality.

(2) It is difficult to only adjust economic development to achieve carbon neutrality. To achieve carbon neutrality, we should improve technical level or make some policy restrictions in some areas such as energy use, forest management, forest carbon sink and so on.

Many scholars have made a mathematical study on carbon emission and absorption, and discussed whether China can achieve the dual carbon goal. In ^[18], Li et al. incorporated the extreme learning machine (ELM) network, the Aquila optimizer (AO) technique, and the Elastic Net (EN) regression method, and established a prediction model with the aim of exploring the net-zero emission pathways. Simulation results shown that the total carbon emission will peaked at 11,441 million tons in 2029, and China has the potential to achieve net-zero $CO_2$ emission by 2060 under the combined effects of reducing emission and increasing forest carbon sink. Yang and Liu ^[19] applied to the grey relation analysis (GRA) to filter the elements influencing carbon emission. They proposed a hybrid prediction with Elman Neural Network (ENN) and Sparrow Search Algorithm (SSA). Through analysing, they obtained that China has a good chance of carbon peaking from 2028 to 2030, and the value of peak is 11,568.6–12,330.5 Mt, while only one scenario can achieve carbon neutraly in 2060.

In references ^{[14,15,18,19]}, scholars have drew consistent conclusions from different research perspectives and methods, which confirmed that China can achieve carbon peak before 2030 and it requires us to make some changes to achieve carbon neutral before 2060. Combined with the above results, it can be shown that the research results of this paper are consistent with the actual situations. Therefore, in view of China's development pattern, we have the following suggestions.

1) On energy use, in order to improve the efficiency of the use of fossil energy, low-carbon technology innovation should be carried out to achieve low carbon emission of high-carbon energy by technical means. On the other hand, it is necessary to realize the transformation in energy use to vigorously promote new energy and reduce the use of high-carbon energy ^[20].

2) In terms of forest management and afforestation, forest is the largest carbon reservoir in terrestrial ecosystems and plays an important role in reducing the concentration of greenhouse gases in the atmosphere and mitigating global warming. Therefore, we need to protect forests. Furthermore, the species of trees has an effect on forest carbon sink. Our country forest carbon sink mainly is concentrated in seven types of tree, spruce forest, fir, larch, oak, birch, sclerophyllous forest and mixed broad-leaved mixed forest. Therefore, increasing the number of the above species of trees will extremely improve the forest carbon sink capacity ^[21].

3) In terms of carbon capture and storage technologies, system optimization technologies such as the internet and artificial intelligence should be closely linked to maximize the use and exploitation of carbon capture and storage technologies, so as to make breakthroughs in low-carbon emission technologies in agriculture, construction industry, forest grassland and other industries ^[22].

4) In the Carbon Emission Trade Exchange (in short CCETE), CCETE has not been fully developed, effective measures should be taken to promote the vitality of China's carbon trading market. The carbon trading price should be regulated to adapt to our economy situation. For example, it is necessary to gradually increase the carbon trading price to reduce the use of carbon emission. In addition, the government should strictly regulate the use of carbon emission permits, and ensure the normal work of the market ^[23].

5) In terms of policy, we should strictly regulate the standards and requirements of tree cutting. Governments should formulate disciplinary measures for illegal cutting and other actions to give basic guarantee in policy.

5.   Conclusions

In this paper, in the view of China's development, we established a dual carbon reaction-diffusion model with Dirichlet boundary condition under the influence of economic development. The existence and stability of positive equilibrium were analyzed, and the existence conditions of bifurcations were given. Finally, through data analysis, a set of appropriate parameters by data analysis were selected for numerical simulations to support theory analysis. Through the study of this paper, we provided some practical suggestions for China's carbon development.

1) Through data analysis, we obtain that China will achieve carbon peak in 2030 and carbon emission will reduce year by year after that.

2) When we select parameter $c_1$ that satisfies certain conditions (Theorem 3.1), carbon emission and absorption can reach a balanced state (but it is not carbon neutrality).

3) When only changing economic development parameter, the positive equilibrium of system (3.3) always is locally asymptotically stable, but the carbon emission is greater than carbon absorption.

4) It is difficult to adjust only economic development to reduce carbon emission or increase carbon absorption, we should make efforts in other aspects.

Acknowledgments

This study was funded by Fundamental Research Funds for the Central Universities of China (Grant No. 2572022DJ06).

Conflict of interest

The authors declare there is no conflict of interest.