The Cauchy problem for general nonlinear wave equations with doubly dispersive

1.   Introduction

The focus of this work is a general nonlinear doubly dispersive wave equation:

associated with the following initial profile:

Hereafter the nonlinearity $h(u)$ indicates the following:

Both $\mathcal{L}$ and $\mathcal{B}$ are linear pseudo-differential operators that can be respectively written as follows:

and

whose symbols are $l(\zeta)$ and $b(\zeta)$ respectively satisfying

and

for all $\zeta \in \mathbb{R}$. Henceforth $\mathcal{F}$ denotes the Fourier transform and $\mathcal{F}^{-1}$ denotes the inverse Fourier transform.

We note that Equation (1.1) arose from an integral-type non-locality of elastic materials (see ^[1,2] and the references therein for more information about its physical background), and some Boussinesq-type equations can be covered by Equation (1.1). For example, with the substitutions $\mathcal{L} = -a\partial _{x}^2+1$, $\mathcal{B} = I$ and $h(u) = \alpha u^2$, (1.1) becomes the classical Boussinesq equation:

for shallow water (see ^[3]). Liu in ^[4] demonstrated that the traveling wave for the corresponding (1.7) may be stable or unstable and established sharp conditions to support this. Using the potential well method, the qualitative behavior for (1.7) with $h(u) = u^2$ was derived in ^[5]. Also Equation (1.1) can be reduced to the improved fourth-order Boussinesq equation:

with the selections $\mathcal{B} = (1-\partial^2_x)^{-1}, \mathcal{L} = (1-\partial^2_x)^{-1}$ and $h(u) = u^2$ in Equation (1.1). Physically, Equation (1.8) can be used to describe the role of inertia in the one-dimensional lateral dynamics of the elastic rod ^[6]. Besides that, a class of fourth-order double-dispersion Boussinesq-type equations with terms $u_{xxxx}$ and $u_{xxtt}$ given by

can also be derived by setting $\mathcal{B} = (1-\partial^2_x)^{-1}$ and $\mathcal{L} = I$ in Equation (1.1). It is well known that Equation (1.9) can yield longitudinal strain waves in a nonlinearly elastic rod ^[6] and has many interesting results involving the initial data. For example, Liu and Xu in ^[7] demonstrated the influence of the nonlinearity as $h(u) = |u|^p$ along with the initial data on dynamical behavior for Equation (1.9) with sub-critical and critical initial energy. Further, a high-order Boussinesq equation written as

can also be included by setting $\mathcal{B} = (1-\partial^2_x+\partial^4_x)^{-1}$, $\mathcal{L} = (1-\partial^2_x+\partial^4_x)^{-1}(1-\partial^2_x)$ and $h(u) = |u|^p$ in (1.1), which can be applied to simulate the surface tension of water waves and the long-time behavior of small initial data. And the nonlinear scattering for Equation (1.10) when $h(u) = u^p$ $(p > 1)$ as $u \rightarrow 0$ was established in ^[8].

From the above statements, we see that the general form, i.e., (1.1), can represent many important interesting mathematical physics models reflecting the meaningful phenomenon from the real world, and that the results on such models will deepen our knowledge regarding these physical problems. Hence, the main goal of our work is to deal with how the structure of the wave Equation (1.1), especially the dispersive effect induced by its pseudo-differential operator $\mathcal{B}$ and $\mathcal{L}$, along with the initial condition (1.2), affects the dynamical characteristics of the corresponding solution. In fact, because the pseudo-differential operators $\mathcal{B}$ and $\mathcal{L}$ in Equation (1.1) are identical and become a convolution integral operator of the form

the considered Equation (1.1) reduces to

which was first considered in ^[2] with $\beta(x) = \mathcal{F}^{-1}(b(\zeta))$ and $b(\zeta)$ satisfying (1.6). And the existence of the local solution and global positive-definite energy solution as well as negative initial energy finite time blowup were constructed in ^[2]. After that, some attention is paid to the case of $\mathcal{B}\neq\mathcal{L}$ in Equation (1.1) given that $\mathcal{B}$ behaves like case (1.11) with $\beta(x) = \mathcal{F}^{-1}(b(\zeta))$ and $b(\zeta)$ satisfies (1.6) or $\mathcal{B} = I$, whereas the symbol $l(\zeta)$ of $\mathcal{L}$ is subject to (1.5).

For the case that the symbol $l(\zeta)$ of $\mathcal{L}$ satisfies (1.5) and $\mathcal{B} = I$, the considered Equation (1.1) becomes

It is noted that for some sufficiently smooth nonlinearities $h(u)$, Babaoglu et al., in ^[1], established the local existence of the solution, the existence of the global positive-definite energy solution, and the global non-existence of the sufficiently negative initial energy solution. Then, some improvements of ^[1] were established in ^[9] and ^[10] by considering the non-positive-definite energy case due to the nonlinearities. For the nonlinearity $h(u) = -|u|^{p-1}u$ as one case of (H), the dynamical behavior for low initial energy was dealt with in ^[10] with the symbol $b(\zeta)$ of $\mathcal{B}$ as follows

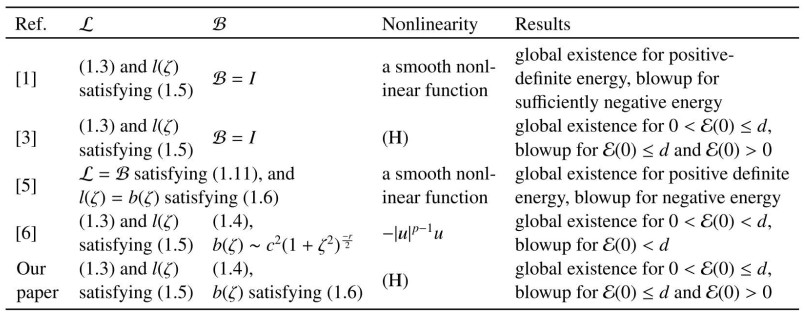
which also satisfies (1.6) considered in our work. In fact we can see that some special cases such as $b(\zeta) = c_{3}^2(1+\zeta^2)^\frac{-\tilde{r}}{2}$ with $\tilde{r} > r\ge 0$ for all $\zeta\in \mathbb{R}$ can be included in (1.6) but not (1.12). The above analysis means that some results obtained in ^[9] and ^[10] are special cases of our work. In fact, although we carefully introduced these established related results above, it is still not easy to distinguish the differences between them from the results of the present paper. Hence we use Table 1 to make it clear.

Hence our work considers the generalized case that the pseudo-differential operators $\mathcal{B}$ and $\mathcal{L}$ satisfy Equations (1.5) and (1.6), respectively. Some typical nonlinear terms like $|h(u)| = \alpha |u|^p$ with $\alpha > 0$ shown in (H) are considered, and the qualitative behavior for non-positive-definite energy (sub-critical, critical and super-critical levels) is derived. Our work also improves some corresponding results for those special cases.

The organization of this paper is as follows. Section 2 gives some preliminaries. The global existence and finite time blowup for $\mathcal{E}(0) < d$ and $\mathcal{E}(0) = d$ are proved in Section 3 and Section 4, respectively. Section 5 proves the finite time blowup for $\mathcal{E}(0) > 0$.

2.   Setup and notations

Throughout this paper,

$\|u\|$ and $(u, v)$ respectively represent the norm of $H^s: = H^s(\mathbb{R})$, the $L^2$ norm and the inner product in $L^2$. Further, we define $\mathcal{K} = \mathcal{L}^\frac{1}{2}$ with $\kappa(\zeta) = \sqrt{l(\zeta)}$ and $\Lambda^{-\alpha}\omega = \mathcal{F}^{-1}[|\zeta|^{-\alpha}\mathcal{F}\omega]$.

Some preliminaries are first introduced to help us consider the well-posedness for the considered problem.

Definition 2.1 (Weak solution) A function $u(t)\in C^1(0, T;H^{\frac{\rho}{2}+\frac{r}{2}})$ with $u_t\in C(0, T;H^{\frac{r}{2}-1})$ is called a weak solution to (1.1) and (1.2) if $u_0\in H^{\frac{\rho}{2}+\frac{r}{2}}$, $u_1\in H^{\frac{r}{2}-1}$ and

where $\omega\in C^1(0, T;H^{\frac{\rho}{2}+\frac{r}{2}})$.

Lemma 2.2 (^[9]). Let (H), $u_0\in H^{\frac{\rho}{2}+\frac{r}{2}}$ and $u_1\in H^{\frac{r}{2}-1}$ hold and $H(u) = \int_0^uh(s){\rm{d}}s$ hereafter; then, the following conditions hold:

(i) $|uh(u)| = \alpha|u|^{\theta+1}$, $|H(u)| = \frac{\alpha}{\theta+1}|u|^{\theta+1}$\ for all $u\in \mathbb{R};$

(ii) $(\theta+1)H(u) = uh(u)$ \ for all $u\in \mathbb{R}.$

Lemma 2.3 (Local existence ^[1]). Let $\frac{\rho}{2}+r\ge1$, $s > \frac{1}{2}$, $u_0\in H^s$, $u_1\in H^{s-1-\frac{\rho}{2}}$ and $h(u)\in C^{[s]+1}(\mathbb{R})$. Then there exist some functions $T(u_0, u_1)\in[0, T_{max}]$ such that the problem (1.1)-(1.2) have a unique solution $u\in C([0, T_{max}], H^s) \cap C^1([0, T_{max}], H^{s-1-\frac{\rho}{2}})$. If the maximum time $T_{max} < \infty$, then

Lemma 2.4 (Law of conservation of energy ^[1]). Let (H), $u_0\in H^{\frac{\rho}{2}+\frac{r}{2}}$, $u_1\in H^{\frac{r}{2}-1}$, $\mathcal{B}^{-1/2}\Lambda^{-1}u_1\in L^2$, $\mathcal{B}^{-1/2}\mathcal{K}u_0\in L^2$ and $H(u_0)\in L^1$. Then, over $t\in[0, T_{max}),$ we have

Now some auxiliary functionals and sets for the problem (1.1)-(1.2) are introduced

and

The following lemmas provide some properties of the functionals $\mathcal{J}(u)$ and $\mathcal{I}(u)$ defined above to consider the depth of the potential well.

Lemma 2.5. Let (H), $u_{0}\in H^{\frac{\rho}{2}+\frac{r}{2}}$, $u_1\in H^{\frac{r}{2}-1}$, $\|\mathcal{B}^{-1/2}\mathcal{K}u\|\neq0$ and $\int_\mathbb{R}uh(u){{\rm d}}x < 0$ hold. Then, we have the following:

(i) $\lim_{\varsigma\to 0} \mathcal{J}(\varsigma u) = 0$, \ $\lim_{\varsigma\to +\infty}\mathcal{J}(\varsigma u) = -\infty;$

(ii) Over $(0, +\infty)$, there is a unique $\varsigma^* = \varsigma^*(u)$ assuring that

and

$(iii)$ $\mathcal{I}(\varsigma u)$ is positive as $\varsigma\in(0, \varsigma^*)$, arrives at zero when $\varsigma = \varsigma^*$ and becomes negative as $\varsigma\in(\varsigma^*, +\infty)$.

Proof.

(ⅰ) From the fact that $\theta > 1$ and $\varsigma\rightarrow 0$, one knows that

For $\varsigma\rightarrow +\infty$ and $\int_\Omega uh(u){\rm{d}}x < 0$, we know that

(ⅱ) The conclusion follows from

(ⅲ) The fact that

directly gives the conclusion.

With a similar argument as in Lemma 2.5, one can infer the following lemma.

Lemma 2.6. Let (H), $u_{0}\in H^{\frac{\rho}{2}+\frac{r}{2}}$, $u_1\in H^{\frac{r}{2}-1}$, $\|\mathcal{B}^{-1/2}\mathcal{K}u\|\neq0$ and $\int_\mathbb{R}uh(u){\rm d}x > 0$ hold. Then, we have the following:

(i) $\lim_{\varsigma\rightarrow 0}\mathcal{J}(\varsigma u) = 0, \ \lim_{\varsigma\rightarrow +\infty}\mathcal{J}(\varsigma u) = +\infty$;

(ii) $\frac{{\rm{d}}\mathcal{J}(\varsigma u)}{{\rm{d}}\varsigma} > 0$ over $(0, +\infty)$;

(iii) $\mathcal{I}(\varsigma u)$ is positive over $(0, +\infty)$.

Now, with the above estimates in hand, the depth of the potential well can be estimated in the following lemma.

Lemma 2.7. Let (H), $u_{0}\in H^{\frac{\rho}{2}+\frac{r}{2}}$ and $u_1\in H^{\frac{r}{2}-1}$ hold. The depth of the potential well $d = \inf_{u\in\mathcal{N}}{\mathcal{J}(u)}$ with

for the problem (1.1)-(1.2) can be formulated as follows:

with

Proof. Given that $u\in\mathcal{N}$, we can infer that

which together with

yields

Now, from (2.3), (2.4), $u\in\mathcal{N}$, (2.7) and (2.8), we have

which completes the proof of this lemma.

3.   Low initial energy case

The following lemma shows that both the sets $\mathcal{W}$ and $\mathcal{V}$ are invariant for $\mathcal{E}(0) < d$.

Lemma 3.1. Let (H), $u_0\in H^{\frac{\rho}{2}+\frac{r}{2}}$, $u_1\in H^{\frac{r}{2}-1}$, $\|\mathcal{B}^{-1/2}\mathcal{K}u\|\neq 0$ and $\mathcal{E}(0) < d$. Then, we have the following:

(i) $u\in \mathcal{W}$ if $u_0\in \mathcal{W}$;

(ii) $u\in \mathcal{V}$ if $u_0\in \mathcal{V}$,

where $u(t)$ denotes a local solution to the problem (1.1)-(1.2).

Proof. Because the proofs of (ⅰ) and (ⅱ) are similar, we only prove one. From the contradiction arguments, it is supposed that there exists a first time $t_1\in(0, T_{max})$ such that $\mathcal{I}(u(t_1))\leq 0$, which together with Lemma 2.3, indicates that $\mathcal{I}(u(t_2)) = 0$ for certain $t_2\in(0, t_1)$. Together with Lemma 2.7 we can conclude the following contradiction

The following theorem presents the global existence for $\mathcal{E}(0) < d$.

Theorem 3.2. If (H), $u_0\in H^{\frac{\rho}{2}+\frac{r}{2}}$, $u_1\in H^{\frac{r}{2}-1}$, $\mathcal{E}(0) < d$ and $u_0\in \mathcal{W}$, then the problem (1.1)-(1.2) admits a global weak solution

Proof. Let

be the corresponding approximate solution that satisfies

and

with a system of base functions denoted by $\{\omega_j(x)\}$ in $H^{\frac{\rho}{2}+\frac{r}{2}}\cap H^{\frac{r}{2}-1}$. From (3.2) and (3.3), we get

as $n\rightarrow +\infty$. Now we claim that

Indeed

where $\varphi _n = u_0+\vartheta (u_n(0)-u_0) \in H^{\frac{\rho}{2}+\frac{r}{2}}$ and $\vartheta \in (0, 1).$ For $\theta > 1$ and $N = 1$, it follows that $\|h(\varphi_n)\|^2 = \alpha^2\|\varphi_n\|_{2\theta}^{2\theta} < C(\alpha, \rho, \theta) \|\varphi_n\|_{H^{\frac{\rho}{2}+\frac{r}{2}}}^{2\theta} < C'$ with $C, C' > 0.$ Thus the claim is proved and

Recalling $u_0\in \mathcal{W}$, and the fact that (3.2) and (3.3) imply that $u_n(0)\in \mathcal{W}$ as $n\rightarrow +\infty$, combining the arguments of Lemma 3.1 and (3.4), one can see that $u_n(t)\in \mathcal{W}$ as $n\rightarrow +\infty$ for $t\in[0, +\infty)$. Consequently, multiplying (3.1) by $\phi_{sn}'(t)$ and summing for $s$ we have

which gives

Incorporating (3.4) we get

and

for $t \in [0, +\infty)$ as $n \rightarrow +\infty$. Thus, by ({1.5}) and (1.6), we have

and

which gives the following:

By an argument similar to that for $h(\varphi_n)$, one can infer that

Integrating (3.1) over $(0, t)$ yields

In (3.5), fix $s$ and let $n\rightarrow +\infty$, then, we get

From (3.2) and (3.3) it follows that $u(x, 0) = u_0(x)$ is bounded in $H^{\frac{\rho}{2}+\frac{r}{2}}$ and $u_t(x, 0) = u_1(x)$ is bounded in $H^{\frac{r}{2}-1}$. Thus, Theorem 3.2 is proved.

The following lemma is used to prove the finite time blowup for $\mathcal{E}(0) < d$.

Lemma 3.3. Let (H), $u_0\in H^{\frac{\rho}{2}+\frac{r}{2}}$ and $u_1\in H^{\frac{r}{2}-1}$ hold. If $u_0 \in \mathcal{V}$ and $\mathcal{E}(0) < d$, then

Proof. Lemma 2.7 implies that

Note that Lemma 3.1 (ⅱ) ensures that $u\in \mathcal{V}$, which together with (2.7) and (3.7) gives (3.6). So this lemma is proved.

The next theorem states the finite time blowup for $\mathcal{E}(0) < d$.

Theorem 3.4. If (H), $u_0\in H^{\frac{\rho}{2}+\frac{r}{2}}$, $u_1\in H^{\frac{r}{2}-1}$, $\mathcal{E}(0) < d$ and $u_0\in \mathcal{V}$, then the problem (1.1)-(1.2) admits a finite time blowup result.

Proof. Arguing by contradiction, we suppose that there exists a global solution $u$. Define

for any $T_1 > 0$. So,

Then,

Applying the definition of $\mathcal{I}(u)$ and taking $\omega = u$ in (2.1), we get

A substitution of both (2.2) and (2.4) into (3.11) gives

Then by Lemma 3.3, we see that

where we use the following relation:

At this point, (3.12) and (3.9) with the estimation

and

imply that

Hence

Set $t \rightarrow T^* < T_1$; then,

Thus we complete the proof.

4.   Critical initial energy case

In this section, we aim to adapt the method used in ^[11,12,13] to the critical initial energy case $\mathcal{E}(0) = d$.

Theorem 4.1. If (H), $u_0\in H^{\frac{\rho}{2}+\frac{r}{2}}$, $u_1\in H^{\frac{r}{2}-1}$, $\mathcal{E}(0) = d$ and $u_0\in \mathcal{W}$, then the problem (1.1)-(1.2) has a global solution \ $u(t)\in C^1 (0, +\infty; H^{\frac{\rho}{2}+\frac{r}{2}})$, $u_t (t)\in C (0, +\infty; H^{\frac{r}{2}-1})$.

Proof. The proof is established by considering the following two cases.

Case Ⅰ. $\|\mathcal{B}^{-1/2}\mathcal{K}u_0\|^2 \neq 0$.

(1) $\int_{\mathbb{R}}u h(u){\rm d}x < 0$. Let $\varsigma_{n} = 1-\frac{1}{n}$ and $u_{0n} = \varsigma_{n}u_0$, $n = 2, 3\cdots$. Consider Equation (1.1) with

From $u_{0} \in \mathcal{W}$ and (2.5), it follows that $\varsigma^{*} = \varsigma^{*}(u_0) > 1$ and hence $1-\frac{1}{n} < 1 < \varsigma^{*}$, which implies that $\mathcal{I}(u_{0n}) > 0, \ \mathcal{J}(u_{0n}) < \mathcal{J}(u_0)$, and

(2) $\int_{\mathbb{R}} uh(u) {\rm{d}}x > 0.$ From $u_0\in \mathcal{W}$ and Lemma 2.6, it follows that $\mathcal{I}(\varsigma u_0)\left|_{\varsigma = 1}\right. = \varsigma \frac{{\rm d}}{{\rm d}\varsigma} \mathcal{J}(\varsigma u_0)\left|_{\varsigma = 1}\right. > 0$, and $\mathcal{I}(\varsigma u_0) > 0$ for $\varsigma\in (\varsigma', \varsigma'')$ with $1\in(\varsigma', \varsigma'')$. Lemma 2.6 yields that $\frac{{\rm d}}{{\rm d}\varsigma} \mathcal{J}(\varsigma u_0) > 0$ over $(\varsigma', \varsigma'')$, which yields a sequence ${\varsigma_n}\in(\varsigma', 1)$, $n = 1, 2, 3, \cdots$ and $\varsigma_n\rightarrow1$ as $n\rightarrow +\infty$. Let $u_{0n} = \varsigma_n u_0$, $n = 1, 2, 3, \cdots$. Consider Equation (1.1) with

At this point

and Lemma 2.6 implies that

and

Case Ⅱ. $\|\mathcal{B}^{-1/2}\mathcal{K}u_0\|^2 = 0$.

Let $\varsigma_{n} = 1-\frac{1}{n}$, $u_{1n}(x) = \varsigma_{n}u_{1}(x), \ n = 2, 3\cdots$. Consider (1.1) with

From $\|\mathcal{B}^{-1/2}\mathcal{K}u_0\|^2 = 0$, it follows that $\mathcal{J}(u_0) = 0$ and $\frac{1}{2}\|u_1\|^2 = \mathcal{E}(0) = d$. As a result,

Combining Case Ⅰ and Case Ⅱ, again by the argument of Theorem 3.2, we can conclude the result of Theorem 4.1.

The next lemma is used to consider the finite time blowup for $\mathcal{E}(0) = d$.

Lemma 4.2. Let (H), $u_0\in H^{\frac{\rho}{2}+\frac{r}{2}}$ and $u_1\in H^{\frac{r}{2}-1}$ hold. Assume that $\mathcal{E}(0) = d$, $(\mathcal{B}^{-1/2}\Lambda^{-1}u_{0}, \mathcal{B}^{-1/2}\Lambda^{-1}u_1)\geq 0$ and $u_0\in \mathcal{V}$; then, $u\in \mathcal{V}$.

Proof. Arguing by contradiction, we suppose that $\mathcal{I}(u(\tilde{t}_0)) = 0$ and $\mathcal{I}(u(t)) < 0$ for $0 < t < \tilde{t}_0$ and $\tilde{t}_0\in(0, T_{max})$. So Lemma 2.7 implies that $\mathcal{J}(u(\tilde{t}_0))\geq d$. By $\mathcal{E}(0) = d$ and Lemma 2.4, we have that $\mathcal{J}(u(\tilde{t}_0)) = d$ and $\|\mathcal{B}^{-1/2}\Lambda^{-1}u_t(\tilde{t}_0)\| = 0$. As a result, (3.8), (3.10) and (3.11) yield

and

also

which implies that $\eta(t)$ is increasing on $[0, \tilde{t}_0]$. It contradicts that $\|\mathcal{B}^{-1/2}\Lambda^{-1}u_t(\tilde{t}_0)\| = 0$. So, we complete the proof.

Theorem 4.3. If (H), $u_0\in H^{\frac{\rho}{2}+\frac{r}{2}}$, $u_1\in H^{\frac{r}{2}-1}$, $(\mathcal{B}^{-1/2}\Lambda^{-1}u_{0}, \mathcal{B}^{-1/2}\Lambda^{-1}u_1) \geq 0$, $\mathcal{E}(0) = d$ and $u_0\in \mathcal{V}$ hold, then the problem (1.1)-(1.2) has a finite time blowup result.

Proof. It is not necessary to write down the completed proof as we can use the proof of Theorem 3.4 to make it. First, Equation (3.8) and the proof of Theorem 3.4 imply Equation (3.11). Then applying Lemma 4.2, we obtain Equation (3.13). The reminder proof is similar to Theorem 3.4.

5.   Finite time blowup when $\mathcal{E}(0) > 0$

Theorem 5.1. Assume that (H), $u_0\in H^{\frac{\rho}{2}+\frac{r}{2}}$, $u_1\in H^{\frac{r}{2}-1}$ and the following three conditions all hold

(i) $\mathcal{I}(u_0) < 0;$

(ii) $(\mathcal{B}^{-1/2}\Lambda^{-1}u_0, \mathcal{B}^{-1/2}\Lambda^{-1}u_1)\geq 0;$

(iii) $\|\mathcal{B}^{-1/2}\Lambda^{-1}u_0\|^2 > \frac{2\gamma(\theta+1)}{\theta-1}\mathcal{E}(0) > 0$ with $\gamma > 0$.

Then, the problem given by Equations (1.1)-(1.2) has an arbitrarily positive initial energy finite time blowup solution.

Proof. Step Ⅰ. We claim over $[0, T_{max})$ that

Arguing by contradiction, suppose that $\mathcal{I}(u(\overline{t}_0)) = 0$ for certain $\overline{t}_0\in [0, T_{max})$ and $\mathcal{I}(u(t)) < 0$ for $0\leq t < \overline{t}_0$. So, (3.11) implies that

where $\dot{\eta}(0)\ge0$, which yields that $\dot{\eta}(t) > 0$ over $[0, \overline{t}_0)$ and

Consequently,

Further (2.2), $\mathcal{I}(u(\overline{t}_0)) = 0$ and Lemma 2.2 imply that

By the multiplier theorem in ^[14], the definitions of operators $\mathcal{B}$, $\Lambda$ and (1.5), we infer that

where

Now, both (5.2) and (5.3) imply that

which contradicts (5.1) and then confirms that $\mathcal{I}(u(t)) < 0$ over $[0, T_{max})$.

Combining (3.11) and $\mathcal{I}(u(t)) < 0$ on $[0, T_{max})$, one has

then $\dot{\eta}(t) > 0$ on $[0, T_{max})$ due to the condition (ⅱ) as follows

which implies that

By the definition of $\eta(t)$ in (3.8) and the condition (ⅲ), we get

Step Ⅱ. By using the claim in Step Ⅰ, i.e., $\|\mathcal{B}^{-1/2}\Lambda^{-1}u\|^2 > \frac{2\gamma(\theta+1)}{\theta-1}\mathcal{E}(0)$ over $[0, T_{max})$, we can infer (3.13) with $\chi(t) = (\theta-1)\|\mathcal{B}^{-1/2}\mathcal{K}u\|^2 -2(\theta+1)\mathcal{E}(0) > \tilde{\delta} > 0$ over $[0, T_{max})$, where (3.8) has been recalled. The proof is similar to Theorem 3.4.

Use of AI tools declaration

The authors declare that they have not used artificial intelligence tools in the creation of this article.

Acknowledgments

Runzhang Xu was supported by the National Natural Science Foundation of China (No. 12271122). Yanbing Yang was supported by the Heilongjiang Provincial Natural Science Foundation of China (No. LH2021A002), the Heilongjiang Postdoctoral Research Start-up Funding Project (No. LBH-Q20086), and the Research Funds for the Central Universities.

Conflict of interest

The authors declare that there is no conflict of interest.