1. Introduction
Agroforestry refers to the land use systems where woody perennials such as trees, shrubs, palms, bamboo etc. are cultivated on the related land units as agricultural crops and/or animal rearing [1,2]. It has been practiced in many countries to offer a wide range of economic, social, and ecological benefits by increasing per capita income of the farms by planting high value tree species [3]. It also leads to improving the soil and land fertility as well as productivity [4], enhances household resilience [3], reduces the impacts of climate variability and change [5,6] by conserving and safeguarding biodiversity [7,8,9] and improving and ameliorating air and water quality [10,11,12]. The agroforestry retains high importance because it provides enormous benefits as well as has capacity to safeguard the food security for the future generation also be discussed in 1st world congress of agroforestry (Working Together for Sustainable Land Use Systems) held in the year 2004 in Florida, USA [13].
In India, around 80% of the population is agrarian and the majority of them live a miserable life due to poor livelihood opportunities especially in the rural areas. More than 80% farmers are small land-holders (having less than two hectares) and comprises of 60% of the total cultivated area. The irrigation depends on the rainfall, primarily. These rainfed farms are always under pressure due to lack of definite means of irrigation unlike rainfalls, which is compounded due to presence of less biodiversity zones. Agroforestry could be one viable solution for to meet the challenges of food, nutrition, energy, livelihood and environmental security [14]. India became the first country in the world to adopt an agroforestry policy. Agroforestry has the potential to achieve sustainable growth in the agriculture sector and it has capability of optimizing the productivity along with diminishing climate change impacts [15].
Why remote sensing, GIS and spatial modeling approach
The traditional approach for accounting and studying biophysical parameters in remote areas is not only expensive but also time-consuming. Moreover, the information obtained by the key users is often incomplete and inadequate with limitations of incorporating time bound changes. Also the current available data is not adequate to allow a well-interpreted, geographic approach to manage land environment or to differentiate between the impact of increased anthropogenic activity and other impacts such as climate change. In the recent time, analysis of remote sensing (RS) data [16,17,18,19,20,21] can provide an important input to the geographic information system (GIS) and it maneuvers the above stated limitations [22].
Few researchers and scientists have evaluated the land for various agroforestry application using remote sensing and GIS. Ritung et al. used climatic, soil and topographical data and evaluated the land based on FAO guidelines for Aceh Barat District of Indonesia and recommended land suitability towards various agroforestry crops [23]. Kihoro et al. carried out the study in Kirinyaga, Embu and Mberee counties in Kenya and evaluated the land suitability analysis using biophysical variables of soil, climate and topography for rice growing sites using a multi-criteria evaluation and GIS approach and found that the potential area for rice growing is 86, 364 ha and out of this only 12% is under rice cultivation [24]. Dengiz carried out land suitability assessment in the Cankiri-Kizilirmak district in the Central Anatolian region of Turkey for rice cultivation based on GIS modeling [25]. The result showed that 55.5% of the study area is highly or moderately suitable for rice cultivation, whereas 34% of that area is not suitable for rice cultivation due to soil and topographic conditions. Yedage et al. evaluated the land suitability based on FAO guidelines towards horticulture crop (Pomegranate) in the Sangola Tahsil of Solapur district of Maharashtra, India using Multi-criteria and GIS application [26]. The parameter used for evaluation were slope, soil pH, soil organic matter, soil NPK, soil texture, soil electric conductivity, soil depth, ground water availability and the land use/land cover. Roughly 27 and 18% of the study area was highly and moderately suitable respectively for Pomegranate cultivation. Sarkar et al. evaluated the land of watershed Beko situated in the Purleia district of West Bengal state of India for wheat suitability analysis using weighted mean calculated by pair-wise comparison and the parameters he took were climate (rainfall), wetness (soil drainage), fertility status (soil pH and organic matter), root condition (soil depth and texture) and slope and found this watershed is moderately suitable for wheat crop [27]. Ayehu and Besufekad studied west central highlands of Amhara Region, Ethiopia and applied GIS-based Multi-Criteria Decision Approach [28]. The soil, climatic conditions, and topography parameter were used to identify the land suitability for rice production. They rank the various suitability factors and the resulting weights were used to construct the suitability map layers using weighted sum overlay technique. The 70% of the total study area was found to be highly and moderately suitable for rice crop production. Resiner et al. has been purposed as alternative land use system Silvoarable agroforestry (SAF) for Europe [29]. Data used and integrated in GIS domain were soil, climate, topography, and land cover for identifying agroforestry target regions for few tree species (Juglans spp., Prunus avium, Populus spp., Pinus pinea, and Quercus ilex) suitability. The SAF systems have the potentiality to reduce the risk of soil erosion, nitrate leaching and increase landscape diversity. Ahmad and Goparaju studied urban area land suitability towards urban forest of Ranchi city, Jharkhand, India (a 30-km radius from city center) and used weighted mean for evaluating land suitability for urban forestry using parameter such as soil wetness, slope, soil organic carbon, drainage buffer and urban buffer [17]. The identified potential area for urban forestry was 27% as highly suitable, 38% as medium suitable and 35% as least suitable sites within the existing open spaces.
Despite the increasing trend of use of the geospatial technology and spatial modeling, still the application of GIS for developing natural resource-based (mainly for agriculture, forestry and fisheries) spatial data/themes and its use in spatial management has tremendous scope in developing decision support system for natural resources including agriculture and forestry sector.
The present study aims for applying RS and GIS towards visualization of various soil and environmental data, to reveal the trends and interrelationships. To create a nutrient availability map for the whole study area and agroforestry suitability map in the open land of the study area were generated. Based on high agroforestry suitability percent the prioritization of blocks in the present study area was done. Three watersheds in the part of study area were evaluated and prioritized based on the high percent of agroforestry suitability. Few village point location buffers were studied towards its proximity with high percent of agroforestry suitability. These tools could be profitably used for the identification of potential sites for agroforestry at block level as well as at state level. Land suitability is the degree of appropriateness of land for a certain use, which could be assessed for actual land suitability [30] and for future potential land suitability [23]. Agroforestry suitability being one special form of land suitability is very pertinent to study in the current times when there is tremendous pressure on the land as limited commodity.
2. Materials and Methods
2.1. The study area
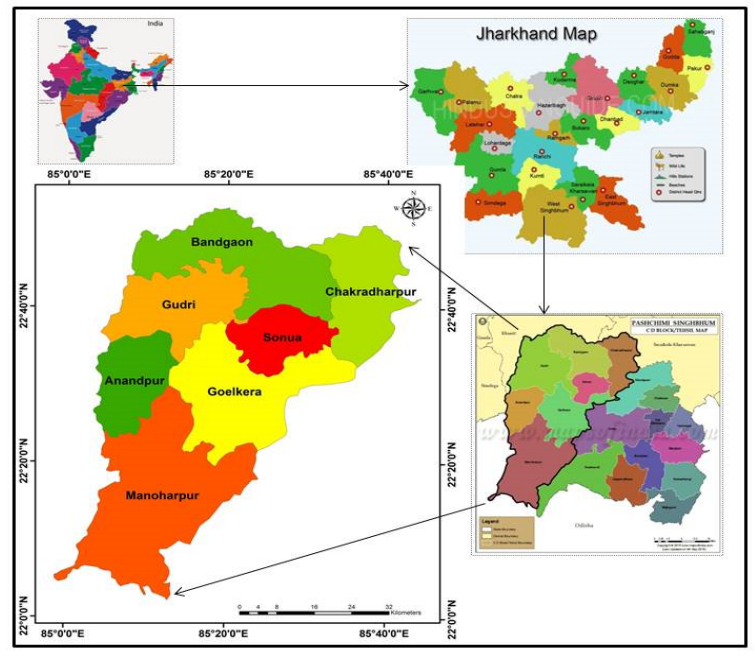
The geographical coordinates of the study area comprise of Latitude 22°04'21''N to 22°52'42''N and Longitude 84°58'08''E to 85°44'32''E, whereas total geographical area is 355, 136 ha (Figure 1). It is Chakardharpur subdivision of West Singhbhum district of the Indian State of Jharkhand, which is primarily a tribal dominated district. This subdivision consists of seven blocks namely Manoharpur, Goelkera, Chakradharpur, Anandpur, Sonua, Bandgaon and Gudri. The elevation varies from 170 to 883 m from the mean sea level. As per Champion and Seth classification [31], the forest of this area is described as moist peninsular valley Shorea robusta (Sal) under the category [3C/C2e (ⅲ)], where natural regeneration is excellent and the tree composition varies with moisture due to variation of aspect and slope of the hills. The area is also dominated by hills alternating with valleys, steep mountains and deep forest on the mountain slopes. Further, Asia's one of the dense Sal forests known as Saranda constitutes part of the district.
2.2. Data preprocessing and analysis
The data used for the analysis was Landsat OLI (Path: 140/44, Row: 140/45, dated 18-12-2015) having 30 m spatial resolution with UTM projection (Zone 45). The satellite data was downloaded from United States Geological Survey (USGS) portal and was obtained in 11 bands. The satellite images were radiometrically and geometrically corrected. Various individual bands were layer stacked to obtain a composite image for further analysis. Various scenes were mosaiced to obtain a single image. The vector layer of the study area was used to extract the desired image from the larger mosaicked image. The satellite image was used to generate wetness and land use/land cover (LULC) map.
The Digital Elevation Data (DEM) was also downloaded from the USGS portal (ASTER DEM, resolution 30m). A subset was generated from the mosaic image using the vector layer of the study area. Thematic maps such as slope and elevation map were generated using spatial analyst tools of ArcGIS Software. Ancillary data was used to obtain rainfall map based on interpolation technique. The detail of the methodology is presented through a schematic flowchart (Figure 2). The software's used in the analysis were ERDAS imaging (Version 9.1) and ArcGIS spatial analyst (Version 10.1).
2.3. Spatial modeling and suitability analysis for agroforestry
Here agroforestry suitability refers to land suitability to various agroforestry crops. Remote sensing and GIS based themes help to integrate information on GIS platform. Computer based decision support tools towards spatial modeling and suitability analysis for agroforestry needs a relevant database, GIS knowledge, appropriate suitability based model concept, and an expert to execute the whole work. Multi-criteria analysis using weighted means was applied for spatial modeling, which is one of the widely acceptable methods for the suitability analysis [28]. The geospatial modeling was executed to obtain agroforestry suitability analysis using weighted overlay technique. Agroforestry suitability surface was obtained by integrating the various factor used and based on their surface values they are classified into three agroforestry suitability class (high, medium and low).
2.4. Nutrient availability mapping parameters
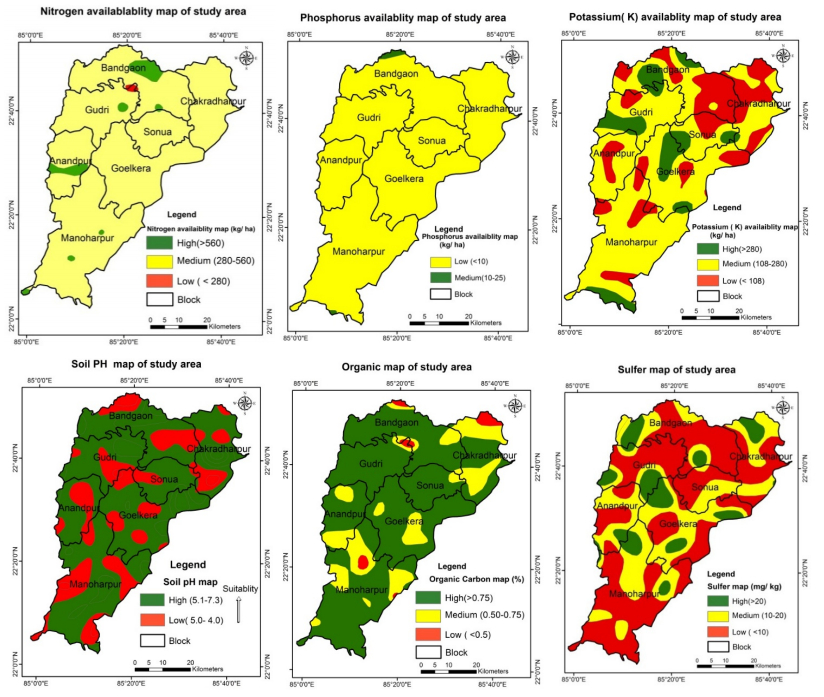
District soil map was used to generate the nutrient availability map by assigning equal weights to all soil nutrient parameter making it as a whole to 100%. They were chosen based upon the findings of various related research works of nutrient suitability also adopted by FAO towards plant growth [26,30]. Accordingly, soil nitrogen (N), phosphorus (P), potassium (K), organic carbon (C), pH and soil sulphur (S) were used for producing nutrient availability map, they are important parameters which manifest soil fertility and can ensure food security. Weights and ranks were assigned for mapping different thematic maps (Table 1).
Table 1. Weight matrix for parameters and ranking for nutrient availability mapping.
| Nutrient factor |
Weights |
Value/Description |
Ranks |
Suitability |
| Nitrogen |
16.67% |
> 560 kg/ha |
3 |
High |
| 280–560 kg/ha |
2 |
Medium |
| < 280 kg/ha |
1 |
Low |
| Phosphorus |
16.67% |
10–25 kg/ha |
2 |
Medium |
| < 10 kg/ha |
1 |
Low |
| Potassium |
16.67% |
> 280 kg/ha |
3 |
High |
| 108–280 kg/ha |
2 |
Medium |
| < 108 kg/ha |
1 |
Low |
| Organic carbon (%) |
16.67% |
> 0.75 |
3 |
High |
| 0.50–0.75 |
2 |
Medium |
| < 0.50 |
1 |
Low |
| pH |
16.67% |
5.1–7.3 |
2 |
Medium |
| 4.0–5.0 |
1 |
Low |
| Sulfur |
16.67% |
> 20 mg/kg |
3 |
High |
| 10–20 mg/kg |
2 |
Medium |
| < 10 mg/kg |
1 |
Low |
2.5. Agroforestry mapping parameters
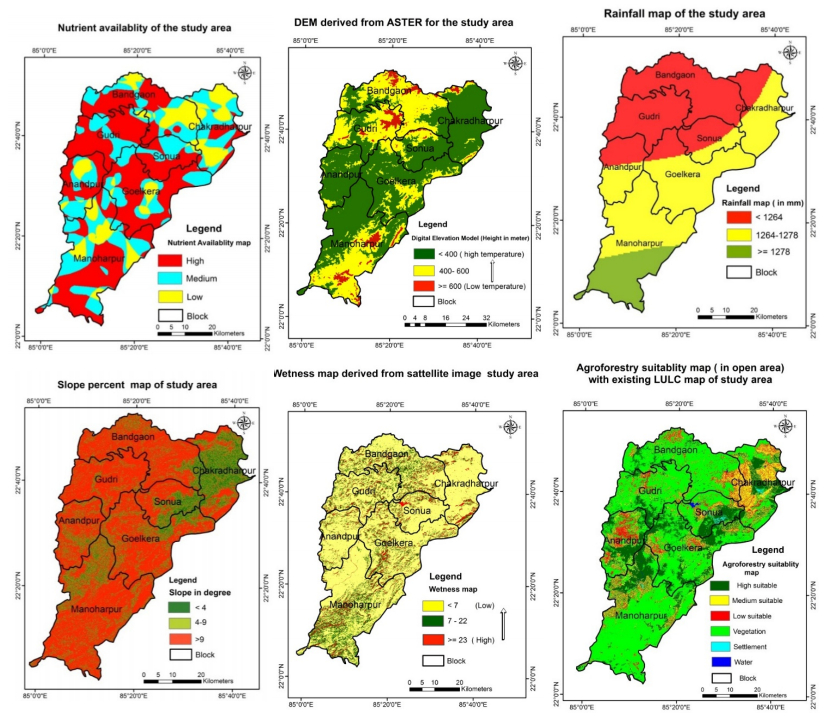
Based upon the empirical results obtained from various research works, the factors which contribute potentially towards agroforestry are nutrient availability [26], slope [24], wetness [17], rainfall [27] and elevation [23] whose weights were scientifically chosen. Ranks and weights were assigned for different thematic maps were chosen based on pair-wise comparison (Table 2). The same was emphasized by Sys et al. [32,33] and has also been adopted by FAO. These parameters have the potential for delineating intensive suitable area for various crops. The above approach helped in assessing the land suitability for 22 crops in north western Syria [33].
Table 2. Weight matrix for parameters for agroforestry suitability mapping.
| Agroforestry factor |
Weight |
Value/Description |
Ranks |
Suitability |
| Nutrient availability |
35 |
Three categories based on weighted average output |
3 |
High |
| 2 |
Medium |
| 1 |
Low |
| Slope |
30 |
< 4 |
3 |
High |
| 4–9 |
2 |
Medium |
| ≥ 9 |
1 |
Low |
| Wetness factor |
15 |
< 7 |
1 |
Low |
| 7–23 |
2 |
Medium |
| ≥ 23 |
3 |
High |
| Rainfall |
12 |
< 1264 mm |
1 |
Low |
| 1264–1278 mm |
2 |
Medium |
| ≥ 1278 mm |
3 |
High |
| Elevation |
8 |
< 400 m |
3 |
High |
| 400–600 m |
2 |
Medium |
| ≥ 600 m |
1 |
Low |
2.6. Agroforestry suitability analysis
To enhance the livelihood of the poor people especially in tribal dominated areas, there is a strong need to adopt agroforestry practices. For an ideal growth, suitability assessment of land which matches the potential agroforestry products (i.e. crops and trees) with certain condition is necessary. It does so by overlaying the data maps with information such as nutrient availability, slope, wetness, rainfall and elevation maps in GIS platform [34]. Each layer (map) was ranked based on its suitability. Agroforesters, however have yet to use these technologies extensively [35]. The agroforestry suitability map was generated using ERDAS imagine model maker based on differential weighs for various parameters (Table 2) and was classified into three groups such as high, medium and low.
2.6.1. Nutrient availability analysis
The nutrient availability map was generated based on the equal weights of all the parameters (Table 1) and it was classified into three groups such as high, medium and low.
2.6.2. Digital elevation model
Elevation is a factor which influences the plant growth to a large extent [36]. As the elevation increases, temperature and vegetation gradually decreases and therefore a concept of tree line exists. Air temperature is an important environmental factor which affects the tree growth rates. It has a direct role to play in the photosynthesis and respiration along with the significant effect on plant tissue temperature. The DEM was further reclassified into five sub groups at 200 m interval and new values were re-assigned based upon the agroforestry suitability rating, were ranked as 3, 2, and 1 and described as high, medium, low, respectively.
2.6.3. Rainfall intensity
Rainfall is considered as an important parameter and its spatial variation show positive correlation with the plant growth adopted by FAO for land evaluation. The rainfall data from year 1990 to 2002 was downloaded [37]. The average annual rainfall for 13 years was used to generate spatial rainfall pattern (continuous surface) in ArcGIS by Kriging Interpolation method [38]. Based upon agroforestry suitability, the map was further classified into three classes (< 1264, 1264–1278, ≥ 1278 mm); were ranked as 1, 2 and 3 and described as low, medium and high, respectively.
2.6.4. Slope percent
Slope is described as the measurement of the rate of change of elevation of the land per unit distance. The steeper slopes allow faster movement of water. It affects the stability of aquatic habitats due to its erosion potential because of higher kinetic energy of flowing water. Land with gentle slopes is good for plant growth as water stays there for some period provide adequate moisture to the soil. Thus the gentle slopes are suitable for agroforestry than steep slopes. The slope was derived from aster DEM and it was reclassified into classes of 0–4°, 4–9°, > 9° using standard reclassification technique and was ranked as 3, 2, and 1 with high, moderate and low description.
2.6.5. Wetness index
Wetness in the soil is necessary for crop growth and agricultural production. Thus, provides a suitable condition for the agroforestry practice. Baig et al. have developed the methodology to map wetness using Landsat 8 data [40]. The wetness coefficient was developed for different bands (Table 3). The wetness map of the study area was generated using the coefficients given in the table. Based upon suitability towards agroforestry, three classes based on the wetness values (< 7, 7–23, > 23) have been identified and were ranked as 1, 2 and 3 with description of low, medium and high, respectively.
Table 3. Wetness coefficients for Landsat 8 OLI reflectance.
| Band |
Blue |
Green |
Red |
NIR |
SWIR 1 |
SWIR 2 |
| wetness |
0.1511 |
0.1973 |
0.3283 |
0.3407 |
−0.7117 |
−0.4559 |
2.6.6. LULC mapping
The FCC was classified to obtain a LULC map and the Normalized Difference Vegetation Index (NDVI) was executed, which was further analyzed to produce a vegetation mask. The settlement class was visually interpreted and delineated from the false color composite (FCC). The other two classes were delineated using supervised classification (maximum likelihood technique) wherein training sets (4–5) were given for each class distributed uniformly throughout the image. All delineated classes were integrated in model maker to obtain classified dataset containing four classes namely vegetation, water, settlement and open area. The open area in this map was declared as the hotspot for the agroforestry studies and was considered as suitable site for further development.
3. Results
3.1. Nutrient availability mapping
Using weight matrix and ranks (Table 1) for various parameters, individual maps were developed on ArcGIS 10.1 platform (Figure 3).
3.2. Agroforestry suitability mapping
Using the weight matrix and ranks (Table 2) for various parameters, individual maps were developed in ArcGIS 10.1 platform (Figure 4). The agroforestry suitability map generated is shown in Figure 4. The result of the study area showed 21.6, 12.5, and 8.3% agroforestry suitability of the area as high, medium and low, respectively (Table 4). The ongoing agroforestry projects can be diverted in these identified areas of high suitability for optimum production.
Table 4. Agroforestry suitability (in open area) of study area with area statistics.
| Agroforestry suitability |
Area in hectares |
Percentage |
| High |
76, 866.8 |
21.6 |
| Medium |
44, 411.7 |
12.5 |
| Low |
29, 441.6 |
8.3 |
3.3. Block, watershed and village point buffer wise agroforestry suitability
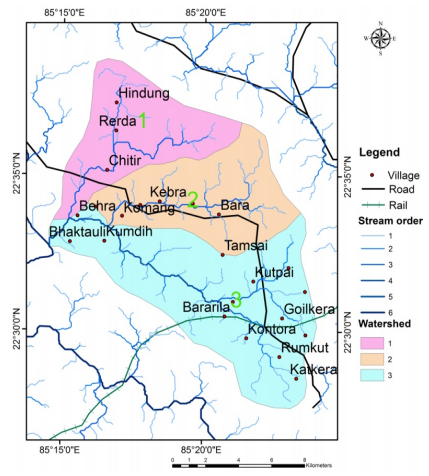
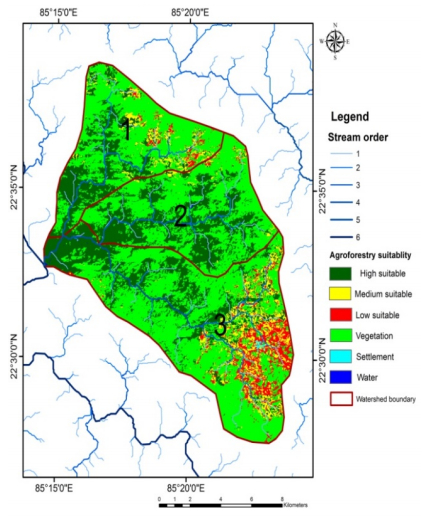
The emphasis of the study was to bring the outcomes to the end users, who are farmers in this case. Blocks are administrative boundary for various fund implementations whereas watershed is the natural boundary best suited to tap local resources in conjunction with the rainwater. The boundaries (vector file) of all the blocks of Chakadharpur subdivision were brought into GIS domain. The block wise agroforestry suitability analysis is given in Figure 5, and based upon the results the decision can be taken for prioritizing a block. Three watersheds were delineated using DEM in central part of the study area mostly covering Goelkera block and were evaluated for prioritizing (Table 5).
Table 5. Agroforestry suitability of watershed (natural boundary) with area statistics.
| Agroforestry suitability |
Area in hectares |
Percentage |
| High |
Medium |
Low |
High |
Medium |
Low |
| Watershed-1 |
1446.0 |
316.6 |
101.8 |
30.8 |
6.7 |
2.2 |
| Watershed-2 |
2096.4 |
80.2 |
18.5 |
40.1 |
1.5 |
0.4 |
| Watershed-3 |
2313.1 |
906.8 |
803.7 |
25.1 |
9.8 |
8.7 |
Within these watersheds 22 village points were identified for further study. The buffer of 1 and 2 km were created around these points (Figures 6 and 7). These buffers were further evaluated showing its proximity to high suitable agroforestry sites (in open areas), which generates tremendous opportunity to the villagers to carry out agroforestry projects locally (Table 6).
Table 6. Agroforestry suitability of village buffer 1 and 2 km (population proximity) with area statistics.
| Agroforestry suitability |
Area in hectares |
Percentage |
| High |
Medium |
Low |
High |
Medium |
Low |
| Village buffer 1-km |
2665.8 |
643.2 |
612.3 |
39.1 |
9.4 |
9.0 |
| Village buffer 2-km |
5079.7 |
1283.7 |
1095.7 |
33.7 |
8.5 |
7.3 |
4. Discussion
4.1. Validation of result
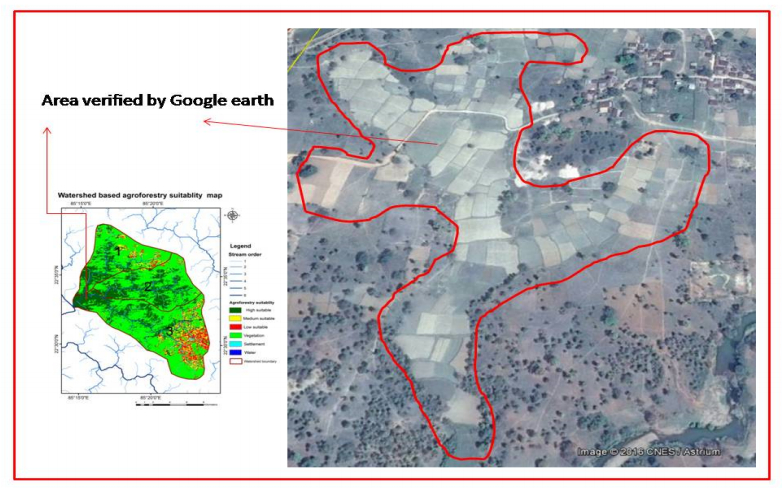
Field verifications were done in watersheds based on agroforestry suitability status as well as it was cross-validated by Google Earth map. It showed a very positive correlation (Figure 8). These high priority areas in open space were found covered with high soil fertility, high soil moisture content with very low slope. Further, drainage pattern provides a suitable environment for practicing agroforestry modules which are locally approved.
4.2. Nutrient availability and agroforestry suitability analysis
Soil is a major source of nutrients which are required for the plant growth. The three main nutrients are nitrogen (N), phosphorus (P) and potassium (K), which makes a trio known of NPK. Sulphur (S) is the fourth major nutrient for the plant growth, which serves many additional functions in plants. It is also used in the formation of amino acids, proteins, and oils and necessary for chlorophyll [41]. One of the important factors to determine quality of soil and source of nutrients for improving physical and biological properties of soils in addition to productivity is the organic carbon (C). Chemical environment of soil is dynamic and reactions that maintain dilute solution of nutrient elements are indispensable for continual plant growth. Nutrient transformation and its availability in soils depend on its pH [42].
The first step to determine potential zones for harnessing agroforestry module is the open areas in proximity to the villages, which were identified as agroforestry suitable sites. This data (Table 7) will greatly help policy makers in prioritizing the geographic area for agroforestry modules. The study has derived agroforestry suitability map which would greatly support several livelihood programs of Chakardharpur subdivision of west Singhbhum District.
Table 7. Agroforestry suitability of blocks with area statistics.
| Agroforestry suitability |
| Block name |
Area in hectares |
Percentage |
| High |
Medium |
Low |
High |
Medium |
Low |
| Manoharpur |
18, 930.6 |
8940.8 |
3267.8 |
19.8 |
9.4 |
3.4 |
| Goelkera |
18, 465.5 |
5820.3 |
3133.0 |
29.5 |
9.3 |
5.0 |
| Chakradharpur |
11, 492.6 |
13, 688.9 |
6110.9 |
27.1 |
32.3 |
14.4 |
| Anandpur |
9454.0 |
4385.1 |
5942.8 |
31.1 |
14.4 |
19.5 |
| Sonua |
7834.0 |
2414.1 |
2070.0 |
36.1 |
11.1 |
9.5 |
| Bandgaon |
1753.2 |
5260.7 |
5600.2 |
4.0 |
11.8 |
12.6 |
| Gudri |
9142.6 |
4002.7 |
3370.6 |
15.5 |
6.8 |
5.7 |
4.3. Agroforestry suitability rating
4.3.1. High priority areas
These areas were found in the open spaces covered with high soil nutrient, adequate wetness, high rainfall with gentle slope and low elevation provides high favorable condition for the plant growth. It comprises of 76, 866.8 ha (21.6% of total study area). These low land areas are highly fertile and best suitable for cultivating paddy crops due adequate availability of water and moisture during rainy season. Also, water conservation practices such as check dam construction in the area ensures required moisture throughout the year including summer seasons, which guarantees an opportunity to harness the agroforestry module.
4.3.2. Medium
These areas were prevalent in moderate soil nutrient; high to moderate wetness, high to moderate rainfall with low to moderate slope and low to medium elevation provided moderate favorable condition towards plant growth. This category accounted for 44, 411.7 ha (12.5%). These areas are moderate to low fertile, not with adequate soil moisture except during rainy seasons; sign of soil erosion is common due to moderate slope. There is serious need of water conservation practice to make it utilizable. These areas can be used for silvi-pastural system and plantation of species such as Acacia nilotica with soil binder grasses is likely to produce the desired results.
4.3.3. Low
These areas exhibit low soil nutrient, wetness, rainfall, steep slope and high elevation which provides the least favorable condition for the plant growth. It accounted for 29, 441.7 ha (8.3%) and it mostly comprises of highly exposed rocks with eroded sloppy waste land. Further, identification of agroforestry suitability zones was done in block wise manner (Table 7). Blocks were rated in terms of agroforestry suitability percentage, which varied from Sonua (36.1%), Anandpur, Goelkera, Chakadharpur, Manoharpur, Gudri and Bandgaon (4.0%), showing a decreasing trend. Similarly prioritization was done for three watersheds of the part of the study area. Watershed-2 has the highest percent of agroforestry suitable area whereas Watershed-3 retains the least percent of agroforestry suitable area (Table 5).
The village buffer of 1 km against 22 points showed 39.1% of open area is highly suitable for agroforestry whereas village buffer of 2 km showed 33.7% of open area is highly suitable for the agroforestry. Tribal and backward people living in this proximity, struggling for their livelihood and are suffering from extreme poverty will have grandeur opportunity to extract agroforestry modules, which will not only provide employment opportunities but a sustainable means of socio-economic growth as well, if supplemented with adequate intervention of government agencies.
4.4. Agroforestry (AGF) modules
The present study has given an opportunity to implement well designed various agroforestry modules. These modules are derived based on regional/national agroforestry practices among this few modules are presented in World Congress on Agroforestry. However, AGF modules and its suitability to the specific area should be validated before implementing and adapting to the specific area. The idea is to facilitate both administration and implementing authorities as well as farmers. The current study is the first step in providing valuable and accurate information to initiate agroforestry practices at suitable sites in the state of Jharkhand, which is one of the agriculture dominated States.
Following agroforestry modules were considered by the Jharkhand Forest Department and other independent studies.
4.4.1. Module based on silk host tree
In Jharkhand, mostly Tassar silk is reared, which is an important forest variety produced by a wild silkworm of Antheraea mylitta, which feeds primarily on Asan (Terminalia tomentosa), Arjun (Terminalia arjuna), Shorearo busta, Lagerstroemia parviflora, and Zizyphus mauritiana trees [43].
4.4.2. Module based on lac host tree
Lac is a natural resin secreted by an insect, Kerrial acca (Kerr.), cultivated on host trees like Palas (Butea monosperma), Kusum (Schleichera oleosa) and Zizyphus mauritiana trees [43].
4.4.3. Module based on the leguminous trees
From soil nitrogen map, it is clear that nitrogen is present in abundance, which gives greater opportunity to explore leguminous trees and plants. These are a good source of nitrogen in agroforestry practices and play a significant role in the soil improvement. Nitrogen fixing trees and shrubs growing within agroforestry system are capable of fixing about 50–100 kg N/ha/year. The nitrogen returned in the litter and pruning may be 100–300 kg N/ha/year [40,44].
4.4.4. Agroforestry with papaya as a horticulture crop
Krishi Gram Vikas Kendra (KGVK), Ranchi grew Taiwanese variety of papaya, which is a case study for peasants and agricultural researchers across the State. The success story of this species will revolutionize papaya cultivation in Jharkhand as per the experts in the farming field technology. A total of 1235 plants could be grown on one acre land, which is capable of yielding a minimum of 50–60 kg fruit per plant. After all the permutation and combination as per the estimate a farmer can earn a profit of at least 1/10 million Rupees per season [45].
4.4.5. Agri-silviculture of bamboo
Bamboo can be cultivated both in hilly areas and in villages along the water streams and irrigation drainage channels where the moisture is adequate. The beginning of the rainy season is the suitable period for the plantation of propagules in the field. It provides fodder to animal in winter and gives tremendous opportunity for cottage industry [46].
4.4.6. AGF module: silvi-pastural system
Acacia nilotica (Kikar) for tannin and gums and Grewia optiva (Beul) for fodder and fiber are well known species. Major activity in the agricultural sector is concerned with the livestock rearing and fodder cultivation. In such circumstance, silvi-pasture on open degraded land and marginal lands could be an alternative to feed livestock. A combination of herbs, shrubs and fodder trees could be a sustainable source of fodder for the cattle [46].
5. Conclusion
This research shows the potentiality of remote sensing data (coarse resolution data) in studying agroforestry practices and in estimating the prominent factors for its optimal productivity. The use of ancillary data in GIS domain can have enormous potential to map the land for the benefit of rural people even up to the village level. This was only possible due to free availability of various data sets such as RS and ancillary data. A systematic use of Google Earth map itself provides a great help at each stage of the workflow. The advancement in computer science and its various modules/ algorithms such as image processing techniques and GIS toward mapping enable to derive a logical conclusion for various research problems.
Agroforestry suitability maps were produced in open area of Chakardharpur subdivision of west Singhbhum District and 21.6% of the total study area was found in high suitability zone. Among all the seven administrative blocks of the subdivision, Sonua shows highest percentage of high agroforestry suitability. Further, proximity towards agroforestry suitable area with respect to village area was evaluated as the inhabitants are end users. Buffers generated at 1 and 2 km of village points were found to be 39.1 and 33.7% of the open area suitable for agroforestry practices. The research finding leads a great hope for the agroforestry to the tribal villagers if it is supported financially along with technology by Government, NGO/s, World Bank and other international donor.
The research findings have greater importance as India has adopted agroforestry policy and the agroforestry policy makers need such data for implementation and extension of various agroforestry projects. The suitability mapping was done based upon similar guidelines of FAO for land suitability. There is an emergent need to generate, evaluate and to implement such data set from state level to village level for the microscopic study. High resolution satellite data sets with better DEM quality and accurate ancillary data sets will certainly provide better result up to the village level at the map scale of 1:5000. Various recommended regional agroforestry modules based on the ground survey must be evaluated carefully before implementing it in the entire area. Research is needed in various agroforestry modules for better suitability and adaptability for the study area.
Conflict of interest
The authors declare that they have no competing interests.









 DownLoad:
DownLoad: