Lifespan estimate of solution to the semilinear wave equation with damping term and mass term

1.   Introduction

Our main goal of the present work is to investigate the following semilinear wave equations with damping term and mass term, namely

and

where $\Delta = \sum\limits _{i = 1}^3\frac{\partial^2}{\partial x_{i}^2}$. The coefficients $b_{1}(t)\in C([0, \infty))\cap L^{1}([0, \infty))$, $b_{2}(t) = \frac{\nu_{0}}{(1+t)^{\beta+1}}\, (\nu_{0} > 0, \, \beta > 1)$ are non-negative functions. $\mu, \, \nu\geq0$. We set $f(u, \, u_{t}) = |u|^{p}$, $|u_{t}|^{p}$, $|u_{t}|^{p}+|u|^{q}$ in problem (1.1) and $f(u, \, u_{t}) = |u_{t}|^{p}+|u|^{q}$ in problem (1.2), respectively. The exponents of nonlinear terms satisfy $1 < p, \, q < \infty$. Let $\Omega = B_{1}(0) = \{x\in \mathbb R^3\big|\, |x|\leq1\}$ and $\Omega ^c = \mathbb R ^3\backslash B_{1}(0)$. $\Omega ^c$ and $\partial \Omega^c$ are smooth and compact. Initial values satisfy $f (x), \, g(x)\in C^{\infty}(\Omega^c)$ and $\mbox{supp} \, (f(x), \, g(x))\subset \Omega ^c\cap B_{R}(0)$, where $B_{R}(0) = \{x\big|\, |x|\leq R\}$, $R > 2$. The small parameter $\varepsilon > 0$ describes the size of initial values. $\frac{\partial u}{\partial n}$ stands for the derivative of external normal direction. It is well known that a solution $u$ has compact support when the initial values have compact supports. As a consequence, we directly suppose that the solution has compact support set.

We briefly review several previous results concerning problem (1.1) with $b_{1}(t) = b_{2}(t) = 0$. It is worth pointing out that the Cauchy problem with $f(u, \, u_{t}) = |u|^{p}$ asserts the Strauss exponent $p_{c}(n)$ (see ^{[31,40,41,42]}), which is the positive root of quadratic equation

The Cauchy problem with $f(u, \, u_{t}) = |u_{t}|^{p}$ admits the Glassey exponent $p_{G}(n) = \frac{n+1}{n-1}$, which has been investigated in ^[14,19]. Ikeda et al. ^[15] establish blow-up dynamic and lifespan estimate of solution to the semilinear wave equation and related weakly coupled system by using a framework of test function approach. The Cauchy problem with $f(u, \, u_{t}) = |u_{t}|^{p}+|u|^{q}$ is discussed in Han et al. ^[13]. Upper bound lifespan estimate of solution is illustrated by making use of test function method and the Kato lemma.

Recently, many researchers have been devoted to the study of Cauchy problem for semilinear wave equation

where $f(u, \, u_{t}) = |u|^{p}, \, |u_{t}|^{p}, \, |u_{t}|^{p}+|u|^{q}$. Problem (1.3) with damping term $g(u_{t}) = u_{t}, \, \frac{\mu}{ 1+t }u_{t}, \, \frac{\mu}{(1+t)^{\beta}}u_{t}\, (\beta > 1), \, (-\Delta)^{\delta}u_{t}\, (\delta\in(0, \frac{1}{2}]), \, a(x)u_{t}\, (a(x)\in C(\mathbb{R}^{n}))$ and power nonlinear term $f(u, \, u_{t}) = |u|^{p}$ is considered in ^{[6,9,18,24,27,30,38]}. Lai et al. ^[27] derive upper bound lifespan estimate of solution to problem (1.3) with damping term $g(u_{t}) = \frac{\mu}{1+t}u_{t}$ by exploiting the Kato lemma. Imai et al. ^[18] investigate problem (1.3) with scale invariant damping in two dimensions. Blow-up result and lifespan estimate of solution are discussed under certain restriction on the constant $\mu$. Applying test function approach and imposing certain integral sign conditions on the initial values, Georgiev et al. ^[9] illustrate blow-up result of solution to problem (1.3) with $g(u_{t}) = u_{t}$ on the Heisenberg group when $1 < p < p_{F}(n)$. Wakasa et al. ^[38] consider formation of singularity of solution to problem (1.3) with scattering damping $\frac{\mu}{(1+t)^{\beta}}u_{t}\, (\beta > 1)$. Lifespan estimate of solution to the variable coefficient wave equation in the critical case is analyzed by employing rescaled test function method and iteration technique, which has been utilized in ^[39]. Problem (1.3) with damping term $g(u_{t}) = \frac{\mu}{(1+t)^{\beta}}u_{t}\, (\beta > 1), \, \frac{\mu}{(1+|x|)^{\beta}}u_{t}\, (\beta > 2), \, \mu(-\Delta)^{\frac{\sigma}{2}}u_{t}\, (\mu > 0, \, 0 < \sigma\leq2)$ and derivative type nonlinear term $f(u, \, u_{t}) = |u_{t}|^{p}$ is considered in ^[7,25,28]. Lai et al. ^[25] derive upper bound lifespan estimate of solution to problem (1.3) with scattering damping term $g(u_{t}) = \frac{\mu}{(1+t)^{\beta}}u_{t}\, (\beta > 1)$ in the sub-critical and critical cases by introducing a bounded multiplier. Lai et al. ^[28] verify blow-up and lifespan estimate of solutions to problem (1.3) with space dependent damping term $g(u_{t}) = \frac{\mu}{(1+|x|)^{\beta}}u_{t}\, (\beta > 2)$ in the case $1 < p\leq p_{G}(n) = \frac{n+1}{n-1}$ by utilizing test function method ($\Psi = \partial_{t}\psi = \partial_{t}(-\eta_M^{2p'}(t)e^{-t}\phi_{1}(x))$). Dao et al. ^[7] investigate formation of singularity of solution to problem (1.3) with structural damping term $g(u_{t}) = \mu(-\Delta)^{\frac{\sigma}{2}}u_{t}\, (\mu > 0, \, 0 < \sigma\leq2)$ and derivative nonlinearity. Problem (1.3) with damping term $g(u_{t}) = \frac{\mu}{ 1+t }u_{t}, \, \frac{\mu}{(1+t)^{\beta}}u_{t}\, (\beta > 1)$ and combined nonlinearities $f(u, \, u_{t}) = |u_{t}|^{p}+|u|^{q}$ is illustrated in ^{[12,26,32,33]}. Applying the rescaled test function approach and iterative method, Ming et al. ^[33] establish upper bound lifespan estimate of solution to problem (1.3) with scattering damping and divergence form nonlinearity in the sub-critical and critical cases. Hamouda et al. ^[12] illustrate influence of scale invariant damping on the formation of singularity of solution. Lifespan estimate of solution is derived by imposing certain assumptions on the parameter $\mu$. Liu and Wang ^[32] consider blow-up of solution to the semilinear wave equation with combined nonlinearities on asymptotically Euclidean manifolds in the case $n = 2, \, \mu = 0$.

Scholars focus widespread attention on the Cauchy problem for semilinear wave equation with damping term and mass term (see detailed illustrations in ^{[1,4,11,17,22,36,37]}). Taking advantage of the iteration method, Lai et al. ^[22] establish blow-up result of solution to the semilinear wave equation with scattering damping term and negative mass term, where the nonlinearity is $|u|^{p}$. Ikeda et al. ^[17] investigate lifespan estimate of solution to the semilinear wave equation with damping term, mass term as well as power nonlinearity in the sub-critical and critical cases by utilizing test function approach ($\psi(x, t) = \rho(t)\phi_{1}(x)$), which is inspired by ^[36]. Lai et al. ^[23] derive upper bound lifespan estimate of solution to the semilinear wave equation with damping term and mass term by employing the Kato lemma and iteration approach. Blow-up phenomenon and lifespan estimate of solution to the semilinear wave equation with scale invariant damping, non-negative mass term and power type of nonlinear term are documented in ^[36], where the iteration method is performed. Hamouda et al. ^[11] show blow-up dynamic of solution to the semilinear wave equation with scale invariant damping, mass term and combined nonlinearities. The proof is based on the multiplier technique and solving the ordinary differential inequality. We refer readers to the works in ^{[2,3,5,8,10,16,20,21,29,34,35]} for more details.

Enlightened by the works in ^{[11,17,22,24,25,26,36]}, our interest is to show blow-up results of solutions to problems (1.1) and (1.2) with Neumann boundary conditions on exterior domain in three dimensions. It is worth pointing out that upper bound lifespan estimates of solutions to the Cauchy problem of semilinear wave equation with scattering damping term $\frac{\mu}{(1+t)^{\beta}}u_{t}\, (\mu > 0, \, \beta > 1)$ and nonlinear terms $|u|^{p}$, $|u_{t}|^{p}$, $|u_{t}|^{p}+|u|^{q}$ are discussed in ^[24,25,26]. Lai et al. ^[22] derive blow-up and lifespan estimate of solution to the semilinear wave equation with scattering damping and negative mass term by exploiting the test function technique and iterative approach, where the nonlinear term is $|u|^{p}$. However, there is no related result about blow-up dynamic of solution to problem (1.1). Thus, we extend the Cauchy problem studied in ^[24,25,26] to problem (1.1) with damping term, negative mass term and Neumann boundary condition on exterior domain in three dimensions. Upper bound lifespan estimate of solution to problem (1.1) is established by making use of a radial symmetry test function $\psi(x, t) = e^{-t}\frac{1}{r}e^{r}$ with $r = \sqrt{x_{1}^{2}+x_{2}^{2}+x_{3}^{2}}$ (see Theorems 1.1, 1.3–1.5). The Cauchy problem investigated in ^[23] is extended to problem (1.1) by utilizing the test function method $(\psi(x, t) = e^{-t}\frac{1}{r}e^{r})$ and the Kato lemma (see Theorem 1.2). We derive lifespan estimate of solution to problem (1.1) with $f(u, \, u_{t}) = |u|^{p}$ (see Theorem 1.6) by taking advantage of the test function approach $(\psi_{1}(x, t) = \rho (t)\frac{1}{r}e^{r})$, which is inspired by the work ^[17]. Making use of a multiplier, Hamouda et al. ^[11] verify blow-up phenomenon of solution to the semilinear wave equation with scale invariant damping and mass term as well as combined nonlinearities. We extend the problem discussed in ^[11] to problem (1.2). Upper bound lifespan estimate of solution to problem (1.2) with combined nonlinearities $f(u, \, u_{t}) = |u_{t}|^{p}+|u|^{q}$ is acquired by applying the test function technique $(\psi_{2}(x, t) = \rho_{1} (t)\frac{1}{r}e^{r})$ and iterative method (see Theorem 1.7). To the best of our knowledge, the results in Theorems 1.1–1.7 are new. Moreover, we characterize the variation of wave by utilizing numerical simulation.

Definitions of weak solutions and the main results in this paper are illustrated as follows.

Definition 1.1. A function $u$ is called a weak solution of problem (1.1) on $[0, T)$ if $u\in C([0, T), H^{1}(\Omega^{c}))\cap C^{1}([0, T), L^{2}(\Omega^{c}))\cap L^{p}_{loc}((0, T)\times\Omega^{c})$ when $f(u, \, u_{t}) = |u|^{p}$, $u\in C([0, T), H^{1}(\Omega^{c}))\cap C^{1}([0, T), L^{2}(\Omega^{c}))\cap C^{1}((0, T), L^{p}(\Omega^{c}))$ when $f(u, \, u_{t}) = |u_{t}|^{p}$, $u\in \cap_{i = 0}^{1}C^{i}([0, T), H^{1-i}(\Omega^{c}))\cap C^{1}((0, T), L^{p}(\Omega^{c}))\cap L^{q}_{loc}((0, T)\times\Omega^{c})$ when $f(u, \, u_{t}) = |u_{t}|^{p}+|u|^{q}$ and

where $\phi\in C^{\infty}_{0}([0, T)\times \Omega^{c})$ and $t\in [0, T)$.

Definition 1.2. A function $u$ is called a weak solution of problem (1.2) on $[0, T)$ if $u\in C([0, T), H^{1}(\Omega^{c}))\cap C^{1}([0, T), L^{2}(\Omega^{c}))$, $u\in L_{loc}^{q}((0, T)\times \Omega^{c})$, $u_{t}\in L_{loc}^{p}((0, T)\times \Omega^{c})$ when $f(u, \, u_{t}) = |u_{t}|^{p}+|u|^{q}$ and

where $\phi\in C^{\infty}_{0}([0, T)\times \Omega^{c})$ and $t\in [0, T)$.

Setting

we rewrite Definition 1.2 by choosing $m(t)\phi(x, t)$ as a test function.

Definition 1.3. A function $u$ is called a weak solution of problem (1.2) on $[0, T)$ if $u\in C([0, T), H^{1}(\Omega^{c}))\cap C^{1}([0, T), L^{2}(\Omega^{c}))$, $u\in L_{loc}^{q}((0, T)\times \Omega^{c})$, $u_{t}\in L_{loc}^{p}((0, T)\times \Omega^{c})$ when $f(u, \, u_{t}) = |u_{t}|^{p}+|u|^{q}$ and

where $\phi\in C^{\infty}_{0}([0, T)\times \Omega^{c})$ and $t\in [0, T)$.

Theorem 1.1. Let $1 < p < p_{c}(3)$. Assume that $(f, g)\in H^1(\Omega ^c)\times L^2(\Omega^c)$ are non-negative functions and $f$ does not vanish identically. If a solution $u$ to problem (1.1) with $f(u, \, u_{t}) = |u|^{p}$ satisfies $\mathit{\mbox{supp}} \, (u, \, u_t) \subset\{(x, t)\in\Omega^{c}\times[0, T)\big |\, |x|\leq t+R\}$, then $u$ blows up in finite time. Moreover, there exists a constant $\varepsilon_{0} = \varepsilon_{0}(f, \, g, \, R, \, p, \, b_{1}(t), \, b_{2}(t)) > 0$ such that the lifespan estimate $T(\varepsilon)$ satisfies

where $0 < \varepsilon\leq \varepsilon_0$, $C > 0$ is independent of $\varepsilon$.

Theorem 1.2. Assume $b_{1}(t) = \frac{\nu_{1}}{(1+t)^{\beta}}$, $b_{2}(t) = \frac{\nu_{2}}{(1+t)^{2}}$, $\nu_{1}\geq 0$, $\beta > 1$, $\nu_{2} > 0$. Let $\delta = 1+4\nu_{2}e^{\frac{\nu_{1}}{1-\beta}} > 1$, $d_{\ast}(3) = 2\sqrt{2}-2\in [0, 2)$, $1 < p < p_{\delta}(3)$ and

Here, $p_{F}(n) = 1+\frac{2}{n}$ is the solution of equation $r_{F}(p, n) = 2-n(p-1) = 0$. Suppose that $(f, g)\in H^1(\Omega ^c)\times L^2(\Omega^c)$ are non-negative functions and do not vanish identically. If a solution $u$ to problem (1.1) with $f(u, \, u_{t}) = |u|^{p}$ satisfies $\mathit{\mbox{supp}} \, (u, \, u_t) \subset\{(x, t)\in\Omega^{c}\times[0, T)\big |\, |x|\leq t+R\}$, then $u$ blows up in finite time. Moreover, the lifespan estimate $T(\varepsilon)$ satisfies

where $C > 0$ is independent of $\varepsilon$.

Theorem 1.3. Let $1 < p\leq p_{G}(3) = 2$. Assume that $(f, g)\in H^1(\Omega ^c)\times L^2(\Omega^c)$ are non-negative functions and $g$ does not vanish identically. If the solution $u$ to problem (1.1) with $f(u, \, u_{t}) = |u_{t}|^{p}$ satisfies $\mathit{\mbox{supp}} \, (u, \, u_t) \subset\{(x, t)\in\Omega^{c}\times[0, T)\big |\, |x|\leq t+R\}$, then $u$ blows up in finite time. Moreover, the lifespan estimate $T(\varepsilon)$ satisfies

where $C > 0$ is independent of $\varepsilon$.

Theorem 1.4. Let $p > 1$ and $1 < q < {\min}\, \{1+\frac{2}{p-1}, \, 6\}$. Assume that $f$ and $g$ satisfy the conditions in Theorem 1.3. If a solution $u$ to problem (1.1) with $f(u, \, u_{t}) = |u_{t}|^{p}+|u|^{q}$ satisfies $\mathit{\mbox{supp}} \, (u, \, u_{t}) \subset\{(x, t)\in\Omega^{c}\times[0, T)\big |\, |x|\leq t+R\}$, then $u$ blows up in finite time. Moreover, the lifespan estimate $T(\varepsilon)$ satisfies

where $C > 0$ is independent of $\varepsilon$.

Theorem 1.5. Let $p > 3$ and $1 < q < 2$. Assume that $f$ and $g$ satisfy the conditions in Theorem 1.3. If the solution $u$ to problem (1.1) with $f(u, \, u_{t}) = |u_{t}|^{p}+|u|^{q}$ satisfies $\mathit{\mbox{supp}} \, (u, \, u_{t}) \subset\{(x, t)\in\Omega^{c}\times[0, T)\big |\, |x|\leq t+R\}$, then $u$ blows up in finite time. Moreover, the lifespan estimate $T(\varepsilon)$ satisfies

where $C > 0$ is independent of $\varepsilon$.

Theorem 1.6. Let $1 < p < p_{c}(3)$. Let $f$ and $g$ satisfy the conditions in Theorem 1.1. Suppose that $b_{1}(t)\in C^{1}([0, \infty))$ and $r_{2}(t)\in L^{1}([0, \infty))$ satisfy

$\rho'(0)$ is the initial value of $\rho'(t)$, where $\rho(t)$ is the solution to problem (5.1). It holds that

There is no sign requirement for $b_{1}(t)$ and $b_{2}(t)$. If a solution $u$ to problem (1.1) with $f(u, u_{t}) = |u|^{p}$ satisfies $\mathit{\mbox{supp}} \, (u, \, u_{t}) \subset\{(x, t)\in\Omega^{c}\times[0, T)\big |\, |x|\leq t+R\}$, then $u$ blows up in finite time. Moreover, the lifespan estimate $T(\varepsilon)$ satisfies

where $C > 0$ is independent of $\varepsilon$.

Theorem 1.7. Let $p > p_{G}(3+\mu)$, $q > q_{S}(3+\mu)$, $\mu, \, \nu^{2}\geq0$ and $\delta = (\mu-1)^{2}-4\nu^{2}\geq0$. Assume that $\lambda(p, \, q, \, 3+\mu) < 4$, where $\lambda(p, \, q, \, n) = (q-1)((n-1)p-2) < 4$. The initial values $(f, g)\in H^1(\Omega ^c)\times L^2(\Omega^c)$ are non-negative functions which do not vanish identically and satisfy

If a solution $u$ to problem (1.2) with $f(u, \, u_{t}) = |u_{t}|^{p}+|u|^{q}$ satisfies $\mathit{\mbox{supp}} \, (u, \, u_{t}) \subset\{(x, t)\in\Omega^{c}\times[0, T)\big |\, |x|\leq t+R\}$, then $u$ blows up in finite time. Moreover, there exists a constant $\varepsilon_{0} = \varepsilon_{0}(f, \, g, \, R, \, p, \, q, \, \mu, \, \nu) > 0$ such that the lifespan estimate $T(\varepsilon)$ satisfies

where $C > 0$ is independent of $\varepsilon$.

Remark 1.1. Utilizing the Sobolev embedding theorem yields $H^1(\Omega^{c})\hookrightarrow L^q(\Omega^{c})$ when $n = 3, \, q < 6$ in Theorems 1.4 and 1.5. Consequently, the nonlinear term $|u|^{q}$ in problem (1.1) is integrable in the domain $\Omega^{c}\subset\mathbb R^3$.

Remark 1.2. Taking advantage of the Poincare's inequality, we conclude

Similar to the proof of Theorem 1.1, we obtain the same result in (1.7) when nonlinear term is $f(u, \, u_{t}) = |\nabla u|^{p}$.

Remark 1.3 We call that $u$ is a global solution of problems (1.1) and (1.2) if the maximal existence time of solution $T_{max} = \infty$. While in the case $T_{max} < \infty$, we call that $u$ blows up in finite time.

2.   Proofs of Theorems 1.1 and 1.2

2.1.   Several related lemmas

Lemma 2.1. ^[35] Let $b_{1}(t)\in C([0, \infty))\cap L^{1}([0, \infty))$ be a non-negative function, which satisfies

$m_{1}(0)\leq m_{1}(t)\leq1$, $\frac{m'_{1}(t)}{m_{1}(t)} = b_{1}(t)$ for $t\geq0$.

Lemma 2.2. Let $\phi_1(x) = \phi_1(r) = \frac{1}{r}e^r$, where $x = (x_1, x_2, x_3)$ and $r = \sqrt{x_1^2+x_2^2+x_3^2}$. It holds that

and $\frac{\partial \phi_1}{\partial r}|_{r = 1} = 0$. Setting $\psi = e^{-t}\phi_{1}(x)$, it satisfies

where $C$ is a positive constant.

Proof of Lemma 2.2. Direct calculation shows

where $i = 1, \, 2, \, 3$. Thus, we obtain

Employing $\psi = e^{-t}\frac{1}{r}e^r$ gives rise to

We complete the proof of Lemma 2.2.

2.2.   Proof of Theorem 1.1

Let us set three functions

where $\psi(x, t) = e^{-t}\phi_{1}(x) = e^{-t}\frac{1}{r}e^{r}$. It holds that

By straightforward computation, we achieve

Choosing the test function $\phi(x, s)\equiv1$ on $(x, s)\in \{\Omega^{c}\times [0, t] \big|\, |x|\leq s+R\}$ in (1.4) with $f(u, \, u_{t}) = |u|^{p}$ and utilizing (2.2) yield

Multiplying (2.3) with $m_{1}(t)$ and integrating on $[0, t]$, we deduce

where we have used the fact $F'_{0}(0)\geq0$ and $F_{0}(t) > 0$.

We are in the position to establish the lower bound of $F_{1}(t)$. Elementary computation leads to

Applying (2.5) and the Green formula yields

Thus, we have

Utilizing (2.1), (2.6) and replacing $\phi(x, s)$ in (1.4) with $f(u, \, u_{t}) = |u|^{p}$ by $\psi(x, s)$, we obtain

That is

which leads to

where $C_{f, g} = \int_{\Omega^{c}}\{f(x)+g(x)\}\phi_{1}(x)dx > 0$.

Thanks to the positivity of $F_{1}(t)$ and $F_{1}(0)$, we deduce

where $t > 2$. Employing (2.4) and the Holder inequality yields

Making use of the Holder inequality and Lemma 2.2 gives rise to

Taking advantage of (2.4), (2.7) and (2.9), we acquire

We denote

where

Combining (2.8) with (2.10), we derive

Thus, we define the sequences $\{D_{j}\}_{j\in\mathbb{N}}, \, \{a_{j}\}_{j\in\mathbb{N}}, \, \{b_{j}\}_{j\in\mathbb{N}}$ by

Exploiting (2.11), (2.12) and iterative argument gives rise to

where $S_{p}(\infty)$ is obtained by using the d'Alembert's criterion. Moreover, $S_{p}(j) = \sum\limits^{j-1}_{k = 1}\frac{2k\log p-\log C_{3}}{p^{k}}$ converges to $S_{p}(\infty)$ as $j\rightarrow \infty$. As a consequence, making use of (2.10) yields

and

where $C_{4} = (p+3)\log2+S_{p}(\infty) > 0$ and $t\geq R > 2$. Utilizing the condition $p < p_{c}(3)$, we arrive at $J(t) > 1$ when $t\geq C_{5}\varepsilon^{-\frac{2p(p-1)}{r(p, 3)}}$. Sending $j\rightarrow \infty$ in (2.13) yields $F_{0}(t)\rightarrow \infty$. Therefore, we derive the lifespan estimate

The proof of Theorem 1.1 is finished.

2.3.   Proof of Theorem 1.2

Integrating (2.3) on $[0, t]$, we acquire

Let us define two functions

and

Thanks to $m_{1}(0) < m_{1}(t) < 1$ and $\nu_{2} > 0$, we achieve

Applying comparison argument, we conclude $F_{0}(t)\geq\widetilde{F_{0}}(t)$. Employing (2.15) and the formula (4.2) with $\mu_{1} = 0, \, \mu_{2} = -m_{1}(0)\nu_{2}$ in ^[23] gives rise to

Similar to the derivation in the proof of Theorem 5 in ^[23], we derive

and

Here, $A\gtrsim B$ means that there exists a positive constant $C$ such that $A\geq CB$. Taking into account (2.7) and the Holder inequality, we obtain

which together with (2.17) results in

where $t\geq T_{1} > 0$, $q = -\frac{1+\sqrt{\delta}}{2}-p+4$. Therefore, we arrive at

Utilizing (2.18)–(2.20) and the Kato lemma in Sub-section 4.3 in ^[23], we finishes the proof of Theorem 1.2.

3.   Proof of Theorem 1.3

Direct computation gives rise to

Making use of (1.4) and (2.6), we acquire

Plugging (3.2) into (3.1) yields

which together with (2.7) results in

Combining (1.4), (2.1) and (2.6), we have

An application of (3.4) and (3.5) gives rise to

We set

where $G(0) = \frac{m_{1}(0)\varepsilon}{2}\int_{\Omega^{c}}g(x)\phi_{1}(x)dx > 0$. Taking into account (3.6), we acquire

It follows that $G(t)\geq e^{-2t}G(0) > 0$ for $t\geq0$. Thus, we conclude

We define

Applying the Holder inequality and (3.8) yields

As a direct consequence, we have

It is worth noticing that $H(0) = \frac{m_{1}(0)}{2}\varepsilon\int_{\Omega^{c}}g(x)\phi_{1}(x)dx$. Therefore, employing the assumption $1 < p\leq2$, we derive the lifespan estimate in Theorem 1.3. The proof of Theorem 1.3 is finished.

4.   Proofs of Theorems 1.4 and 1.5

4.1.   Proof of Theorem 1.4

We are in the position to establish the estimate of $F_{0}(t)$. Choosing the test function $\phi(x, t) = 1$ in (1.4) yields

Multiplying (4.1) with $m_{1}(t)$ and integrating on $[0, t]$ yield

where we have applied the fact $F'_{0}(0)\geq0$ and $F_{0}(t) > 0$.

Similar to the estimates in (2.7) and (3.8), we obtain the estimates

when nonlinear term is $f(u, \, u_{t}) = |u_{t}|^{p}+|u|^{q}$. Taking advantage of Lemma 2.2 and (3.8), we derive

where $\overline{C}_1 = C^{1-p}(\frac{m_{1}(0)}{2}\int_{\Omega^{c}}g(x)\phi_{1}(x)dx)^p$. Plugging (4.3) into (4.2) leads to

Recalling (4.2), we acquire

We set

where

Inserting (4.6) into (4.5), we come to the estimate

Therefore, we denote the sequences $\{D_{j}\}_{j\in\mathbb{N}}, \, \{a_{j}\}_{j\in\mathbb{N}}, \, \{b_{j}\}_{j\in\mathbb{N}}$ by

Taking advantage of (4.7), (4.8) and iterative argument gives rise to

where $S(j) = \sum\limits^{j-1}_{k = 1}\frac{2k\log q-\log \overline{C}_{4}}{q^{k}}$ converges to $S(\infty)$ as $j\rightarrow \infty$.

From (4.6), we have

and

where $\overline{C}_{5} = (p+3)\log2+S(\infty) > 0, \, \, t\geq R > 2$. Recalling the assumption $q < 1+\frac{2}{p-1}$, we deduce that $J(t) > 1$ when $t > \overline{C}_{6}\varepsilon^{\frac{-p(q-1)}{q+1-p(q-1)}}$. Sending $j\rightarrow \infty$ in (4.9) yields $F_{0}(t)\rightarrow \infty$. Therefore, we achieve the lifespan estimate

The proof of Theorem 1.4 is finished.

4.2.   Proof of Theorem 1.5

We set $I[f] = \int_{\Omega^{c}}f(x)dx$. Utilizing (4.4) gives rise to

for sufficiently large $t$, where $C > 0$ is independent of $\varepsilon$. Thus, we deduce that (4.4) is weaker than the linear growth when $p > 3$. An application of (4.2) leads to

That is

It is deduced from (4.5) and (4.11) that

We assume

where

Plugging (4.12) into (4.5), we derive

with

Making use of (4.13) and (4.15), we conclude

Applying (4.12) gives rise to

and

Bearing in mind $1 < q < 2$, we arrive at the lifespan estimate in Theorem 1.5. This completes the proof of Theorem 1.5.

5.   Proof of Theorem 1.6

To outline the proof of Theorem 1.6, we recall the following Lemmas.

Lemma 5.1. ^[17] Let $\rho(t)$ be a solution of the second order ODE

where $\rho(t)$ decays as $e^{-t}$ for large $t$.

Lemma 5.2. Let $\phi_1(x) = \phi_1(r) = \frac{1}{r}e^r$, where $x = (x_1, x_2, x_3)$ and $r = \sqrt{x_1^2+x_2^2+x_3^2}$. Setting $\psi_{1}(x, t) = \rho(t)\phi_{1}(x)$, it holds that

where $\rho(t)\sim e^{-t}$, $C$ is a positive constant.

Proof of Lemma 5.2. Taking into account $\psi_{1} = \rho(t)\frac{1}{r}e^r$, we obtain

We finish the proof of Lemma 5.2.

Proof of Theorem 1.6. Let us define the functions

where $\psi_{1}(x, t) = \rho(t)\phi_{1}(x)$.

Choosing the test function $\phi(x, s)\equiv1$ on $\{(x, s)\in\Omega^{c}\times[0, t]\big|\, |x|\leq s+R\}$ in (1.4) with $f(u, \, u_{t}) = |u|^{p}$, we have

We rewrite (5.2) into the form

where $r_{1}(t)$ and $r_{2}(t)$ satisfy

Multiplying both sides of (5.3) by $\exp \int^{s_{1}}_{s_{2}}r_{1}(\tau)d\tau$, integrating over $[0, s_{2}]$ and applying $g(x)+r_{2}(0)f(x)\geq 0$ yield

Multiplying (5.4) by $\exp \int^{s_{2}}_{t}r_{2}(\tau)d\tau$ leads to

Replacing $\phi(x, s)$ with $\psi_{1}(x, s)$ in (1.4) in the case $f(u, \, u_{t}) = |u|^{p}$ and employing (2.6), we derive

Employing Lemma 5.1 and (5.6), we deduce

where $C_{f, \, g} = \int_{\Omega^{c}}(g(x)+(b_{1}(0)-\rho'(0))f(x))\phi_{1}(x)dx > 0$.

Multiplying (5.7) with $\frac{1}{\rho^{2}(t)}e^{\int^{t}_{0}b_{1}(\tau)d\tau}$ yields

Utilizing Lemma 5.2 gives rise to

where $\langle t\rangle = 3+|t|$. Taking advantage of (5.5) and Lemma 2.1 in ^[17] leads to

Similar to the iteration argument in Theorem 1.1, we derive the lifespan estimate in Theorem 1.6. The proof of Theorem 1.6 is finished.

6.   Proof of Theorem 1.7

6.1.   Several lemmas

Lemma 6.1. ^[11] Assume that $\rho_{1}(t)$ is solution of

The expression of $\rho_{1}(t)$ is

where $K_{\xi}(t) = \sqrt{\frac{\pi}{2t}}e^{-t}(1+O(t^{-1}))$ as $t\rightarrow \infty$ and $K'_{\xi}(t) = -K_{\xi+1}(t)+\frac{\xi}{t}K_{\xi}(t)$. It holds that

Let $\phi_1(x) = \phi_1(r) = \frac{1}{r}e^r$, where $x = (x_1, x_2, x_3)$ and $r = \sqrt{x_1^2+x_2^2+x_3^2}$. Setting $\psi_{2}(x, t) = \rho_{1}(t)\phi_{1}(x)$, it holds that

and

for some positive constant $C$.

Proof of Lemma 6.1. Applying $\psi_{2} = \rho_{1}(t)\frac{1}{r}e^r$ gives rise to

We complete the proof of Lemma 6.1.

We denote two functions

Lemma 6.2. Let $u$ be a weak solution of problem (1.2). If $(p, \, q)$ and $(f(x), \, g(x))$ satisfy the conditions in Theorem 1.7, then there exists $T_{0} = T_{0}(\mu, \nu) > 1$ such that

where $t\geq T_{0}$, $C_{G_{1}}$ is a positive constant which depends on $f, \, g, \, \mu, \, \nu$.

Proof of Lemma 6.2. Replacing $\phi(x, t)$ in (1.5) by $\psi_{2}(x, t) = \rho_{1}(t)\phi_{1}(x)$ and employing (6.3), we derive

where

Thus, we obtain

Multiplying (6.7) by $\frac{1}{\rho_{1}^{2}(t)}(1+t)^{\mu}$, integrating over $(0, t)$ and exploiting Lemma 6.1 yield

for $t > T_{0}(\mu, \nu) > 1$, where $G_{1}(0) = \varepsilon K_{\frac{\sqrt{\delta}}{2}}(1)\int_{\Omega^{c}}f(x)\phi_{1}(x)dx > 0$. This finishes the proof of Lemma 6.2.

Lemma 6.3. Let $u$ be a weak solution of problem (1.2). If $(p, \, q)$ and $(f(x), \, g(x))$ satisfy the conditions in Theorem 1.7, it holds that

where $C$ is a positive constant which depends on $p, \, f, \, g, \, R, \, \varepsilon_{0}, \, \mu$ but not on $\varepsilon, \, \nu$.

Proof of Lemma 6.3. We define two functions

Replacing $\phi(x, s)$ in (1.6) by $\psi(x, t)$ and using the fact $F'_{1}(t)+F_{1}(t) = F_{2}(t)$ lead to

where $C (f, g) = \int_{\Omega^{c}}\big(f(x)+g(x)\big)\phi_{1}(x)dx$.

Therefore, we arrive at

Combining (6.8), (6.10) and (6.11), we deduce

where $C(f, g) = \int_{\Omega^{c}}\big(f(x)+g(x) \big)\phi_{1}(x)dx$, we have applied the facts $G_{1}(t) = e^{t}\rho_{1}(t)F_{1}(t)$, $F'_{1}(t)+F_{1}(t) = F_{2}(t)$ and $m(t)\geq1$.

Taking advantage of the Holder inequality and Lemma 2.2 yields

Making use of (6.12) and (6.13), we have

As a consequent, it holds that

where we have used $G_{2}(t) = e^{t}\rho_{1}(t)F_{2}(t)$.

An application of (6.15) and the fact $\rho_{1}(t)e^{t}\leq C(1+t)^{\frac{\mu}{2}}$ gives rise to

This ends the proof of Lemma 6.3.

Lemma 6.4. Let $u$ be a weak solution of problem (1.2). If $(p, \, q)$ and $(f(x), \, g(x))$ satisfy the conditions in Theorem 1.7, then there exists $T_{1} > 0$ such that

where $C_{G_{2}}$ is a positive constant which depends on $p, \, f, \, g, \, R, \, \varepsilon_{0}, \, \nu, \, \mu$.

Proof of Lemma 6.4. Applying (6.7) and the fact $G'_{1}(t)-\frac{\rho_{1}'(t)}{\rho_{1}(t)}G_{1}(t) = G_{2}(t)$ leads to

Taking into account (6.1), (6.2), (6.18) and Lemma 6.2, we derive

where

for $t > \widetilde{T}_{1}(\mu, \nu)\geq T_{0}$,

for $t > \widetilde{T}_{2}(\mu, \nu)\geq \widetilde{T}_{1}(\mu, \nu)$.

Utilizing (6.19) and Lemma 6.3, we conclude

for $t\geq T_{1} = -\ln\varepsilon$. This completes the proof of Lemma 6.4.

6.2.   Proof of Theorem 1.7

We define the function

Choosing the test function $\phi(x, t)\equiv 1$ in (1.5) yields

Therefore, we obtain

where $r_{1} = \frac{\mu-1-\sqrt{\delta}}{2}$ and $r_{2} = \frac{\mu-1+\sqrt{\delta}}{2}$ are real roots of the quadratic equation $r^{2}-(\mu-1)r+\nu^{2} = 0$.

It is deduced from (1.8) and (6.23) that

Making use of the Holder inequality and (6.24), we acquire

where $C = (means(B_{1}))^{1-q}R^{-3(q-1)} > 0$.

Employing Lemma 6.4, (6.4) and the fact $\rho_{1}(t)e^{t}\leq C(1+t)^{\frac{\mu}{2}}$ gives rise to

Plugging (6.26) into (6.24), we deduce

for $t > T_{0}$.

We set

where

Utilizing (6.25) and (6.28), we have

We denote the sequences $\{D_{j}\}_{j\in\mathbb{N}}$, $\{a_{j}\}_{j\in\mathbb{N}}$, $\{b_{j}\}_{j\in\mathbb{N}}$ by

Taking advantage of (6.29), (6.31) and (6.32) leads to

where $S_{q}(j) = \frac{2q\log q}{(q-1)^{2}}-\frac{q\log C}{q-1}$ converges to $S_{q}(\infty)$ as $j\rightarrow \infty$.

Employing (6.28), (6.29) and (6.33)–(6.35), we achieve

and

for $t > 2T_{0}+1$. Recalling $p > p_{G}(3+\mu)$, $q > q_{S}(3+\mu)$ and $\lambda(p, q, 3+\mu) < 4$, we conclude lifespan estimate (1.9) in Theorem 1.7. This completes the proof of Theorem 1.7.

7.   Numerical simulation

We are in the position to show variation of wave for the Cauchy problem of semilinear wave equation in two dimensions. All codes are written and run with Matlab2014a on Windows 10 (64bite), RAM:8G and CPU 3.60 GHz. That is,

Suppose that the initial values satisfy

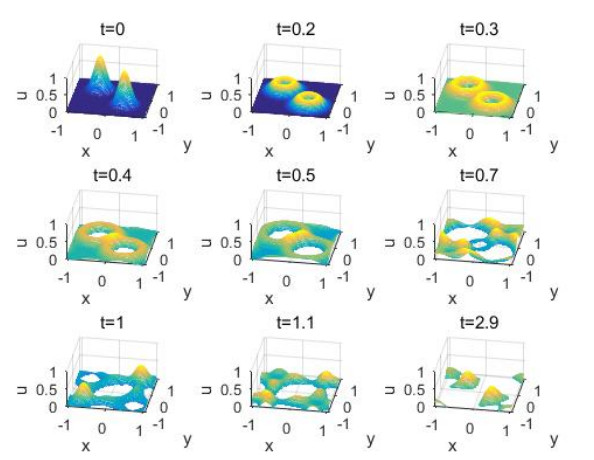
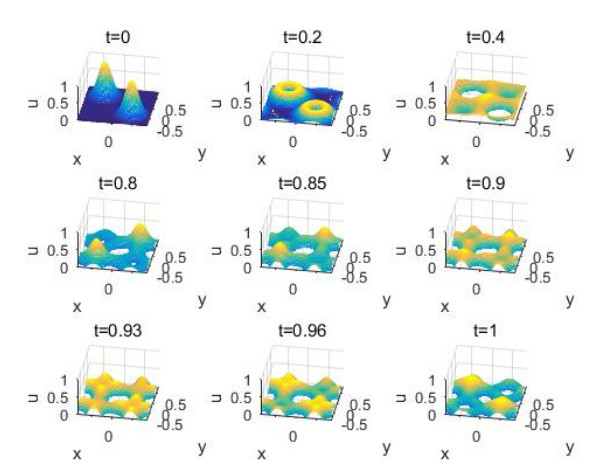
The following two group figures indicate the propagation of wave in two dimensions.

Figure 1 represents the trend of wave from $t = 0$ s to $t = 1$ s when nonlinear term is $|u|^{3}$ in problem (7.1). It indicates that there are two peaks of wave when $t = 0$ s. With the increase of time, two peaks of wave move downward until they disappear. Then, two new wave peaks appear at different positions when $t = 0.8$ s. From $t = 0.8$ s to $t = 0.9$ s, the new wave amplitudes decrease gradually. However, when $t = 0.9$ s $\sim 1$ s, the old wave amplitudes increase constantly. When $t = 1$ s, the wave peaks appear again and the position of wave peaks is same as the position of $t = 0$ s.

Figure 2 stands for the trend of wave from $t = 0$ s to $t = 2.9$ s when nonlinear term is $|u|^{3}$ in problem (7.2). When $t = 0$ s, it shows the initial state of wave with two peaks. From $t = 0$ s to $t = 0.5$ s, the wave peaks continue to drop and begin to stack when they meet. When $t = 0.5$ s $\sim 1$ s, two new waves appear at different positions and the amplitude increases continuously to form two new wave peaks. When $t = 1$ s $\sim 1.1$ s, the amplitudes of wave decreases gradually. When $t = 2.9$ s, two wave peaks appears again and the position is same as $t = 0$ s.

From our observation of the above two groups of figures, we obtain that the frictional damping and negative mass terms have an effect on the wave propagation and wave amplitude.

8.   Conclusions

This article is dedicated to investigating blow-up results and lifespan estimates of solutions to the initial boundary value problems of semilinear wave equations with damping term and mass term as well as Neumann boundary conditions on exterior domain in three dimensions. Our main new contribution is that upper bound lifespan estimates of solutions are related to the Strauss exponent and Glassey exponent. We extend the Cauchy problem investigated in the related papers to problems (1.1) and (1.2) with damping term, mass term and Neumann boundary condition on exterior domain in three dimensions. Applying test function technique ($\psi_{2}(x, t) = \rho_{1}(t)\frac{1}{r}e^{r}$ with $r = \sqrt{x_{1}^{2}+x_{2}^{2}+x_{3}^{2}}$) and iterative approach, upper bound lifespan estimates of solutions to problems (1.1) and (1.2) are deduced (see Theorems 1.1–1.7). In addition, we characterize the variation of wave by employing numerical simulation.

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The author Sen Ming would like to express his sincere thanks to Professor Yi Zhou for his guidance and encouragement during the postdoctoral study in Fudan University. The author Sen Ming also would like to express his sincere thanks to Professors Han Yang and Ning-An Lai for their helpful suggestions and discussions. The project is supported by the Fundamental Research Program of Shanxi Province (No. 20210302123021, No. 20210302123045, No. 20210302124657, No. 20210302123182), the Program for the Innovative Talents of Higher Education Institutions of Shanxi Province, the Innovative Research Team of North University of China (No. TD201901), National Natural Science Foundation of China (No. 11601446).

Conflict of interest

This work has no conflict of interest.