1.
Introduction
Let Cm×n and Z+ denote the set of all m×n complex matrices and the set of all positive integers, respectively. The symbols r(A) and Ind(A) stand for the rank and the index of A∈Cn×n, respectively. For a matrix A∈Cn×n, we assume that A0=In. Let Cn×nk be the set of all n×n complex matrices with index k. By CCMn we denote the set of all core matrices (or group invertible matrices), i.e.,
The Drazin inverse [1] of A∈Cn×nk, denoted by AD, is the unique matrix X∈Cn×n satisfying:
Especially, when A∈CCMn, then X that satisfies (1.1) is called the group inverse of A and is denoted by A#. The Drazin inverse has been widely applied in different fields of mathematics and its applications. Here we will mention only some of them. The perturbation theory and additive results for the Drazin inverse were investigated in [2,3,4,5]. In [6], the algorithms for the computation of the Drazin inverse of a polynomial matrix are presented based on the discrete Fourier transformation. Karampetakis and Stanimiroviˊc [7] presented two algorithms for symbolic computation of the Drazin inverse of a given square one-variable polynomial matrix, which was effective with respect to CPU time and the elimination of redundant computations. Some representations of the W-weighted Drazin inverse were investigated and the computational complexities of the representations were also estimated in [8]. Kyrchei [9] generalized the weighted Drazin inverse, the weighted DMP-inverse, and the weighted dual DMP-inverse [10,11,12] for the matrices over the quaternion skew field and provided their determinantal representations by using noncommutative column and row determinants. In [13], the authors considered the quaternion two-sided restricted matrix equations and gave their unique solutions by the DMP-inverse and dual DMP-inverse. For interesting properties of different kinds of generalized inverses see [14].
In 2018, Wang [15] introduced the weak group inverse of complex square matrices using the core-EP decomposition [16] and gave its certain characterizations.
Definition 1.1. Let A∈Cn×nk. Then the unique solution of the system
is the weak group inverse of A denoted by AⓌ.
Recently, there has been a huge interest in the weak group inverse. For example, Wang et al. [17] compared the weak group inverse with the group inverse of a matrix. In [18], the weak group inverse was introduced in *-rings and characterized by three equations (see also [19,20]). The weak group inverse in the setting of rectangular matrices was considered in [21]. In 2021, Zhou and Chen [19] introduced the m-weak group inverse in the ring and presented its different characterizations.
Definition 1.2. Let R be a unitary ring with involution, a∈R and m∈Z+. If there exist x∈R and k∈Z+ such that
then x is called the m-weak group inverse of a and in this case, a is m-weak group invertible.
In general, the m-weak group inverse of a may not be unique. If the m-weak group inverse of a is unique, then it is denoted by aⓌm.
In [22], we can find a relation between the weak core inverse and the m-weak group inverse as well as certain necessary and sufficient conditions that the Drazin inverse coincides with the m-weak group inverse of a complex matrix. It is interesting to note that X which satisfies (1.1) coincides with the m-weak group inverse on complex matrices, in which case X exists for every A∈Cn×n and is unique.
Now, we consider the system of equations
Motivated by the above discussion, we introduce a new characterization of the m-weak group inverse related with (1.2) and proved the existence and uniqueness of a solution of (1.2), for every A∈Cn×n. Some new characterizations of the m-weak group inverse are derived in terms of the range space, null space, rank equalities, and projectors. We present some representations of the m-weak group inverse involving some known generalized inverses and limit expressions as well as certain relations between the m-weak group inverse and other generalized inverses. Finally, we consider a relation between the m-weak group inverse and the nonsingular bordered matrix, which is applied to the Cramer's rule for the solution of the restricted matrix equation.
The paper is organized as follows: In Section 2, we present some well-known definitions and lemmas. In Section 3, we provide a new characterization, as well as certain representations and properties of the m-weak group inverse of a complex matrix. In Section 4, we provide several expressions of the m-weak group inverse which are useful in computation. In Section 5, we present some properties of the m-weak group inverse as well as the relationships between the m-weak group inverse and other generalized inverses by core-EP decomposition. In Section 6, we show the applications of the m-weak group inverse concerned with the bordered matrices and the Cramer's rule for the solution of the restricted matrix equation.
2.
Preliminaries
The symbols R(A), N(A) and A∗ denote the range space, null space and conjugate transpose of A∈Cm×n, respectively. The symbol In denotes the identity matrix of order n. Let PL,M be the projector on the space L along the M, where L,M≤Cn and L⊕M=Cn. For A∈Cm×n, PA represents the orthogonal projection onto R(A), i.e., PA=PR(A)=AA†. The symbols CPn and CHn represent the subsets of Cn×n consisting of all idempotent and Hermitian matrices, respectively, i.e.,
Let A∈Cm×n. The MP-inverse A† of A is the unique matrix X∈Cn×m satisfying the following four Penrose equations (see [14,23,24]):
A matrix X∈Cn×m that satisfies condition (1) above is called an inner inverse of A and the set of all inner inverses of A is denoted by A{1}, while a matrix X∈Cn×m that satisfies condition (2) above is called an outer inverse of A. A matrix X∈Cn×m that satisfies both conditions (1) and (2) is called a reflexive g-inverse of A. If a matrix X∈Cn×m satisfies
where T and S are the subspaces of Cn and Cm respectively, then X is an outer inverse of A with prescribed range and null space and it is denoted by A(2)T,S. If A(2)T,S exists, then it is unique. The notion of the core inverse on the CCMn was proposed and was denoted by  [25,26,27]. The core inverse of A∈Cn×nk is the unique matrix X∈Cn×n satisfying
[25,26,27]. The core inverse of A∈Cn×nk is the unique matrix X∈Cn×n satisfying
In addition, it was proved that
The core-EP inverse of A∈Cn×nk, denoted by  is given in [28,29,30]. The core-EP inverse of A∈Cn×nk is the unique matrix X∈Cn×n satisfying
is given in [28,29,30]. The core-EP inverse of A∈Cn×nk is the unique matrix X∈Cn×n satisfying
Moreover, it was proved that
The DMP-inverse of A∈Cn×nk, denoted by AD,† was introduced in [10,11]. The DMP-inverse of A∈Cn×nk is the unique matrix X∈Cn×n satisfying
Moreover, it was shown that
Also, the dual DMP-inverse of A was introduced in [10], as A†,D=A†AAD.
The (B,C)-inverse of A∈Cm×n, denoted by A(B,C) [31,32], is the unique matrix X∈Cn×m satisfying
where B,C∈Cn×m.
To discuss further properties of the m-weak group inverse, several auxiliary lemmas will be given. The first lemma gives the core-EP decomposition of a matrix A∈Cn×nk which will be a very useful tool throughout this paper.
Lemma 2.1. [16] Let A∈Cn×nk. Then there exists a unitary matrix U∈Cn×n such that
where T∈Ct×t is nonsingular with t=r(T)=r(Ak) and N is nilpotent of index k. The representation (2.1) is called the core-EP decomposition of A, while A1 and A2 are the core part and nilpotent part of A, respectively.
Following the representation (2.1) of a matrix A∈Cn×nk, we have the following representations of certain generalized inverses (see [15,16,33]):
where Tk=k−1∑j=0TjSNk−1−j.
By direct computations, we get that A∈CCMn is equivalent with N=0, in which case
and
Let A∈Cn×nk be of the form (2.1) and let m∈Z+. The notations below will be frequently used in this paper:
Lemma 2.2. [34,Lemma 6] Let A∈Cn×nk be of the form (2.1). Then
From (2.8) and [16,Theorem 2.2], we get that
where t=r(Ak).
Lemma 2.3. [29,35,36] Let A∈Cn×nk and let m∈Z+. Then

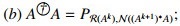
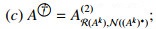
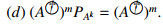
Lemma 2.4. Let A∈Cn×nk and let m∈Z+. Then 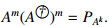
Proof. Assume that A∈Cn×nk is of the form (2.1). By (2.3), (2.12) and (2.13), it follows that
3.
m-weak group inverse on complex matrices
In this section, using the core-EP decomposition of a matrix A∈Cn×nk we will give another definition of the m-weak group inverse. Furthermore, some properties of the m-weak group inverse will be derived.
Theorem 3.1. Let A∈Cn×nk be given by (2.1) and let X∈Cn×n and m∈Z+. The system of equations
is consistent and has a unique solution X given by
Proof. If m=1, then X coincides with AⓌ. Clearly, X is the unique solution of (3.1) according to the definition of the weak group inverse. If m≠1, by (3.1), Lemmas 2.3 (d) and 2.4, it follows that
Thus, by (2.3) and (2.12), we have that
Definition 3.2. Let A∈Cn×nk and m∈Z+. The m-weak group inverse of A, denoted by AⓌm, is the unique solution of the system (3.1).
Remark 3.3. The m-weak group inverse is in some sense a generalization of the weak group inverse and Drazin inverse. We have the following:
(a) If m=1, then 1-weak group inverse of A∈Cn×nk coincides with the weak group inverse of A;
(b) If m≥k, then m-weak group inverse of A∈Cn×nk coincides with the Drazin inverse of A.
In the following example, we will show that the m-weak group inverse is different from some known generalized inverses.
Example 3.4. Let A=[I3I30N], where N=[010001000]. It can be verified that Ind(A)=3. By computations, we can check the following:
where H1=[1200010001], H2=[111011001], H3=[110010000], H4=[121212011000] and N†=[000100010].
It is clear that 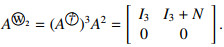
Theorem 3.5. Let A∈Cn×nk be decomposed by A=A1+A2 as in (2.1) and let m∈Z+. Then
(a) AⓌm is an outer inverse of A;
(b) AⓌm is a reflexive g-inverse of A1.
Proof. (a) By Lemmas 2.3 (d), 2.4 and the definition of AⓌm, it follows that
(b) By (2.2) and (3.2), we get that
From [16,Theorem 3.4], we get 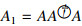 . By the fact that
. By the fact that 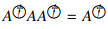 and the statement (a) above, it follows that
and the statement (a) above, it follows that
Hence AⓌm is a reflexive g-inverse of A1.
Theorem 3.6. Let A∈Cn×nk and m∈Z+. Then
(a) r(AⓌm)=r(Ak).
(b) R(AⓌm)=R(Ak), N(AⓌm)=N((Ak)∗Am).
(c) AⓌm=A(2)R(Ak),N((Ak)∗Am).
Proof. (a) Assume that A is given by (2.1). From (2.11) and (3.2), it is clear that r(AⓌm)=t=r(Ak).
(b) Since 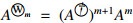 implies that
implies that 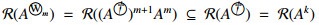 and since r(AⓌm)=r(Ak), we get R(AⓌm)=R(Ak). From
and since r(AⓌm)=r(Ak), we get R(AⓌm)=R(Ak). From 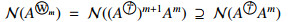 and
and 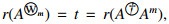 we get
we get 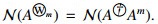 If
If 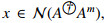 we get that
we get that 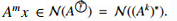 Then
Then 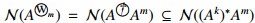 , and by r(AⓌm)=r((Ak)∗Am), it follows that
, and by r(AⓌm)=r((Ak)∗Am), it follows that 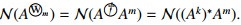
(c) It is a direct consequence from Theorems 3.5 (a) and 3.6 (b).
Theorem 3.7. Let A∈Cn×nk and m∈Z+. Then
(a) AAⓌm=PR(Ak),N((Ak)∗Am);
(b) AⓌmA=PR(Ak),N((Ak)∗Am+1).
Proof. (a) From Theorem 3.5 (a), it follows that AAⓌm∈CPn. By the definition of AⓌm and (3.2), it can be proved that  and r(AAⓌm)=r(AⓌm)=r(Ak)=t. Hence R(AAⓌm)=R(Ak). Similarly, we get that N(AAⓌm)=N(AⓌm)=N((Ak)∗Am). Therefore, AAⓌm=PR(Ak),N((Ak)∗Am).
and r(AAⓌm)=r(AⓌm)=r(Ak)=t. Hence R(AAⓌm)=R(Ak). Similarly, we get that N(AAⓌm)=N(AⓌm)=N((Ak)∗Am). Therefore, AAⓌm=PR(Ak),N((Ak)∗Am).
(b) The proof follows similarly as for the part (a).
4.
Some characterizations of the m-weak group inverse
In this part, we represent some characterizations of the m-weak group inverse in terms of the range space, null space, rank equalities, and projectors.
The next theorem gives several characterizations of AⓌm.
Theorem 4.1. Let A∈Cn×nk, X∈Cn×n and let m∈Z+. Then the following hold:
(a) X=AⓌm.
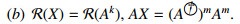
(c) R(X)=R(Ak), Am+1X=PAkAm.
(d) R(X)=R(Ak), (Ak)∗Am+1X=(Ak)∗Am.
Proof. (a)⇒(b): This follows directly by Theorem 3.6 (b) and the definition of AⓌm.
(b)⇒(c): Premultiplying 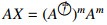 by Am, and by Lemma 2.4, it follows that
by Am, and by Lemma 2.4, it follows that
(c)⇒(d): Premultiplying Am+1X=PAkAm by (Ak)∗, it follows that
(d)⇒(a): Let A be of the form (2.1). By (2.11) and R(X)=R(Ak), we obtain that
where X1∈Ct×t and X2∈Ct×(n−t). Thus (Ak)∗Am+1X=(Ak)∗Am implies that
i.e., X1=T−1 and X2=(Tm+1)−1Tm, which imply X=U[T−1(Tm+1)−1Tm00]U∗=AⓌm.
By Theorem 3.5, it is known that AⓌm is an outer inverse of A∈Cn×nk, i.e., AⓌmAAⓌm=AⓌm. Using this result, we obtain some characterizations of AⓌm.
Theorem 4.2. Let A∈Cn×nk, X∈Cn×n and let m∈N+. Then the following conditions are equivalent:
(a) X=AⓌm.
(b) XAX=X, R(X)=R(Ak), N(X)=N((Ak)∗Am).
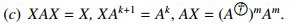
(d) XAX=X, R(X)=R(Ak), (Am)∗Am+1X∈CHn.
Proof. (a)⇒(b): It is a direct consequence of Theorem 3.6 (c).
(b)⇒(c): By XAX=X and R(X)=R(Ak), it follows that
and
Since AX, 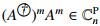 , we have
, we have 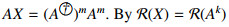 and XAX=X, we obtain that XAk+1=Ak.
and XAX=X, we obtain that XAk+1=Ak.
(c)⇒(d): We have that
and by R(Ak)=R(XAk+1)⊆R(X), we get R(XA)=R(Ak). Since 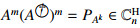 , it follows that
, it follows that
(d)⇒(a): Assume that A is of the form (2.1). From XAX=X and R(X)=R(Ak), we get that XAk+1=Ak. Then it is easy to conclude that
where X2∈Ct×(n−t).
Since
we obtain that X2=T−(m+1)Tm. Hence X=U[T−1T−(m+1)Tm00]U∗=AⓌm.
Motivated by the first two matrix equations XAk+1=Ak and XAX=X, we provide several characterizations of AⓌm.
Theorem 4.3. Let A∈Cn×nk, X∈Cn×n and let m∈Z+. Then the following conditions are equivalent:
(a) X=AⓌm.
(b) XAk+1=Ak, AX2=X, (Am)∗Am+1X∈CHn.
(c) XAk+1=Ak, AX2=X, Am+1X=PAkAm.

Proof. (a)⇔(b): This follows by Proposition 4.2 in [19].
(a)⇒(c): It is a direct consequence of Theorems 4.1 (c) and 4.2 (c).
(c)⇒(d): Assume that A is given by (2.1). By XAk+1=Ak, we get that
where X2∈Ct×(n−t) and X4∈C(n−t)×(n−t).
By AX2=X, we have that X4=NX42, which implies that
Using (2.12) and (2.13) and that Am+1X=PAkAm, we get
Now, the proof follows directly.
(d)⇒(a): Since XAk+1=Ak, it follows that R(Ak)=R(XAk+1)⊆R(X) and by r(X)=r(Ak), we get R(Ak)=R(X). Hence, according to Theorem 4.1 (b), we get X=AⓌm.
According to Theorem 3.7, it follows that AX=PR(Ak),N((Ak)∗Am) and XA=PR(Ak),N((Ak)∗Am+1) when X=AⓌm. Conversely, the implication does not hold. Here's an example below.
Example 4.4. Let A=[I3L0N], X=[I3L0L], where N=[010001000], L=[001000000]. Then it is clear that k=Ind(A)=3 and AⓌ2=[I3L00]. It can be directly verified that AX=PR(A3),N((A3)∗A2),XA=PR(A3),N((A3)∗A3). However, X≠AⓌ2.
Based on the example above, the next theorem, we consider other characterizations of AⓌm by using AX=PR(Ak),N((Ak)∗Am) and XA=PR(Ak),N((Ak)∗Am+1).
Theorem 4.5. Let A∈Cn×nk be of the form (2.1), X∈Cn×n and m∈Z+. Then the following statements are equivalent:
(a) X=AⓌm;
(a) AX=PR(Ak),N((Ak)∗Am),XA=PR(Ak),N((Ak)∗Am+1) and r(X)=r(Ak);
(a) AX=PR(Ak),N((Ak)∗Am),XA=PR(Ak),N((Ak)∗Am+1) and XAX=X;
(a) AX=PR(Ak),N((Ak)∗Am),XA=PR(Ak),N((Ak)∗Am+1) and AX2=X.
Proof. (a)⇒(b): It is a direct consequence of Theorems 3.6 (a) and 3.7.
(b)⇒(c): Since XA=PR(Ak),N((Ak)∗Am+1) and r(X)=r(Ak), we get that R(X)=R(Ak) and by XA=PR(Ak),N((Ak)∗Am+1), we obtain XAX=X.
(c)⇒(d): From XA=PR(Ak),N((Ak)∗Am+1) and r(X)=r(Ak), we have that R(X)=R(Ak) and by AX=PR(Ak),N((Ak)∗Am), it follows that AX2=X.
(d)⇒(a): By XA=PR(Ak),N((Ak)∗Am+1) and AX2=X, it follows that
which implies R(X)=R(Ak). By AX=PR(Ak),N((Ak)∗Am), we get that
According to Theorem 4.1 (d), we have that X=AⓌm.
Analogously, we characterize AⓌm using that AX=PR(Ak),N((Ak)∗Am) or XA=PR(Ak),N((Ak)∗Am+1) as follows:
Theorem 4.6. Let A∈Cn×nk, X∈Cn×n and let m∈Z+. Then
(a) X=AⓌm is the unique solution of the system of equations:
(b) X=AⓌm is the unique solution of the system of equations:
Proof. (a) By Theorems 3.6 (b) and 3.7 (a), it follows that X=AⓌm is a solution of the system of Eq (4.1). Conversely, if the system (4.1) is consistent, it follows that (Ak)∗AmAX=(Ak)∗Am. Hence by Theorem 4.1, X=AⓌm (d).
(b) By Theorems 3.6 (b) and 3.7 (b), it is evident that X=AⓌm is a solution of (4.2). Next, we prove the uniqueness of the solution.
Assume that X1,X2 satisfy the system of Eq (4.2). Then X1A=X2A and N(X1)=N(X2)=N((Ak)∗Am). Thus, we get that R(X∗1−X∗2)⊆N(A∗)⊆N((Ak)∗) and R(X∗1−X∗2)⊆R((Am)∗Ak). For any η∈N((Ak)∗)∩R((Am)∗Ak), we obtain that (Ak)∗η=0, η=(Am)∗Akξ for some ξ∈Cn. Since Ind(A)=k, we derive that R(Ak)=R(Ak+m), and it follows that Akξ=Ak+mξ0 for some ξ0∈Cn×n. Then we have that
Premultiplying the equation above by ξ∗0, we derive that (Ak+mξ0)∗Ak+mξ0=0, which implies Ak+mξ0=0. Hence η=0, i.e., R(X∗1−X∗2)={0}, which implies X1=X2.
Remark 4.7. Notice that the condition R(X)=R(Ak) in Theorem 4.6 (a) can be replaced by R(X)⊆R(Ak). Also the condition N(X)=N((Ak)∗Am) in Theorem 4.6 (b) can be replaced by N(X)⊇N((Ak)∗Am).
5.
Representations of the m-weak group inverse
From Theorem 3.1, we get an expression of AⓌm in terms of 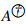 . In the next results, we present several expressions of AⓌm in terms of certain generalized inverses.
. In the next results, we present several expressions of AⓌm in terms of certain generalized inverses.
Theorem 5.1. Let A∈Cn×nk and let m∈Z+. Then the following statements hold:
(a) AⓌm=(AD)m+1PAkAm.
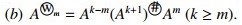
(c) AⓌm=(Ak)#Ak−m−1PAkAm (k≥m+1).
(d) AⓌm=(Am+1PAk)†Am.
(e) AⓌm=Am−1PAk(Am)Ⓦ.
Proof. Assume that A is given by (2.1). By (2.3)–(2.7) and (2.11)–(2.13), we get that
(a) By (2.12), (2.13) and (5.3), it follows that
Hence AⓌm=(AD)m+1PAkAm.
The proofs of (b)–(e) are analogous to that of (a).
Next, we consider the accuracy of the expression in Theorem 5.1 (a) for computing the m-weak group inverse.
Example 5.2. Let
Assume that A is given by (2.1). Then
It is clear that k = Ind(A) = 3. According to (2.12), (2.13), (3.2) and (5.3), a straightforward computation shows that
Let K=(AD)3PA3A2. Then
and
where ∥⋅∥ is the Frobenius norm.
Hence, Theorem 5.1 (a) gives a good result in terms of computational accuracy.
In the following theorem, we present a connection between the (B,C)-inverse and the m-weak group inverse showing that the m-weak group inverse of A∈Cn×nk is its (Ak,(Ak)∗Am)-inverse.
Theorem 5.3. Let A∈Cn×nk and let m∈Z+. Then AⓌm=A(Ak,(Ak)∗Am).
Proof. By Theorem 3.7 we have that AⓌmAAk=Ak and ((Ak)∗Am)AAⓌm=(Ak)∗Am. From Theorem 3.6 (b), we derive that R(AⓌm)=R(Ak) and N(AⓌm)=N((Ak)∗Am). Evidently, AⓌm=A(Ak,(Ak)∗Am).
Now we will give some limit expressions of AⓌm, but before we need the next auxiliary lemma:
Lemma 5.4. [37] Let A∈Cm×n,X∈Cn×p and Y∈Cp×m. Then the following hold:
(a) limλ→0X(λIp+YAX)−1Y exists;
(b) r(XYAXY)=r(XY);
(c) A(2)R(XY),N(XY) exists,
in which case,
Theorem 5.5. Let A∈Cn×nk be given by (2.1) and let m∈N+. Then the following statements hold:
(a) AⓌm=limλ→0Ak(λIn+(Ak)∗Ak+m+1)−1(Ak)∗Am;
(b) AⓌm=limλ→0Ak(Ak)∗(λIn+Ak+m+1(Ak)∗)−1Am;
(c) AⓌm=limλ→0Ak(Ak)∗Am(λIn+Ak+1(Ak)∗Am)−1;
(d) AⓌm=limλ→0(λIn+Ak(Ak)∗Am+1)−1Ak(Ak)∗Am.
Proof. (a) It is easy to check that r(Ak(Ak)∗Am)=r((Ak)∗Am)=r(Ak)=t. By Theorem 3.6, we get that R(Ak)=R(Ak(Ak)∗Am), N((Ak)∗Am)=N(Ak(Ak)∗Am). From Theorem 3.6, we get
Let X=Ak,Y=(Ak)∗Am. By Lemma 5.4, we get that
The statements (b)–(d) can be similarly proved.
The following example will test the accuracy of expression in Theorem 5.5 (a) for computing the m-weak group inverse.
Example 5.6. Let
with k = Ind(A) = 3. By 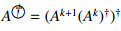 , we get
, we get
Together with (3.2), it follows that
Let L=limλ→0A3(λIn+(A3)∗A6)−1(A3)∗A2. Then
and
where ∥⋅∥ is the Frobenius norm. Hence, the representation in Theorem 5.5 (a) is efficient for computing the m-weak group inverse.
6.
Relationships between the m-weak group inverse and other generalized inverses
In this section, we consider some relations between the m-weak group inverse and other generalized inverses as well as certain matrix classes. The symbols COPn, CEPn, Ci−EPn and  stand for the subsets of Cn×n consisting of orthogonal projectors (Hermitian idempotent matrices), EP (Range-Hermitian) matrices, i-EP matrices and k-core-EP matrices, respectively, i.e.,
stand for the subsets of Cn×n consisting of orthogonal projectors (Hermitian idempotent matrices), EP (Range-Hermitian) matrices, i-EP matrices and k-core-EP matrices, respectively, i.e.,
First, we will state the following lemma auxiliary lemma:
Lemma 6.1. Let A∈Cn×nk be given by (2.1). Then Tm=0 if and only if S=0.
Proof. Notice that Tm=0 can be equivalently expressed by the equation below:
Multiplying the equation above from the right side by Nk−1, we get SNk−1=0. Then multiplying from the right by Nk−2, we get SNk−1=0,. Similarly, we get SNk−3=0,⋯,SN=0. Now by (6.1), it follows that Tm−1S=0, i.e, S=0.
The next theorem provides some necessary and sufficient conditions for AⓌm to be equal to various transformations of A∈Cn×nk.
Theorem 6.2. Let A∈Cn×nk and let m∈Z+. Then the following statements hold:
(a) AⓌm∈A{1} if and only if A∈CCMn.
(b) AⓌm∈CCMn.
(c) AⓌm=A if and only if A=A3.
(d) AⓌm=A∗ if and only if AA∗∈COPn and A∈CEPn.
(e) AⓌm=PA if and only if A∈COPn.
Proof. Let A be given by (2.1).
(a) By (3.2), it follows that
(b) By (3.2), it is clear that r(AⓌm)=r((AⓌm)2)=t, which implies AⓌm∈CCMn.
(c) From (3.2), we get that
(d) According to (3.2), we obtain that
(e) By (2.9) and (3.2), it follows that
Hence AⓌm=PA if and only if A∈COPn.
Using the core-EP decompositio, we proved that A∈Ci−EPn if and only if AⓌm∈CEPn. Therefore, we will consider certain equivalent conditions for AⓌm∈CEPn.
Lemma 6.3. [17] Let A∈Cn×nk be of the form (2.1). Then A∈Ci−EPn if and only if S=0.
Moreover, S=0 if and only if 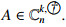
Theorem 6.4. Let A∈Cn×nk and let m∈Z+. The following statements are equivalent:
(a) AⓌm∈CEPn;
(b) A∈Ci−EPn;
(c) AⓌ∈CEPn;
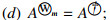
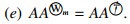
Proof. Let A∈Cn×nk be of the form (2.1). According to Lemma 6.3, we will prove that each of the statements (a), (c), (d) and (e) is equivalent to S=0.
(a) According to (3.2) and Lemma 6.1, it follows that
(c) By (2.4), we get that
(d) By (2.3), (3.2) and Lemma 6.1, it follows that
(e) From (2.3), (3.2) and Lemma 6.1, we get that
In [22], the authors proved that AⓌm=AD if and only if SNm=0. In the following results, we investigate the relation between the m-weak group inverse and other generalized inverses such as the MP-inverse, group inverse, core inverse, DMP-inverse, dual DMP-inverse, weak group inverse by core-EP decomposition.
Theorem 6.5. Let A∈Cn×nk be given by (2.1) and let m∈Z+. Then the following statements hold:
(a) AⓌm=A†⟺A∈CEPn;
(b) AⓌm=A#⟺A∈CCMn;
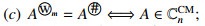
(d) AⓌm=AD,†⟺Tk−mTm=TkNN†;
(e) AⓌm=A†,D⟺SNm=0 and S=SN†N;
(f) AⓌm=AⓌ⟺SN=0 (m>1).
Proof. (a) It follows from (2.8) and (3.2) that
(b) Since A# exits if and only if A∈CCMn, which is equivalent to N=0, we get by (2.6) and (3.2) the following:
(c) The proof follows similarly as in (b).
(d) Using (2.5) and (2.9) to AD,†=ADAA†, we derive
and by (3.2), it follows that
(e) Using (2.5) and (2.10) and the faact that A†,D=A†AAD, we obtain that
which together with (3.2), gives
(f) If m>1, from (2.4) and (3.2), we get
Clearly, (Tm+1)−1Tm=T−2S is equivalent to T−3SN+⋯+(Tm+1)−1SNm−1=0, which is further equivalent to SN=0. Hence AⓌm=AⓌ if and only if SN=0.
7.
Applications of the m-weak group inverse
In this section, we consider a relation between the m-weak group inverse and the nonsingular bordered matrix, which will be applied to the Cramer's rule for the solution of the restricted matrix equation.
Theorem 7.1. Let A∈Cn×nk be such that r(Ak)=t and let m∈Z+. Let B∈Cn×(n−t) and C∗∈Cn×(n−t) be of full column rank such that N((Ak)∗Am)=R(B) and R(Ak)=N(C). Then the bordered matrix
is invertible and its inverse is given by
Proof. Let X=[AⓌm (In−AⓌmA)C†B†(In−AAⓌm) B†(AAⓌmA−A)C†]. Since R(AⓌm)=R(Ak)=N(C), we have that CAⓌm=0. Since C is a full row rank matrix, it is right invertible and CC†=In−t. From
we get BB†(In−AAⓌm)=In−AAⓌm. Hence,
Thus, X=K−1.
In the next result, we will discuss the solution of the restricted matrix equation
using the m-weak group inverse.
Theorem 7.2. Let A∈Cn×nk, X∈Cn×p and D∈Cn×p. If R(D)⊆R(Ak), then the restricted matrix equation
has a unique solution X=AⓌmD.
Proof. Since R(Ak)=R(AAk) and R(D)⊆R(Ak), we get that R(D)⊆AR(Ak), which implies solvability of the matrix Eq (7.1). Obviously, X=AⓌmD is a solution of (7.1). Then we prove the uniqueness of X. If X1 also satisfies (7.1), then
Based on the nonsingularity of the bordered matrix given in Theorem 7.1, we will show in the next theorem how the Cramer's rule can be used for solving the restricted matrix Eq (7.1).
Theorem 7.3. Let A∈Cn×nk be such that r(Ak)=t and let X∈Cn×p and D∈Cn×p. Let B∈Cn×(n−t) and C∗∈Cn×(n−t) be full column rank matrices such that N((Ak)∗Am)=R(B) and R(Ak)=N(C). Then the unique solution of the restricted matrix Eq (7.1) is given by X=[xij], where
where dj denotes the j-th column of D.
Proof. Since X is the solution of the restricted matrix Eq (7.1), we get that R(X)⊆R(Ak)=N(C), which implies CX=0. Then the restricted matrix Eq (7.1) can be rewritten as
By Theorem 7.1, we have that [ABC0] is invertible. Consequently, (7.2) follows from the Cramer's rule for the above equation.
Example 7.4. Let
It can be verified that Ind(A)=3. Then we get that
It is easy to check that
satisfies the restricted matrix equation AX=D and R(X)⊆R(A3). By simple calculations, we can also get that the components of X can be expressed by (7.2).
8.
Conclusions
This paper gives a new definition of the m-weak group inverse for the complex matrices, which extends the Drazin inverse and the weak group inverse. Some characterizations of the m-weak group inverse in terms of the range space, null space, rank, and projectors are presented. Several representations of the m-weak group inverse involving some known generalized inverses as well as limitations are also derived. The representation in Theorem 5.1 gives a better result in terms of the computational accuracy (see Examples 5.2 and 5.6). The m-weak group inverses are concerned with the solution of a restricted matrix Eq (7.1). The solution of (7.1) can also be expressed by the Cramer's rule (see Theorem 7.3). In [38,39,40], there are some iterative methods and algorithms to compute the outer inverses. Motivated by these, further investigations deserve more attention as follows:
(1) The applications of the m-weak group inverse in linear equations and matrix equations;
(2) Perturbation formulae as well as perturbation bounds for the m-weak group inverse;
(3) Iterative algorithm, a splitting method for computing the m-weak group inverse;
(4) Other representations of the m-weak group inverse.
Acknowledgement
This research is supported by the National Natural Science Foundation of China (No. 11961076).
Conflict of interest
The authors declare no conflict of interest.









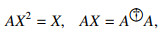
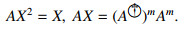
 [
[
 is given in [
is given in [
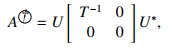
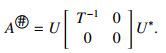

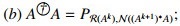
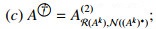
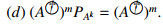
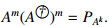

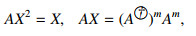
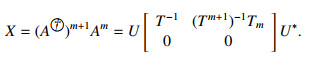

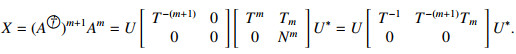

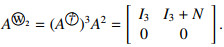

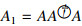 . By the fact that
. By the fact that 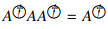 and the statement
and the statement 
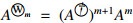 implies that
implies that 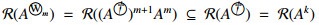 and since
and since 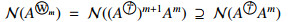 and
and 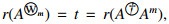 we get
we get 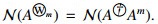 If
If 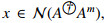 we get that
we get that 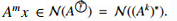 Then
Then 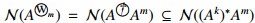 , and by
, and by 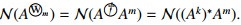
 and
and 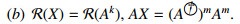
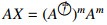 by
by 
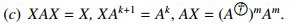


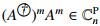 , we have
, we have 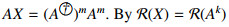 and
and 
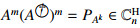 , it follows that
, it follows that

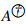 . In the next results, we present several expressions of
. In the next results, we present several expressions of 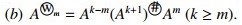






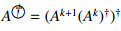 , we get
, we get


 stand for the subsets of
stand for the subsets of 
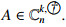
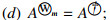
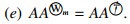
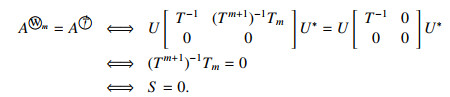

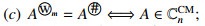





 DownLoad:
DownLoad: