1.
Introduction
In population dynamics, the study of traveling waves can help us to understand the mechanisms behind various propagating wave patterns better [1,2]. The existence and stability of various types of wave solutions have been theoretically proved in many population models [3].
Based on the delayed Nicholson's blowflies equation [4], So and Yang [5] proposed the following diffusive version of delayed Nicholson's blowflies equation
which describes the population growth of Nicholson's blowflies with spatial diffusion. So and Yang [5] studied the stability of the steady-state solutions for system (1.1) under the Dirichlet problem. So and Zou [6] proved the existence of traveling wavefronts. Stability of traveling wavefronts were investigated in [7,8]. Yang [9] studied the existence and stability of periodic traveling waves. In order to describe the individual diffuse phenomenon during the reproductive cycle and more general birth functions, So et al. [10] derived the following reaction-diffusion equation involving nonlocal delay effects
and obtained Hopf bifurcation of model (1.2). See [11,12,13,14,15,16] for more progress on various types of traveling waves of system (1.2). In [17], Liang and Wu considered a delayed reaction diffusion equation with advection
which describes the pattern dynamics of a single-species population with two age classes and a fixed maturation period living in a spatial transport field and proved the existence of traveling wavefront. Stability of the traveling wavefronts were investigated in [18,19,20]. Of particular interest is the influence of advection terms on the propagation of traveling wave solutions. The influence of advection terms on traveling wavefronts were studied by many researchers [19,21]. To the best of our knowledge, however, the work on periodic traveling wave solution in the population model like system (1.3) with advection effect is relatively rare. Moreover, in the previous studies on periodic traveling wave solutions mainly focused on theoretical proof, see [9,11,22], which lacked the support of numerical simulation.
The objective of this paper is to derive the influence of advection terms on periodic traveling wave solutions and illustrate our main results by using numerical simulations. In order to make the influence of advection terms more obvious, in model (1.3), we suppose that the diffusion rate d1=0 and replace the heat kernel function Jα(y) by the Dirac function to eliminate the influence of diffusion and nonlocal delay. Moreover, we choose the Nicholson's birth function on account of facilitate numerical simulations. Therefore, we consider the Nicholson's blowflies model with delay and advection as follows:
with the following initial condition
Here, u(x,t) denotes the total mature population at location x∈R and time t≥0;
d is the advection rate of the mature represents the velocity of the spatial transport field; δ is the per capita adult death rate; p is the maximum per capita daily egg production rate; τ is a delay represents the maturation time; 1a is the size at which the population reproduces at its maximum rate, where d, δ, p, a and τ are positive constants. Moreover, we assume that p>δ such that system (1.4) always has two equilibria u⋆=0 and u∗=1alnpδ∈R+.
2.
Main results
In this part, the existence, stability and bifurcation direction of periodic traveling wave solutions of system (1.4) is proved theoretically by using the Hopf bifurcation theorem, center manifold theorem as well as normal form theory [23], which are bifurcated from two equilibria, respectively.
For the equilibrium u⋆=0, we have the following result.
Theorem 2.1. For each fixed wave velocity c, when the advection rate d>c, Eq (1.4) has a branch of periodic traveling waves bifurcating from u⋆ for the bifurcation parameter τ near τ⋆n, with period approximately equals to 2πω⋆. Moreover, if κ⋆>0 (resp. <0), the bifurcation is backward (resp. forward) and periodic traveling waves are unstable (resp. have the same stability as u⋆ before the bifurcation), where ω⋆, τ⋆n and κ⋆ are shown in Eqs (2.6), (2.7) and (2.15), respectively.
Proof. Let the traveling wave variable s=x+ct and u(x,t)=v(s), then Eq (1.4) can be rewriten as
Linearizing system (2.1) around v⋆=u⋆=0, we have
The characteristic equation of system (2.2) is
When τ=0, Eq (2.3) reduces to
which has a real root λ=p−δc−d. If 0<c<d, we have λ<0; if c>d, then λ>0.
Assume that there is some ω>0 such that λ=iω is a root of Eq (2.3), i.e.,
From Euler's formula, we obtain
In view of sin2(cτω)+cos2(cτω)=1, from system (2.4), we have
Equation (2.5) have a positive solution
Substituting ω⋆ into Eq (2.4), we obtain
It implies that Eq (2.3) has a pair of purely imaginary roots λ=±iω⋆ when τ=τ⋆n. Moreover, we have
i.e., λ=iω⋆ is a simple root of Eq (2.3). Moreover, we need to check the transversality condition in the Hopf bifurcation theorem. To this end, we discuss the sign of
Taking derivatives of system (2.3) with respect to τ yields that
Noticing that pe−cτλ=(c−d)λ+δ, we rewrite Eq (2.8) as
Hence, we obtain
Separating the real part, we yield
It implies that the purely imaginary roots of Eq (2.3) will fall into the right half plane of the complex plane when the delay τ increases slightly around the critical value τ=τ⋆n. Based on the continuity of function λ(τ), we know that only the case of c<d satisfies the transversality condition, i.e., when c<d, equation (1.4) has 2πω⋆−periodic traveling waves bifurcating from u⋆.
Next, we study the stability and bifurcation direction of periodic traveling wave solutions. Let y(s)=v(cτs) and τ=τ⋆n+γ, γ∈R. Equation (2.1) can be rewritten as
From Taylor's formula, we have
where L(γ) and H(γ,⋅) are given by
and
for ϕ∈C([−1,0],R), respectively.
Define a bounded variation function as
such that
where ˉδ(⋅) is the Delta function. Moreover, for ψ∈C([−1,0],R), we define
and
Hence, we can rewrite Eq (2.10) as
where ys(θ)=y(s+θ), θ∈[−1,0].
Define the adjoint operator A∗ of A(0) and the bilinear form as
and
where ψ∈C([−1,0],R) and φ∈C([0,1],R). Then, we have ⟨φ,A(0)ψ⟩=⟨A∗φ,ψ⟩. Hence, we obtain that q∗(χ)=Deicω⋆τ⋆nχ is the eigenfunction of A∗ associated with −icω⋆τ⋆n, and q(θ)=eicω⋆τ⋆nθ is the eigenfunction of A(0) associated with icω⋆τ⋆n, where D=11+cτ⋆nδc−d−icω⋆τ⋆n. So, we yield
As a solution ys of system (2.11) with γ=0, we define z(s)=⟨q∗,ys⟩ and
Then, we yield ⟨q∗,W⟩=0 and
It follows ⟨q∗,˙ys⟩=⟨q∗,A(0)ys⟩+⟨q∗,N(0)ys⟩ that
Let
where
and
Next, we compute W11(θ) and W20(θ). Taking derivatives of system (2.12) with respect to s yields that
where
Moreover, we have
Hence, we yield
and
As θ∈[−1,0), we have
Hence, we have
When θ=0, we obtain
Substituting Eq (2.13) into (2.14), we yield
It follows [23] that stability and bifurcation direction of periodic orbit is determined by the quantity
When κ⋆>0 (resp. <0), there exists ζ>0 such that for τ∈(τn−ζ,τn) (resp. τ∈(τn,τn+ζ)), periodic traveling waves of Eq (1.4) bifurcating from u⋆ are unstable (resp. have the same stability as u⋆ before the bifurcation).
Furthermore, for the positive equilibrium u∗=1alnpδ, we obtain the similar result.
Theorem 2.2. For each fixed wave velocity c, when the advection rate d<c and p>δe2, there exists a branch of periodic traveling wave solutions of system (1.4) with period approximately equals to 2πω∗, which is bifurcated from u∗ for the bifurcation parameter τ near τ∗n. Moreover, the bifurcation is backward (resp. forward) and periodic traveling waves are unstable (resp. have the same stability as u∗ before the bifurcation) if κ∗>0 (resp. <0), where ω∗, τ∗n and κ∗ are shown in Eqs (2.17)–(2.19), respectively.
In view of the proof of Theorem 2.2 is similar to Theorem 2.1, we only give the simple proof of Theorem 2.2 included some key relations.
Proof of Theorem 2.2. Linearizing Eq (2.1) at v∗=u∗, we obtain the following characteristic equation
When τ=0, the characteristic equation reduces to (c−d)λ+δlnpδ=0, which has a real root λ=δd−clnpδ. We know that λ>0 as 0<c<d, and λ<0 with c>d. Similarly to the above process, we know that when p>e2δ, Eq (2.16) have a pair of simple purely imaginary roots λ=±iω∗, where
with
Moreover, we have
It implies that when p>δe2 and c>d, there exists a branch of periodic traveling wave solutions of system (1.4) with period approximately equals to 2πω∗, which is bifurcated from u∗ for the bifurcation parameter τ near τ∗n.
Similarly to the above calculation, we get the quantity determined stability and bifurcation direction of periodic orbit bifurcating from u∗ as follows:
where
and
When κ∗>0 (resp. <0), the bifurcation is backward (resp. forward) and periodic traveling waves are unstable (resp. have the same stability as u∗ before the bifurcation).
3.
Numerical simulations
In this section, we illustrate our results by showing some numerical simulations. For Theorem 2.1, we choose the parameters δ=1, p=8, a=1 and d=27. Set the wave speed c=4<d. Hence, we have the following system
Substituting the traveling wave variable s=x+4t and u(x,t)=v(s) into Eq (3.1), we have
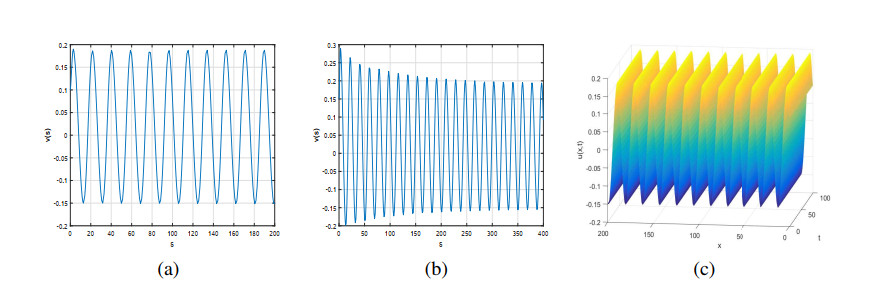
Here, we only consider the case of the first critical bifurcation parameter τ⋆0. Based on Section 2, we know that system (3.2) has periodic solution bifurcating from u⋆=0 with τ near τ⋆0≈1.0471, whose period approximately equal to 2πω⋆≈18.2068. Moreover, by using Mathematica 12.0, we can calculate that κ⋆≈−1.8848, i.e., the periodic solution is stable, whose bifurcation direction is forward. Let the initial function v1(s)=cos(s)10. In Figure 1(a), we see that the numerical periodic solution of system (3.2) is bifurcated from u⋆ when τ=1.0662>τ⋆0. It follows Figure 1(b) that solution of (3.2) converges to the periodic solution. Figure 1(c) shows the numerical periodic traveling waves of Eq (3.1) after translating back to the original variable.
For Theorem 2.2, choosing the parameters d=0.5, a=1, δ=1 and p=10 such that p>δe2. Let the wave speed c=1.2>d=0.5. Hence, we obtain the following system
The differential equation with wave profile of system (3.3) is
It follows Theorem 2.2 that system (3.4) has periodic solution bifurcating from u∗=ln10≈2.303 with τ near τ∗0≈1.709, where the period approximately equal to 2πω∗≈5.269. Moreover, we yield κ∗≈0.1851, i.e., the periodic solution is unstable, whose bifurcation direction is backward. Set the initial function v2(s)=ln10+cos(s)5. The numerical periodic solution of system (3.4) with τ=1.685<τ∗0 bifurcating from u∗ is shown in Figure 2(a). In Figure 2(b), we see that the periodic solution is unstable. By translating back to the original variable, the numerical periodic traveling wave solution of Eq (3.3) bifurcating from u∗ is shown in Figure 2(c).
4.
Conclusions
In this paper, we investigate the existence, stability and bifurcation direction of periodic traveling waves for the delayed Nicholson's blowflies model with advection. By using traveling wave transformation, the construction of periodic traveling wave solutions of the original model (1.4) is equivalent to looking for periodic solutions of the delayed Nicholson's blowflies model (2.1). When introducting the advection term into the system, we obtain the influence of advection term on the bifurcation position, stability and bifurcation direction of periodic solutions (see Theorems 2.1 and 2.2). Moreover, we illustrate our theoretical results by using numerical simulations.
There exist some natural extensions of this article. Indeed, we take the diffusion coefficient of system (1.3) to be zero in this paper, which greatly reduces the difficulty of our research. In practice, we also derive the theoretical results of system (1.3) similar to Theorems 2.1 and 2.2 by using the perturbation technique [22]. However, the numerical simulations of periodic traveling waves for reaction-diffusion models is very difficult, because the equilibrium points of the corresponding delayed differential system become hyperbolic in this case. How to use mathematical software to simulate periodic solutions bifurcated from hyperbolic equilibria of delayed differential equations? These raise us the future direction.
Acknowledgments
We are grateful to the editors and referees for their helpful comments and suggestions. This research is supported by Scientific and Technological Research Program of Chongqing Municipal Education Commission (Grant NO. KJQN201900610 and KJQN202000730), Natural Science Foundation of Chongqing, China (Grant NO. CSTB2022NSCQ-MSX1204) and National Natural Science Foundation of China (Grant No. 12001076).
Conflict of interest
The authors declare that they have no known competing financial interests or personal relationships that could have appeared to influence the work reported in this paper.









 DownLoad:
DownLoad:



