1.
Introduction
Hypericum alpestre (Hypericaceae family) is a perennial plant with worldwide distribution. It has been used in traditional medicine in Armenia since the ancient times [1]. There is very scarce scientific data about biological or pharmacological properties of plant H. alpestre [1]–[4]. Hypericum spp. includes about 500 species of shrubs, herbs and trees [5]. There are a huge number of scientific articles demonstrating the biological activities of different other species of plants belonging to the Hypericum genus. Literature data suggest antibacterial [4],[6],[7], antifungal [8], antiviral, antitumor [7] and antioxidant activities [7],[9] of compounds extracted from Hypericum species. H. perforatum is the best-known and the most investigated species of the genus [10]. More than 2500 studies on Hypericum have been published to date, including several reviews focused on the phytochemistry of H. perforatum [11],[12] and its pharmacology [13], or both aspects [10],[14], nomenclature and taxonomy [15]. The most thorough investigated property of the Hypericum genus is the phenomenon of neuroprotective activity [7],[16]. Different mechanisms of possible neuroprotective action have been suggested: some authors proposed that it can be due to the ability of extracts to prevent different types of neurotoxicity [16], other sources mentioning the antioxidant capacity as the reason of neuroprotective action [7]. According to literature data there are several major compounds that could be responsible for the neuroprotective activity of H. perforatum through direct (in vitro and in vivo cytoprotective effect, etc.) and indirect (through antioxidant properties, etc.) pathways [7]. These major compounds include quercetin, hyperoside, quercitrin, rutin, hypericin, kaempferol, biapigenin, and hyperforin [7]. Besides these major active components, there are other compounds from genus Hypeicum with possible neuroprotective activity reported in several studies. For instance Xu et al. [17] showed that polyprenylated tetraoxygenated xanthones from roots of H. monogynum possess neuroprotective activity as well. In the other recent investigation, a new previously non described compounds (hyperelatones B–D and H, cinchonain Ib, and tenuiside A) were isolated from aerial parts of H. elatoides with potential neuroprotective effect [18]. In another study 3 new compounds (derivatives of polycyclic polyprenylated acyl phloroglucinol) with promising neuroprotective influence were isolated from H. monogynum [19]. Various compounds can be responsible for the neuroprotective activity of the extracts of Hypericum spp. and their spectre expands overtime.
According to the principle of chemosystematics [20] it was expected that H. alpestre extracts will have some potential neuroprotective actions. During our previous research works, H. alpestre methanolic extracts expressed antioxidant potential based on chemical tests including DPPH, H2O2, MDA reduction and Fe2+ chelation assays. High total phenolic content was also shown [21].
Based on this surmise and the fact of scarce scientific data, H. alpestre growing in high altitude of Armenian flora was selected as an investigation object. Several features of the phytochemicals extracted from H. alpestre including chemical composition, biological activity and possible neuroprotective action will be discussed in this article.
For implementation of proposed study we decided to employ two types of neuroglial cell lines—BV-2 wild type and acyl-CoA oxidase 1 (ACOX1) deficient cells as models for our investigations [4],[22],[23]. The selected models meet all necessary criteria: they are well-studied, easy-to cultivate and possess central role in the formation of neurodegenerative disorders associated with aging or some deviations in antioxidant system. These cell models were selected as one of the well-studied models for the investigation of neurodegenerative disorders (acyl-CoA oxidase 1 (ACOX1) deficiency which affect specifically the oxidation of very-long-chain fatty acids (VLCFA) and leading to their accumulation in plasma and tissues and that may provoke the cellular oxidative damage) [24].
2.
Materials and methods
2.1. Plant material
The H. alpestre subsp. polygonifolium (Rupr.) Avet. & Takht. aerial parts were harvested from the Tavush region (40.8683° N, 45.3392° E) of Armenia (1400–1600 m above sea level) at flowering period. Identification of plant materials was performed at the Department of Botany and Mycology, YSU (Armenia) by Dr. Narine Zakaryan. Plant materials were deposited to the Herbarium of Yerevan State University Voucher specimen serial number was given which is ERCB 13206. Dried plant materials were fine grounded with a homogenizer (Homogenizer type MPW-302, Poland) and stored in hermetically sealed glass jars at room temperature. Plant material was extracted by maceration technique using methanol (98%) as solvent. Grinded plant materials (sample size was up to 1 mm) were soaked with solvent at 10:1 ratio (solvent-to sample, v/w). The mixture was vortexed for 1–2 minutes and left in refrigerator at 5 °C for 24 h [25],[26]. Further, the residue was extracted three times at the same manner in order to achieve maximal extraction of active compounds. Then, the supernatant was filtered by Whatmann N1 filter and the filtrate was dried at room temperature (in BOV-50V drying oven with 2XZ-2 vacuum pump, Biobase China). Dried crude extracts were weighed and kept at –20 °C till further use.
2.2. Determination of total phenolic content
Total phenolic content of plant extracts was measured exploiting the Folin–Ciocalteu (FC) reagent [26] employing a calibration curve of gallic acid (GA) (0–250 µg/mL) and distilled water was used as blank. The total phenolic content was expressed in terms of GA equivalents (GAE) per g extract dry weight.
2.3. Cell cultures used
Investigated cell lines (BV-2, Acyl-CoA oxidase 1 (ACOX1) deficient mutants (Acox1-/-) and WT cells) were provided by the Laboratory BioPeroxIL: Laboratoire de Biochimie du Peroxysome, Inflammation et MétabolismeLipidique, Université de Bourgogne, Dijon, France.
2.4. BV-2 microglia cell culture
Murine microglial BV-2 cell lines (BV-2, Acyl-CoA oxidase 1 (ACOX1) deficient mutants (Acox1-/-) and WT cells) (the passage numbers of cell lines were 10–12) were grown in a 5% CO2 incubator at 37 °C in Dulbecco's modified Eagle medium (DMEM) supplemented with 10% (v/v) heat inactivated fetal bovine serum (FBS) and 1% antibiotics (penicillin, streptomycin). BV-2 cells were seeded on 96-well microplates at 25 × 104 cells per well for viability assay while 6-well microplates at 5 × 105 cells per well—for enzymatic activity determination.
2.5. MTT assay
Cell proliferation (mitochondrial activity) was measured using 3-(4,5-dimethyltrazol-2-yl)-2,5-diphenyltetrazolium bromide (MTT) assay. Cells, plated in 96-wells plates, were treated for 24–72 h with various concentrations of extract (0.004–400 µg/mL) and were incubated for 2 h with MTT followed by the absorbance (Abs) measurement at the 570 nm. The sub-cytotoxic concentration of extract was selected for further investigations [27],[28]. For all assays the extract was dissolved in 1% dimethyl sulfoxide (DMSO). The MTT test was carried out to clarify the effect of H. alpestre extract on microglial cell viability and mitochondrial function. The untreated cells have been considered as control cells in all experiments.
2.6. BV-2 cell lysate preparation
After the treatment of BV-2 microglia cells with plant extract, cells were washed with Phosphate-buffered saline (PBS) and were lysed in 50 µL of radioimmunoprecipitation (RIPA) buffer: 50 mM Tris-HCl, pH 7.4, 1% NP-40, 0.5% Na-deoxycholate, 0.1% sodium dodecylsulfate, 150 mM NaCl, 2 mM ethylenediaminetetraacetic acid (EDTA), 50 mM NaF. Lysate was cleared by centrifugation at 20,000 × g for 20 min (at –4 °C) as described previously.
Protein content was measured according to Smith et al., 1985 [29].
2.7. Enzymatic activity measurement
Catalase activity was measured according to Cherkaoui-Malki et al. [30] and was expressed as units/mg of protein.
ACOX1 activity measurement was carried out, as described by Oaxaca-Castillo D. et al. [31] using fresh cell lysate.
Total SOD activity measurement was performed according to the Beauchamp and Fridovich [32].
2.8. Data processing
A statistical analysis was performed with the Student-t test (Excel software) for calculating the probability values; and data were considered statistically significant at a p-value of 0.05 or less.
3.
Results and discussion
H. alpestre extract can be considered as a source of polyphenolic chemicals with significant antiradical and antioxidant activities, according to several chemical-based assays, as previously stated. The total phenolic components concentration in this extract was 263.3 ± 0.61 mg GAE g–1 DW. Furthermore, a prior GC-MS investigation of crude extracts from the examined plant material identified a several chemicals, including catechol, guaiacol, vanillic acid, and 2,3-dihydro-3,5-dihydroxy-6-methyl-4H-Pyran-4-one, all of which potentially contribute to the antioxidant activity [21],[22]. Assuming these data, the further investigation of possible antioxidant and protective influence of H. alpestre crude extract was carried out using the main antioxidant enzymes of microglial cell lines as targets, described above.
3.1. The effect of H. alpestre extracts on BV2 cell viability
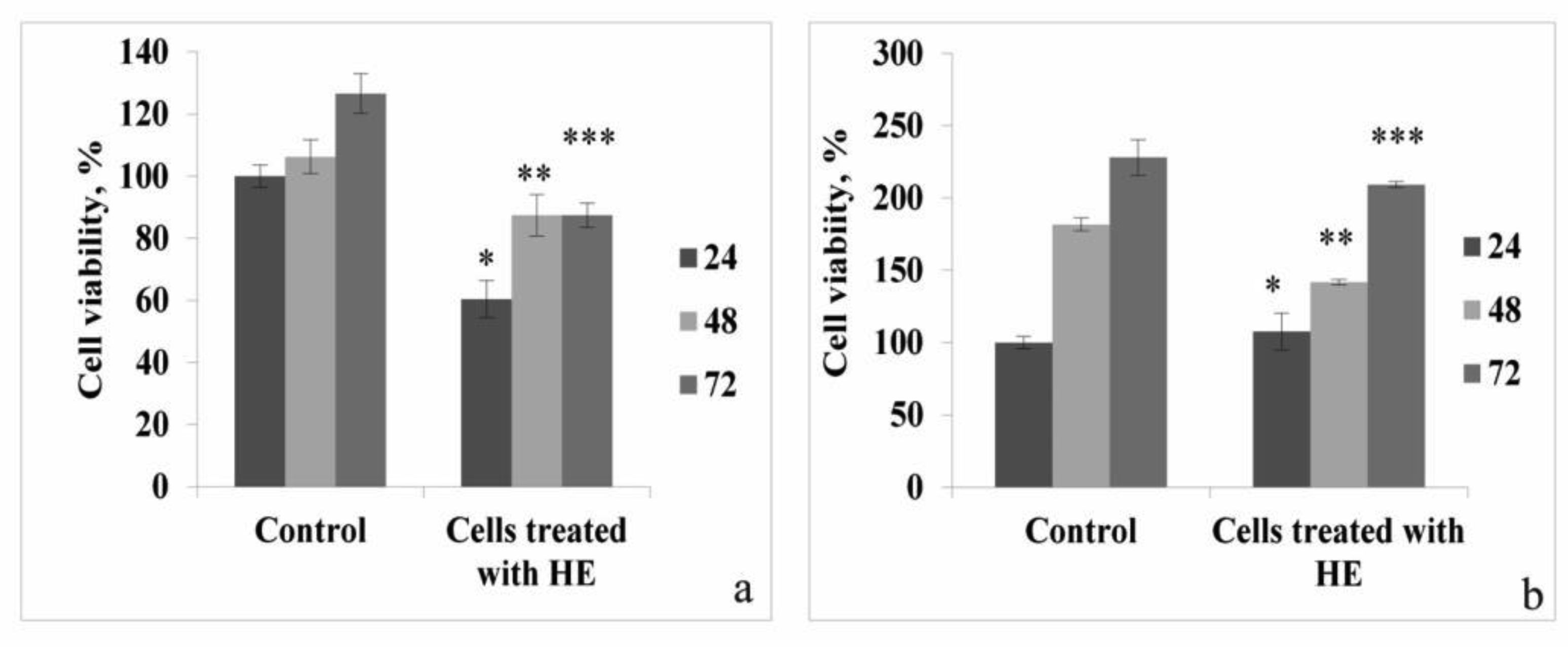
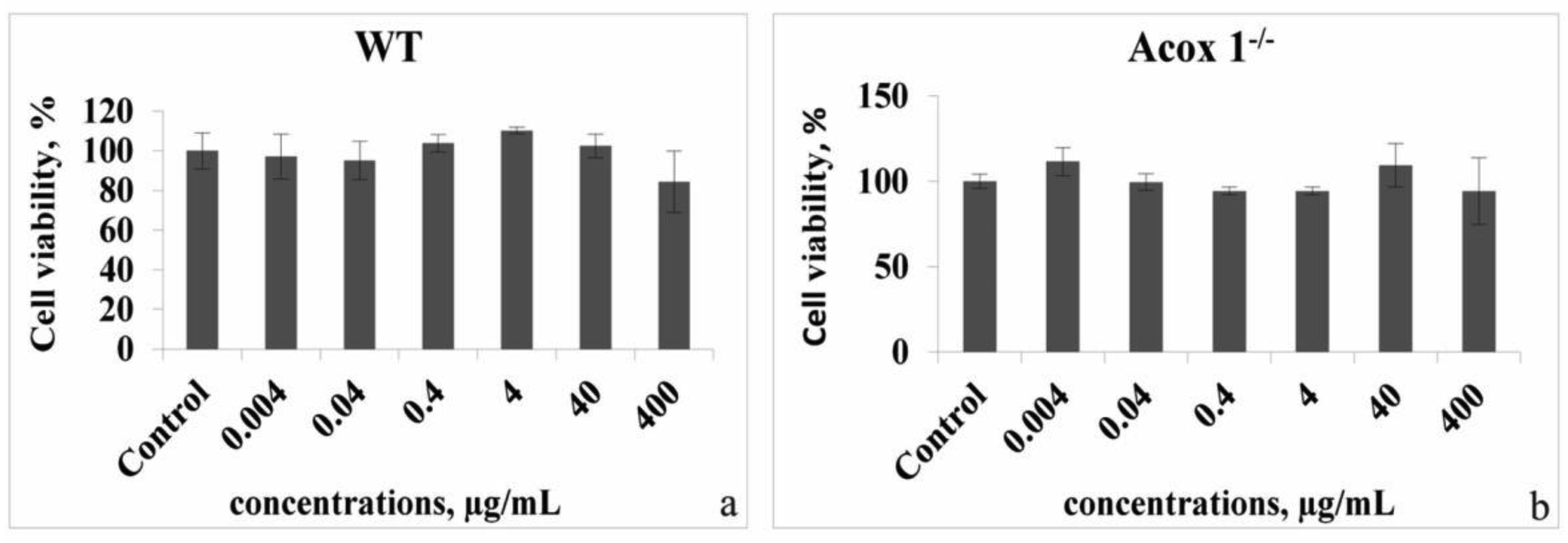
MTT test showed that the sub-cytotoxic concentration of investigated extract for both BV-2 microglial wild type (WT) cells and acyl-CoA oxidase 1 (ACOX1) deficient cell (Acox1-/-) lines was 40 µg/mL (Figure 1). This concentration of extract did not lead to adverse effects on the applied cell lines.
The concentration of 40 µg/mL of investigated extract was applied for the further treatment of both cell lines in order to investigate the dynamics of cell viability.
The results of our experiments showed that the viability of the both cell lines generally was decreased under the treatment by H. alpestre extract (HE) during the all period of treatment (24–72 h). But surprisingly, the WT cells were more susceptible to the suppression effect of extracts obtained from the high-altitude H. alpestre herb. This phenomenon may be explained by the delayed “response” of some enzymes in these cells. Meanwhile changes in the viability of Acox1 deficient cells were not significant (Figure 2).
In this case several compensatory mechanisms should be activated by adaptive response of cells, namely some increase in the activity of the main antioxidant enzymes should occur [33]. In some investigations there were mentioned the dose-depended influence of extracts of some species of Hypericum genus [34], but there were not any data concerning H. alpestre.
3.2. The effect of H. alpestre extracts on peroxisomal function on microglial BV-2 cells
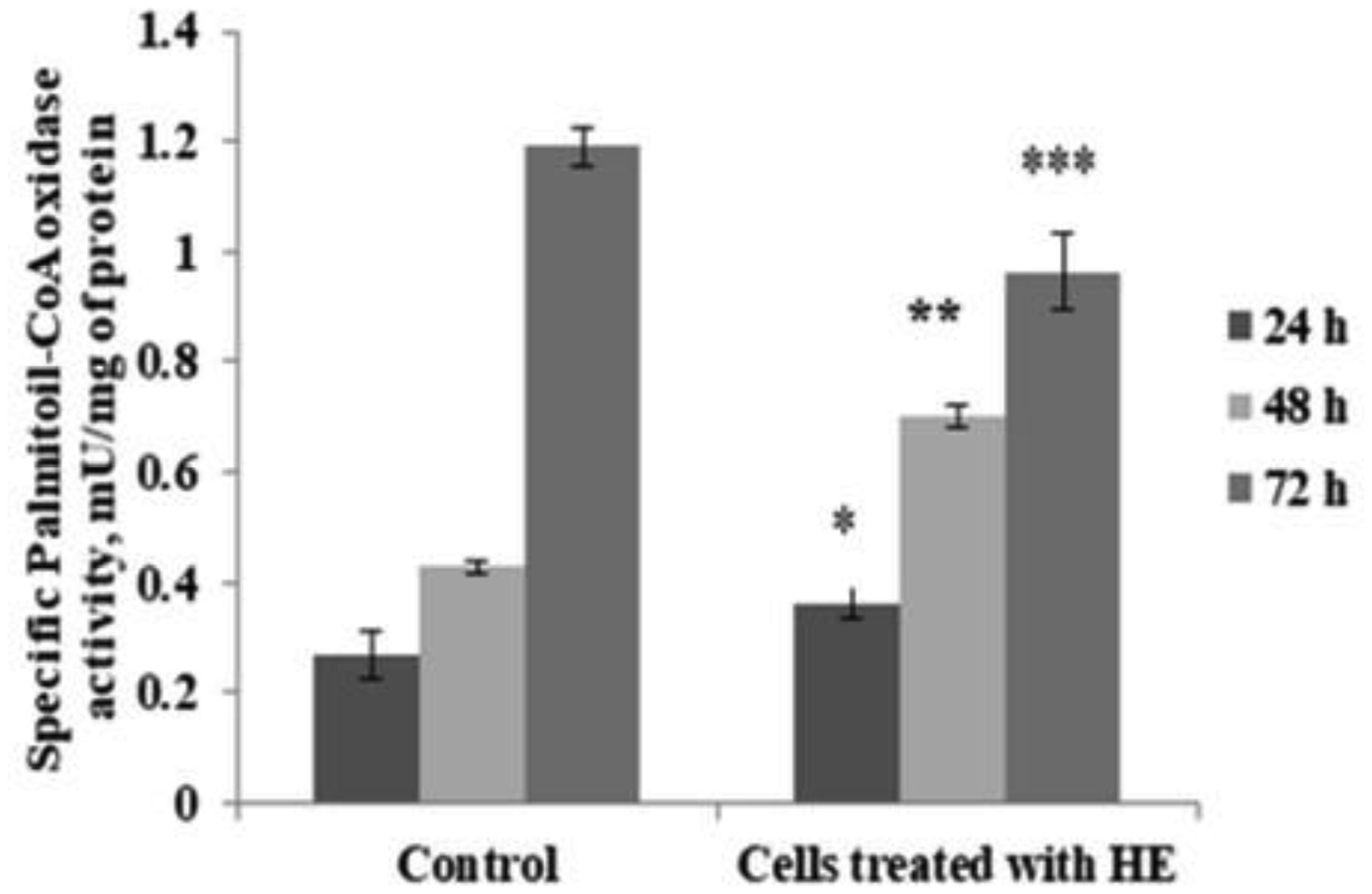
The evaluation of activity of the main antioxidant enzymes in peroxisomes of BV-2 cells (ACOX1—the rate-limiting enzyme of β-oxidation process in cells; catalase—antioxidant enzyme, quenching hydrogen peroxide) under the treatment of HE is of interest in order to assess the neuroprotective capacity of test-extract. The similar effect observed in case of using the extracts obtained from Hypericum other species [34]. The results showed that during the treatment, some statistically significant changes were revealed in case of the activity of palmitoyl-CoA oxidase (Figure 3.). Our results showed that the significant modulation in activity of palmitoyl-CoA oxidase was observed only during the 48 h treatment of BV-2 WT cells by HE sub-cytotoxic concentration. The optimal treatment period of 48 h was observed also in case of the other similar experiments [35].
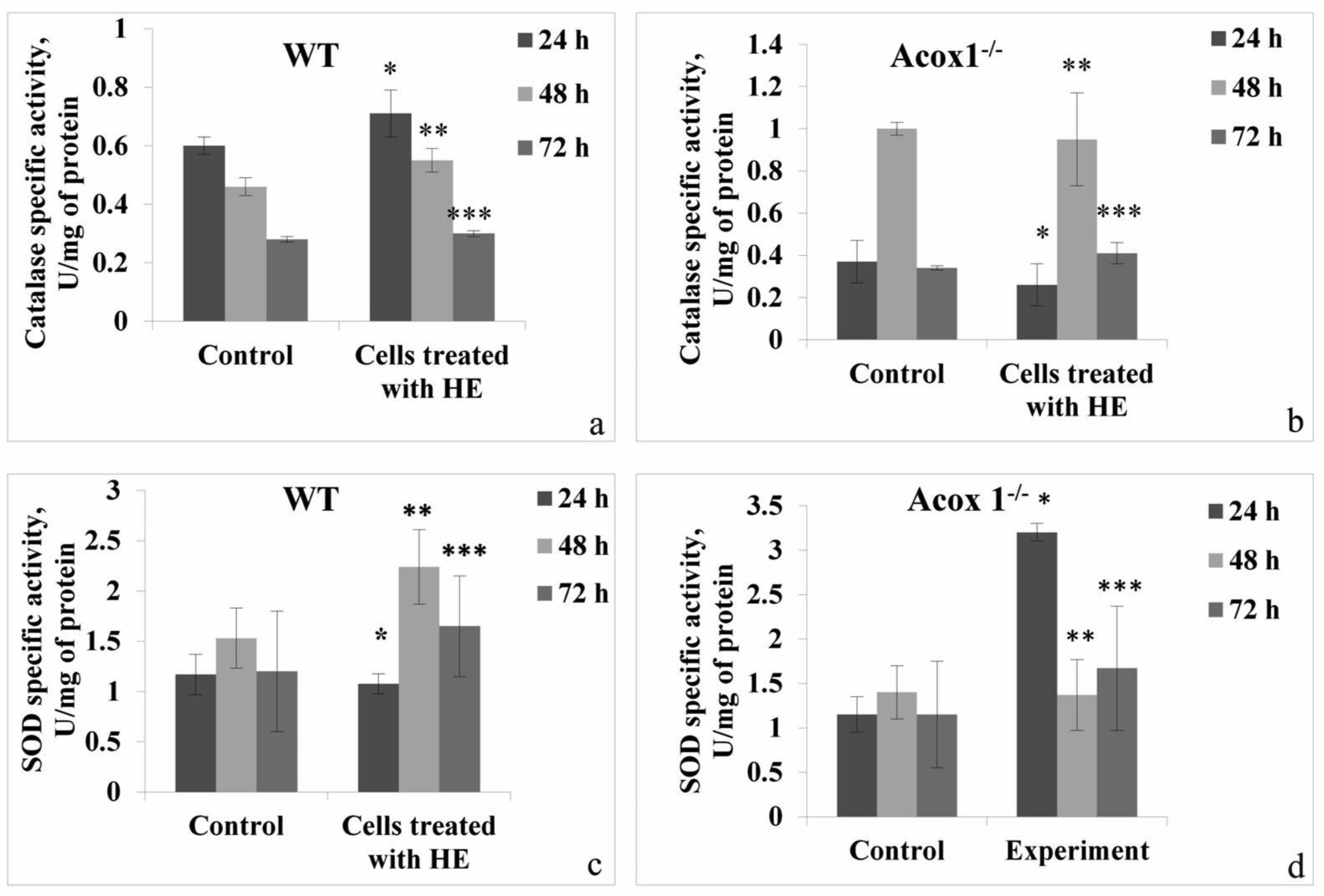
The further investigations showed that catalase activity was not significantly changed during the all period of treatment in both cell lines, meanwhile, SOD activity increased (up to 30%) in WT cells during the 48 h treatment. In case of Acox-/- cells a significant increase in SOD activity (up to 50%) was observed under the 24 h treatment (Figure 4). The upregulation of the SOD activity and downregulation of catalase activity can be the evidence of the stress in both BV2 cell lines under the influence of phytochemicals extracted from the aerial parts of H. alpestre. Therefore, it can be supposed that these substances are acting in the applied cell lines mainly as pro-oxidants. On the other hand, as the palmitoil-CoA oxidase deficient cells are serving as a model of oxidative stress [23],[35] the increase of SOD activity in these cells under the treatment of H. alpestre extracts, as well as the fact of high viability (in comparison to the WT cells) (Figure 2) can speak about the protective influence of investigated extract. There were reports about prooxidant role of extracts H. perforatum one of the well-known species within this genus, which could be due to the compound hypericin contained in the extract, known to be acting as pro-oxidant [36],[37]. Based on our results it can be concluded that H. alpestre extract also have pro-oxidant activity. This feature can be taken into the consideration in the development of anti-cancer preparations.
It is known that VLCFAs, under the influence of palmitoil-CoA oxidase, converted to the acetyl-CoA, which, in turn, comes out of peroxisomes and are reused by cells, entering the tricarboxylic acid cycle and serving as an energy source [31]. The energy released in this process can be used to overcome the oxidative stress. Thus, it can be supposed that in case of the increase of the palmitoil-CoA oxidase activity in WT cells the additional portion of energy may release in order to overcome the oxidative stress which, in its turn, was possibly generated by the plant extracts. At the same time, in palmitoil-CoA oxidase-deficient cells, the responsibility for overcoming the oxidative stress lies with SOD, where an immediate and almost two-fold high activity was observed (Figures 3 and 4).
On the other hand, it is well-known that plant metabolites can exhibit anticancer action through their selective pro-oxidant activity. ROS manipulation can induce selective apoptosis to the cancer cell as normal cells have a different redox environment compared to cancer cells and are less sensitive to redox manipulation [38]. Taking into account above mentioned as well as the obtained results it is meaningful also to evaluate the pro-oxidant activity of the H. alpestre extracts in cancer cell lines as well as to assess the anticancer potential of this plant extract in in vitro and in vivo models.
4.
Conclusions
Summarizing the obtained data, it can be concluded that H. alpestre methanolic extract possessed remarkable antioxidant property in chemical-based tests. However, the applied concentration rather exhibits pro-oxidant activity in cellular models, thereby expressing dose-dependent cytotoxic effect. Considering that phytochemicals can exhibit anticancer activity through their pro-oxidant influence, this finding can serve as a basis for further evaluation of plant extracts anticancer potential. Although, according to literature data, many species within Hypericum possess neuroprotective activity we were not able to state this surmise in H. alpestre extracts.









 DownLoad:
DownLoad: