Search Advanced

AIMS Environmental Science

2020, Volume 7, Issue 4: 302-319. doi: 10.3934/environsci.2020019
Previous Article Next Article
Research article Special Issues

Occurrence and aquatic toxicity of contaminants of emerging concern (CECs) in tributaries of an urbanized section of the Delaware River Watershed

  • 1.
    NSF - Water and Environmental Technology (WET) Center, Civil and Environmental Engineering Department, Temple University, Philadelphia PA, USA
  • 2.
    Delaware River Basin Commission (DRBC), West Trenton, NJ, USA
  • Received: 13 May 2020 Accepted: 06 July 2020 Published: 09 July 2020

  • The presence of contaminants of emerging concern (CECs) in environmental matrices is an ongoing issue. This research project was carried out to increase our understanding of the loading, distribution and potential risk of CECs by sampling large and small tributaries in a specific area of the Delaware River watershed (in northeast USA) that is highly urbanized and significantly impacted by wastewater treatment plant effluents. Fifteen target compounds were selected for analysis based on their high frequency of detection in a previous multiyear study conducted on the Delaware River mainstem. Ten sampling sites were chosen on tributaries receiving numerous municipal and industrial discharges. Sampling locations were above and below potential source discharges. Sampling was designed to assess seasonal differences in CECs loadings. The measured environmental concentrations of the target compounds present a detailed picture of urban and industrial impacts on subwatershed receiving waters. An index of concern ranking system was applied to the sample locations by comparing measured environmental concentrations, existing target compound water quality criteria or predicted no effects levels and developing a concern summary variable. Triclocarban and diphenhydramine demonstrated to be compounds of high relative risk (RR) to the aquatic life of the Pennsylvania tributaries to the Delaware River.

    Citation: Djordje Vilimanovic, Gangadhar Andaluri, Robert Hannah, Rominder Suri, A. Ronald MacGillivray. Occurrence and aquatic toxicity of contaminants of emerging concern (CECs) in tributaries of an urbanized section of the Delaware River Watershed[J]. AIMS Environmental Science, 2020, 7(4): 302-319. doi: 10.3934/environsci.2020019

    Related Papers:

  • The presence of contaminants of emerging concern (CECs) in environmental matrices is an ongoing issue. This research project was carried out to increase our understanding of the loading, distribution and potential risk of CECs by sampling large and small tributaries in a specific area of the Delaware River watershed (in northeast USA) that is highly urbanized and significantly impacted by wastewater treatment plant effluents. Fifteen target compounds were selected for analysis based on their high frequency of detection in a previous multiyear study conducted on the Delaware River mainstem. Ten sampling sites were chosen on tributaries receiving numerous municipal and industrial discharges. Sampling locations were above and below potential source discharges. Sampling was designed to assess seasonal differences in CECs loadings. The measured environmental concentrations of the target compounds present a detailed picture of urban and industrial impacts on subwatershed receiving waters. An index of concern ranking system was applied to the sample locations by comparing measured environmental concentrations, existing target compound water quality criteria or predicted no effects levels and developing a concern summary variable. Triclocarban and diphenhydramine demonstrated to be compounds of high relative risk (RR) to the aquatic life of the Pennsylvania tributaries to the Delaware River.


    加载中


    [1] Fent K, Weston AA, Caminada D (2006) Ecotoxicology of human pharmaceuticals. Aquat Toxicol 76: 122-159. doi: 10.1016/j.aquatox.2005.09.009
    [2] Barceló D, Petrovic M (2007) Pharmaceuticals and personal care products (PPCPs) in the environment. Anal Bioanal Chem 387: 1141-1142. doi: 10.1007/s00216-006-1012-2
    [3] Kim JW, Jang HS, Kim JG, et al. (2009) Occurrence of Pharmaceutical and Personal Care Products (PPCPs) in Surface Water from Mankyung River, South Korea. J Heal Sci 55: 249-258. doi: 10.1248/jhs.55.249
    [4] Vidal-Dorsch DE, Bay SM, Maruya K, et al. (2012) Contaminants of emerging concern in municipal wastewater effluents and marine receiving water. Environ Toxicol Chem 31: 2674-2682. doi: 10.1002/etc.2004
    [5] Pal A, Gin KYH, Lin AYC, et al. (2010) Impacts of emerging organic contaminants on freshwater resources: Review of recent occurrences, sources, fate and effects. Sci Total Environ 408: 6062-6069. doi: 10.1016/j.scitotenv.2010.09.026
    [6] Blair BD, Crago JP, Hedman CJ, et al. (2013) Pharmaceuticals and personal care products found in the Great Lakes above concentrations of environmental concern. Chemosphere 93: 2116-2123. doi: 10.1016/j.chemosphere.2013.07.057
    [7] Fairbairn DJ, Karpuzcu ME, Arnold WA, et al. (2015) Sediment-water distribution of contaminants of emerging concern in a mixed use watershed. Sci Total Environ 505: 896-904. doi: 10.1016/j.scitotenv.2014.10.046
    [8] Paíga P, Santos LHMLM, Ramos S, et al. (2016) Presence of pharmaceuticals in the Lis river (Portugal): Sources, fate and seasonal variation. Sci Total Environ 573: 164-177. doi: 10.1016/j.scitotenv.2016.08.089
    [9] Andaluri G, Suri RPS, Graham K (2017) Steroid hormones in environmental matrices: extraction method comparison. Environ Monit Assess 189: 626. doi: 10.1007/s10661-017-6345-0
    [10] Andaluri G, Suri RPS, Kumar K (2012) Occurrence of estrogen hormones in biosolids, animal manure and mushroom compost. Environ Monit Assess 184: 1197-1205. doi: 10.1007/s10661-011-2032-8
    [11] Bean TG, Rattner BA, Lazarus RS, et al. (2018) Pharmaceuticals in water, fish and osprey nestlings in Delaware River and Bay. Environ Pollut 232: 533-545. doi: 10.1016/j.envpol.2017.09.083
    [12] Jones OAH, Voulvoulis N, Lester JN (2002) Aquatic environmental assessment of the top 25 English prescription pharmaceuticals. Water Res 36: 5013-5022. doi: 10.1016/S0043-1354(02)00227-0
    [13] Carlsson C, Johansson AK, Alvan G, et al. (2006) Are pharmaceuticals potent environmental pollutants?. Part I: Environmental risk assessments of selected active pharmaceutical ingredients. Sci Total Environ 364: 67-87.
    [14] Sui Q, Huang J, Deng S, et al. (2010) Occurrence and removal of pharmaceuticals, caffeine and DEET in wastewater treatment plants of Beijing, China. Water Res 44: 417-426. doi: 10.1016/j.watres.2009.07.010
    [15] Hansen M, Krogh KA, Björklund E, et al. (2009) Environmental risk assessment of ionophores. TrAC - Trends Anal Chem 28: 534-542. doi: 10.1016/j.trac.2009.02.015
    [16] EEA (2014) Chapter 6: Ecological Risk Assessment. Eur Environ Agency 4-7.
    [17] Higgins CP, Paesani ZJ, Chalew TEA, et al. (2009) Pharmaceuticals and Personal Care Products in the Environment BIOACCUMULATION OF TRICLOCARBAN IN LUMBRICULUS VARIEGATUS. Environ Toxicol 28: 2663-2670. doi: 10.1897/08-485.1
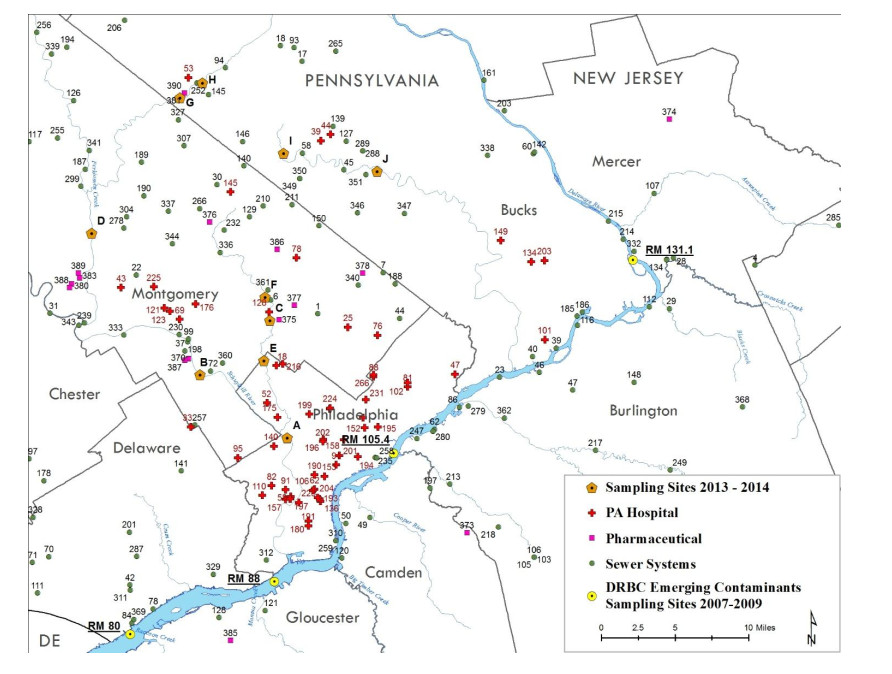
    [18] DRBC (2019) State of the Basin.
    [19] MacGillivray AR (2013) Contaminants of Emerging Concern In the Tidal Delaware River.
    [20] USEPA (2007) Method 1694: Pharmaceuticals and Personal Care Products in Water, Soil, Sediment, and Biosolids by HPLC / MS / MS.
    [21] CENSUS UBO (2010) U.S. Census Bureau, US Census Bureau 2010 Census, 2010. Available from: http://www.census.gov/2010census/.
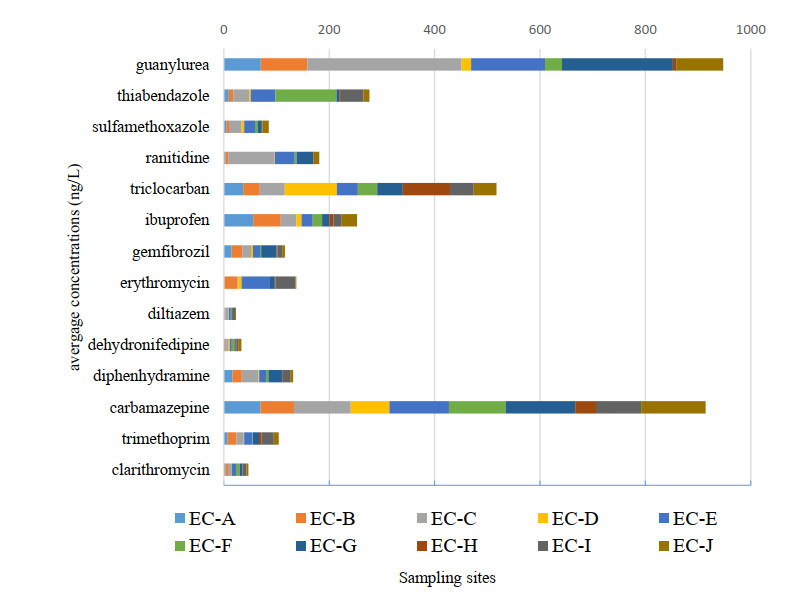
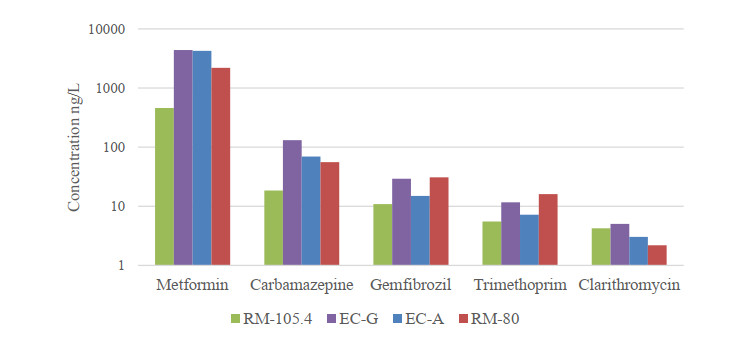
    [22] Scheurer M, Michel A, Brauch HJ, et al. (2012) Occurrence and fate of the antidiabetic drug metformin and its metabolite guanylurea in the environment and during drinking water treatment. Water Res 46: 4790-4802. doi: 10.1016/j.watres.2012.06.019
    [23] Kosma CI, Lambropoulou DA, Albanis TA (2015) Comprehensive study of the antidiabetic drug metformin and its transformation product guanylurea in Greek wastewaters. Water Res 70: 436-448. doi: 10.1016/j.watres.2014.12.010
    [24] Niemuth NJ, Jordan R, Crago J, et al. (2015) Metformin exposure at environmentally relevant concentrations causes potential endocrine disruption in adult male fish. Environ Toxicol Chem 34: 291-296. doi: 10.1002/etc.2793
    [25] Karpuzcu ME, Fairbairn D, Arnold WA, et al. (2014) Identifying sources of emerging organic contaminants in a mixed use watershed using principal components analysis. Environ Sci Process Impacts 16: 2390-2399. doi: 10.1039/C4EM00324A
    [26] Trautwein C, Kümmerer K (2011) Incomplete aerobic degradation of the antidiabetic drug Metformin and identification of the bacterial dead-end transformation product Guanylurea. Chemosphere 85: 765-773. doi: 10.1016/j.chemosphere.2011.06.057
    [27] Tamura I, Kagota KI, Yasuda Y, et al. (2013) Ecotoxicity and screening level ecotoxicological risk assessment of five antimicrobial agents: Triclosan, triclocarban, resorcinol, phenoxyethanol and p-thymol. J Appl Toxicol 33: 1222-1229.
    [28] WET Center (2016) WET Center Pharmaceutical PNEC list.
    [29] Ferrari B, Mons R, Vollat B, et al. (2004) Environmental Risk Assessment of Six Human Pharmaceuticals: Are the Current Environmental Risk Assessment Procedures Sufficient for the Protection of the Aquatic Environment? Environ Toxicol Chem 23: 1344. doi: 10.1897/03-246
    [30] Ferrari G, Junghans M, Korkaric M, et al. (2019) Antibiotikaresistenzbildung in der Umwelt. Herleitung von UQK für Antibiotika unter Berücksichtigung von Resistenzbildung. Aqua Gas 52-59.
    [31] Isidori M, Parrella A, Pistillo P, et al. (2009) Effects of ranitidine and its photoderivatives in the aquatic environment. Environ Int 35: 821-825. doi: 10.1016/j.envint.2008.12.002
    [32] Isidori M, Nardelli A, Pascarella L, et al. (2007) Toxic and genotoxic impact of fibrates and their photoproducts on non-target organisms. Environ Int 33: 635-641. doi: 10.1016/j.envint.2007.01.006
    [33] US EPA (2014) Ecological Structure Activity Relationships (ECOSAR).
    [34] Deo RP (2014) Pharmaceuticals in the Surface Water of the USA: A Review. Curr Environ Heal reports 1: 113-122. doi: 10.1007/s40572-014-0015-y
    [35] Kim Y, Choi K, Jung J, et al. (2007) Aquatic toxicity of acetaminophen, carbamazepine, cimetidine, diltiazem and six major sulfonamides, and their potential ecological risks in Korea. Environ Int 33: 370-375. doi: 10.1016/j.envint.2006.11.017
    [36] Cunningham VL, Buzby M, Hutchinson T, et al. (2006) Effects of human pharmaceuticals on aquatic life: Next steps. Environ Sci Technol 40: 3456-3462. doi: 10.1021/es063017b
    [37] ECHA (2008) Guidance on information requirements and chmical safety assessment. Chapter R.10: Characterisation of dose [concentration]-response for environment. Eur Chem Agency 1-65.
    [38] Caldwell JC, Evans M V., Krishnan K (2012) Cutting edge PBPK models and analyses: Providing the basis for future modeling efforts and bridges to emerging toxicology paradigms. J Toxicol 2012: 1-10.
    [39] Heidler J, Sapkota A, Halden RU (2006) Partitioning, persistence, and accumulation in digested sludge of the topical antiseptic triclocarban during wastewater treatment. Environ Sci Technol 40: 3634-3639. doi: 10.1021/es052245n
    [40] Brausch JM, Rand GM (2011) A review of personal care products in the aquatic environment: Environmental concentrations and toxicity. Chemosphere 82: 1518-1532. doi: 10.1016/j.chemosphere.2010.11.018
    [41] DeLeo PC, Sedlak RI (2014) Comment on 'on the need and speed of regulating triclosan and triclocarban in the United States'. Environ Sci Technol 48: 11021-11022. doi: 10.1021/es503494j
    [42] U.S. Food and Drug Administration (2016) Focus on Surfactants, FDA issues final rule on safety and effectiveness of antibacterial soaps, 2016. Available from: https://www.fda.gov/news-events/press-announcements/fda-issues-final-rule-safety-and-effectiveness-antibacterial-soaps.
    [43] Halden RU (2020) Triclosan and Triclocarban: Exposures, Toxicity and Testing - Environmental Health Symposium, 2020. Available from: http://environmentalhealthsymposium.com/blog/2020/2/3/triclosan-and-triclocarbon-exposures-toxicity-and-testing.
    [44] Berninger JP, Du B, Connors KA, et al. (2011) Effects of the antihistamine diphenhydramine on selected aquatic organisms. Environ Toxicol Chem 30: 2065-2072. doi: 10.1002/etc.590
    [45] Ramirez AJ, Mottaleb MA, Brooks BW, et al. (2007) Analysis of pharmaceuticals in fish using liquid chromatography-tandem mass spectrometry. Anal Chem 79: 3155-3163. doi: 10.1021/ac062215i
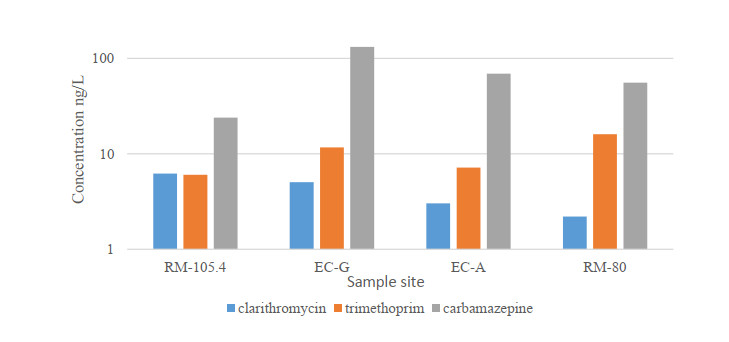
    [46] Andreozzi R, Marotta R, Pinto G, et al. (2002) Carbamazepine in water: Persistence in the environment, ozonation treatment and preliminary assessment on algal toxicity. Water Res 36: 2869-2877. doi: 10.1016/S0043-1354(01)00500-0
    [47] Garber AJ, Duncan TG, Goodman AM, et al. (1997) Efficacy of metformin in type II diabetes: Results of a double-blind, placebo-controlled, dose-response trial. Am J Med 103: 491-497. doi: 10.1016/S0002-9343(97)00254-4
    [48] Ecotox Centre Eawag-EPFL (2017) Proposals for Acute and Chronic Quality Standards | Oekotoxzentrum, 2017. Available from: http://www.ecotoxcentre.ch/expert-service/quality-standards/proposals-for-acute-and-chronic-quality-standards/.
    [49] Isidori M, Lavorgna M, Nardelli A, et al. (2005) Toxic and genotoxic evaluation of six antibiotics on non-target organisms. Sci Total Environ 346: 87-98. doi: 10.1016/j.scitotenv.2004.11.017
    [50] Danner MC, Robertson A, Behrends V, et al. (2019) Antibiotic pollution in surface fresh waters: Occurrence and effects. Sci Total Environ 664: 793-804. doi: 10.1016/j.scitotenv.2019.01.406
    [51] Luo Y, Guo W, Ngo HH, et al. (2014) A review on the occurrence of micropollutants in the aquatic environment and their fate and removal during wastewater treatment. Sci Total Environ 473-474: 619-641. doi: 10.1016/j.scitotenv.2013.12.065
    [52] Hirsch R, Ternes T, Haberer K, et al. (1999) Occurrence of antibiotics in the aquatic environment. Sci Total Environ 225: 109-118. doi: 10.1016/S0048-9697(98)00337-4
    [53] US EPA (2004) Overview of the Ecological Risk Assessment Process in the Office of Pesticide Programs - Endangered and Threatened Species Effects Determinations.
    [54] He W, Goodkind D, Kowal P (2016) An Aging World: 2015 International Population Reports. Aging (Albany NY) 165.
    [55] Nakashima M, Canda ER (2005) Positive dying and resiliency in later life: A qualitative study. J Aging Stud 19: 109-125. doi: 10.1016/j.jaging.2004.02.002
    [56] OECD (2019) Pharmaceutical Residues in Freshwater - Hazards and Policy Responses
    [57] Christensen NS, Wood AW, Voisin N, et al. (2004) The effects of climate change on the hydrology and water resources of the Colorado River basin. Clim Change 62: 337-363. doi: 10.1023/B:CLIM.0000013684.13621.1f
    [58] Pennsylvania Environmental Council (2020) Stormwater Resources for Philadelphia & Urban Centers, 2020. Available from: https://pecpa.org/stormwater-philadelphia-urban-centers/.
    [59] Kricun A (2018) Using a Triple Bottom Line Approach To Reduce Combined Sewage Flooding and Provide Community Benefit in Camden City.
    [60] Jeffries KM, Brander SM, Britton MT, et al. (2015) Chronic exposures to low and high concentrations of ibuprofen elicit different gene response patterns in a euryhaline fish. Environ Sci Pollut Res 22: 17397-17413. doi: 10.1007/s11356-015-4227-y
    [61] MedlinePlus (NIH) (2015) Diphenhydramine: MedlinePlus Drug Information, 2015. Available from: https://www.nlm.nih.gov/medlineplus/druginfo/meds/a682539.html.
    [62] National Cancer Institute (2019) NCI Thesaurus, 2019. Available from: https://ncit.nci.nih.gov/ncitbrowser/ConceptReport.jsp?dictionary=NCI_Thesaurus&ns=ncit&code=C873.
    [63] Cerner Multum I (2010) Thiabendazole Uses, Side Effects & Warnings - Drugs.com, 2010. Available from: https://www.drugs.com/mtm/thiabendazole.html.
    [64] Johnson AC, Keller V, Dumont E, et al. (2015) Assessing the concentrations and risks of toxicity from the antibiotics ciprofloxacin, sulfamethoxazole, trimethoprim and erythromycin in European rivers. Sci Total Environ 511: 747-755. doi: 10.1016/j.scitotenv.2014.12.055
    [65] Wright SW, Wrenn KD, Haynes ML (1999) Trimethoprim-sulfamethoxazole resistance among urinary coliform isolates. J Gen Intern Med 14: 606-609. doi: 10.1046/j.1525-1497.1999.10128.x
    [66] Heberer T (2002) Tracking persistent pharmaceutical residues from municipal sewage to drinking water. J Hydrol 266: 175-189. doi: 10.1016/S0022-1694(02)00165-8
    [67] PWD (2011) Schuylkill | Philadelphia Water Department, 2011. Available from: http://www.phillywatersheds.org/your_watershed/schuylkill.
    [68] Sunger N, Teske SS, Nappier S, et al. (2012) Recreational use assessment of water-based activities, using time-lapse construction cameras. J Expo Sci Environ Epidemiol 22: 281-290. doi: 10.1038/jes.2012.4
    [69] Cunningham VL, Binks SP, Olson MJ (2009) Human health risk assessment from the presence of human pharmaceuticals in the aquatic environment. Regul Toxicol Pharmacol 53: 39-45. doi: 10.1016/j.yrtph.2008.10.006
    [70] Kostich MS, Lazorchak JM (2008) Risks to aquatic organisms posed by human pharmaceutical use. Sci Total Environ 389: 329-339. doi: 10.1016/j.scitotenv.2007.09.008
    [71] Kostich MS, Batt AL, Lazorchak JM (2014) Concentrations of prioritized pharmaceuticals in effluents from 50 large wastewater treatment plants in the US and implications for risk estimation. Environ Pollut 184: 354-359. doi: 10.1016/j.envpol.2013.09.013
    [72] Schwab BW, Hayes EP, Fiori JM, et al. (2005) Human pharmaceuticals in US surface waters: A human health risk assessment. Regul Toxicol Pharmacol 42: 296-312. doi: 10.1016/j.yrtph.2005.05.005
    [73] Collier AC (2007) Pharmaceutical contaminants in potable water: Potential concerns for pregnant women and children. Ecohealth 4: 164-171. doi: 10.1007/s10393-007-0105-5
    [74] Bruce GM, Pleus RC, Snyder SA (2010) Toxicological relevance of pharmaceuticals in drinking water. Environ Sci Technol 44: 5619-5626. doi: 10.1021/es1004895
    [75] Kumar A, Xagoraraki I (2010) Human health risk assessment of pharmaceuticals in water: An uncertainty analysis for meprobamate, carbamazepine, and phenytoin. Regul Toxicol Pharmacol 57: 146-156. doi: 10.1016/j.yrtph.2010.02.002
    [76] Kumar A, Xagoraraki I (2010) Pharmaceuticals, personal care products and endocrine-disrupting chemicals in U.S. surface and finished drinking waters: A proposed ranking system. Sci Total Environ 408: 5972-5989.
    [77] Ottmar KJ, Colosi LM, Smith JA (2010) Development and application of a model to estimate wastewater treatment plant prescription pharmaceutical influent loadings and concentrations. Bull Environ Contam Toxicol 84: 507-512. doi: 10.1007/s00128-010-9990-3
  • Environ-07-04-19-s1.pdf
  • Reader Comments
  • © 2020 the Author(s), licensee AIMS Press. This is an open access article distributed under the terms of the Creative Commons Attribution License (http://creativecommons.org/licenses/by/4.0)
通讯作者: 陈斌, bchen63@163.com
  • 1. 

    沈阳化工大学材料科学与工程学院 沈阳 110142

  1. 本站搜索
  2. 百度学术搜索
  3. 万方数据库搜索
  4. CNKI搜索
AIMS Environmental Science
0.7

Metrics

Article views(1176) PDF downloads(261) Cited by(2)

Download PDF
Download XML
Export Citation
Article outline

Figures and Tables

Figures(4)  /  Tables(3)

Other Articles By Authors

Related pages

Tools

/

DownLoad:  Full-Size Img  PowerPoint
Return
Return

Catalog