On a class of fixed points for set contractions on partial metric spaces with a digraph

1.   Introduction

Fixed point theory is a powerful tool for solving a variety of mathematical problems with various types of applications ^[18,31]. The study of fixed points of metric spaces equipped with a graph structure occupies a prominent role in many aspects. Initially, the existence of fixed points in ordered metric spaces was studied by Ran and Reurings ^[25]. Many researchers have obtained fixed-point results for single-valued and set-valued mappings defined on partially ordered metrics spaces (see^{[4,13,17,20,21,22,23,24,28]}). Jachymski and Jozwik ^[15] introduced a new approach in metric fixed-point theory by replacing the order structure with a graph structure on a metric space. Abbas et al. ^[2] obtained some fixed point of multivalued contraction mappings on metric spaces with a directed graph. Several useful fixed-point results for single-valued and multivalued mappings appear in ^[5,11,12,14].

Matthews ^[19] introduced the concept of a partial metric as a part of the study of denotational semantics of dataflow networks. He gave a modified version of the Banach contraction principle, which is more suitable in the aforementioned context (see also ^{[26,27,29,30]}).

Abbas et al. ^[1,3] established the existence of fixed-point results for set-contractions in the setup of a metric space and partial metric space, respectively, with a graph structure. Recently, Latif et al. ^[16] established some fixed point results for a class of set-contraction mappings endowed with a digraph structure.

In this paper, we prove fixed-point results for set-valued maps based on the family of closed and bounded subsets of a partial metric space endowed with a graph structure while satisfying generalzied graph $\phi$-contractive conditions. It is worth emphasizing that we do not rely on the imposed strong conditions used to obtain the results in ^[1]. To reiterate, our main components in the proofs are relying on the Pompeiu-Hausdorff partial metric $H_p$, the generalized graph contraction $T$ and the generalized rational graph contraction $S$. These results extend and strengthen various known results in ^{[1,6,7,8,9,10,11,21,32]}.

Here, we use $\mathfrak{X}$ to represent the Cartesian product $X \times X$ that we will use in the following definitions and in the sequel.

Definition 1.1. ^[19] Given a non-empty set $X$, a partial metric is a function $p: \mathfrak{X} \rightarrow [0, +\infty)$ satisfying, for every element $\eta_1, \eta_2, \eta_3 \in X$ the following conditions:

(i) $p(\eta_1, \eta_1) = p(\eta_2, \eta_2) = p(\eta_1, \eta_2) \Leftrightarrow \eta_1 = \eta_2$;

(ii) $p(\eta_1, \eta_1) \leq p(\eta_1, \eta_2)$;

(iii) $p(\eta_1, \eta_2) = p(\eta_2, \eta_1)$;

(iv) $p(\eta_1, \eta_3) \leq p(\eta_1, \eta_2) + p(\eta_2, \eta_3) - p(\eta_2, \eta_2)$.

The pair $(X, p)$ is then called a partial metric space.

Notice that, $p(\eta_1, \eta_2) = 0$, then by $(ii)$, we have: $\eta_1 = \eta_2$. The converse is not always true.

An example is the pair $(\mathbb{R}^2, p)$, where the partial metric is defined by

where $\mu = \left(\mu _{1}, \mu _{2}\right)$ and $\eta = \left(\eta _{1}, \eta _{2}\right)$.

Definition 1.2. ^[19,30] Let $(X, p)$ be a partial metric space and $\{\eta_{n}\}_{n \geq 1}$ a sequence in $X$. We say that

(i) $\{\eta_{n}\}_{n \geq 1}$ converges to an element $\eta \in X$ w.r.t. the topology $\tau_{p}$ if and only if $\lim\limits_{n \rightarrow \infty} p(\eta, \eta_{n}) = p(\eta, \eta)$;

(ii) $\{\eta_{n}\} \subset X$ is a Cauchy sequence if $\lim\limits_{n, m \rightarrow \infty}p(\eta_{n}, \eta_{m})$ exists and is finite;

(iii) the set $X$ is a complete set if every Cauchy sequence $\{\eta_{n}\} \subset X$ converges to a point $\eta \in X$ such that $\lim\limits_{n, m \rightarrow \infty}p(\eta_{n}, \eta_{m}) = p(\eta, \eta)$.

We are now ready to identify a partial metric space $(X, p)$ with a graph structure. Let $G = \big(V(G), E(G)\big)$ be a directed graph, where the vertex set $V(G) = X$ and the edge set $E(G) \subseteq \mathfrak{X}$ such that $\Delta \subseteq E(G)$. Here, $\mathfrak{X}$ represents the Cartesian product $X \times X$ and $\Delta$ denotes the diagonal of $\mathfrak{X}$. The graph is allowed to have loops, but no parallel edges are allowed between distinct pairs of vertices.

Noting that, whenever $u$ and $v$ are two vertices of $G$, then a path in $G$ from $u$ to $v$ of length $k \in \mathbb{N}$ is a finite sequence $\{\eta_{n}\}_{n = 0}^k$ of vertices such that $u = \eta_{0}, v = \eta_{k}$ and $(\eta_{i-1}, \eta_{i}) \in E(G)$ for $i = 1, 2, \ldots, k$.

Graph $G$ is said to be connected if there is a directed path between any two vertices in $G$. Also, graph $G$ is said to be weakly connected if $\tilde{G}$ is connected, where $\tilde{G}$ denotes the undirected graph obtained from $G$ by ignoring the directions of edges. $G^{-1}$ called a conversion of $G$ by reversing the direction of the edge set $E(G)$. Namely,

Here and in the sequel, we consider $(X, p)$ to be a partial metric space unless specified. Denote $CB^{p}(X)$ as the set of closed and bounded subsets of $X$, with respect to the partial metric $p$. We refer the reader to the paper of Aydi et al. ^[9], where ample details of the terms closedness and boundedness are discussed in detail. Furthermore, they proved that, indeed, the mapping $H_{p}: CB^{p} \times CB^{p} \rightarrow \mathbb{R^+}$ defined as

is the analogue to the Pompeiu-Hausdorff metric induced by $p$. Here, $\delta_{p}(\chi_1, \chi_2) = \mathrm{sup}\{p(\eta_1, \chi_2) : \eta_1 \in \chi_1\}$ with $p(\eta, \chi_1) = \mathrm{inf}\{p(\eta, \chi_1) : \eta \in \chi_1\}$.

If $(X, p)$ is a complete partial metric space, then $(CB^{p}, H_{p})$ is also complete Pompeiu-Hausdorff partial metric space.

We consider the graph $G$ as defined previously. Thus, we consider that the graph $G$ is weighted; that is for each pair of edges $(\eta_{1}, \eta_2)$ in $E(G)$, the weight $p(\eta_1, \eta_2)$ is assigned to be the value of the distance $p$ at the edge $(\eta_1, \eta_2)$. Note that, since $p$ is a partial metric, we infer that the weight $p(\eta_1, \eta_1)$ assigned to the loop $(\eta_1, \eta_1)$ is not necessarily zero. Furthermore the partial Hausdorff weight that we assign to each element $U, V \in CB^{p}(X)$ need not vanish, i.e., it does not have to be zero. In particular, $U = V$ whenever $H_{p}(U, V) = 0$.

Definition 1.3. ^[1,16] Let $\chi_1$ and $\chi_2$ be elements of $CB^{p}(X)$. We say that

(i) the pair $(\chi_1, \chi_2)\subset E(G)$ forms an edge between $\chi_1$ and $\chi_2$, which means that there exists an edge for some vertices $\eta_1$ and $\eta_2$ with $(\eta_1, \eta_2) \in \chi_1 \times \chi_2$;

(ii) there exists a path between $\chi_1$ and $\chi_2$ if there exists a path for some vertices $\eta_1$ and $\eta_2$ with $(\eta_1, \eta_2) \in \chi_1 \times \chi_2$.

The relation $\mathcal{R}$ is defined as follows: We say that $\chi_1$ is in relation with $\chi_2$ ($\chi_1 R \chi_2$) if and only if there exists a path between the elements $\chi_1$ and $\chi_2$.

Note that the reflexivity, symmetry and transitivity are defined in the usual manner.

In order to study graph contraction mappings we consider the mapping: $T: CB^{p}(X) \rightarrow CB^{p}(X)$, and introduce the set below

From now onward, we set $e: = e (\chi_1 \chi_2)$ to denote the edge that connects both nodes $\chi_1$ and $\chi_2$. Similarly, we use $e_T: e(T(\chi_1) T(\chi_2))$ to denote the edge connecting $T(\chi_1)$ to $T(\chi_2)$. In a similar fashion, we also set $e_S: = e (S (\chi_1) S(\chi_2))$. Denoting $W: = (\chi_1, \chi_2 \ldots)$ and $W_T: = (T(\chi_1), T(\chi_2), \ldots)$ as the path between $\chi_1$ and $\chi_2$ and the path connecting $T(\chi_1)$ and $T(\chi_2)$, respectively. Similarly, we define the path $W_S$. With this notations in hand, we can now introduce the notions of both the generalized graph contraction and generalized rational graph contraction in the following two definitions.

Definition 1.4. We say that a set-valued mapping $T: CB^{p}(X) \rightarrow CB^{p}(X)$ is called a generalized graph $\phi$- contraction whenever the following conditions hold:

(i) $e_T$ is an edge that links $T(\chi_1)$ to $T(\chi_2)$ whenever $e$ is the preceding edge that links $\chi_1$ and $\chi_2$.

(ii) $W_T$ is a path from $T(\chi_1)$ to $T(\chi_2)$ whenever $W$ is a path from $\chi_1$ to $\chi_2$.

(iii) There exists a function $\phi: \mathbb{R}^{+} \rightarrow \mathbb{R}^{+}$ such that $\phi$ is upper semicontinuous, monotonic and non-decreasing, and that $\phi(t) < t$ for every $t > 0$, with $\sum \limits_{r = 0}^{\infty }\phi ^{r}\left(t\right)$, is convergent; and, if $e$ is an edge from $\chi_1$ to $\chi_2$, we infer that

where

Definition 1.5. Let $S: CB^{p}(X) \rightarrow CB^{p}(X)$ be the set-valued mapping defined from $CB^{p}(X)$ into itself as above. We call $S$ a generalized rational graph $\phi$- contraction whenever the following conditions hold:

(i) $e_S$ is an edge that links $S(\chi_1)$ to $S(\chi_2)$ whenever $e$ is the preceding edge that links $\chi_1$ and $\chi_2$.

(ii) $W_S$ is a path from $S(\chi_1)$ to $S(\chi_2)$ whenever $W$ is a path from $\chi_1$ to $\chi_2$.

(iii) There exists a function $\phi: \mathbb{R}^{+} \rightarrow \mathbb{R}^{+}$ such that $\phi$ is upper semicontinuous, monotonic and non-decreasing, and that $\phi(t) < t$ for every $t > 0$, with $\sum \limits_{r = 0}^{\infty }\phi ^{r}\left(t\right)$, is convergent; and, if $e$ is an edge from $\chi_1$ to $\chi_2$, we infer that

where

Definition 1.6. Let $T: CB^{p}(X) \rightarrow CB^{p}(X)$. A fixed point of $T$ is a set $\chi \in CB^{p}(X)$ whenever $T(\chi) = \chi$. Then, the mapping $T$ generates the set $F(T) = \{\chi \in CB^{p}(X): T(\chi) = \chi \}$, which denotes the collection of fixed points of $T$.

A subset $\mathcal{C}$ of $CB(X)$ is said to be complete if, for any set $\chi_1, \chi_2 \in \mathcal{C}$, there is an edge between $\chi_1$ and $\chi_2$.

We say that a graph $G$ has a property $(P^{*})$ if, for any converging sequence $\{X_{n}\}_{n\geq 1} \subset CB^{p}(X)$, that is, $\lim\limits_{n\rightarrow \infty} H_p(X_{n}, \chi) = H_p(\chi, \chi)$ for some $\chi$ in $CB^{p}(X)$, one has an edge between the two consecutive terms $X_{n}$ and $X_{n + 1}$; we can extract a subsequence $\{X_{n_{k}}\}_{k \in \mathbb{N}}$ from $\{X_{n}\}$, from which one deduces that there also exists an edge that connects $X_{n_{k}}$ and the limiting set $\chi$ to each other.

2.   Fixed point results of graph contractions

We obtain analogous fixed-point results for set-valued self-maps on $CB^{p}(X)$ based on the partial metric $p$, and with some conditions on graph contraction.

Theorem 2.1. Let $(X, p)$ be a complete partial metric space equipped with a digraph $G$ having both vertex and edge sets satisfying $V(G) = X$ and $\Delta \subseteq E(G)$, respectively. We assume that the map $T: CB^{p}(X) \rightarrow CB^{p}(X)$ is a generalized graph $\phi$-contraction. Then,

(i) the partial Hausdorff weight associated with $U, V \in F(T)$ is zero whenever the non-empty set $F(T)$ is complete;

(ii) if $F(T) \neq \emptyset$, then $X_{T} \neq \emptyset$. Furthermore, for any $U \in F(T)$, one has $H_{p}(U, U) = 0$;

(iii) assume that $\tilde{G}$ has the property $(P^{*})$ and that $X_{T} \neq \emptyset$. Then, the map $T$ has a fixed point;

(iv) $F(T)$ is a complete set if and only if $F(T)$ is reduced to a singleton set.

Proof. $(i)$ Let $U, V \in F(T)$ and $F(T)$ be complete; then, there is an edge between $U$ and $V$, and a partial Hausdorff weight can be assigned to $U$ and $V$. Now, suppose, by way of contradiction, that $H_{p}(U, V) \neq 0$. Since the map $T$ is a graph $\phi$-contraction map, we easily infer that

where

Note that $T(U) = U$ and $T(V) = V$, we have $H_{p}(U, U) \leq H_{p}(U, V)$, $H_{p}(V, V) \leq H_{p}(U, V)$ and $H_{p}(U, U) + H_{p}(V, V) \leq 3H_{p}(U, V)$.

Therefore,

which is a contradiction. Hence, the result follows.

$(ii)$ Let $U \in F(T)$, which implies that $T(U) = U$. Now, since $\Delta \subseteq E(G)$, we have that $(u, u)$ is in $E(G)$ for all $u \in U$. Hence, $(U, U)$ is in $E(G)$, so $(U, T(U))$, where $U \in CB^{p}(X)$. Therefore, $X_{T} \neq \emptyset$.

Furthermore, note that $U$ is a fixed point of $T$; then, $H_{p}(U, U) = 0$. Assume otherwise, that is, $H_{p}(U, U) > 0$. Then, as $T$ is a generalized graph $\phi$-contraction, taking $\chi_1 = \chi_2 = U$ in Eq (1.1), we have

where

Therefore,

which is a contradiction. Hence, $H_{p}(U, U) = 0$.

$(iii)$ We consider $U \in X_{T} \neq \emptyset$. Then, since $U \in CB^{p}(X)$ and $\tilde{G}$ is weakly connected, it follows that $CB^{p}(X) \subseteq [U]_{\tilde{G}} = \mathcal{P}(X)$, where $\mathcal{P}(X)$ is the non-empty power set on $X$. Since $T$ is a self-map and the equivalence class satisfies the transitive property on $CB^{p}(X)$, we have $T(U) \in [U]_{\tilde{G}}$.

As such, by an argument, we have $T(U_{i}) \in [U]_{\tilde{G}}$ for each $U_{i} \in [U]_{\tilde{G}}$. Since $U \in X_{T}$, there is an edge between $U$ and $T(U)$. It follows that, since $T$ is a graph $\phi$-contraction, we have $\big(T^{n}(U), T^{n+1}(U)\big) \in E(\tilde{G})$ for all $n \in \mathbb{N}$.

We now define a recursive iterative sequence, as follows:

We assume that $U_{n+1} \neq U_n$ for all $n \in \{0, 1, 2, ...\}$. In the case that $U_{k+1} = U_k$ for some $k$, then $T(U_k) = U_{k+1} = U_{k}$, that is, $U_k$ is the fixed point of $T$. Since $\tilde{G}$ is weakly connected, there exists a sequence $\{x_{i}\}_{i = 1}^{n}$ for $x_0 = x$ and $x_{n} = y$ and $(x_{i-1}, x_{i}) \in E(\tilde{G})$ for $i = 1, 2, \cdots, n$ such that $x_{i} \in U_{i}$ for $i = 1, 2, \cdots, n$. Owing to the graph $\phi$-contraction $T$, we infer that

where

that is

Now, if $M_{p}(U_{n-1}, U_{n}) = H_{p}(U_{n}, U_{n+1})$, then clearly we have a contradiction, since

Therefore, the only value $M_{p}(U_{n-1}, U_{n})$ can yield is $H_{p}(U_{n-1}, U_{n})$. It now follows that

Now, for $m, n \in \mathbb{N}$ with $m > n$,

On taking the upper limit as $n, m \rightarrow \infty$, this shows that $\{T^{m}(U)\}$ is Cauchy; also, since, by assumption, $(X, p)$ is a complete partial metric space, one finds a set $U^{*}$ in $CB^{p}(X)$ such that $\lim\limits_{m\rightarrow \infty} H_p(T^{m}(U), U^{*}) = H_p(U^{*}, U^{*})$.

Now bringing all of the above results together, it follows that we have $\{T^{n}(U)\}$ such that $\lim\limits_{m\rightarrow \infty} H_p(T^{m}(U), U^{*}) = H_p(U^{*}, U^{*})$ and we have $(T^{n}(U), T^{n+1}(U)) \in E(\tilde{G})$ for all $n \in \mathbb{N}$.

First, we are going to show that $H_{p}(U^{*}, U^{*}) = 0$. Suppose, by way of contradiction, that this is not true. Then, since $T$ is a generalized graph $\phi$-contraction, for $(U_{n-1}, U_{n}) \subseteq E(G)$, we have

where

By taking limits on both sides of the above equation, we get: $\lim \limits_{n\rightarrow \infty} M_{p}(U_{n-1}, U_{n}) = H_{p}(U^{*}, U^{*})$. Thus taking upper limit on both sides of inequality (2.3), we obtain

which is a contradiction. This obviously yields $H_{p}(U^{*}, U^{*}) = 0$.

By virtue of the property $P^{*}$, we can extract the subsequence $\{T^{n_k}(U)\}_{k \geq 1}$ that provides us with an edge connecting $T^{n_k}(U)$ and $U^{*}$ for every $k \in \mathbb{N}$. It follows, the triangle inequality $(H4)$ and property $(iii)$ of the definition of a generalized graph $\phi$-contraction, as considered in Definition 1.4, that

where

Now since $T^{n_k}(U)$ is closed and the second term on the left-hand side of the above inequality reduces to $p(v, v)$, thus

We know that $\lim\limits_{k\rightarrow \infty} M_{p}(U^{*}, T^{n_{k}-1}(U)) = H_{p}(U^{*}, T(U^{*}))$ since any subsequence of a convergent sequence obviously converges to the same limit due to the uniqueness of limits. Hence, $\lim\limits_{k\rightarrow \infty} H_p(T^{n_k}(U), U^{*}) = H_p(U^{*}, U^{*})$.

Therefore, from the preceding inequality we get

which gives us a contradiction. Hence, $U^{*} \in F(T)$.

$(iv)$ Let $U, V \in F(T)$ and $F(T)$ be complete; then, by Item $(ii)$, we have that the Pompeiu-Hausdorff weight associated with $U$ and $V$ vanishes, which implies the equality $U = V$. Therefore, $|F(T)| = 1$. Also, any singleton is closed and bounded.

Proving the sufficiency, let $F(T)$ be a singleton; then, $\big(U, T(U)\big) = (U, U) \in E(\tilde{G})$; hence, $F(T)$ is clearly complete.

Example 2.2. Let $X = \{0, 1, 4\} = V(G)$ and $p:\mathfrak{X}\rightarrow \mathbb{R^{+}}$ be defined below:

where, $p(1, 1) = \frac{1}{2},$ $p(4, 4) = 2$ and $p(0, 0) = 0$. Also,

Indeed, $p$ as defined above, is a partial distance that equips $X$.

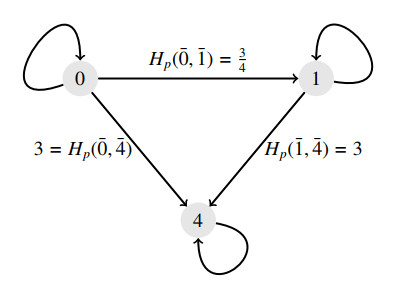
The $K_{3}$ graph with the defined edge and vertex sets above is shown in Figure 1 with the Pompeiu-Hausdorff weights.

Furthermore, note that the sets $\{0\}, \{0, 1\}$ and $\{0, 4\}$ are bounded in $X$. In particular, they are closed sets in $X$. Sets $\{0\}$ and $\{0, 1\}$ are shown as closed in Aydi et al. ^[9]. We show that $\{0, 4\}$ is indeed closed. We have

from which we deduce that the set $\{0, 4\}$ is, in fact, closed. Here, the closedness is understood in the sense of the partial metric $p$.

Now, for ease of readability, we define the following notation: $\{0\} = \bar{0}, \{0, 1\} = \bar{1}$ and $\{0, 4\} = \bar{4}$, where $CB^{p}(X) = \{ \bar{0}, \bar{1}, \bar{4} \}$. Employing the definition of the Pompeiu-Hausdorff metric and applying it to the elements of $CB^{p}(X)$, we get the following measure between the elements of $CB^{p}(X)$:

Define the map $T: CB^{p}(X) \rightarrow CB^{p}(X)$, as follows:

Notice that, between any two elements $\chi_1$ and $\chi_2$ of $CB^{p}(X)$, there is an edge (path) between them. Furthermore, there is an edge (path) between $T(\chi_1)$ and $T(\chi_2)$.

Define $\phi :[0, \infty)\rightarrow \lbrack 0, \infty)$ by

An easy computation is sufficient to prove that the map $\phi$ is actually continuous on $[0, \infty)$, satisfying the bound $\phi (t) < t$ for every $t > 0$.

Now, for all $\chi_1, \chi_2\in CB^{p}\left(X\right)$, we consider the occurring cases:

$\bf (a)$ For $\chi_1, \chi_2\in \{ \overline{0}, \bar{1}\}$, we have $H_{p}(T\left(\chi_1\right), T\left(\chi_2\right)) = H_{p}\left(\bar{0}, \bar{0}\right) = 0.$

$\bf (b)$ If $\chi_1\in \{ \overline{0}, \bar{1}\} \ $and $\chi_2 = \bar{4},$ then we have

$\bf (c)$ If $\chi_1 = \chi_2 = \bar{4},$ then we have

Clearly, the inequality (1.1) is valid for all of the above three cases, (a)–(c). We henceforth deduce that, for any $\chi_1, \chi_2\in CB^{p}\left(X\right)$, one has an edge linking $\chi_1$ and $\chi_2$. Since (1.1) holds true, we deduce that $T$ is a generalized graph $\phi$-contraction. Thus far, the four conditions of the main theorem, Theorem 2.1, hold true. Moreover, $T(\{0\}) = \{ 0\}$, making the singleton $\{0\}$ the fixed point for $T$ from which we infer that $F\left(T\right)$ is reduced to the unit set $\{0\}$. Equivalently, the set $F(T)$ is a complete set.

The next example shows that, although it holds in a partial metric space, it does not carry over to a metric space where the metric $p^{S}$ is induced from $p$.

Example 2.3. We set $X : = \{0, 1, 2\} = V(G)$ to be equipped with a partial metric $p:X \times X \mathfrak \rightarrow \mathbb{R^{+}}$ that is defined as follows:

Define $E(G) = \{(0, 0), (1, 1), (2, 2), (0, 1), (0, 2), (1, 2)\}$. Furthermore, the sets $\{0\}$ and $\{0, 1\}$ are mentioned as closed in ^[9]. However, we demonstrate that $\{0\}, \{0, 1\}$ and $\{0, 2\}$ are indeed closed.

Hence, $\{0\}$ is a closed set again w.r.t. the partial distance $p$. In the same fashion, we have

Hence, we hereby confirmed that the set $\{0, 1\}$ is also a closed set w.r.t. $p$. Finally,

Hence, $\{0, 2\}$ is also closed. Clearly, the above sets are also bounded. As a result, we have $CB^{p}(X) = \{\bar{0}, \bar{1}, \bar{2} \}$, where $\bar{0} = \{0\}, \bar{1} = \{0, 1\}$ and $\bar{2} = \{0, 2\}$. We employ the Pompeiu-Hausdorff metric and apply it to the elements of $CB^{p}(X)$, as follows:

Define $T : CB^{p}(X) \rightarrow CB^{p}(X)$, as follows:

Notice that, between any two elements $\chi_1$ and $\chi_2$ of $CB^{p}(X)$, there is an edge (path) between them. Furthermore, there is an edge resp. (path) connecting $T(\chi_1)$ and $T(\chi_2)$. We consider a function $\phi : [0, \infty) \rightarrow [0, \infty)$ as defined in Example 2.2.

Now, for all $\chi_1, \chi_2 \in CB^{p}(X)$, we look into the following cases:

1) $H_{p}(T(\chi_1), T(\chi_2)) = H_{p}(\bar{0}, \bar{0}) = 0$ whenever $\chi_1, \chi_2 \in \{\bar{0}, \bar{1}\}$.

2) If $\chi_1 \in \{\bar{0}, \bar{1}\}$ and $\chi_2 = \bar{2}$, then it follows that, in the ase $\chi_1 = \bar{0} \ and \ \chi_2 = \bar{2}$, then

And, when $\chi_1 = \bar{1} \ and \ \chi_2 = \bar{2}$, we have

3) If $\chi_1 = \chi_2 = \bar{2}$, then we have

Clearly, (1.1) is satisfied in the above enumerated cases. Hence, for all $\chi_1, \chi_2 \in CB^{p}(X)$, there is an edge between $\chi_1$ and $\chi_2$, condition (1.1) is satisfied and $T$ is a generalized graph $\phi$-contraction. Thus, all conditions of Theorem 2.1 hold true. Furthermore, $T(\bar{0}) = \bar{0}$, making $\bar{0}$ the fixed point of $T$ from which we infer that the set $F(T)$ is complete.

Now, $p^{S}$ is the metric induced by the partial metric $p$, as defined below:

Notice that the pair $(X, p^{S})$ is a metric space. From the above, we have the following:

We now demonstrate that Theorem 2.1 in ^[1] cannot be applicable for $\chi_1 = \bar{0}$ and $\chi_2 = \bar{2}$; we then compute the following:

Let us denote by $\Upsilon$ the set of functions

for any compact set $I \subset \mathbb{R^{+}}$. Consequently, applying the result in Theorem 2.1, we derive the result below concerning the existence of a fixed point for a mapping with the contractive conditions of integral type.

Corollary 2.4. Let $(X, p)$ be a complete partial metric space equipped with a graph $G$ with the vertex set $V(G) = X$ and the edge set $E(G)\supseteq \Delta$. We assume that $T: CB^{p}(X) \rightarrow CB^{p}(X)$ is a mapping such that for all $\chi_1, \chi_2 \in CB^{p}(X)$, the conditions below hold true.

(A1) If $e$ is an edge linking $\chi_1$ and $\chi_2$, we infer that $e_T$ is the edge connecting $T(\chi_1)$ and $T(\chi_2)$.

(A2) A path $W$ from $\chi_1$ to $\chi_2$ implies that $W_T$ is also a path connecting $T(\chi_1)$ to $T(\chi_2)$.

(A3) There exists a function $\phi: \mathbb{R}^{+} \rightarrow \mathbb{R}^{+}$ such that $\phi$ is upper-semicontinuous, monotonic and non-decreasing, and that $\phi(t) < t$ for every $t > 0$, with $\sum \limits_{r = 0}^{\infty }\phi ^{r}\left(t\right)$, is convergent; and, if $e$ is an edge from $\chi_1$ to $\chi_2$, we infer that

where

Then, the statements below are valid.

(i) If $F(T) \neq \emptyset$ is complete, then the partial Hausdorff weight assigned to the $U, V \in F(T)$ is zero.

(ii) If $F(T) \neq \emptyset$, then $X_{T} \neq \emptyset$. Furthermore, for any $U \in F(T)$, one has $H_{p}(U, U) = 0$.

(iii) If $X_{T}$ is not empty and $(P^{*})$ holds true for the weakly connected graph $\tilde{G}$, then the mapping $T$ has a fixed point.

(iv) $F(T)$ is a complete set if and only if the set $F(T)$ is reduced to a singleton.

Proof. Define $\Psi :[0, \infty)\rightarrow \lbrack 0, \infty)$ by $\Psi (x) = \int_{0}^{x}\varphi (t)dt$; then, from (2.4), we have

which can be expressed in the form

where $\phi ^{*} = \Psi \circ \phi$. Clearly, the function $\phi^{*} : \mathbb{R}^{+} \rightarrow \mathbb{R}^{+}$ is upper-semicontinuous and non-decreasing with $\phi ^{*} (t) < t$ for every $t > 0$. Hence, by Theorem 2.1, the result follows.

Corollary 2.5. Let $(X, p)$ be as in Corollary 2.4. We assume that $T: CB^{p}(X) \rightarrow CB^{p}(X)$ is a mapping such that, for all $\chi_1, \chi_2 \in CB^{p}(X)$, the conditions below hold true.

(1) If $e$ is an edge linking $\chi_1$ and $\chi_2$, we infer that $e_T$ is the edge connecting $T(\chi_1)$ and $T(\chi_2)$.

(2) A path $W$ from $\chi_1$ to $\chi_2$ implies that $W_T$ is also a path connecting $T(\chi_1)$ to $T(\chi_2)$.

(3) There exists a constant $0\leq \kappa < 1$ such that, if $e$ is an edge from $\chi_1$ to $\chi_2$, we infer that

where

Then, the statements below are valid.

(i) If $F(T) \neq \emptyset$ is complete, then the partial Hausdorff weight assigned to the $U, V \in F(T)$ is zero.

(ii) If $F(T) \neq \emptyset$, then $X_{T} \neq \emptyset$. Furthermore, for any $U \in F(T)$, one has $H_{p}(U, U) = 0$.

(iii) If $X_{T}$ is not empty and $(P^{*})$ holds true for the weakly connected graph $\tilde{G}$, then the mapping $T$ has a fixed point.

(iv) $F(T)$ is a complete set if and only if the set $F(T)$ is reduced to a singleton.

Proof. By taking $\phi(t) = \kappa t$ in Theorem 2.1, the result follows.

Remark 2.6. Let $\mathcal{S}^{p}(X)$ denote the collection of all singleton subsets of the given space $X$. Then clearly, $\mathcal{S}^{p}(X)\subseteq \mathcal{CB}^{p}(X)$. In this case, the operator $T$ becomes a self-mapping on $X$.

Consequently, the following fixed-point result is obtained.

Corollary 2.7. Let $(X, p)$ be as in Corollary 2.4. Assume that $T: S^{p}(X) \rightarrow S^{p}(X)$ is a mapping such that, for all $\chi_1, \chi_2 \in S^{p}(X)$, the conditions below hold true.

(1) $e_T$ is an edge that links $T(\chi_1)$ to $T(\chi_2)$ whenever $e$ is the preceding edge that links $\chi_1$ and $\chi_2$.

(2) $W_T$ is a path from $T(\chi_1)$ to $T(\chi_2)$ whenever $W$ is a path from $\chi_1$ to $\chi_2$.

(3) There exists a function $\phi: \mathbb{R}^{+} \rightarrow \mathbb{R}^{+}$ such that $\phi$ is upper-semicontinuous, monotonic and non-decreasing, and that $\phi(t) < t$ for every $t > 0$, with $\sum \limits_{r = 0}^{\infty }\phi ^{r}\left(t\right)$, is convergent; also, if $e$ is an edge from $\chi_1$ to $\chi_2$, we infer that

where

Then, the statements below are valid.

(i) If $F(T) \neq \emptyset$ is complete, then the partial Hausdorff weight assigned to the $U, V \in F(T)$ is zero.

(ii) If $F(T) \neq \emptyset$, then $X_{T} \neq \emptyset$. Furthermore, for any $U \in F(T)$, one has $H_{p}(U, U) = 0$.

(iii) If $X_{T}$ is not empty and $(P^{*})$ holds true for the weakly connected graph $\tilde{G}$, then the mapping $T$ has a fixed point.

(iv) $F(T)$ is a complete set if and only if the set $F(T)$ is reduced to a singleton.

Theorem 2.8. Let $(X, p)$ be a complete partial metric space equipped with a digraph $G$ having both vertex and edge sets satisfying $V(G) = X$ and $\Delta \subseteq E(G)$, respectively. We assume that the map $S: CB^{p}(X) \rightarrow CB^{p}(X)$ is a generalized graph $\phi$-contraction. Then, it holds that

(I) the partial Hausdorff weight associated with $U, V \in F(S)$ is zero whenever the non-empty set $F(S)$ is complete;

(II) if $F(S) \neq \emptyset$, then $X_{S} \neq \emptyset$. Furthermore, for any $U \in F(S)$, one has $H_{p}(U, U) = 0$;

(III) assume that $\tilde{G}$ has the property $(P^{*})$ and that $X_{S} \neq \emptyset$. Then, the map $S$ has a fixed point;

(IV) $F(S)$ is a complete set if and only if $F(S)$ is reduced to a singleton set.

Proof. (I) Let $U, V \in F(S)$ and $F(S)$ be complete; then, there is an edge between $U$ and $V$. Suppose, by way of contradiction, that $H_{p}(U, V) \neq 0$. It follows that, since $S$ is a graph rational $\phi$-contraction, we have

where

Now, from inequality (2.9) and Eq (2.10), it follows that

which is a contraction. Hence, our result follows.

(II) Let $U \in F(S)$; then, $S(U) = U$, a similar argument to Theorem 2.4, shows that $X_{S} \neq \emptyset$.

Furthermore, if $S(U) = U$, then $H_{p}(U, U) = 0$. Suppose otherwise, that is, $H_{p}(U, U) > 0$. Then, as $S$ is a generalized rational graph $\phi$-contraction, and by taking $\chi_1 = \chi_2 = U$ in Eq (1.2), we have

where

It follows that

which is obviously a contradiction.

In order to show that the result in Item (III) holds true; it is sufficient to prove that $U^{*} \in F(S)$. For this purpose, let $U \in X_{S} \neq \emptyset$. Then, since $U \in CB^{p}(X)$ and $\tilde{G}$ is weakly connected, it follows that $CB^{p}(X) \subseteq [U]_{\tilde{G}} = \mathcal{P}(X)$, where $\mathcal{P}(X)$ is the non-empty power set on $X$. Since $S$ is a self-map and the equivalence class satisfies the transitive property on $CB^{p}(X)$, we have $S(U) \in [U]_{\tilde{G}}$.

As such by a similar argument, we have $S(U_{i}) \in [U]_{\tilde{G}}$ for each $S_{i} \in [U]_{\tilde{G}}$. Since $U \in X_{S}$, there is an edge between $U$ and $S(U)$. It follows that, since $S$ is a generalized rational graph $\phi$-contraction, we have $\big(S^{n}(U), S^{n+1}(U)\big) \in E(\tilde{G})$ for all $n \in \mathbb{N}$.

We now define a recursive iterative sequence, as follows:

Since $\tilde{G}$ is weakly connected, then there exists a sequence $\{x_{i}\}_{i = 1}^{n}$ for $x_0 = x$ and $x_{n} = y$ and $(x_{i-1}, x_{i}) \in E(\tilde{G})$ for $i = 1, 2, \cdots, n$ such that $x_{i} \in U_{i}$ for $i = 1, 2, \cdots, n$. It follows that, since $S$ is a generalized rational graph $\phi$-contraction, we have

where

That is,

Now, if $\max \big\{H_{p}(U_{n+1}, U_{n}), H_{p}(U_{n-1}, U_{n})\big\} = H_{p}(U_{n+1}, U_{n})$, then, from Eq (2.13), we have

which is a contradiction.

Therefore,

and it follows that

Now, for $m, n \in \mathbb{N}$ with $m > n$,

On taking the upper limit as $n, m \rightarrow \infty$, this shows that $\{S^{m}(U)\}$ is Cauchy; also since, by assumption, $(X, p)$ is a complete partial metric space, we deduce that we will find some set $U^{*}$ in $CB^{p}(X)$ such that $\lim\limits_{m\rightarrow \infty} H_p(S^{m}(U), U^{*}) = H_p(U^{*}, U^{*})$.

Now bringing all of the above results together, it follows that $\lim\limits_{m\rightarrow \infty} H_p(S^{m}(U), U^{*}) = H_p(U^{*}, U^{*})$, and we have $(S^{n}(U), S^{n+1}(U)) \in E(\tilde{G})$ for all $n \in \mathbb{N}$. First, we are going to show that $H_{p}(U^{*}, U^{*}) = 0$. Suppose, by way of contradiction, that this is not true. Then, since $S$ is a generalized rational graph $\phi$-contraction, for $(U_{n-1}, U_{n}) \subseteq E(G)$, we have

where

By taking the limits on both sides of the above equation, we get $\lim \limits_{n\rightarrow \infty} N_{p}(U_{n-1}, U_{n}) = H_{p}(U^{*}, U^{*})$. Thus, taking the limits on both sides of Inequality (2.13), we get

which is a contradictory result. Hence, $H_{p}(U^{*}, U^{*}) = 0$.

Now, by virtue of the property $(P^{*})$, we can extract a subsequence $\{S^{n_k}(U)\}$ such that there is an edge between $S^{n_k}(U)$ and $U^{*}$ for each $k \in \mathbb{N}$. It follows from the triangle inequality $(H4)$ and property $(iii)$ of the definition of the generalized rational graph $\phi$-contractions that we have the following inequalities:

where

Taking the limits on both sides, we obtain

Now since $S^{n_k}(U)$ is closed, the second term on the left-hand side of the above inequality reduces to $p(v, v)$. Thus,

We know, from Eq (2.15) above that $\lim\limits_{n_{k} \rightarrow \infty} N_{p}(U^{*}, S^{n_{k}-1}(U)) = H_{p}(U^{*}, S(U^{*}))$, since a subsequence of a convergent sequence converges to the same limit due to the uniqueness of limits. Hence, $\lim\limits_{k\rightarrow \infty} H_p(S^{n_k}(U), U^{*}) = H_p(U^{*}, U^{*})$. Therefore, from the preceding inequality, we get

which gives us a contradiction. Hence, $U^{*} \in F(S)$.

(IV) It is enough to show that the set $F(S)$ can be reduced to a unit set. We consider $U, V \in F(S)$ with $F(S)$ as complete; then, by (II), the partial Hausdorff weight associated with $U$ and $V$ is zero, which implies $U = V$. Therefore, $|F(S)| = 1$. Also, any singleton is closed and bounded. Proving the sufficiency, let $F(S)$ be a singleton; then, $\big(U, S(U)\big) = (U, U) \in E(\tilde{G})$, and it is clearly complete.

Corollary 2.9. Let $(X, p)$ be as in Corollary 2.4. We assume that $S: CB^{p}(X) \rightarrow CB^{p}(X)$ is a mapping such that, for all $\chi_1, \chi_2 \in CB^{p}(X)$, the conditions below hold true.

(i) $e_S$ is an edge connecting $S(\chi_1)$ and $S(\chi_2)$ whenever $e$ is an edge connecting $\chi_1$ and $\chi_2$.

(ii) From a path $W$ from $\chi_1$ to $\chi_2$, one can infer a path $W_S$ from $S(\chi_1)$ to $S(\chi_2)$.

(iii) There exists a function $\phi: \mathbb{R}^{+} \rightarrow \mathbb{R}^{+}$ such that $\phi$ is upper semicontinuous, monotonic and non-decreasing, and that $\phi(t) < t$ for every $t > 0$, with $\sum \limits_{r = 0}^{\infty }\phi ^{r}\left(t\right)$, is convergent; also, if $e$ is an edge from $\chi_1$ to $\chi_2$, we infer that

where

Then it holds that:

(1) If $F(S) \neq \emptyset$ is complete, then the partial Hausdorff weight assigned to the $U, V \in F(S)$ is zero.

(2) If $F(S) \neq \emptyset$, then $X_{S} \neq \emptyset$. Furthermore, for any $U \in F(S)$, one has $H_{p}(U, U) = 0$.

(3) If $X_{S} \neq \emptyset$ and $\tilde{G}$ is a weakly connected graph having the property $(P^{*})$, then $S$ has a fixed point.

(4) $F(S)$ is complete if and only if $F(S)$ is reduced to a singleton.

3.   Well-posedness result in partial metric spaces

Now, we will define the well-posedness of fixed-point-based problems of generalized graph contractive operators in the framework of partial metric spaces.

Definition 3.1. For a complete partial metric space $(X, p)$, we say that a fixed-point-based problem of mapping $T: CB^{p}(X) \rightarrow CB^{p}(X)$ is called well-posed if $T$ has a unique fixed point $\chi^* \in CB^{p}(X)$, and for any sequence $\{\chi_n\}$ in $CB^{p}(X)$, $\lim\limits_{n \rightarrow \infty}H_p (T(\chi_n), \chi_n) = H_p (\chi^*, \chi^*)$ implies that $\lim\limits_{n \rightarrow \infty} H_p (\chi_n, \chi^*) = H_p (\chi^*, \chi^*)$.

Theorem 3.2. Given a complete partial metric space $(X, p)$ and an operator mapping $T:CB^{p}(X) \rightarrow CB^{p}(X)$, as defined in Corollary 2.5, then the fixed-point-based problem of $T$ is well-posed.

Proof. From Corollary 2.5, we infer that the map $T$ has a unique fixed point, say $\chi^{*}$. Let $\chi_{n}$ be a sequence in $CB^{p}(X)$ such that $\lim\limits_{n \rightarrow \infty} H_{p}(T(\chi_{n}), \chi_{n}) = H_{p}(\chi^{*}, \chi^{*})$. We want to show that $\lim\limits_{n \rightarrow \infty} \chi_{n} = \chi^{*}$. From (2.7), we then have

where

We now consider the following cases:

Case 1: If $M_{p}(\chi_{n}, \chi^{*}) = H_{p}(\chi_{n}, \chi^{*})$, then, by Eq (3.1) above, we have

that is,

Now, taking the limits on both sides of the above inequality implies $\lim\limits_{n \rightarrow \infty} H_{p}(\chi_{n}, \chi^{*}) = 0$, that is, $\lim\limits_{n \rightarrow \infty} \chi_{n} = \chi^{*}$.

Case 2: If $M_{p}(\chi_{n}, \chi^{*}) = H_{p}(\chi_{n}, T(\chi_{n}))$, then, by Eq (3.1) above, we have

Again, by taking the limits on both sides, we have

Hence, $\lim\limits_{n \rightarrow \infty} H_{p}(\chi_{n}, \chi^{*}) = 0,$ that is, $\lim\limits_{n \rightarrow \infty} \chi_{n} = \chi^{*}$.

Case 3: If $M_{p}(\chi_{n}, \chi^{*}) = H_{p}(\chi^{*}, T(\chi^{*}))$, then, by Eq (3.1) above, we have

By limiting, we get $\lim\limits_{n \rightarrow \infty} H_{p}(\chi_{n}, \chi^{*}) = 0$, that is, $\lim\limits_{n \rightarrow \infty} \chi_{n} = \chi^{*}.$

Case 4: If $M_{p}(\chi_{n}, \chi^{*}) = \dfrac{H_{p}(\chi_{n}, T(\chi^{*})) + H_{p}(\chi^{*}, T(\chi_{n}))}{3}$, then, by Eq (3.1) above, we have

that is,

By taking the limit, we get $\lim\limits_{n \rightarrow \infty} H_{p}(\chi_{n}, \chi^{*}) = 0,$ that is, $\lim\limits_{n \rightarrow \infty} \chi_{n} = \chi^{*}$.

This completes the proof.

4.   Application

We are applying our obtained results to obtain the solution of a functional equation arising in the dynamic programming.

Let $B_{1}$ and $B_{2}$ be two Banach spaces with $U\subseteq B_{1}$ and $V\subseteq B_{2}$. Suppose that

If we consider $U$ and $V$ as the state and decision spaces, respectively, then the problem of dynamic programming reduces to the problem of solving the following functional equation:

Equation (4.1) can be reformulated as

where $b > 0$.

We study the existence and uniqueness of the bounded solution of the functional equation (4.2) arising in dynamic programming in the setup of the partial metric spaces.

Let $B(U)$ denotes the set of all bounded real-valued functions on $U$. For an arbitrary $\eta \in B(U)$, define $\left \Vert \eta \right \Vert = \sup \limits_{t\in U}\left \vert \eta (t)\right \vert$. Then, $(B(U), \left \Vert \cdot \right \Vert)$ is a Banach space. Now, consider

where $\eta, \xi \in B(U)$. Then, $p_{_{B}}$ is a partial metric on $B(U)$ (see also ^[3]).

Consider the graph $G$ with a partial order relation by

Then, $\left(B(U), p_{_{B}}\right)$ is a complete partial metric space with a directed graph $G,$, where

Assume that:

(C$_{1}$) $f,$ $\sigma _{1}$ and $\sigma _{2}$ are bounded and continuous.

(C$_{2}$) For $x\in U$, $\eta \in B(U)$ and $b > 0$, take $T:B(U)\rightarrow B(U)$ as

Moreover, for every $(x, y)\in U\times V$, $\left(\eta, \xi \right) \in E(G)$ and $t\in U$ implies

where a function $\phi : \mathbb{R} ^{+}\rightarrow \mathbb{R} ^{+}$ such that $\phi$ is upper semicontinuous, monotonic and non-decreasing, and that $\phi (t) < t$ for every $t > 0$, with $\sum_{r = 0}^{\infty }\phi ^{r}\left(t\right)$, is convergent; also

(C$_{3}$) For any converging sequence $\left \{ \eta _{n}\right \} \ $of $B(U)$, that is, $\lim \limits_{n\rightarrow \infty }p_{B}(\eta _{n}, \eta ^{\ast }) = p_{B}(\eta ^{\ast }, \eta ^{\ast })$ for some $\eta ^{\ast }$ in $B(U)$, with $(\eta _{n}, \eta _{n+1})\in E\left(G\right)$ for $n\in \mathbb{N},$ there exists a subsequence $\{ \eta _{n_{k}}\} \ $of $\{ \eta _{n}\}$ that satisfies $\left(\eta _{n_{k}}, \eta ^{\ast }\right) \in E\left(G\right)$.

Theorem 4.1. Assume that the conditions (C$_{1}$)–(C$_{3}$) hold. Then, the functional equation (4.2) has a unique bounded solution in $B(U)$.

Proof. Note that $(B(U), p_{B})$ is a complete partial metric space. By (C$_{1}$), $T\ $is a self-mapping of $B(U)$. By (4.3) in (C$_{2}$), it follows that for any $\left(\eta, \xi \right) \in E\left(G\right)$ and $b > 0$, choose $x\in U$ and $y_{1}, y_{2}\in V$ such that

which further implies that

From (4.5) and (4.8), and together with (4.4), we can obtain

From (4.6) and (4.7), and together with (4.4), we can obtain

From (4.10), we get

From (4.11), we obtain that

where

Therefore, all conditions of Corollary 2.7 hold. Thus, there exists a fixed point of $T$, that is, $\eta ^{\ast }\in B(U)$, where $\eta ^{\ast }\left(t\right)$ is a solution of the functional equation (4.2).

5.   Conclusions

In this paper, we proved the existence of fixed-points for various different generalized contractive mappings in partial metric spaces endowed with a graph structure. Moreover, we were able to present some non-trivial examples to illustrate the main result and an application regarding the existence and uniqueness of the bounded solution of the functional equation arising in dynamic programming in the setup of partial metric spaces. Furthermore, we presented the well-posedness of fixed-point-based problems of generalized graph contractive operators in the framework of partial metric spaces.

Acknowledgments

The authors are very grateful to the Basque Government for Grant IT1207-19. Moreover, the authors are thankful to anonymous reviewers and academic editor for their very useful comments, which undoubtedly has helped us to improve the overall presentation of the paper.

Conflict of interest

The authors declare that they do not have any conflicts of interest regarding this paper.