1. Introduction
Executive functions cover a wide range of complex cognitive processes responsible for the coordination of neural activity in order to produce goal-oriented behaviors in a consistent manner over time [1]. Recently, working memory, cognitive flexibility and inhibitory control were regarded as central executive processes [2], which together enable individuals to plan and monitor their actions, as well as to evaluate the consequences of these, make decisions, solve problems, resist to interference, sustain attention over a task, deal with novelty, anticipate the consequences of the actions of others and regulate their behavior according to what is socially accepted [2,3,4,5].
The concept of cognitive control relates to the dichotomy between automatic and controlled processing. Therefore executive functions involve shifting the focus towards controlled processes that are influenced by individuals' goals and compete with automatic and more usual processes [1]. Most times, these activities also comprise social rules and/or emotional states, resulting in the so-called emotional/motivational executive functions.
Inhibitory control can be considered as an emotional/motivational executive function, essential for successfully living in society [6], responsible for inhibiting inappropriate behaviors, thoughts or emotions in response to certain stimulus [7]. It allows the interference control through the inhibition at the level of attention, which requires selectively attending to a given attribute of a stimulus while ignoring other [2], e.g., attending to the color of a word and not to its meaning, as in the case of the Stroop task. This may also lead to the inhibitory control at the level of behavior [2], which allows reporting the color of the word instead of reading it. Inhibitory control is frequently assessed by the Stroop task [8].
In its classical version, the test is divided into two stages. In both stages, words that represent color names are presented to the subject. In the first stage, the word and the color of the word match (the word "blue" is written in blue, for example). In the second stage, an interference factor is added, since these attributes may or may not match ("blue" written in red, for example). Participants are instructed to report the color in which the words are written, inhibiting the automatic response to read them. Stroop interference effect is defined as the resultant increase in the number of errors and in the amount of time necessary to respond to incongruent stimuli, supposedly due to the conflict between the two attributes of the word (its meaning and its color) in this condition [9].
Currently there are variations to this version, such as the protocol followed in this study, which includes a stage with words that are phonetically similar to color names. Other studies also included stages composed of words that did not represent colors and found that those stages are generally easier than incongruent stages but more difficult than the congruent ones [10,11,12,13].
Considering the notorious relationship between brain and behavior, it is important to study not only behavioral performance on a test but also its neural and physiological correlates. Thayer, Hansen, Saus-Rose et al. [14] argued in favor of an integrated inhibitory neural network responsible for mediating executive functions, emotional regulation and heart rate (HR) through an integrated neurovisceral mechanism. The role of the prefrontal cortex both in the executive control and in the inhibition of the heart rate acceleration is essential for such integration. Thus, the aim of this study was to assess the electroencephalographic activity and heart rate variability (HRV) of young adults during the execution of the Stroop task. Such a broad study is especially important considering the hypothesis presented by Thayer, Hansen, Saus-Rose et al. [14] addressed above.
The electrophysiological activity was assessed using the electroencephalogram (EEG). This technique allows establishing correlations between the patterns of cortical activity and the behavior displayed by individuals, without attributing, however, a causal relationship between them. The brain oscillations obtained can be decomposed using the Fourier Fast Transformation into frequency oscillations, the most commonly studied being Theta, Alpha, Beta and Gamma. The dominance of each frequency of brain oscillations can be interpreted according to its associated physiological and psychological states.
These oscillations can be subdivided into two types of processing modes that act together in dynamic interactions: global modes which are composed of Delta, Theta and Alpha oscillations and span relatively large brain regions promoting its integration, and local modes distributed across more limited topographical areas and composed of Beta and Gamma oscillations [15].
A review of studies using the Stroop task and other executive tests indicates that they are associated to a complex brain system composed of circuits that connect several cortical and subcortical structures, including the prefrontal cortex [16] and its main subdivisions, such as the dorsolateral (DLPFC), ventrolateral (VLPFC), orbitofrontal (OFPFC), and ventromedial (VMPFC) prefrontal cortices, and the anterior cingulate cortex (ACC).
This is in line with a neural network model that helps explaining the brain dynamics during the Stroop task [17,18]. This model stated that mental representation of the color of the word is attributed to the occipital cortex while the superior temporal cortex is concerned with the representation of the word itself. The automatic process of reading the word usually overcomes the process of naming the color, generating a verbal response mediated by the motor cortex. However, individuals are able to give the correct response due both to a conflict monitor, supposedly mediated by the ACC, and to an attentional control system mediated by the DLPFC. The former monitors the conflict between two possible responses (the color and the word itself) which is solved by enhancing the attention using the latter system. Thus, the attentional control system takes into account the purpose of the task (naming the color of the word) and increases the contribution of the mental representation of the color for the generation of the verbal response. Other studies using EEG during the Stroop task also highlighted the involvement of the frontal lobe [10], left occipital lobe [19], temporal lobe [20], and ACC [21], especially during incongruent conditions.
Several studies also addressed the physiological aspects of the Stroop task by examining the subjects' heart rate variability (HRV). HRV measures are based on changes in the duration of RR intervals, i.e. duration of intervals between successive R (or N) peaks of typical heartbeat oscillations.
HRV analysis can be conducted considering time or frequency domains [22]. In the time domain, RR intervals can be considered individually for obtaining the standard deviation of all normal RR intervals (SDNN), or as adjacent intervals for the root mean square of the difference of successive RR intervals (rMSSD) and the percentage of differences between adjacent normal RR intervals that are greater than 50 milliseconds (pNN50). Additionally, the ratio of low (0.04-0.15 Hz) and high (0.15-0.4 Hz) frequencies (LF/HF ratio) can be calculated on the frequency domain.
HRV is modulated by the combined action of sympathetic and parasympathetic divisions of the Autonomic Nervous System (ANS) and therefore is responsive to stress. When an individual is exposed to acute stress, the sympathetic division of the ANS becomes more active increasing the LF/HF ratio and decreasing SDNN and rMSSD parameters [23]. SDNN, rMSSD as well as pNN50 reflect the parasympathetic activity in short term variations on the heart rate at each heartbeat [24], thus they decrease in mental stress situations.
According to Thayer, Hansen, Saus-Rose et al. [14], HRV can be considered as an index of the functional capacity of brain structures that support executive success. Such statement is based on their results in which higher vagally mediated HRV values are associated with better performance of individuals during executive tasks [14].
Some studies have assessed HRV during the execution of the Stroop task, but they favored the comparison of the parameters during this test and a baseline or between two groups of subjects and so did not emphasize the possible HRV parameter differences between the stages of the task [23,24,25,26,27,28].
We consider that this study is original and relevant as it investigates the possible interaction between nervous and cardiac systems of young adults while performing a version of the Stroop task that includes a stage with words phonetically similar to color names.
2. Materials and Methods
2.1. Subjects
The subjects were 50 young adults (24 women) with an age range from 17 to 28 years old and mean age of 21.6 (SD = 2.8). They were healthy undergraduate students recruited by written announcement at the University of Brasilia. All subjects were right-handed according to the Edinburgh Inventory [29], and reported no personal or family history of neurological or psychiatric disorders. The participants declared that they had not used drugs or drank alcoholic beverages within the 24 hours that preceded the research. All subjects gave informed consent to a protocol approved by the Ethics Committee of the Health Sciences Faculty, University of Brasilia, Brazil (CAAE 24418013.2.0000.0030).
The performance on the Stroop task was analyzed using the data from 22 participants (10 females) with an age range from 18 to 27 years old and mean age 21.6 (SD = 2.9). The behavioral assessment was methodologically designed as a manipulation check in order to assure that the version of the test worked properly and demanded executive functions.
2.2. Data acquisition and processing
The electrophysiological recordings were taken using the Neuron-Spectrum-4/EPM device (Neurosoft®, Ivanovo, Russia) with A/D conversion and sampling rate of 2000 Hz. For the electrocardiographic registration (EKG), a standard bandpass filter (0.5-75 Hz) and a common-mode rejection ratio of 100 mV were used. The device allows the simultaneous registration of EEG and EKG and other electrophysiological signals using 29 channels. 28 channels were used: 24 for the EEG registration, 1 for the EKG, 1 for the galvanic skin response (GSR) measure and 2 for the electromyography (EMG). The GSR and EMG data were not analyzed in this study.
EEG was recorded using the 10:20 International Electrode System [30] and reference electrodes positioned on the mastoids. Ag/AgCl individual electrodes were attached by a conductive paste (Ten20, Weaver and Company, Aurora, USA) on sites previously cleaned using an abrasive gel (Nuprep®, Weaver and Company, Aurora, USA) and the impedances were kept under 5 kΩ during the entire session. For electrocardiography, one self-adhesive electrode was allocated on the neck, above the right external jugular vein, and another on the cubital fossa over the radial artery [31].
Neuron-Spectrum-4/EPM were connected to a computer (portable, Satellite INTR®, AMD Athlon processor of 1, 1 GHz, 256 MB of RAM, and 15" screen) and used the Neuron-Spectrum software (Neurosoft®, Ivanovo, Russia), version 2.3.56.0, for the display of the registration in real-time. This computer was also used to run the cognitive tests, but a keyboard and a mouse were connected to it for the participant use.
EEG data were analyzed using the open source EEGLab toolbox, version 9.0.4.5 [32], considering the epochs corresponding to the test execution (64s intervals between the onset and end of each stage of the Stroop task). The data were resampled to 500 Hz and subjected to an Infomax algorithm in order to be decomposed into their independent components [33]. The components related to artifacts presented standard attributes and were then removed using the independent components analysis (ICA). Afterwards, EEG data were recalculated with the remaining components. A study was generated and was pre-computed for the calculation of the spectral power (in μV). Data were analyzed according to the standard frequency oscillations: Theta (4-8 Hz), Alpha (8-13 Hz), Beta (13-30 Hz) and Gamma (30-70 Hz). Topographical maps were generated for the stages of the Stroop task on the abovementioned frequency oscillation using the power registered on each electrode and the smoothing technique of spherical interpolation around the channels [31,34].
HR data corresponding to the task execution (64s intervals between the onset and end of each stage of the Stroop task) were digitally isolated using scripts developed in our laboratory in MatLab, v. 7.8.0 (R2009a) and were later processed using the EKG module of the software Protolize! [35] for the detection of R peaks and for the calculation of the heart rate variability (HRV) parameters in time (SDNN, rMSSD, pNN50) and frequency (LF/HF ratio, i.e. ratio of low (0.04 to 0.15 Hz) and high (0.18 to 0.4 Hz) frequency components) domains calculated according to international standard guidelines [36] and as in the study developed by Garcia, Uribe, Tavares et al. [31].
2.3. Procedure
The experiment was conducted in the laboratory of Neuroscience and Behavior of the University of Brasilia, inside a Faraday cage (WxHxD: 259 × 223 × 396 cm), in order to reduce electromagnetic interferences on the electrophysiological registration. The session began by marking and cleaning the sites for electrode placement with an abrasive gel (Nuprep®, Weaver and Company, Aurora, USA) for the EEG and with alcohol for the EKG. After positioning the electrodes, the electrophysiological signals were taken continuously as the task was performed by the subjects. At the end of the session, the electrodes were removed and the scalp of the subjects cleaned using gauze soaked in alcohol and water.
2.4. Stroop task
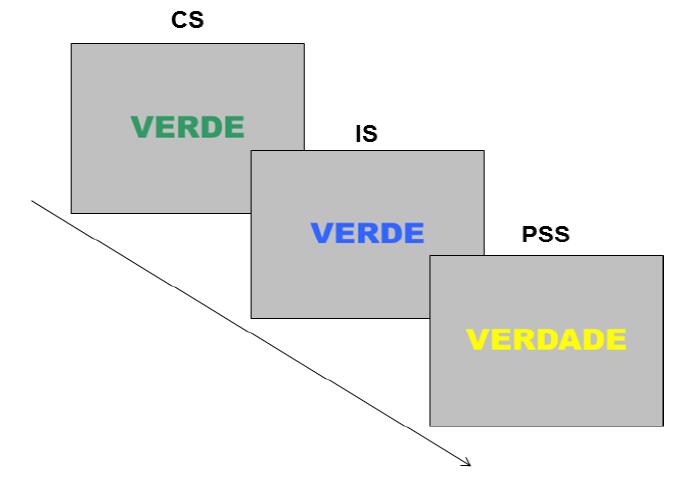
A computerized version of the Stroop task resembling the Victoria version [37], developed in our laboratory, was used (STROOP software, Borland Delphi, v. 7.0) in which a word at a time was presented to the participants during 800 milliseconds over a gray background (Figure 1). The subjects were instructed to name the color of the word aloud as quickly and accurately as possible. The task had three stages with 32 trials, each. The interval between the stages was solely used to change the stage and repeat the instructions, and the subjects were told to keep their eyes closed.
The words used were names of colors on the first two stages (red, blue, yellow and green, in Portuguese, vermelho, azul, amarelo, andverde) and phonetically similar to those words (in Portuguese, velho, cabul, marmelo, and verdade) on the last stage. At the first stage (congruent, CS) the two attributes of the stimuli (the word itself, and its color) matched, while at the second (incongruent, IS) and third (phonetic similarity, PSS) stages, those attributes could be different. The words were ordered pseudo-randomly in all stages. The audio was recorded using the MSWindows® Sound Recorded and later the responses given by the subjects were noted by the experimenters and compared to the words presented. The possible results were: a) hits, when the subjects' responses matched the color of the word presented; b) errors, when the responses differed from the color; and c) omission errors, when no responses were given.
2.5. Statistical analysis
The data was normally distributed according to visual inspections of QQ plots and interquartile range (IQR) test. Outliers were removed from data. One-way within subjects ANOVA (repeated measures) using the PSAW Statistics software (v. 18.0 for Windows) was used to compare the performance (hit rate and omission error rate) and HRV measures (SDNN, rMSSD, pNN50 and LF/HF ratio) between the stages (3). Post-hoc pairwise comparisons were performed using t-tests. The level of statistical significance was set at 5% (p ≤ 0.05) for all tests and adjusted for the post hoc tests by the Bonferroni method (p ≤ 0.0167 i.e. 0.05/3). Bonferroni adjustment for multiple comparisons was used on the reported p-values (LSD p-values × 3) and therefore these are significant at the .05 level. The results are presented as mean ± standard deviation (SD). Pearson bivariate correlations were also conducted using PSAW Statistics software and the same level of statistical significance (p ≤ 0.05).
The statistical analysis of the EEG data was performed using the parametric statistical tools of the open source EEGLAB platform, version 9.0.4.5 [32]. The statistical significance was 5% corrected by the false discovery rate method (FDR). The EEG results are presented in topographical maps for each frequency oscillation (Theta, Alpha, Beta and Gamma) and stage of the Stroop task. The cortical activity of each electrode was compared between the maps of the stages, two at a time, using paired samples t-tests (CS vs. IS, CS vs. PSS and IS vs. PSS).
Pearson bivariate correlation coefficients (r) and bootstrap bias-corrected and accelerated confidence intervals (BCa 95% CI) were calculated between the mean power (μV) measured in each EEG electrode and the HRV parameters. Correlations were considered significant and reliable for coefficients (r) that did not occur in the interval between −0.40 and 0.40, for p-values of less than or equal to 0.05 and confidence intervals that did not cross zero.
3. Results
3.1. Behavioral results
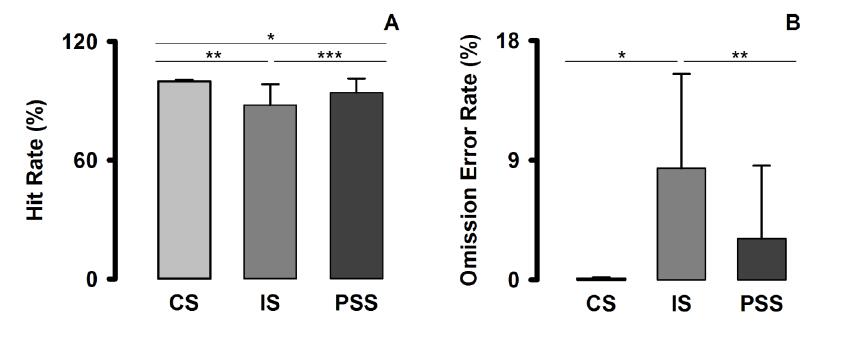
A significant difference was observed between the hit rate for all stages (F2, 42 = 18.673, p < 0.001, ηp² = 0.471, pairwise comparisons shown in Figure 2A). Analysis of variance also showed a main effect of stage for the omission error rate (Mauchly's test of sphericity: χ²(2) = 6.133, p = 0.047; Huynh-Feldt correction: ε = 0.845; ANOVA: F1.69, 35.48 = 16.318, p < 0.001, ηp² = 0.437), Figure 2B), but the pairwise comparison of omission errors between CS and PSS showed only a marginal effect after the Bonferroni adjustment (p = 0.051).
3.2. EEG Results
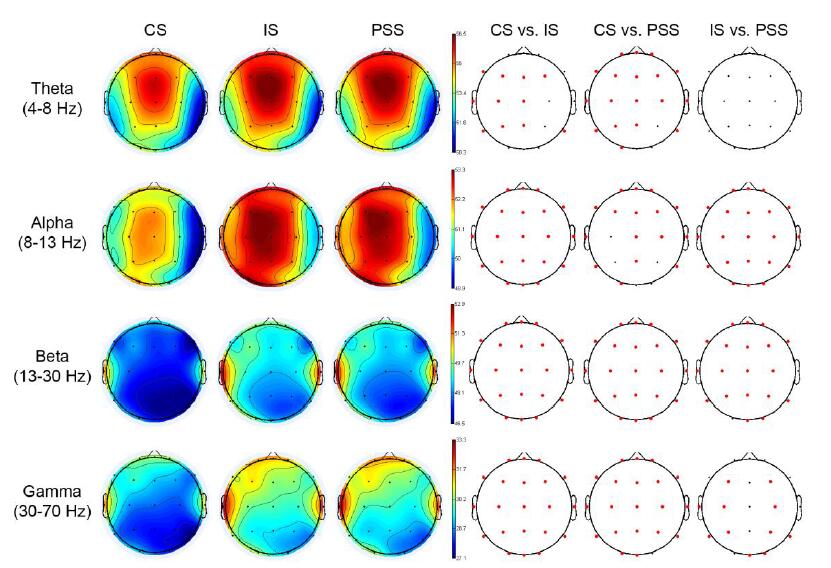
Electroencephalographic data were filtered and divided into traditional frequency oscillations: Theta (4-8 Hz), Alpha (8-13 Hz), Beta (13-30 Hz), and Gamma (30-70 Hz). Figure 3 shows the topographic maps of activity for each stage of the Stroop task. The colored bar on the topographical map indicates the power (μV) measured in each electrode in a gradient, where high power is represented by dark red color and low power by dark blue. The power "estimates the magnitude of oscillatory amplitude within a defined time window" [38]. Paired samples t-tests with correction by the FDR method were used to compare power in each electrode between two conditions. Therefore, comparison maps on the right in Figure 3 depicts significant differences, represented by red dots, between CS vs. IS, CS vs. PSS, and IS vs. PSS, respectively. T and p-values comparing each electrode across stages of the Stroop task are presented in Table 1.
Table 1. Paired samples t-test results, t and p values, for comparisons of mean power at EEG electrode sites between the stages of the Stroop task, two at a time, for each frequency oscillation. Degrees of freedom were equal to 49 for all the comparisons.
EEG
Electrode
Site | CS vs. IS | CS vs. PSS | IS vs. PSS |
| θ | α | β | γ | θ | α | β | γ | θ | α | β | γ |
| Fp1 | 2.13* | 4.16*** | 4.26ns | 3.61*** | 2.85** | 2.68* | 3.98*** | 3.56*** | -0.70ns | 3.36** | 2.87** | 1.34ns |
| F3 | 2.75** | 4.54*** | 4.77* | 4.02*** | 2.87** | 3.08** | 3.74*** | 2.98** | -0.17ns | 3.20** | 3.29** | 2.59* |
| C3 | 2.83** | 4.10*** | 5.32** | 4.12*** | 2.88** | 2.09* | 3.89*** | 2.90** | 0.67ns | 3.71*** | 3.99*** | 3.17** |
| P3 | 2.61* | 3.61*** | 5.23** | 3.81*** | 2.81** | 1.77ns | 3.98*** | 2.97** | 0.47ns | 3.21** | 4.41*** | 2.52* |
| O1 | 1.99ns | 4.17*** | 5.22** | 3.81*** | 2.57* | 2.97** | 4.26*** | 2.93** | -0.74ns | 2.56* | 1.78ns | 1.15ns |
| F7 | 2.32* | 4.28*** | 4.51* | 3.86*** | 3.05** | 3.37** | 4.49*** | 3.42** | -0.82ns | 3.21** | 2.38* | 1.71ns |
| T3 | 2.42* | 4.67*** | 4.90* | 4.68*** | 3.13** | 4.04*** | 4.84*** | 4.04*** | -0.37ns | 2.81** | 2.53* | 3.02** |
| T5 | 2.91** | 5.13*** | 5.14** | 4.48*** | 2.79** | 3.65*** | 4.30*** | 3.15** | 1.57ns | 4.08*** | 3.55*** | 3.84*** |
| Fp2 | 1.71ns | 3.60*** | 4.01ns | 3.36** | 2.59* | 2.20* | 3.78*** | 3.15** | -1.11ns | 3.10** | 2.44* | 1.41ns |
| F4 | 2.38* | 4.11*** | 4.99** | 3.99*** | 2.93** | 2.46* | 4.36*** | 3.23** | -0.30ns | 3.60*** | 3.42** | 2.47* |
| C4 | 2.21* | 3.90*** | 5.44** | 4.10*** | 2.59* | 2.21* | 3.96*** | 2.97** | -0.05ns | 3.29** | 3.87*** | 3.02** |
| P4 | 2.01ns | 3.75*** | 5.11** | 3.86*** | 2.05* | 1.73ns | 3.68*** | 3.21** | 0.70ns | 3.19** | 4.25*** | 2.45* |
| O2 | 1.17ns | 3.87*** | 4.08ns | 2.97** | 1.17ns | 1.87ns | 3.22** | 1.82ns | 0.35ns | 3.47** | 2.95** | 2.57* |
| F8 | 1.55ns | 3.48** | 4.23ns | 2.97** | 2.42* | 2.36* | 3.73*** | 2.50* | -1.26ns | 3.28** | 2.89** | 1.77ns |
| T4 | 1.63ns | 4.42*** | 5.48** | 4.08*** | 2.12* | 2.91** | 3.00** | 2.49* | -0.20ns | 3.14** | 1.51ns | 1.55ns |
| T6 | 2.36* | 5.31*** | 5.54** | 4.44*** | 2.62* | 3.65*** | 4.80*** | 3.95*** | 0.46ns | 3.95*** | 3.35** | 2.44* |
| Fz | 2.31* | 4.13*** | 4.80* | 3.72*** | 2.92** | 2.41* | 4.15*** | 3.25** | -0.45ns | 3.30** | 3.68*** | 1.78ns |
| Cz | 2.34* | 4.65*** | 5.05** | 4.54*** | 2.54* | 2.70** | 3.79*** | 3.93*** | 0.31ns | 3.57*** | 4.54*** | 1.96ns |
| Pz | 2.43* | 4.39*** | 5.09** | 4.44*** | 2.76** | 2.59* | 3.92*** | 4.09*** | 0.16ns | 3.14** | 4.34*** | 1.45ns |
| Fpz | 1.77ns | 3.83*** | 3.85ns | 3.51** | 2.64* | 2.37* | 3.76*** | 3.41** | -1.23ns | 3.28** | 2.38* | 1.09ns |
| Oz | 1.95ns | 4.18*** | 4.57* | 3.43** | 1.42ns | 2.07* | 3.30** | 2.04* | 1.06ns | 3.32** | 3.42** | 2.50* |
| CS: Congruent stage, IS: Incongruent stage, PSS: Phonetic similarity stage, θ: Theta oscillations, α: Alpha oscillations, β: Beta oscillations, γ: Gamma oscillations, ns: non:significant p-values, *p ≤0.05, **p ≤ 0.01, ***p ≤ 0.001. |
Theta activity (Figure 3A) at all stages was more evident among Fz and Cz electrode, but there was also activation from frontopolar to parietal regions in the midline with attenuation around. For IS and PSS, there was also higher activation in F3 electrode. For all conditions it was possible to notice left lateralization towards the left occipital region (O1 electrode). Only comparisons between IS and PSS did not show significant differences.
Topographic maps of IS and PSS in Alpha oscillation (Figure 3B) showed high activity from the left frontal pole to the ipsilateral occipital region through the midline. Noticeable activity occurred in F3, Fz, Cz. Although congruent stage is topographically similar to the other two, activity in frontopolar and F3 region are not as marked as observed in other stages. For all electrodes significant differences were found, except in the comparison between CS and PSS for C3, P3, P4, Oz, and O2 electrodes.
Beta activity (Figure 3C) at all stages was high in the superior temporal region in both hemispheres, and also activity in Fp1, Fp2, F3, F4 and C3 electrodes with decreased power in the other regions. Only O1 and T4 electrodes did not differ in the comparison between IS and PSS.
At all stages, Gamma activity (Figure 3D) was high in superior temporal regions of both hemispheres. Left lateralization for the frontopolar region, F3 and O1 electrodes was observed as well. Attenuation towards the other brain regions was more pronounced in CS but also present in IS and PSS. Power in all electrodes was significantly different for the comparisons between stages, except for the one between IS and PSS.
Power in all oscillations was lower in CS when compared to the other two.
3.3. HRV Results
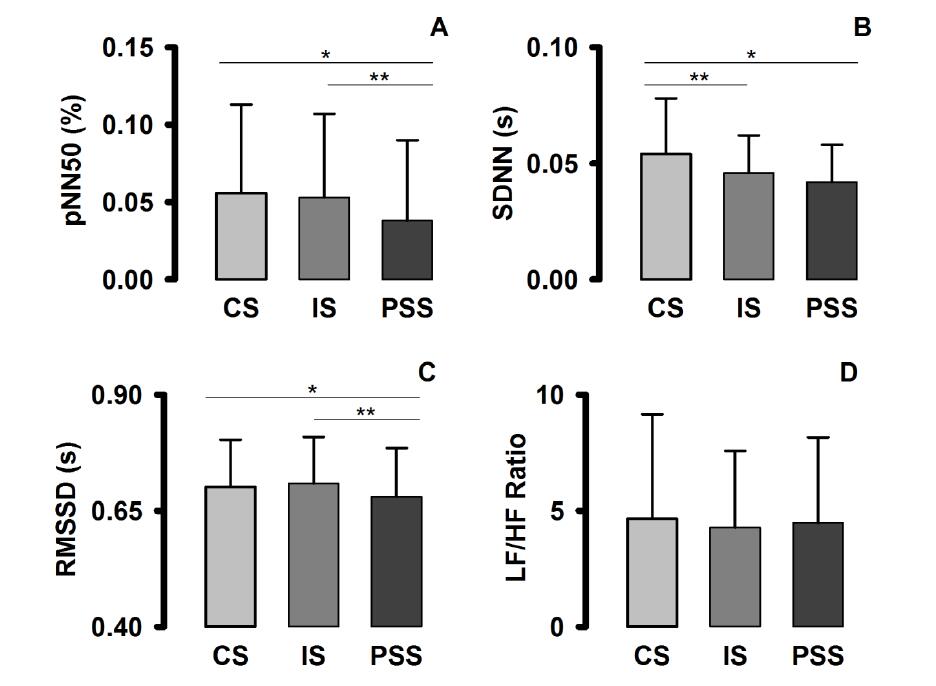
Heart rate variability (HRV) was analyzed in the time domain for SDNN, rMSSD, and pNN50 parameters and in the frequency domain for the LF/HF ratio. Main effect of stage was observed for pNN50 (F2, 96 = 6.211, p < 0.003, ηp² = 0.115, Figure 4A). Pairwise comparisons indicated that pNN50 was lower for PSS when compared with CS (p = 0.007) and IS (p = 0.031). The same pattern was found for rMSSD (Figure 4C), i.e. main effect of stage (F2, 96 = 12.631, p < 0.001, ηp² = 0.208) and significantly lower values for PSS when compared to CS (p = 0.006) and IS (p < 0.001). SDNN was also mainly influenced by the stage (Mauchly's test of sphericity: χ²(2) = 6.889, p = 0.032; Huynh-Feldt correction: ε = 0.911; ANOVA: F1.82, 87.45 = 8.586, p = 0.001, ηp² = 0.152; Figure 4B), with significant pairwise comparisons for CS versus IS (p = 0.029) and PSS (p = 0.003). There was no significant main effect of stage on the LF/HF ratio (Mauchly's test of sphericity: χ²(2) = 18.578, p < 0.001; Huynh:Feldt correction: ε = 0.773; ANOVA: F1.55, 74.21 = 0.252, p = 0.72, ηp² = 0.005), Figure 4D).
3.4. Correlation results
Pearson bivariate correlation coefficients were calculated between mean power (μV) measured at each EEG electrode and mean HRV parameters at Stroop stages. Correlations were considered significant and reliable for coefficients (r) that did not occur in the interval between −0.40 and 0.40, for p-values of less than or equal to 0.05 and confidence intervals that did not cross zero.
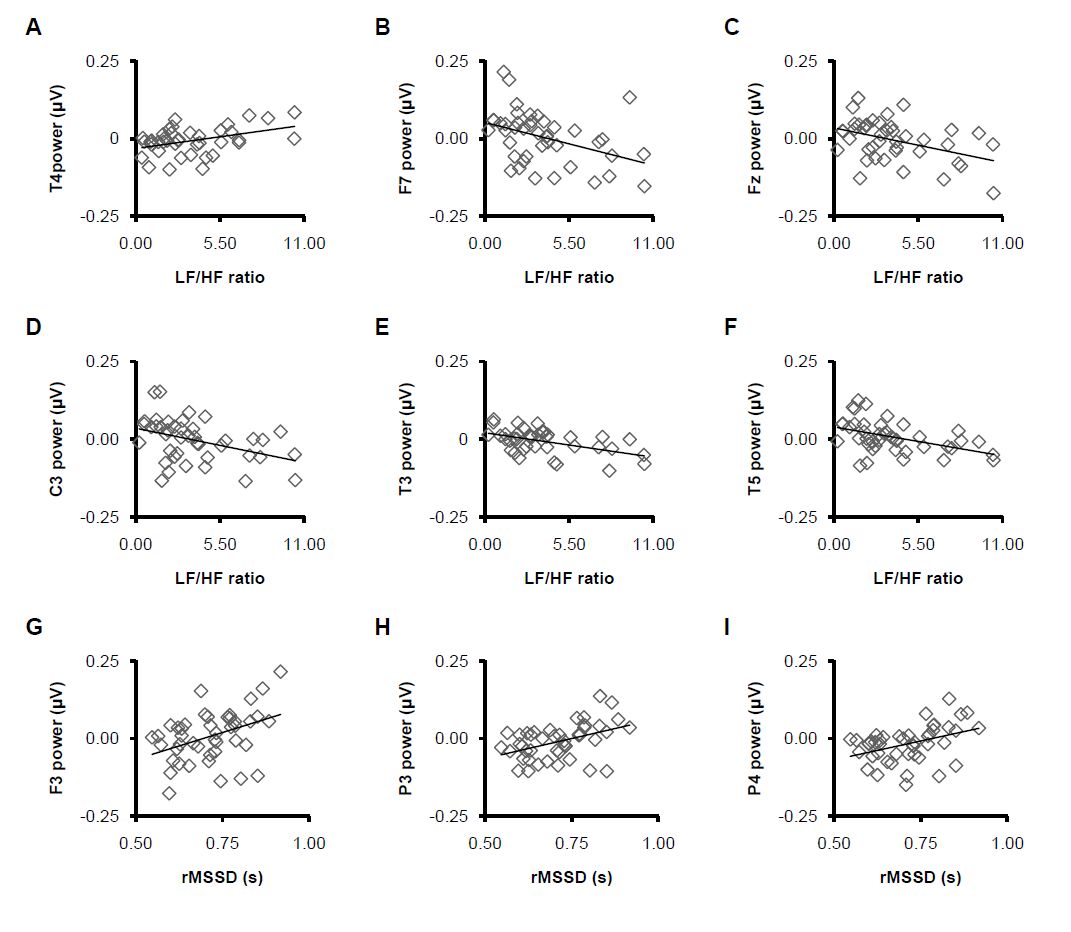
A significant positive correlation was found between LF/HF ratio and power in T4 electrode for CS stage (r = 0.41, BCa 95% CI [0.12, 0.64], p = 0.01). For PSS stage, there were negative correlations between LF/HF and F7 (r = −0.40 [−0.66, −0.05]), Fz (r = -0.42 [-0.69, −0.09]), C3 (r = −0.40 [−0.62, −0.15]), T3 (r= −0.50 [−0.70, −0.24]), and T5 (r = −0.46 [−0.64, −0.24], all ps ≤ 0.01). Positive correlations were also found in PSS between rMSSD and F3 (r = 0.40 [0.10, 0.65]), P3 (r = 0.44 [0.11, 0.67]), and P4 (r = 0.40 [0.14, 0.63], all ps ≤ 0.01). Scatterplots in Figure 5 summarize these results. No significant or reliable correlations were observed for IS, neither in the remaining variables for CS, nor PSS. Table 2 shows correlations found for all stages (ps ≤ 0.05), including those with coefficients occurring in the interval between -0.40 and 0.40.
Table 2. Correlation coefficient (r) and bootstrap bias-corrected and accelerated confidence intervals (BCa 95% CI, reported in square brackets) resulting from significant (p ≤ 0.05) Pearson correlations between HRV parameters and EEG electrodes at each Stroop stage. Only underlined correlations were considered for discussion, as their coefficients are higher than 0.40 or lower than -0.40 and their confidence intervals did not cross zero.
Stroop
stage | HRV
parameter | EEG
electrode
site | r
[BCa 95% CI] |
| CS | SDNN | O2 | 0.35*
[0.12, 0.57] |
| CS | LF/HF | T4 | 0.41**
[0.12, 0.64] |
| IS | rMSSD | Oz | -0.33*
[-0.58, -0.03] |
| IS | LF/HF | F3 | -0.33*
[-0.63, -0.08] |
| PSS | SDNN | C4 | -0.32*
[-0.52, -0.08] |
| PSS | SDNN | O2 | -0.37*
[-0.57, -0.13] |
| PSS | SDNN | F8 | -0.39**
[-0.59, -0.15] |
| PSS | SDNN | Cz | -0.35*
[-0.63, -0.01] |
| PSS | rMSSD | F3 | 0.40**
[0.10, 0.65] |
| PSS | rMSSD | C3 | 0.37**
[0.00, 0.67] |
| PSS | rMSSD | P3 | 0.44***
[0.11, 0.67] |
| PSS | rMSSD | C4 | 0.32*
[-0.01, 0.58] |
| PSS | rMSSD | P4 | 0.40**
[0.14, 0.63] |
| PSS | rMSSD | T6 | 0.33*
[0.03, 0.56] |
| PSS | rMSSD | Fz | 0.37**
[0.01, 0.66] |
| PSS | rMSSD | Cz | 0.35*
[0.04, 0.61] |
| PSS | rMSSD | Pz | 0.31*
[-0.01, 0.59] |
| PSS | LF/HF | F3 | -0.31*
[-0.63, 0.04] |
| PSS | LF/HF | C3 | -0.40**
[-0.62, -0.15] |
| PSS | LF/HF | P3 | -0.35*
[-0.61, -0.05] |
| PSS | LF/HF | F7 | :0.40**
[-0.66, -0.05] |
| PSS | LF/HF | T3 | -0.50***
[-0.70, -0.24] |
| PSS | LF/HF | T5 | -0.46***
[-0.64, -0.24] |
| PSS | LF/HF | T4 | -0.30*
[-0.52, -0.05] |
| PSS | LF/HF | Fz | -0.42**
[-0.69, -0.09] |
| PSS | LF/HF | Cz | -0.34*
[-0.67, 0.05] |
| PSS | LF/HF | Pz | -0.33*
[-0.56, -0.09] |
| CS: Congruent stage, IS: Incongruent stage, PSS: Phonetic similarity stage, * p ≤ 0.05, ** p≤ 0.01, *** p ≤ 0.001. |
4. Discussion
4.1. Discussion of behavioral results
Significant differences for the hit rate were found between all stages with increasing difficulty in congruent, phonetic similarity and incongruent stages, in this order. This suggests that there was a Stroop interference effect on the accuracy of the subjects' responses. Therefore, we assumed that the test was working properly and demanded executive functions.
PSS may be regarded as a stage of intermediate difficulty between CS and IS based both on the hit rate and on the omission error rate as for the last one it does not differ from CS. This is in line with other studies, in which the accuracy and reaction times in stages using strings of letters or words that are not color names are intermediate compared to the congruent and incongruent stages [10,11,12]. Another study that used words phonetically similar to color names showed the same pattern of difficulty between stages as ours, based on reaction times [13].
According to the neural network model of the Stroop task mentioned in the Introduction session, the congruent stage does not induce conflict between the two attributes of the stimulus (word itself and color) and consequently the subject is able to present a correct response based on any of those attributes [18]. Therefore, although it is expected that the subjects present baseline levels of focused attention because they keep attending to the rule of the task as it is performed, their behavior does not necessarily demand high levels of selective attention and inhibitory control, which makes CS less complex.
As the word in the PSS does not refer to a color, but phonetically resembles the name of a color, it is expected a higher conflict than when both attributes are coincident (CS), but less than when they are incongruent (IS) [10]. This hypothesis was supported by the results of the hit rate and the omission error rate presented by the participants in this study.
In the study by Salo, Henik and Robertson [12], the reaction time for neutral stimuli consisting of words unrelated to color names was higher than for non-lexicons neutral stimuli (e.g., "XXXX") in the Stroop task. This indicates that there is conflict when neutral words are presented to the subjects, since the subjects are also more prone to reading them than to naming its color. However, the conflict is reduced because there cannot be incongruity between the two attributes of the stimulus since the word is not a color name. Milham, Erickson, Banich et al. [20]noted that the congruent stage of the Stroop task actually involves less conflict than a neutral one and possibly demands less inhibitory control; but the congruent and incongruent stages demands more attentional control than the neutral stage because there are two sources of information about color, the meaning of the word itself and the color in which it is written, and if this information are not coincident, the demand for attention increases further.
Both hit ratio and omission error rate were significantly different between incongruent stage and the others. Therefore, this stage possibly increased the demand for inhibitory control and attention. The increase in omission error rate indicates that for this stage, the conflict generated was enough to impair the performance of subjects, possibly because of the failure in inhibiting the tendency to read the word.
4.2. Discussion of EEG results
EEG results showed a difference in the pattern of cortical activation when comparing the lower (Theta and Alpha) to the higher oscillations (Beta and Gamma), in line with the evidence of the existence of two processing modes in the human EEG, respectively global and local modes [15]. Moreover, the power in the congruent stage was lower than in the other two stages for all the oscillations analyzed, which highlights the evidence of reduced demand for executive resources in CS.
The topographic distribution of Theta and Alpha oscillations occurred in a frontoparietal gradient. There is evidence that executive functions do not depend on the isolated activity of the prefrontal cortex, but also on the activity of the posterior cortex [16] in a synchronized frontoparietal network between Theta and Alpha oscillations, especially when there is greater demand of the central executive [39].
Although there was activation of both hemispheres, there was marked activity on the left hemisphere in all stages of the Stroop task. This result is in line with the traditional notion of specialization of the left hemisphere for language [40]. This might relate to the verbal response required from the subjects what could have led to the activation of regions on the left hemisphere involved in spoken language and in cases in which the subjects were not able to inhibit reading the word, even with regions involved in written language, also in the left hemisphere.
Regarding Theta oscillation, the marked activity in Fz, Cz and F3 electrode sites, corresponding to prefrontal and motor cortices, during IS and PSS may indicate that these stages require more cognitive resources related to the PFC such as attention, mental effort, and inhibitory control than CS. This is consistent with the behavioral results, for which the incongruent stage was regarded as the most difficult, followed by PSS. However, cortical activity in IS and PSS did not show significant differences for the Theta oscillation.
The marked activity on the F3 electrode site during the incongruent stage is of particular interest, since this site is related to the region of the dorsolateral prefrontal cortex (DLPFC), and the activation of this region is related to the manipulation of data stored in working memory [41], which might induce to the behavioral success on the inhibition of inappropriate responses [42]. The activation in the left occipital region (O1 electrode) may be related to the mental representation and the processing of the color of the word, as both occur in the visual cortex [41
]. The involvement of the left occipital region at Theta oscillation was also observed in the study by Ghimire, Paudel, Khadka et al. [19] and was more evident in the incongruent condition. This can be interpreted as evidence of greater influence of the irrelevant attribute of the stimulus (the word itself) at the incongruent stage, which may have contributed to the performance cost when compared to the congruent stage.
The topographic distribution in Alpha oscillation was similar to Theta. However, the activity in the frontal poles was even less marked in Alpha during the congruent stage, indicating again that this stage demands less activity in areas related to the PFC and is less difficult than the other two. In another study, activity in the frontal poles (Fp electrode sites) in IS and PSS, near the orbitofrontal prefrontal cortex (OFPFC) region, was positively correlated with success in behavioral performance in a Go/No-Go task [18], indicating that this region is important for inhibitory mechanisms. Increased activity of the frontal poles was also observed during the incongruent stage on the study by Hanslmayr, Pastotter, Bauml et al. [10].
The neural network model for the Stroop task [17] contributes for the comprehension of the topographical power spectrum distribution pattern found in this study, with involvement of the DLPFC (F3) during IS and PSS. This region is supposedly associated with the activation of an attentional control system in response to the detection of a conflict by the anterior cingulate cortex (even knowing that the conflict is smaller in PSS), leading to increased attentional demand. The cingulate cortex activity, important in tasks that require sustained attention, is depicted on the frontal midline activity in the Theta oscillation [21,43] and was evident in incongruent and phonetic similarity stages in this study.
Activation at T3 electrode, corresponding to the superior and middle temporal gyri [44], shown in Beta and Gamma oscillations might relate to the mental representation of the word itself, according to the neural network model for the Stroop task [17,18]. It could also be related to the verbal processing of word, which also occurs in temporal regions, as shown in the fMRI study by Milham, Erickson, Banich et al. [20] while subjects were performing the Stroop task. Activity on the left temporal lobe has also been observed when nouns are being processed [45]. A study with a task in which subjects had to generate color names showed bilateral activity of the temporal lobe more marked in the left hemisphere and also activation of the left DLPFC, which was interpreted by the authors as related to the evocation of the word [46]. This pattern is similar to the one found in our study.
The color processing was evidenced by the activity of the left occipital lobe (O1 electrode), similar to that observed in Theta and Alpha oscillations. Also, similarly to those oscillations, there was activity in the frontal poles and in the left DLPFC.
In Beta oscillation, there was a significant increase in the power of the temporal activity during IS and PSS, especially on the left side. In Gamma, no significant differences between IS and PSS were observed in frontal regions (except for F3 and F4), in the left occipital region, as well as in central midline region, which is similar to the comparison of the power spectrum distribution between these two stages in Theta oscillation.
The electroencephalographic evidence of the color processing (occipital region) and of the word processing (temporal region) indicates that both of the stimuli's attributes were processed. The fact that the activity on those regions was higher during the incongruent and phonetic similarity stages indicates that there really existed a conflict, which detection and resolution demanded higher activity of prefrontal regions.
4.3. Discussion of HRV results
Time-domain HRV parameters results indicated the existence of an interference effect for the Stroop task as it was observed for the behavioral results. However, only the comparison between CS and PSS was significant for all measures in the time domain. This indicates that there was a dominance of parasympathetic activity during the congruent stage, suggesting that this stage demanded less executive abilities from the subjects.
rMSSD and pNN50 results differ between PSS and IS, whereas SDNN differed between CS and IS. The results in studies that assess the HRV during the Stroop task are controversial regarding these time domain measures. Sometimes they are lower during the execution of this task in comparison with a baseline [24,25], indicating sympathetic dominance during the test. In another study, SDNN and rMSSD tended to be lower during a neutral stage, and comparable between the congruent and incongruent stages [26]. When the version of the Stroop task had only one stage, similar to the incongruent stage of this study, rMSSD was significantly lower during the task than in a resting condition [23]. Similarly, rMSSD correlated positively with the high frequency content (HF: 0.15 to 0.40 Hz, modulated by the parasympathetic division of the ANS), which was in turn positively associated with the behavioral performance in this stage [27]. Despite being difficult to interpret, it is noticeable that in most studies, time-domain parameters are lower during difficult and cognitively loaded conditions.
In the frequency domain, for the LF/HF ratio, there were no significant differences between the stages. These results are consistent with the comparison between the Stroop task and a baseline performed by [25], in which there were no significant differences in the LF/HF ratio. However they contrast with other studies where this measure was higher in an interference condition of the task compared to the baseline [24,28]. Dupuy, Lussier, Fraser et al. [26] also did not find significant differences in LF/HF ratio among incongruent, congruent and neutral stages, which is in line with our study.
Taken together, the results of the HRV time domain measures indicate that there was a decrease in the autonomic parasympathetic activity during the Stroop task for PSS and IS compared to CS, which indicates an increase in mental stress in the former stages.
4.4. Discussion of correlation results
Interaction between neural and cardiac systems during the execution of the Stroop task (shown in Figure 5 and Table 2) was found at CS stage solely between LF/HF ratio and electrode T4. In the PSS stage, correlations were found among LF/HF ratio and F7, Fz, C3, T3, and T5 electrodes, and also among rMSSD and F3, P3, and P4 electrodes. No significant and reliable correlations were found for the IS stage.
In view of all the variables that influence HRV—such as those related to subcortical activity, ANS activity, endocrine system, HR itself, among others—correlations between cortical activity and HRV are impressive, despite of being moderate.
Considering the possible existence of an integrated inhibitory neural network responsible for mediating HRV and executive functions, Thayer, Hansen, Saus-Rose et al. [14] suggested that decreased activity in the prefrontal cortex would lead to a concomitant sympathetic dominance on HR control. This would be indexed by decreased time-domain HRV and increased LF/HF ratio, for example. These authors also state that adaptation to each specific behavioral situation, i.e., different executive tests, is complex and can recruit additional central nervous system structures. However the relation between these other regions and HRV was not clear in their revision.
There was a moderate, positive correlation between power in T4 electrode and LF/HF ratio during CS stage. Scatterplot shows a distribution mainly towards low electrode power associations with low LF/HF ratio (Figure 5A). Therefore, the majority of subjects presented parasympathetic dominance associated with recruitment of temporal regions, possibly for word processing. This is in line with EEG results showing lower power in CS and also with HRV results indicating vagal dominance for this stage. However, as the relation between HRV and the activity of neural regions outside the integrated inhibitory neural network is not clear, it was not possible to state whether this finding goes in line with the theory by Thayer, Hansen, Saus-Rose et al. [14].
During PSS, LF/HF ratio was negatively correlated with frontal, central and temporal region activity (Figure 5B-F). This indicates a somewhat consistent relationship between prefrontal cortical activity and distributed posterior cortical activation. Increases in EEG power were correlated with decreases in LF/HF ratio. This suggests that the higher executive demand during PSS stage recruited prefrontal cortex, as well as in central and temporal cortical regions, was associated with higher levels of parasympathetic dominance. This is in line with the integrated inhibitory neural network model suggested by Thayer, Hansen, Saus-Rose et al. [14].
Also during PSS, rMSDD was positively correlated with mean power in F3, P3, and P4 electrodes (Figure 5G-I). Therefore, increased cortical activity was correlated with an increased time-domain HRV parameter. High EEG power was therefore associated with parasympathetic dominance, which again is in line with the network model of Thayer, Hansen, Saus-Rose et al. [14].
4.5. Final discussion
Considering the results together we may infer lower difficulty and absence of conflict on the congruent stage as evidenced by the behavioral (higher hit rate compared to the other two stages, lower errors of omission rate when compared to IS), electrophysiological (decrease in the power of cortical activation, attenuation in the OFPFC activity for the Alpha oscillation and increased time-domain HRV parameters) and interaction results (showing parasympathetic dominance associated with temporal cortical activity).
Behavioral results suggested an intermediate difficulty for PSS. However, EEG results showed no differences in cortical activation between IS and PSS for Theta oscillation. In Alpha and Beta, the differences observed between IS and PSS are apparently related more to the decrease on the power spectrum than to the topographic distribution of the cortical activity. Decreased pNN50 and rMSSD parameters during PSS compared to CS and IS, and decreased SDNN compared to CS indicated that mental stress for PSS was higher than in the other stages.
Considering that HRV is an index of the success of executive brain mechanisms as suggested by Thayer, Hansen, Saus-Rose et al. [14], HRV results for the PSS should be associated with a greater behavioral collapse in this stage when compared to the others, as they indicate high mental stress. Three factors help understanding why the impairment in PSS was not as great as in IS. Firstly, the interaction results among LF/HF ratio, rMSSD, and EEG power suggested that at PSS, higher recruitment of prefrontal cortex was associated with parasympathetic dominance. Hansen, Johnsen and Thayer [47]showed that a group of subjects with high HRV (i.e. parasympathetic dominance) performed better in terms of speed and accuracy on executive tests than the low HRV group. Secondly, there was conflict but not incongruity between the two attributes of the word in PSS. Finally, cortical activation in PSS stage was very similar to IS. These are important factors to consider when explaining the intermediate difficulty described for PSS. Nevertheless, stating only that PSS presented intermediate difficulty is probably insufficient to describe the results found since there is evidence that it resembled IS at the electroencephalographic level but generated higher mental stress.
Although HRV results indicated higher mental stress in PSS and IS in comparison with CS, correlations and scatterplots showed overall parasympathetic dominance due to the increased prefrontal cortex activity observed in EEG topographical maps. Both recruitment of areas associated with executive functions and high levels of time-domain HRV (and therefore low levels of LF/HF ratio) were important for the general behavioral success. That is, although IS and PSS were more difficult for the subjects, their behavior was not as impaired as it would be for patients with dysexecutive syndrome and/or older subjects [16], for instance.
Two factors can be highlighted as limitations of the present study as an attempt to improve future protocols: a) the absence of reaction time measures in the Stroop task, and b) the difficulty in separating the contributions of the topographic distribution and the power spectrum values to account for the significant differences observed in the topographic EEG maps.
5. Conclusion
This study reinforces the importance of proper PFC functioning and related success in executive functions for real-world and everyday activities. These activities commonly demand inhibition and therefore need adequate physiological and behavioral responses in order to allow appropriate adaptation of individuals to environment and society.
Acknowledgments
This research was supported by a Social Demand scholarship from Brazilian Federal Agency for Support and Evaluation of Graduate Education, Ministry of Education (CAPES) to S. L. S. Canabarro. The authors would like to express their gratitude for the Brazilian National Research Council (CNPq) that supported this work (M.C. H. Tavares, PQ 2015, 311582/2015-0). We would also like to thank Prof. Concepta McManus Pimentel for her extensive English review and feedback on the manuscript.
Conflict of Interest
All authors declare no conflicts of interest in this paper.









 DownLoad:
DownLoad: