The rise of nanotoxicology: A successful collaboration between engineering and biology

1.   Introduction

For the spatial Newtonian $n$-body problem, the equations of motion for the $n$ masses $m_{k} > 0$ and positions $x_{k}\in\mathbb{R}^{3}$ with $k\in\{1, \ldots, n\}$ can be described by Newton's second law and Newton's universal gravitation law:

Define

and let

be the collision set. As usual, the set $\Omega\setminus\triangle$ is called the configuration space. First, we introduce the definition of central configuration for the Newtonian $n$-body problem (see ^[1]).

Definition 1.1. Given $n$ masses $m_{k} > 0$ with positions $q_k \in \mathbb{R}^{3}$, $k = 1, \ldots, n$, we say a configuration $q\in \Omega\backslash\triangle$ is a central configuration at some moment if there exists a constant $\lambda\in\mathbb{R}$ such that

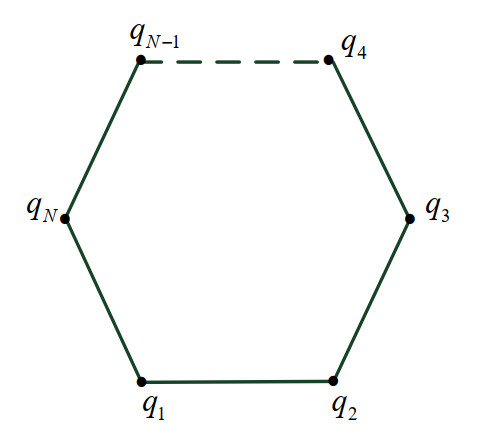
Central configurations play a very important role in the study of the Newtonian $n$-body problem, and especially, central configurations can lead to rigid-motion solutions and homothetically collapsing solutions ^[1]. Central configurations of the Newtonian three-body ($n = 3$) problem with any given three masses have long been known, and there are always exactly two kinds of central configurations: Euler collinear central configuration and Lagrange equilateral-triangle central configuration ^[2,3]. For a planar Newtonian $N$-body problem with $n = N\geq4$, Perko and Walter ^[4] proved that if $N$ masses are located at the vertices of a regular $N$-polygon (see Figure 1), then they can form a regular polygonal central configuration if and only if all the values of $N$ masses are equal to each other. For more results of planar central configuration with one regular $N$-polygon, one can refer to ^[5,6,7,8].

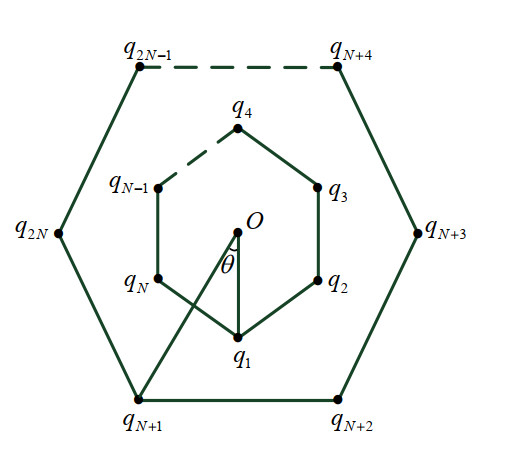
For a planar central configuration with $n = 2N$ and $N\geq2$ such that two regular $N$-polygons are concentric and that $2N$ equal masses are placed at the vertices of the two regular $N$-polygons (see Figure 2), Zhang and Zhou ^[9] proved that the values of masses in each separate regular $N$-polygon were equal. We say that $p$ regular $N$-polygons with $p\geq2$ are nested if they are coplanar and have the same number of vertices $N$ and the same center, and the positions of the vertices of the innermost regular $N$-polygon $\textbf{R}^{(1)}_{j}$ and those of the remaining $p$-1 regular $N$-polygons $\textbf{R}^{(k)}_{j}$ with any $k\in\{2, \ldots, p\}$ satisfy the relation that $\textbf{R}^{(p)}_{j} = s_{1}\textbf{R}^{(p-1)}_{j} = s_{2}\textbf{R}^{(p-2)}_{j} = \ldots = s_{p-1}\textbf{R}^{(1)}_{j}$ for some scale factors $s_{p-1} > s_{p-2} > \ldots > s_{1} > 1$ and for all $j = 1, 2, \ldots, N$. For the central configuration such that two regular $N$-polygons are nested, masses on different regular $N$-polygons may be different, and Moeckel and Simó ^[10] proved that for every mass ratio $b$ between the two masses, there were exactly two planar central configurations. Also, for the case of $n = 2N$ such that $N$ equal masses are placed at the vertices of one regular $N$-polygon and the remaining $N$ equal masses are placed at the vertices of the other regular $N$-polygon, which is rotated exactly at an angle $\theta = \pi/N$ with respect to the former regular $N$-polygon, Barrabés and Cors ^[11] proved the existence of the planar central configuration with any value of the mass ratio. For the case of $n = pN$ and $p\geq2$, Corbera, Delgado, and Llibre ^[12] proved the existence of the nested central configuration such that $pN$ masses were at the vertices of the $p$ nested regular $N$-polygon with a common center. Moreover, all the masses on the same regular $N$-polygon were equal, but masses on a different regular $N$-polygon could be different. For the case of $n = pN+gN$ with $p\geq1$ and $g\geq1$, Zhao and Chen ^[13] proved the existence of planar central configurations such that $p$ regular $N$-polygons were nested, and $g$ regular $N$-polygons were rotated exactly at an angle $\pi/N$ with respect to the other ones. For more details in this direction, we refer to ^{[14,15,16,17,18,19,20,21]} and the references therein.

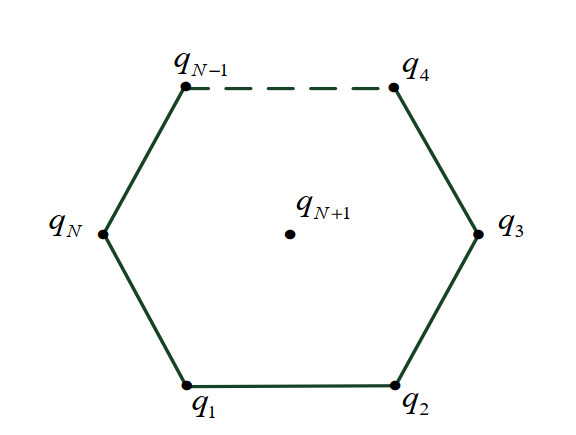
Note that for a planar central configuration with $n = N+1$, Chen and Luo ^[22] proved that if $N$ masses are located at the vertices of one regular $N$-polygon and the position of the ($N$+1)-th mass is on the plane containing the regular $N$-polygon (see Figure 3), then all the values of $N$ masses located at the vertices of the regular $N$-polygon are equal to each other. For a spatial central configuration with $n = N+1$ and the ($N$+1)-th mass off the plane containing the regular $N$-polygon (see Figure 4), Ouyang, Xie, and Zhang ^[23] showed that the distance between the ($N$+1)-th mass and the regular $N$-polygon was unique.

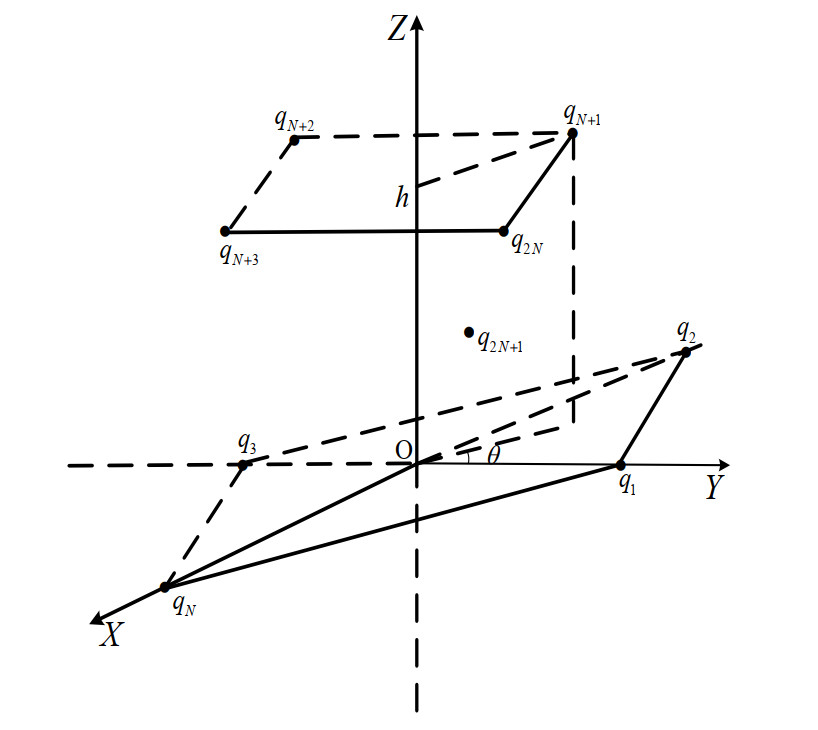
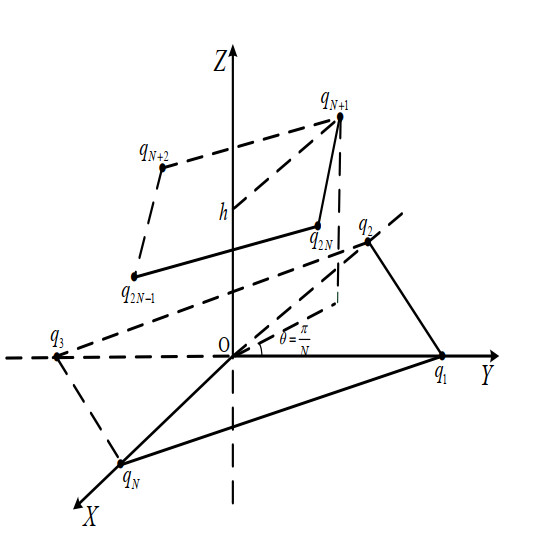
In this paper, we consider the spatial central configuration of a Newtonian ($2N$+1)-body problem in $\mathbb{R}^{3}$ formed by $2N$ masses placed at the vertices of two paralleled regular $N$-polygons with distance $h > 0$. It is assumed that the lower layer regular $N$-polygon lies in a horizontal plane, and the upper regular $N$-polygon parallels the lower one in $\mathbb{R}^{3}$ with distance $h$, and the $z$-axis passes through both centers of the two regular $N$-polygons (see Figure 5). For convenience, when choosing the coordinates, we treat $\mathbb{R}^{3}$ as the direct product of the complex plane and real axis. For the positions of the $2N$+1 masses $q = (q_{1}, q_{2}, \ldots, q_{2N}, q_{2N+1})\in\Omega\backslash\triangle$, we have

where $a$ is the ratio of the sizes of the two regular $N$-polygons, $\rho_{d}$ is the $d({\rm mod} \, \, N)$-th complex root of unity, i.e., $\rho_{k} = e^{i\theta_{k}}$ with $k = 1, 2, \ldots, N$, and $\rho_{l} = e^{i\theta_{l}}$ with $l = N+1, N+2, \ldots, 2N$ and $\theta_{d} = 2 d\pi/N$ with $d\in \mathbb{Z}$. Here, we define $\theta$ as the twist angle between the two paralleled regular $N$-polygons with distance $h > 0$. Moreover, for the position of the ($2N$+1)-th mass and the barycenter $x_{0} = (c_{0}, \, h_0)$, we define

Then, for the spatial twisted configuration with $n = 2N+1$ and the notations (1.2)–(1.3), we have the following results.

For the existence, we have the following:

Theorem 1.1. Suppose the values of $N$ masses with $N\geq2$ located at the vertices of one regular $N$-polygon are equal to each other, and all of the sides in the two regular $N$-polygons have the same size. Define the position of $q_{2N+1}$ as (1.3). Then, the $2N$+1 masses form a central configuration if and only if all the values of the $2N$ masses located at the vertices of the two regular $N$-polygons are equal to each other, $a_{1} = 0$ and $h_{2N+1} = h/2$, and the twist angle is $\theta = s\pi/N$ with $s\in\{0, 1, \ldots, 2N-1\}$.

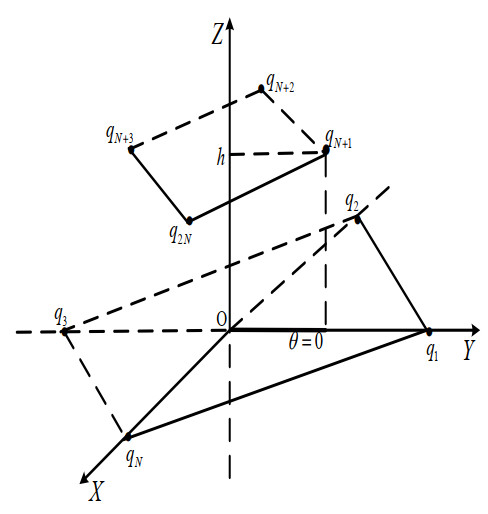
Remark 1.1. For the spatial twisted central configuration of the Newtonian $2N$-body problem, under the assumption that the values of masses in each separate regular $N$-polygon were equal, Yu and Zhang ^[24] proved that the twist angle must be $\theta = 0$ or $\theta = \pi/N$ (see Figures 6 and 7). Meanwhile, in Theorem 1.1, we consider the spatial twisted central configuration of the Newtonian ($2N$+1)-body problem. Under the assumptions that the values of the $N$ masses located at the vertices of one regular $N$-polygon are equal and that all of the sides in the two regular $N$-polygons have the same size, we not only obtain the values of the twist angle; but also prove that all $2N$ masses must be equal. Moreover, we know the position of the ($2N$+1)-th mass is $(0, 0, h/2)$.

For the uniqueness, we have the following:

Theorem 1.2. For the spatial twisted central configuration, if the values of the $N$ masses located at the vertices of one regular $N$-polygon are equal to each other and all of the sides in the two regular $N$-polygons have the same size, then for any $N\geq2$, both the distance between the two regular $N$-polygons and the position of the ($2N$+1)-th mass are unique.

2.   Preliminaries

Lemma 2.1. [24, Lemma 2.9] For any $a > 0$, any $\gamma\in(-\infty, \, +\infty)$, any $h > 0$, and any $N\geq2$, let

Then,

Remark 2.1. In Lemma 2.1, if we choose $a = 1$ and $\gamma = \pi/N$ where $N\geq2$, then

Lemma 2.2. [24, Lemma 2.10] If $\gamma\in(0, \pi/N)$ with $N\geq2$, then for any $a > 0$ and any $h > 0$, we have $f(\gamma) > 0$.

Lemma 2.3. If $\theta = s\pi/N$ with $s\in\{0, 1, \ldots, 2N-1\}$ and $N\geq2$, then for any $k^{\prime}\in\{1, 2, \ldots, N\}$, any $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$, and any $h > 0$, we have

Proof. Let $\hat{\mu}\in\{1, 2, \ldots, N\}$, $\tilde{\mu}\in\{0, 1, \ldots, N-1\}$, $\tilde{\alpha}\in(-2\pi, 2\pi)$, and $\kappa\in \{0, 1\}$. We define a mapping $\sigma$ by

Notice that both $\{\kappa N+1, \kappa N+2, \ldots, \kappa N+N\}$ and $\{\tilde{\alpha}, (2\pi)/N+\tilde{\alpha}, \ldots, 2(N-1)\pi/N+\tilde{\alpha}\}$ are finite sets; the mapping $\sigma$ is a surjection. Let us show $\sigma$ is an injective mapping. The proof for $\kappa = 0$ is similar to $\kappa = 1$; we only check for $\kappa = 1$. Let $\mu_{1}\neq \mu_{2}$ and $\mu_{1}, \mu_{2}\in\{N+1, N+2, \ldots, 2N\}$. If $\sigma(\mu_{1}) = \sigma(\mu_{2})$, then there exist $s_{1}, \, s_{2}\in\mathbb{Z}$ such that

Hence, $\mu_{1}-\mu_{2} = (s_{2}-s_{1})N$. By the facts that $-N < \mu_{1}-\mu_{2} < N$ and $s_{2}-s_{1}\in\mathbb{Z}$, $s_{2} = s_{1}$, and thus $\mu_{1} = \mu_{2}$, which is a contradiction. Therefore, $\sigma$ is injective, which implies that $\sigma$ is a bijection.

Similarly, for $l\in\{N+1, N+2, \ldots, 2N\}$, $\tilde{s}\in\{0, 1, \ldots, N-1\}$, and $\theta\in[0, \, 2\pi)$, if we define another mapping $\sigma_{1}$ by

then $\sigma_1$ is a bijection as well. Together with the fact that $\theta = s\pi/N$ with $s\in\{0, 1, \ldots, 2N-1\}$ is equivalent to $\theta = 2\tilde{s}\pi/N$ or $\theta = 2\tilde{s}\pi/N+\pi/N$ with $\tilde{s}\in\{0, 1, \ldots, N-1\}$, let us show (2.2) holds by considering the following two cases.

Case 1. $\theta = 2\tilde{s}\pi/N$ with $\tilde{s}\in\{0, 1, \ldots, N-1\}$.

Observing that $\theta_{j} = 2j\pi/N$ with $j\in\{1, 2, \ldots, N\}$,

which implies that

Let $k^{\prime}\in\{1, 2, \ldots, N\}$ and $\tilde{s}\in\{0, 1, \ldots, N-1\}$. In (2.3), if we choose $\mu = l$, $\hat{\mu} = k^{\prime}$, $\tilde{\mu} = \tilde{s}$, $\kappa = 1$, and $\tilde{\alpha} = 0$, then the mapping

is a bijection. Combining (2.6), and $\theta_{d} = 2\pi d/N$ with $d\in \mathbb{Z}$ and

for any $k^{\prime}\in\{1, 2, \ldots, N\}$ and any $\tilde{s}\in\{0, 1, \ldots, N-1\}$, we have

On the other hand, in (2.3), for any $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$ and any $\tilde{s}\in\{0, 1, \ldots, N-1\}$, if we let $\mu = k$, $\hat{\mu} = l^{\prime}$, $\tilde{\mu} = N-\tilde{s}$, $\kappa = 0$, and $\tilde{\alpha} = 0$, then we know that the mapping

is a bijection as well. Thus, similar to the procedure of obtaining (2.7), for any $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$, any $\tilde{s}\in\{0, 1, \ldots, N-1\}$, and any $h > 0$, we have

Employing (2.7), (2.8), and $\theta_{d} = 2\pi d/N$ with $d\in \mathbb{Z}$, we have

and

where $\tilde{s}\in\{0, 1, \ldots, N-1\}$, $k^{\prime}\in\{1, 2, \ldots, N\}$, and $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$. Thus, (2.2) holds for the case of $\theta = 2\tilde{s}\pi/N$ with $\tilde{s}\in\{0, 1, 2, \ldots, N-1\}$.

Case 2. $\theta = 2\tilde{s}\pi/N+\pi/N$ with $\tilde{s}\in\{0, 1, 2, \ldots, N-1\}$.

For any $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$ and any $\tilde{s}\in\{0, 1, \ldots, N-1\}$, in (2.3), if we choose $\mu = k$, $\hat{\mu} = l^{\prime}$, $\tilde{\mu} = N-\tilde{s}$, $\kappa = 0$, and $\tilde{\alpha} = -\pi/N$, then the mapping

is a bijection. Then, we have

where $\tilde{s}\in\{0, 1, \ldots, N-1\}$, $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$, and $h > 0$.

By the first equation of (2.9) and Remark 2.1, for any $\tilde{s}\in\{0, 1, \ldots, N-1\}$, any $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$, and any $h > 0$, we see that

Moreover, in (2.5), for any $l\in\{N+1, N+2, \ldots, 2N\}$ and any $\tilde{s}\in\{0, 1, \ldots, N-1\}$, if we choose $\theta = \pi/N$, then the mapping

is a bijection. Hence, combining (2.10), this leads to

Furthermore, one can verify that

and this implies that

Employing (2.9), (2.10), (2.11), and (2.12), for any $l, \, l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$ and any $\tilde{s}\in\{0, 1, \ldots, N-1\}$, we have

Thus, (2.2) holds for the case of $\theta = 2\tilde{s}\pi/N+\pi/N$, where $\tilde{s}\in\{0, 1, 2, \ldots, N-1\}$.

By Cases 1–2, we arrive at the conclusion that (2.2) holds for $\theta = s\pi/N$ with $s\in\{0, 1, \ldots, 2N-1\}$. □

Remark 2.2. Similar to dealing with the mappings $\sigma$ and $\sigma_{1}$, for any $k^{\prime}\in\{1, 2, \ldots, N\}$ and any $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$ with $N\geq2$, if one defines $\sigma_2$ and $\sigma_3$ by

and

then $\sigma_{2}$ and $\sigma_{3}$ are bijections.

Lemma 2.4. For any $\theta\in\mathbb{R}$, $N\geq2$, $a > 0$, $h > 0$, and $m > 0$, we have

Proof. In fact, (2.13) is equivalent to

It is easy to see that $k-l^{\prime}\in\mathbb{Z}$. Moreover, in (2.3), for any $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$ and any $\theta\in[0, \, 2\pi)$, if we let $\mu = k$, $\hat{\mu} = l^{\prime}-N$, $\tilde{\mu} = 0$, $\kappa = 0$, and $\alpha = \theta$, then the mapping

is a bijection, which implies that

Thus, Lemma 2.4 is true. □

Lemma 2.5. [4, Page 304] For any $N\geq2$, $\sum\limits_{{\atop{{1\leq j\leq N-1}}}}(1-e^{i\theta_{j}})/|1-e^{i\theta_{j}}|^{3} = [\sum\limits_{{\atop{{1\leq j\leq N-1}}}}\csc(j\pi/N)]/4$.

Lemma 2.6. [20, Pages 1431 and 1437] For any $N\geq2$, the inequality

holds.

Now, we introduce the definition of circulant matrix and state its properties.

Definition 2.1. [25, Pages 65–66] A matrix $\tilde{C} = (\tilde{c}_{kj})_{N\times N}$ is circulant if $\tilde{c}_{\hat{k}, \hat{j}} = \tilde{c}_{\hat{k}-1, \hat{j}-1}$ where $1\leq k, j, \hat{k}, \hat{j}\leq N$ and $N\geq2$.

In Definition 2.1, we take the circulant matrix $\tilde{C}$ as the following:

We have some properties for the special circulant matrix $C$.

Lemma 2.7. [4, Page 303] The circulant matrix $C$ has the same forms of the eigenvalues $\lambda_{j}(C)$ and the corresponding eigenvectors $\xi_{j}$; more precisely,

where $N\geq2$ and $\rho_{j-1} = e^{i\theta_{j-1}} = e^{2(j-1)\pi i/N}$.

Lemma 2.8. [4, Corollary and Lemma 12] For the eigenvalues of $C$ with $j\neq N$ and $N\geq4$, $\lambda_{j}\neq0$ except that $\lambda_{(N+1)/2} = 0$ for odd $N$.

Lemma 2.9. [22, Proposition 2.2] The eigenvectors $\xi_{j}$ $(j = 1, 2, \ldots, N$ and $N\geq3)$ of circulant matrix $C$ form a basis of $\mathbb{C}^{N}$.

Lemma 2.10. [25, Page 65] Denote the conjugate transpose of $\nu_{k}$ by $(\bar{\nu}_{k})^{T}$. Then,

3.   Proof of Theorem 1.1

3.1.   To prove the necessity

Let $k^{\prime}\in\{1, 2, \ldots, N\}$ and $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$. By Definition 1.1, it suffices to study the following system:

Thanks to (1.2), (1.3), and (3.1), the $2N$+1 masses form a central configuration if and only if

By the assumption that the values of $N$ masses located at the vertices of one regular $N$-polygon are equal to each other, without loss of generality, we suppose that $m_{1} = m_{2} = \ldots = m_{N}: = m > 0$, and we divide the proof of the necessity into four steps.

Step 1. We prove that $a_{1} = 0$.

Employing $m_{1} = m_{2} = \ldots = m_{N} = m > 0$ and the second equation of (3.2), we have

where $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$. Combining Lemma 2.4, (3.3),

and that $\lambda$ is independent of the choice of $l^{\prime}$, we deduce that

Thus, for any $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$, we have $|a_{1}e^{i\alpha}-ae^{i(\theta_{l^{\prime}}+\theta)}|^{2}\equiv {\rm constant}$, i.e.,

Then, one computes that

Since $a$ represents the ratio of the sizes of the two regular $N$-polygons, $a > 0$. Hence, if $a_{1}\neq0$, then

In what follows, we assume that $a_{1}\neq0$, and we divide the proof of impossibility of $a_{1}\neq0$ into two cases: $N = 2$ and $N\geq3$.

(i) $N = 2$:

In this case, $l^{\prime}\in\{3, 4\}$, and $a_{1}\neq0$. Then, by (3.4), we have $\cos(3\pi+\theta-\alpha) = \cos(4\pi+\theta-\alpha)$, which implies that $\cos(\theta-\alpha) = 0$.

Under the assumption that $m_{1} = m_{2} = m$, we convert (1.2) and (1.3) into

First, in (2.3), for any $\theta\in[0, \, 2\pi)$ and any $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$ with $N\geq2$, if we let $\mu = k$, $\hat{\mu} = l^{\prime}-N$, $\tilde{\mu} = 0$, $\kappa = 0$, and $\tilde{\alpha} = -\theta\in(-2\pi, 0]$, then the mapping

is a bijection. Then, by the second equation of (3.2) and $m_{1} = m_{2} = \ldots = m_{N} = m > 0$, we have

Note that all of the sides in the two regular $N$-polygons have the same size, and $a$ represents the ratio of the sizes of the two regular $N$-polygons, so $a = 1$. Choosing $\theta_{l^{\prime}} = 4\pi$ with $l^{\prime} = 4$, and then employing (3.6) with $a = 1$ and $N = 2$, we have

Combining $N = 2$, (3.7) and the definition of circulant matrix $C$ in (2.14),

where

$M = (m_{3}, m_{4})^{T}$, $\tilde{\xi}_{1} = (1, 1)^{T}$, and $\tilde{\xi}_{2} = (\rho_{1}, \rho^{2}_{1})^{T} = (-1, 1)^{T}$. Thus, we have

Then, by $\rho_{-1} = \rho_{-1+2} = \rho_{1} = -1$, one computes that $\tilde{b}_{2} = (m_{4}-m_{3})/8$.

On the other hand, by (3.5), we have

In addition, according to lines 1-8 of page 109 of ^[7], we have $\lambda > 0$ for Definition 1.1. Combining $a\in\mathbb{R}$ and $a_{1}\neq0$, one computes that $Im(e^{i(\alpha-\theta)}) = 0$, i.e., $\sin(\alpha-\theta) = 0$, which contradicts with $\cos(\theta-\alpha) = 0$. Thus, $\cos(\theta-\alpha) = 0$ is impossible, which implies that for the spatial twisted central configuration with $N = 2$, we deduce the conclusion that $a_{1} = 0$.

(ii) $N\geq3$:

For (3.4), if we let $\beta = \theta-\alpha$ and choose $l^{\prime} = N+1, N+2$, and $N+3$, then

and this is equivalent to

Observing that $N\geq3$, $\sin(\pi/N)\neq0$, and $\sin(2\pi/N)\neq0$. Combining with (3.8), we have

which implies that $k_{1}\pi-3\pi/N = k_{2}\pi-4\pi/N$. So, $(k_{2}-k_{1})\pi = \pi/N$ where positive integer $N\geq3$, and this is impossible. Therefore, (3.4) does not hold. Then, for the spatial twisted central configuration with $N\geq3$, we arrive at the conclusion that $a_{1} = 0$, too.

Step 2. We prove that $\theta = s\pi/N$ with $s\in\{0, 1, \ldots, 2N-1\}$, and we divide the proof into two sub-steps.

Step 2.1. We show that $m_{N+1} = m_{N+2} = \ldots = m_{2N}$.

In fact, inserting $a = 1$ and $a_{1} = 0$ into (3.6), we have

Combining $N\geq2$, $m_{1} = m_{2} = \ldots = m_{N} = m$, (3.9), $\rho_{d} = e^{i\theta_{d}}$ with $\theta_{d} = 2 d\pi/N$ and $d\in \mathbb{Z}$, along with the definition of circulant matrix $C$ in (2.14),

where

$M = (m_{N+1}, m_{N+2}, \ldots, m_{2N})^{T}$, $\xi_{1} = (1, 1, \ldots, 1)^{T}$ and $\xi_{N} = (\rho_{N-1}, \rho^{2}_{N-1}, \ldots, \rho^{N}_{N-1})^{T}$.

In the following, we divide the proof of $m_{N+1} = m_{N+2} = \ldots = m_{2N}$ into three cases: $N = 2$, $N = 3$, and $N\geq4$.

(i) $N = 2$:

By (3.10) with $N = 2$,

Moreover, when $N = 2$, it is easy to see that $\rho_{1}+\rho_{2} = 0$ and $\rho_{-1} = \rho_{1} = -1$. Thus,

which implies that $b_{2} = (m_{4}-m_{3})/8$. Thus, when $N = 2$, then inserting $\rho_{3} = -1$, and $\rho_{4} = 1$ into (3.11) and combining with $b_{2} = (m_{4}-m_{3})/8$, we have

In what follows, we prove that for the spatial twisted central configuration with $N = 2$, $m_{3} = m_{4}$, and we prove it by contradiction. We assume $m_{3}\neq m_{4}$.

In fact, on the one hand, by $m_{3}\neq m_{4}$, $m_{1} = m_{2} = m$, and (3.12), we have

Moreover, thanks to $N = 2$, $a = 1$, $a_{1} = 0$, and the fourth equation of (3.5), one computes that

Summing the equations of the first part of (3.2) over $k^{\prime} = 1$ and $k^{\prime} = 2$, by $N = 2$, $a_{1} = 0$, $m_{1} = m_{2} = m$, (3.13), and (3.14), we have

Then, combining $e^{i\theta_{1}} = e^{i\theta_{-1}} = -1$ ($N = 2$), we have

i.e., $m_{3} = m_{4}$, and this contradicts with the assumption that $m_{3}\neq m_{4}$. Hence, for the spatial twisted central configuration with $N = 2$, we deduce that $m_{3} = m_{4}$.

(ii) $N = 3$:

By (3.10),

From $N = 3$, we have $\rho_{-1} = \rho_{2}$ and $\rho_{-2} = \rho_{1}$; thus, $\rho_{1}+\rho_{2}+\rho_{3} = 0$. Together with (3.15) and

there is

which implies that $b_{1}\in\mathbb{R}$.

On the other hand, for $N = 3$, Lemma 2.9 gives us information that there exist constants $c_{1}$, $c_{2}$, and $c_{3}$ such that $M = c_{1}\xi_{1}+c_{2}\xi_{2}+c_{3}\xi_{3}$ where $M = (m_{4}, m_{5}, m_{6})^{T}$. Thus, combining (3.10), we obtain

Then, it follows from (3.16) and Lemma 2.9 that $c_{1}\lambda_{1}(C)\xi_{1} = b_{1}\xi_{1}$ and $c_{3}\lambda_{3}(C)\xi_{3} = -b_{2}\xi_{3}$.

Employing Lemma 2.5, Lemma 2.7, $\rho_{3} = 1$, $\rho_{4} = \rho_{1}$, $\rho_{1}+\rho_{2} = -1$, and $|1-\rho_{1}| = |1-\rho_{2}|$, we have

Then, thanks to $b_{1}\in\mathbb{R}$, $\xi_{1} = (1, 1, \ldots, 1)^{T}$, and $c_{1}\lambda_{1}(C)\xi_{1} = b_{1}\xi_{1}$, one computes that $c_{1}\in\mathbb{R}$. In what follows, we will prove that $c_{2}\in\mathbb{R}$ and $c_{3}\in\mathbb{R}$.

By $\xi_{1} = (\rho_{0}, \rho_{0}, \rho_{0})$, $\xi_{2} = (\rho_{1}, \rho_{2}, \rho_{3})$, $\xi_{3} = (\rho_{2}, \rho_{1}, \rho_{3})$, and $M = c_{1}\xi_{1}+c_{2}\xi_{2}+c_{3}\xi_{3}$ with $N = 3$, we have

Based upon $\rho_{0} = \rho_{3} = 1$, $c_{1}\in\mathbb{R}$, and the third equation of (3.18), we have $c_{2}+c_{3}\in\mathbb{R}$.

Employing $\rho_{0} = 1$, $c_{1}\in\mathbb{R}$, and the first equation of (3.18), we see that $c_{2}\rho_{1}+c_{3}\rho_{2}\in\mathbb{R}$. Note that

and then $c_{2} = c_{3}$. Therefore, with the help of $c_{2}+c_{3}\in\mathbb{R}$, we have $c_{3}\in\mathbb{R}$.

In virtue of (3.17), $\lambda_{3}(C)\in\mathbb{R}$. Moreover, combining $c_{3}\in\mathbb{R}$ and $c_{3}\lambda_{3}(C)\xi_{3} = -b_{2}\xi_{3}$ where $\xi_{3} = (\rho_{2}, \rho_{4}, \rho_{6})^{T} = (\rho_{2}, \rho_{1}, \rho_{3})^{T}$, one computes that $b_{2}\in\mathbb{R}$.

By now, for (3.15), by the accumulated facts $b_{1}\in\mathbb{R}$, $b_{2}\in\mathbb{R}$, $|1-\rho_{1}| = |1-\rho_{2}|$, $\rho_{-1} = \rho_{2}$, $\rho_{-2} = \rho_{1}$, and $Im(\rho_{1}) = -Im(\rho_{2})$, we have $m_{4} = m_{5} = m_{6}$.

(iii) $N\geq4$:

Lemma 2.9 gives us information that there exist constants $\tilde{c}_{1}, \tilde{c}_{2}, \ldots, \tilde{c}_{N}$ such that $M = \tilde{c}_{1}\xi_{1}+\tilde{c}_{2}\xi_{2}+\ldots+\tilde{c}_{N}\xi_{N}$ where $M = (m_{N+1}, m_{N+2}, \ldots, m_{2N})^{T}$. We can regard (3.10) as (3.11) of ^[26]; moreover, we regard $C$, $b_{1}$, and $b_{2}$ of this paper as $A_{\alpha}$, $\sum_{k = 1}^{N}m_{k}$, and $\sum_{k = 1}^{N}m_{k}q_{k}$ of ^[26], respectively. Then, combining $N\geq4$ and Lemmas 2.8–2.10, similar to the procedure of Case 2.1 on pages 6–7 of ^[26], we obtain $m_{N+1} = m_{N+2} = \ldots = m_{2N}$.

Step 2.2. Based on Step 1 and Step 2.1, we prove that $\theta = s\pi/N$ with $s\in\{0, 1, \ldots, 2N-1\}$.

Inserting $m_{1} = m_{2} = \ldots = m_{N}$, $a_{1} = 0$, and $m_{N+1} = m_{N+2} = \ldots = m_{2N}$ into the second equality of (1.3), we have $c_{0} = 0$. Then, with the help of the first equation of (3.2), for any $k^{\prime}\in\{1, 2, \ldots, N\}$, we obtain

For any $k^{\prime}\in\{1, 2, \ldots, N\}$, it follows from Remark 2.2 that the mapping

where

is a bijection. Thus, by the procedure of obtaining (2.6), and $\theta_{d} = 2\pi d/N$ with $d\in \mathbb{Z}$, we have

and then

Combining (3.19) with (3.21), we have

For any $l\in\{N+1, N+2, \ldots, 2N\}$, in (2.5), if we let $\tilde{s} = 0$, then the mapping

is a bijection, too. Hence, we have

Using the definition of $f(\theta)$ in (2.1), (3.22), and the second equation of (3.23), we can see that

On the one hand, if $\theta\in(2\tilde{s}\pi/N, \, 2\tilde{s}\pi/N+\pi/N)$ where $\tilde{s}\in\{0, 1, \ldots, N-1\}$, then by Lemmas 2.1-2.2, there is $f(\theta) > 0$, which contradicts (3.24).

On the other hand, if $\theta\in(2\tilde{s}\pi/N+\pi/N, \, 2\tilde{s}\pi/N+2\pi/N)$ where $\tilde{s}\in\{0, 1, \ldots, N-1\}$, then by Lemma 2.1, we have $f(\theta) = -f(-\theta) = -f(-\theta+2\pi/N)$ and $-\theta+2\pi/N\in(-2\tilde{s}\pi/N, \, -2\tilde{s}\pi/N+\pi/N)$. Therefore, it follows from Lemma 2.2 that $f(\theta) < 0$, which also contradicts (3.24).

Thus, combining $\theta\in[0, \, 2\pi)$, we conclude that the twist angle must be $\theta = 2\tilde{s}\pi/N$ or $\theta = 2\tilde{s}\pi/N+\pi/N$ with $\tilde{s}\in\{0, 1, \ldots, N-1\}$, and then $\theta = s\pi/N$ with $s\in\{0, 1, \ldots, 2N-1\}$.

Step 3. We show that $m_{1} = m_{2} = \ldots = m_{N} = m_{N+1} = m_{N+2} = \ldots = m_{2N}$.

Based on the first part of Step 2, we can assume that $m_{N+1} = m_{N+2} = \ldots = m_{2N}: = bm$ where constant $b > 0$. By the assumption that $m_{1} = m_{2} = \ldots = m_{N}: = m$, it suffices to show that the value of $b$ can only take $b = 1$, and we prove it by contradiction. We assume that $b\neq1$.

Thanks to $a = 1$, $m_{1} = m_{2} = \ldots = m_{N}: = m$, $a_{1} = 0$, and $m_{N+1} = m_{N+2} = \ldots = m_{2N}: = bm$, for (3.2) we have

where $k^{\prime}\in\{1, 2, \ldots, N\}$ and $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$. Combining the first and second equations of (3.25) with (2.7),

Let

Thus, (3.26) can be simplified into

On the one hand, by (3.28), we see that

and

Then, it follows from (3.29), (3.30), and $b\neq1$ that

Thus,

Combining with

we have

which implies that $(1+b)h_{2N+1} = bh$. Moreover, with the help of (3.31), one computes that $h_{0} = h_{2N+1}$. Then, it follows from the second equation of (3.29) that $x = -by$. Hence, with the aid of (3.27) and $(1+b)h_{2N+1} = bh$, we have

that is, $b = \pm1$, which contradicts $b > 0$ and the assumption $b\neq1$. So, $b = 1$, and we arrive at the conclusion that $m_{1} = m_{2} = \ldots = m_{N} = m_{N+1} = m_{N+2} = \ldots = m_{2N}$.

Step 4. We prove that $h_{2N+1} = h/2$.

In virtue of (3.30), we have

Note that lines 1–8 of page 109 of ^[7] show us that in Definition 1.1, $\lambda$ must be a positive number. Then, combining the last equality of (1.3) and $m_{1} = m_{2} = \ldots = m_{N} = m_{N+1} = m_{N+2} = \ldots = m_{2N} = m$,

which implies that $N = 1$ or $h = 2h_{2N+1}$. Combining with $N\geq2$, for the spatial twisted central configuration, we have $h_{2N+1} = h/2$. □

Remark 3.1. The proof of Step 1 is independent of the condition that $a = 1$. That is, for the twisted central configuration of the ($2N$+1)-body problem with the assumption that $m_{1} = m_{2} = \ldots = m_{N}$, and without the assumption that $a = 1$, we have $a_{1} = 0$. That is, the ($2N$+1)-th mass must be in the vertical line of the two paralleled planes containing the two regular $N$-polygons, respectively, and the vertical line segment passes through the geometric centers of the two regular $N$-polygons.

3.2.   To prove the sufficiency

We divide the proof into two steps.

Step 1. Based on the assumptions that $\theta = s\pi/N$ with $s\in\{0, 1, \ldots, 2N-1\}$, $a = 1$, $h_{2N+1} = h/2$, $a_{1} = 0$, and $m_{1} = m_{2} = \ldots = m_{N} = m_{N+1} = m_{N+2} = \ldots = m_{2N} = m$, we show that if there exists a constant $\lambda\in\mathbb{R}$ such that

then the $2N$+1 masses can form a central configuration.

In fact, by (1.3), in this situation we get

Employing $a = 1$, $h_{2N+1} = h/2$, $a_{1} = 0$, $m_{1} = m_{2} = \ldots = m_{N} = m_{N+1} = m_{N+2} = \ldots = m_{2N} = m$, (3.33) and

we see that (3.2) holds if and only if

Therefore, it suffices to verify (3.34) holds. Clearly, (3.34) is equivalent to

On the other hand, for any $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$, it follows from Remark 2.2 that the mapping

where

is a bijection. Thus, by $\theta_{d} = 2\pi d/N$ with $d\in \mathbb{Z}$, we have

Together with (3.20), for any $k^{\prime}\in\{1, 2, \ldots, N\}$ and any $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$, it follows that

Employing Lemma 2.3, (3.35), and (3.36), we conclude that if there exists a constant $\lambda\in\mathbb{R}$ such that (3.32) holds, then by Definition 1.1, the $2N$+1 masses form a central configuration.

Step 2. We prove the existence of the spatial twisted central configuration, i.e., we prove the existence of $\lambda$ of Step 1.

Define the function $g$ as follows:

where $h > 0$ and $\theta = s\pi/N$ with $s\in\{0, 1, \ldots, 2N-1\}$. Thanks to Lemmas 2.3, 2.5, and (3.37), we see that $g(h)\in\mathbb{R}$, which implies that

In what follows, we prove that there exists $h = \bar{h}(N)$ such that $g(\bar{h}(N)) = 0$. Note that $\theta = s\pi/N$ with $s\in\{0, 1, \ldots, 2N-1\}$ is equivalent to $\theta = 2\tilde{s}\pi/N$ or $\theta = 2\tilde{s}\pi/N+\pi/N$ with $\tilde{s}\in\{0, 1, \ldots, N-1\}$. We divide the following proof into two cases.

Case 1. $\theta = 2\tilde{s}\pi/N$ with $\tilde{s}\in\{0, 1, \ldots, N-1\}$.

On the one hand, since $m > 0$, $\theta = 2\tilde{s}\pi/N$, and $1+\cos\theta_{j}\geq0$ with $j\in\mathbb{Z}$, we have

Moreover, if $h\rightarrow0^{+}$, then

which implies that when the twist angle is $\theta = 2\tilde{s}\pi/N$ with $\tilde{s}\in\{0, 1, \ldots, N-1\}$, there exists $h = h_{1}(N)$ such that $g(h_{1}(N)) < 0$.

On the other hand, notice that if $h\rightarrow +\infty$, then

Then, by (3.38)–(3.39), we see that when the twist angle is $\theta = 2\tilde{s}\pi/N$ with $\tilde{s}\in\{0, 1, \ldots, N-1\}$, there exists $h = h_{2}(N)$ such that $g(h_{2}(N)) > 0$.

Hence for the case of $\theta = 2\tilde{s}\pi/N$ with $\tilde{s}\in\{0, 1, \ldots, N-1\}$, employing the fact that $g$ is a continuous function, there exists $h = \bar{h}(N)$ such that $g(\bar{h}(N)) = 0$.

Case 2. $\theta = 2\tilde{s}\pi/N+\pi/N$ with $\tilde{s}\in\{0, 1, \ldots, N-1\}$.

In this case, by (3.38), $\tilde{s}\in\{0, 1, \ldots, N-1\}$, and $\theta_{d} = 2\pi d/N$ with $d\in \mathbb{Z}$, we have

Then, it follows from (3.40) and Lemma 2.5 that

If $h\rightarrow0$, then with the help of (3.41) and Lemma 2.6, $\lim_{h\rightarrow0}g(h) < 0$, which implies that when the twist angle is $\theta = 2\tilde{s}\pi/N+\pi/N$ with $\tilde{s}\in\{0, 1, \ldots, N-1\}$, there exists $h = h_{3}(N)$ such that $g(h_{3}(N)) < 0$.

On the other hand, if $h\rightarrow +\infty$, then

which implies that when the twist angle is $\theta = 2\tilde{s}\pi/N+\pi/N$ with $\tilde{s}\in\{0, 1, \ldots, N-1\}$, there exists $h = h_{4}(N)$ such that $g(h_{4}(N)) > 0$.

Therefore, for the case of $\theta = 2\tilde{s}\pi/N+\pi/N$ with $\tilde{s}\in\{0, 1, \ldots, N-1\}$, combining with the continuity of function $g$, there exists $h = \bar{h}(N)$ such that $g(\bar{h}(N)) = 0$.

By now, Cases 1–2 show us that when $\theta = s\pi/N$ with $s\in\{0, 1, \ldots, 2N-1\}$, there exists $h = \bar{h}(N)$ such that $g(\bar{h}(N)) = 0$, which implies that

Moreover, note that

Then, there is a constant $\lambda\in\mathbb{R}$ such that

i.e.,

which implies that there exists a constant $\lambda\in\mathbb{R}$ such that (3.32) holds. Then, by Step 1, the $2N$+1 masses form a central configuration. □

4.   Proof of Theorem 1.2

For the spatial twisted central configuration, (3.2) holds. Moreover, note that $m_{1} = m_{2} = \ldots = m_{N} = m$ and $a = 1$. Then, all the assumptions of Theorem 1.1 are satisfied, so we have $a_{1} = 0$, $h_{2N+1} = h/2$, and $m_{1} = m_{2} = \ldots = m_{N} = m_{N+1} = \ldots = m_{2N} = \, m$. Thus, $c_{0} = 0$, $h_{0} = h/2$, and $q_{2N+1} = (0+0i, h/2)$. Thus, in the following, it suffices to prove the uniqueness of $h$.

In fact, by (3.36) and the first equation of (3.2), for any $k^{\prime}\in\{1, 2, \ldots, N\}$ and any $l^{\prime}\in\{N+1, N+2, \ldots, 2N\}$, one computes that

where $h > 0$ and $\theta\in[0, 2\pi)$. Hence, it follows from Lemma 2.3 that

Let

Combining $\lambda\in\mathbb{R}$, $h\in\mathbb{R}$, (4.1), and (4.2), we have

which implies that

and then we obtain $\bar{x} = \bar{y}(h)+\bar{z}(h)$. Hence, if the $2N$+1 masses form a central configuration, then the distance $h$ must satisfy that $\bar{x} = \bar{y}(h)+\bar{z}(h)$. Next, we prove the uniqueness of the distance $h$.

We take $G(h) = \bar{y}(h)+\bar{z}(h)-\bar{x}$ for $h > 0$, and by (4.2), it is easy to verify that $G^{\prime}(h) < 0$. Moreover, note that $m_{1} = m_{2} = \ldots = m_{N} = m$ and $a = 1$. Then, employing Theorem 1.1, we obtain that the existence of the central configuration implies that $\theta = s\pi/N$ with $s\in\{0, 1, \ldots, 2N-1\}$ holds. Then, due to the rotational symmetry of the central configuration, in order to prove Theorem 1.2, it suffices to consider the following two cases: $\theta = 0$ and $\theta = \pi/N$.

Case 1. $\theta = 0$.

Lemma 2.5 shows that

and then by (4.2),

Employing (4.3), one verifies that there exist a small enough constant $h = \bar{h} > 0$ and a big enough constant $h = \tilde{h} > 0$ such that $G(\bar{h}) > 0$ and $G(\tilde{h}) < 0$. Then, combining the continuity and monotonicity of function $G$, for the case of $\theta = 0$, there is a unique $h = \hat{h} > 0$, such that $G(\hat{h}) = 0$, i.e., $\bar{x} = \bar{y}(\hat{h})+\bar{z}(\hat{h})$.

Case 2. $\theta = \pi/N$.

Employing (4.2), we have

By Lemma 2.6, $\lim_{h\rightarrow0}G(h) > 0$. Furthermore, by Lemma 2.5, we see that $\lim_{h\rightarrow +\infty}G(h) < 0$. Thus, for the case of $\theta = \pi/N$, due to the continuity and monotonicity of function $G$, there is a unique $h = \breve{h} > 0$, such that $G(\breve{h}) = 0$, i.e., $\bar{x} = \bar{y}(\breve{h})+\bar{z}(\breve{h})$.

Based upon Cases 1–2, there exists only one $h > 0$ such that $\bar{x} = \bar{y}(h)+\bar{z}(h)$, i.e., there is only one $h > 0$ such that the $2N$+1 masses form a central configuration. Moreover, combining the other two conclusions that $a_{1} = 0$ and $h_{2N+1} = h/2$, we obtain that $q_{2N+1} = (a_{1}e^{i\alpha}, h_{2N+1})$ is unique, i.e., there are only one positive distance $h$ between the two paralleled regular $N$-polygons and only one position $q_{2N+1}$ of the ($2N$+1)-th mass such that the $2N$+1 masses form a central configuration. □

Use of AI tools declaration

The authors declare they have not used Artificial Intelligence (AI) tools in the creation of this article.

Acknowledgments

The authors sincerely thank the referees and the editors for their valuable comments and suggestions, and the authors also express their sincere gratitude to professor Shiqing Zhang for his helpful discussions and suggestions. This work was supported by the National Natural Science Foundation of China (Grant Nos. 12361022 and 12371163), the China Postdoctoral Science Foundation (Grant No. 2021M700968), the Guizhou Provincial Basic Research Program (Natural Science, Grant Nos. ZK[2021]003 and ZK[2023]138), and the Natural Science Research Project of the Department of Education of Guizhou Province (Grant No. QJJ2022047).

Conflict of interest

The authors declare there is no conflict of interest.