A progressive capital income tax — a bad idea, or just a useless idea? Lessons for Bulgaria (1999-2018)

1.   Introduction and motivation

Taxing capital is an old and still a controversial issue among economists, and the literature is inconclusive whether it should be taxed, and how exactly it should be taxed.^* We are not going to argue whether capital income should be taxed or not; instead, we take it as an empirical regularity across the globe. What is novel in this paper is the pursuit of the question whether taxing capital income should be done in a progressive- or a proportional manner, especially in a developing country context. On the one hand, it is believed that capital taxation is the most distortionary tax, as it affects capital accumulation and investment decisions. On the other hand, it can be argued that a cyclical capital income tax, and a progressive capital income tax in particular, may act as a built-in stabilizer for the economy, in the same way a progressive labor income tax could by decreasing demand during expansions, and by increasing demand during downturns.^†

^* For a survey of recent discussion, see Bastani and Wladenstrom (2020), Fehr and Kindermann (2015), Conesa et al. (2009), and the references therein.

^† For a survey of different tax reforms in Bulgaria and their effect on the business cycle, the reader is referred to Vasilev (2017a), Vasilev (2015a, 2015b), Di Nola et al. (2019), as well as the references therein.

This proposal is taken seriously, and this paper incorporates a progressive capital income tax schedule in an RBC model with government. We take an agnostic view and let a computational experiment guide us, given that there is no other way, consistent with theory, to conduct a disciplined counterfactual examination. The micro-founded model based in optimization is calibrated for Bulgaria in the period 1999–2018, as Bulgaria makes a good example for the application of the theory.^‡ The paper then proceeds to quantitatively evaluate the effect of such a progressive capital income tax as a tool for business cycle stabilization, and the implications for public finances. This study is one of the few quantitative ones on the issue of tax reforms in emerging Eastern European economies, which utilizes modern macroeconomic analysis, and thus aims to bring novelty to the literature on transition economies. Unfortunately, for reasonable degree of capital income tax progressivity, the quantitative effects in the calibrated model are miniscule. Therefore, we conclude that such a tax is neither a good instrument for agammaegate demand management over the cycle, nor is it an appropriate measure for raising additional tax revenue in Bulgaria.^§,¶

^‡ Before the introduction of proportional income taxation of 10 percent in 2008, Bulgaria operated a progressive income taxation regime during the period 1993–2007 with the same effective rate. In addition, the corporate income tax rate has been reduced, in several steps, to a proportional rate of 10 percent in 2007 as well, to avoid incentives to move earnings across the income categories.

^§ It is important to note that the results in this paper should be interpreted with respect to the intrinsic characteristics of the Bulgarian economy, and caution should be applied before simply "extending" the result to other economies. However, similar quantitative results were obtained for a proposed introduction of a progressive consumption tax in Vasilev (2020).

^¶ Vasilev (2016, 2017b) show that progressive income taxes, when levied on both capital and labor, produce indeterminacy and multiplicity of equilibria in the system.

The rest of the paper is as follows: Section 2 outlines the model setup and derives the decentralized competitive equilibrium system, Section 3 presents the calibration procedure, and Section 4 documents the steady-state model solution. Sections 5 focuses on the out-of-steady-state dynamics of model variables, and compares the simulated moments of model variables against the empirical ones. Section 6 concludes the paper.

2.   Model description

There is a stand-in household in the model, which derives utility out of consumption and leisure. The time endowment of the household can be spent working, or enjoyed as leisure. The government taxes final private consumption and income in order to finance its expenditure. Lastly, there is a stand-in firm, which rents labor and capital from the household to produce the final good.

2.1.   Representative household

There is a stand-in household, which maximizes an expected utility function of the form

where $E_{0}$ is the household's expectations as of period 0, $c_{t}$ is the household's private consumption in period $t$, $h_{t}$ are the hours worked in period $t$, $0 < \beta < 1$ denotes the discount factor, $0 < \gamma < 1$ is the relative weight attached to leisure.

The household starts with an initial quantity of physical capital $k_{0} > 0$, and chooses how much to grow it. Physical capital evolves as follows:

and $0 < \delta < 1$ denotes the linear depreciation rate on capital. The real interest rate is denoted by $r_t$, hence the before-tax capital income of the household in period $t$ is expressed as $r_{t}k_{t}$. In addition to capital income, the household can obtain labor income. Hours supplied to the stand-in firm are remunerated at the hourly wage rate of $w_t$, thus pre-tax labor income can be expressed as $w_{t}h_{t}$. Lastly, the household owns the firm in the economy and all of its profit, denoted by $\pi_t$.

The household's problem is thus to

s.t.

where $\tau^{c}$ is the tax on consumption, $\tau^l$ is the proportional income tax rate on labor ($0 < \tau^{c}, \tau^{l} < 1$), $\tau_{t}^{k}$ is the progressive rate applied to capital income, and $g_{t}^{t}$ denotes government transfers. The household takes all tax rates $\{\tau^{c}, \tau^{l}, \tau_{t}^{k}\}_{t = 0}^{\infty}$, government spending (consumption and transfers) categories, $\{g_{t}^{c}, g_{t}^{t}\}_{t = 0}^{\infty}$, profit $\{\pi_{t} \}_{t = 0}^{\infty}$, prices $\{w_{t}, r_{t} \}_{t = 0}^{\infty}$, and chooses $\{c_{t}, h_{t}, k_{t+1} \}_{t = 0}^{\infty}$ to maximize its utility subject to the budget constraint.

The progressivity of the capital tax schedule in this paper is captured by adopting the functional form of Guo and Lansing (1998), later also utilized in Vasilev (2016, 2017b) for the progressivity of the income tax schedule, as a function of capital income (expressed relative to the steady-state capital income):

where $\eta > 0$ is the effective average capital tax rate, and $0 < \phi < 1$ captures the degree of progressivity of the capital tax.

The first-order optimality conditions as as follows:

where $\lambda_{t}$ denotes period-$t$ Lagrangian multiplier. The intuition obtained from the optimality conditions above is as follows: the household balances the utility from consumption at the margin, with the shadow price of spending, including the consumption tax rate. Next, when choosing labor hours, the gain from working balances with the disutility cost. In the third equation, the household allocates physical capital over time optimally, taking into consideration the progressivity of capital taxation. The last condition is called the "transversality condition" (TVC): it states that the value of physical capital should be zero at the boundary of the optimization horizon.

2.2.   Firm problem

There is a stand-in firm in the economy, which produces the final output, with output price normalized to unity. The production technology is Cobb-Douglas and uses both physical capital, $k_t$, and hours, $h_t$, to maximize profit

where $A_{t}$ is the level of technology in period $t$. In equilibrium, there is no profit, and

2.3.   Government

In the model in this paper, the government taxes income and consumption, in order to finance its spending. The government budget constraint is as follows:

2.4.   Dynamic competitive equilibrium (DCE)

For a given process for technology $\{A_{t}\}_{t = 0}^{\infty}$, the tax schedules $\{\tau^{c}, \tau^{l}, \tau_{t}^{k}\}_{t = 0}^{\infty}$, and initial capital endowment $\{k_{0}\}$, the DCE for this economy is a list of allocations $\{c_{t}, i_{t}, k_{t}, h_{t}\}_{t = 0}^{\infty}$ for the household, a pair of public consumption and transfers allocations for the government $\{g_{t}^{c}, g_{t}^{t}\}_{t = 0}^{\infty}$, and prices $\{w_{t}, r_{t}\}_{t = 0}^{\infty}$ such that (i) the stand-in household maximizes its expected utility function s.t. its budget constraint; (ii) the stand-in firm maximizes its profit; (iii) the government runs a balanced budget; (iv) all markets clear.

3.   Data and model calibration

To study business cycle fluctuations in Bulgaria, we will focus on the period 1999–2018. Quarterly data on output, consumption and investment was extracted from National Statistical Institute (2020) database, while the real interest rate was taken from Bulgarian National Bank Statistical Database (2020). The calibration strategy is as follows: first, as in Vasilev (2016), the discount factor is set to match the steady-state capital-to-output ratio in Bulgaria, $k/y = 13.964$. Next, the labor share parameter, $1-\alpha = 0.571$, is set equal to the average value of the wage bill in agammaegate output. Next, $\tau^{l} = \tau^{k} = \eta = 0.1$, which is the average effective tax rate on income between 1999–2007, when Bulgaria used progressive income taxation, and equal to the proportional income tax rate introduced as of 2008. Similarly, $\tau^{c} = 0.2$. The degree of capital tax progressivity was set equal to $\phi = 0.43$.^||

^|| This corresponds to the degree of progressivity of the labor income tax schedule during the period 1993–2007 in Bulgaria. Robustness checks with different (higher and lower) values did not change the results in any major way.

Next, $\gamma$, is calibrated to match the steady-state hours worked, $h = 1/3$. This is in line with the estimates for Bulgaria (Vasilev, 2017a) as well over the period studied. Next, $\delta = 0.013$, was estimated as the average quarterly depreciation rate. Finally, the process followed by the TFP process is estimated from the detrended Solow residuals. Table 1 below presents the values of all model parameters used in the paper.

4.   Steady-State

Once the values of model parameters were obtained, the steady-state equilibrium system solved, the "big ratios" can be compared to their averages in Bulgarian data. The results are reported in Table 2 below. The model matches the major ratios in data quite well, which is amazing for such a parsimonious model.

5.   Out of steady-state model dynamics

The model does not possess an analytical solution for the general case; in order to study the equilibrium behavior of variables outside their steady-state values, we need to solve the model numerically. This is done by linearizing the equilibrium system around the steady-state. Then, we investigate the behavior of major model variables to a one-time technology shock; then, we proceed to simulate the model fully.

5.1.   Impulse Response Analysis

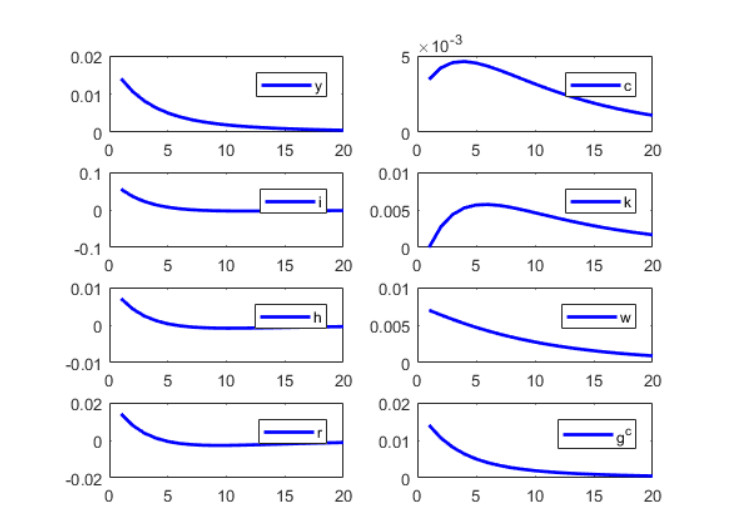
This subsection discusses the impulse responses of model variables to a 1$\%$ shock to technology, presented in Figure 1 and Figure 2 for the progressive- and proportional ("flat") capital taxation case, respectively. In both cases, output increases upon impact, as do the uses of output—consumption, investment, and government consumption. Interestingly, the impulse responses across the two tax regimes are almost identical.^** Next, the increase in productivity increases the after-tax return on labor and capital, so the household works and saves more. The increase in capital and labor inputs feed back in output through the production function and further expand output.

^** We present them on separate figures for better inspection; otherwise impulse responses across the two regimes would be on top of each other.

Over time, as capital is being accumulated, the diminishing marginal returns enter the picture, and its after-tax marginal product starts to decrease; this lowers the households' incentives to save, and physical capital stock slowly returns to its steady-state, following a hump-shaped dynamics. The rest of the model variables return to their old steady-states monotonically.

5.2.   Simulation and moment-matching

We will now simulate the model 10, 000 times, and then detrend both the empirical and model simulated data using the Hodrick-Prescott (1997) filter. Table 3 on the next page summarizes the second moments of data (relative volatilities to output, and contemporaneous correlations with output) versus the same moments computed from the model-simulated data at quarterly frequency. The "Model" is the case with the progressive capital taxation schedule, while the "Benchmark RBC" is a setup with proportional capital income tax rate. In addition, to minimize the sample error, the simulated moments are averaged out over the computer-generated draws. Both models overpredict the consumption and investment volatilies. Still, the setups are at least qualitatively consistent with the stylized fact for Bulgaria that consumption is smoother than output, while investment is almost twice as volatile than output. The model with progressive capital taxation produces a bit less variable consumption- and investment series, but the two models are almost identical to each other.

With respect to the labor market dimension, the predicted employment variability is lower than the empirical one; still, model wages vary as much as in data. This is yet another confirmation that perfectly-competitive labor markets are not very good description of the situation in Bulgaria. Next, the model over-predicts the pro-cyclicality of the major variables; This, however, is a common limitation of such RBC models: in addition, the contemporaneous correlation of employment with output is too low, and the setup generates highly-procyclical wages, while in data those acyclical.

Next, we proceed to investigate the dynamic properties model variables. In particular, we discuss the auto-(ACFs) and cross-correlation functions (CCFs) of the major model variables, thus evaluating how the model captures the phase dynamics with output. The coefficients of the empirical functions are presented in Table 4 and compared to the averaged simulated AFCs and CCFs from the model with progressive capital income taxation.

Overall, the model compares relatively well vis-a-vis data, even though the empirical ACFs for output and investment are slightly outside the confidence band predicted by the model; The persistence of the major labor market variables is also relatively well-captured by the model dynamics. Next, as seen from Table 5 below, over the business cycle, in data labor productivity leads employment. The model, however, cannot account for this fact, which is again an artifact of the perfectly-competitive labor market assumption.

6.   Conclusions

Progressive capital income taxation is introduced into an otherwise standard RBC model with government. The artificial economy is calibrated to Bulgarian data for the period 1999–2018. The quantitative role of progressive taxation on capital income is investigated for the stabilization of cyclical fluctuations in Bulgaria. Unfortunately, the quantitative effect of such a tax is found to be very small, and thus not important for either business cycle stabilization, or public finance issues. However, it is important to note that the results in the paper should be interpreted with respect to the intrinsic characteristics of the Bulgarian economy, and caution should be applied before simply extending the result to other economies, and/or using it as a policy advice in an arbitrary developing-economy context.

Acknowledgments

We would like to thank the Editor, and two referees for their helpful comments.

Conflict of interest

The author declares no conflicts of interest in this paper.